Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


to
the
inclusion
map
rk:
c-cs
1
)
~
L
2
k(B
1
)
and
moreover
ind(P<k>'rk) e
K(c-(B
1
))
is
independent
of
k
and
hence
defines
an
element
i
8
(P) e
K(c-(B
1
))
called
the
analytic
index
of
P.
For
more
detailed
definitions
of
topological
and
analytic
index
of
operators,
the
index
theorem
for
manifolds
with
boundary
and
other
applications
of
the
index
theorem,
see
articles
in
Palais
[1965].
This
chapter,
in
particular,
discusses
the
subjects
which
are
not
extensively
utilized
in
the
subsequent
chapters,
at
least
not
explicitly.
Nonetheless,
some
of
the
concepts
and
even
terminology
do
find
their
way
to
our
later
discussions.
This
chapter
is
included,
and
indeed
is
lectured,
to
prepare
the
students
with
some
concepts
and
understanding
about
global
analysis
in
general,
and
some
techniques
important
to
the
global
theory
of
dynamical
systems.
In
particular,
the
reader
may
find
it
useful
when
they
venture
to
theoretically
oriented
research
literature.
We
have
reviewed
a
broad
range
of
mathematical
concepts
and
techniques
useful
for
our
discussion,
we
can
now
turn
to
our
main
interest,
namely,
the
nonlinear
dynamical
systems
and
their
structural
stability.
144

Chapter
4
General
Theory
of
Dynamical
systems
4.1
Introduction
In
order
to
place
our
discussion
of
dynamical
systems
in
proper
perspective,
let
us
discuss
briefly
three
aspects
of
the
theory
of
dynamical
systems.
For
lack
of
a
better
description,
we
shall
refer
to
them
as
the
local,
the
global,
and
the
abstract
theories.
The
local
theory
is
concerned
with
the
application
of
geometrical
and
topological
methods
to
the
qualitative
study
of
differential
equations.
The
general
setting
is
a
set
of
differential
equations
in
R"
and
one
is
interested
in
asking:
"What
does
the
omega-limit
set
look
like?":
"What
happens
in
the
neighborhood
of
a
fixed
point?":
"Is
it
stable?",
etc.
The
object
for
the
study
of
the
global
theory
is
the
set
of
vector
fields
on
a
manifold.
One
is
interested
in
characterizing
the
structurally
stable
vector
fields
and
in
studying
the
"orbit
picture"
of
the
flow
associated
with
a
given
vector
field.
The
setting
of
the
abstract
theory
is
a
general
transformation
group
but
the
notions
studied
are
those
arising
in
the
qualititive
study
of
differential
equations.
One
can
show
that
many
of
the
results
for
differential
equations
are
valid
in
a
much
broader
domain.
In
current
literature,
the
abstract
theory
is
known
as
topological
dynamics,
the
global
theory
is
known
as
smooth
dynamical
systems,
and
the
local
theory
is
known
as
qualitative
theory
of
diffferential
equations.
Topological
dynamics
deals
with
continuous
actions
of
any
topological
group
G
on
a
topological
space
X.
Smooth
dynamical
systems
are
smooth
actions
of
the
group
R
or
z
on
a
differentiable
manifold
M.
We
shall
begin
by
illustrating
a
few
fundamental
definitions
with
some
simple
examples.
Most
of
these
definitions
and
examples
are
also
common
to
the
qualitative
theory
of
differential
equations.
Indeed,
the
latter
theory
provides
the
proper
intuition
and
phenomena
for
the
145

development
of
dynamical
systems.
Let
G
be
either
the
additive
topological
group
R
of
real
numbers
or
the
additive
topological
group
Z
of
integers.
A
dynamical
system
on
a
topological
space
X
is
a
continuous
map
~=
G x X
~
X
such
that
for
all
x £ X,
for
all
g,
h £ G,
~(g+h,x)
=
~(g,
~(h,x)),
and
~(O,x)
=
x.
(4.1-1)
The
space
X
is
called
the
phase
space
of
~.
If
X
is
a
differentiable
manifold
and
~
is
a cr
map,
r
~
o,
then
we
call
~
a cr
dynamical
system.
For
instance,
for
any
X
the
trivial
dynamical
system
is
defined
by
~(t,x)
=
x.
For
X =
R·,
~(t,x)
=
etx
defines
a
cw
dynamical
system
on
X.
Let
~
be
a
dynamical
system
on
X.
Given
t £ G, we
define
the
partial
map
~t:
X~
X
by
~t(x)
=
~(t,x).
If
G = R,
~tis
sometimes
called
the
time
map
of
0.
Likewise,
given
x £
X,
we
define
the
partial
map
~x=
G
~X
by
~x(t)
=
~(t,x).
Note
that
if~
is
cr,
then
so
are
~t
and
~x·
Then
Eq.(4.1-1)
can
be
written
as
~g+h
=
~g~h,
and
~o
=
id.
(4.1-2)
Sometimes
for
brevity
we
denote
~(t,x)
by
t·x,
when
under
the
context
there
is
no
confusion.
With
this
convention,
Eqs.
(4.1-1)
and
(4.1-2)
become:
(g
+
h)·x
=
g·
(h·x),
and
o·x
=
x.
(4.1-3)
Proposition
4.1.1
For
all
t £ G,
~t
is
a
homeomorphism.
If
~
is
cr
then
~
is
a cr
diffeomorphism.
Exercise
Prove
this
by
the
definition
of
homeomorphism
and
diffeomorphism.
Note
that,
if
G =
R,
then
the
dynamical
system
~
is
called
a
flow
on
X,
or
an
one-parameter
group
of
homeomorphism
of
X.
Let
~
be
a
dynamical
system
on
X.
We
define
a
relation
-
on
X
by
putting
x - y
iff
there
exists
t £ G
such
that
~t(x)
=
y.
Proposition
4.1.2
The
relation
-
is
an
equivalence
relation.
The
equivalence
classes
of
-
are
called
orbits
of
~
(or
of
the
homeomorphism~·
in
the
case
G =
Z).
For
each
x
£X,
the
equivalence
class
containing
x
is
called
the
orbit
146

throuah
x.
It
is
the
image
of
the
partial
map
~x=
G
~
X.
We
sometimes
denote
it
by
G·x.
Thus
Prop.
4.1.2
implies
that
two
orbits
either
coincide
or
are
disjoint.
We
denote
the
quotient
space
X/-
by
X/~
and
call
it
the
orbit
space
of
~
The
quotient
map,
which
takes
x
to
its
equivalence
class,
is
denoted
by
y-:
X
~
X/~
or
just
y:
X
~
X/~-
As
usual,
we
give
X/~
the
finest
topology
with
respect
to
which
y
is
continuous.
(That
is,
a
subset
U
of
X/~
is
open
in
X/~
iff
y"
1
(U)
is
open
in
X).
Now
let
us
look
at
some
examples
of
dynamical
systems.
Earlier
we
have
pointed
out
that
if
G = R,
the
trivial
dynamical
system
on
any
X
is
the
point
x E
X,
and
the
orbit
is
the
set
of
single
element
{x}.
For
the
nontrivial
flow
t·x
=
etx,
there
are
three
orbits,
namely,
the
origin,
the
positive
and
negative
half
lines.
0
The
arrows
on
the
orbits
indicate
the
orientations
induced
by
the
flow.
If,
however,
for
all
t,x
E R we
put
t·x
x +
t,
then
this
flow
has
only
one
orbit,
R
itself.
For
any
6 E
R,
put
t·
[X]
= [X +
6t]
where
[X]
is
a
fixed
value
of
s
1
•
If
6 = o, we
have
the
trivial
flow
on
S
1
,
otherwise
we
have
the
single
orbit
s
1
•
If
we
imbed
s
1
in
the
plane
by
the
standard
imbedding
[x]
~
(cos
2~x,
sin
2~x),
then
the
rotation
is
counter-clockwise
if
6
is
positive,
and
clockwise
if
6
is
negative.
We
call
6
the
angular
speed
of
the
flow.
For
flows
on
R
2
,
it
is
sometimes
more
convenient
to
identify
R
2
with
the
complex
line
C
because
the
two
are
topologically
indistinguishable.
The
simple
non-trivial
flow
is
for
all
t E R,
and
for
all
(x,y)
E R
2
,
put
t·
(x,y)
(xet,yet).
The
origin
is
the
only
point
orbit,
and
all
other
orbits
are
open
rays
radiating
from
the
origin.
Fig.4.1-1(a).
If
we
change
the
formula
slightly
tot·
(x,y)
=
(xet,ye-t),
the
phase
portrait
is
radically
changed,
this
is
because
the
new
flow
has
only
two
orbits
beginning
at
the
147
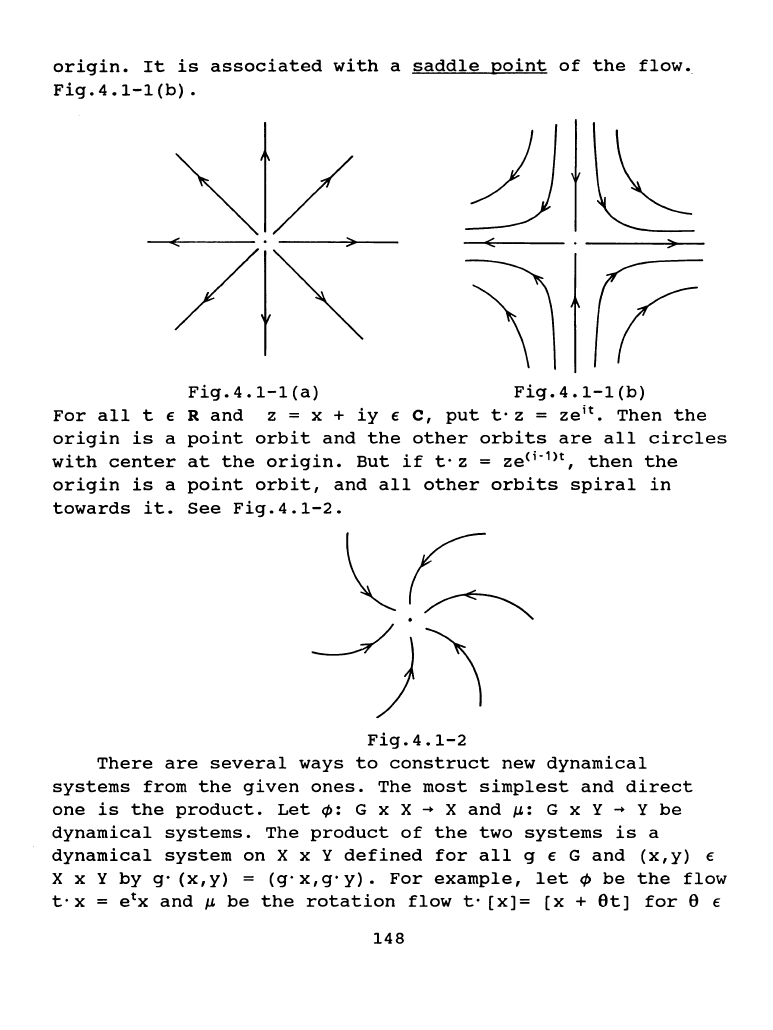
or1g1n.
It
is
associated
with
a
saddle
point
of
the
flow.
Fig.4.1-l(b).
Fig.4.1-l(a)
Fig.4.1-l(b)
For
all
t e
Rand
z = x +
iy
E
c,
put
t·z
=zeit.
Then
the
origin
is
a
point
orbit
and
the
other
orbits
are
all
circles
with
center
at
the
origin.
But
if
t·
z =
ze<i·l>t,
then
the
origin
is
a
point
orbit,
and
all
other
orbits
spiral
in
towards
it.
See
Fig.4.1-2.
Fig.4.1-2
There
are
several
ways
to
construct
new
dynamical
systems
from
the
given
ones.
The
most
simplest
and
direct
one
is
the
product.
Let
~:
G x X
~
X
and
~=
G x Y
~
Y
be
dynamical
systems.
The
product
of
the
two
systems
is
a
dynamical
system
on
X x Y
defined
for
all
g E G
and
(x,y)
E
X x Y
by
g·
(x,y)
=
(g·x,g·y).
For
example,
let~
be
the
flow
t·x
=
etx
and~
be
the
rotation
flow
t·
(X]=
(X+
6t]
for
6 E
148

R.
Then
~
x
~
is
a
flow
on
the
circular
cylinder
R x s
1
•
The
circle
{o}xS
1
is
an
orbit,
and
all
the
other
orbits
spiral
away
from
it.
0
,
sl
/
0
Fig.4.1-3
,
,
,
Let
~:
R x X
~
X
be
any
flow.
For
any
t e
R,
~t:
X
~
X
is
a
homeomorphism
which
generates
a
discrete
dynamical
system
~=
z x X
~
X,
then
we
say
~t
(or
~)
is
imbedded
in
~.
For
instance,
any
rotation
of
s
1
is
imbedded
in
any
non-trivial
rotation
flow
on
s
1
•
It
is
not
true
that
every
homeomorphism
of
every
topological
space
X
can
be
imbedded
in
a
flow
on
X.
In
general,
every
flow
on
X
yields
a
homeomorphism
of
X
(in
fact,
many
homeomorphisms),
but
the
reverse
is
not
usually
true.
Nonetheless,
we
can
associate
with
a
given
homeomorphism
f a
flow
with
similar
properties
provided
we
allow
the
flow
to
be
on
a
larger
spaceY.
Thus
we
have
the
space
X
imbedded
in
Y
and
the
homeomorphism
of
X
imbedded
in
a
flow~
on
Y.
The
way
to
construct
the
larger
space
Y
and
the
flow
~
is
known
as
suspension.
It
should
be
noted
that
this
is
different
from
the
suspension
discussed
in
algebraic
topology
(see,
for
instance,
Bourgin
[1963);
Greenberg
[1967);
Eilenberg
and
Steenrod
[1952]).
We
shall
not
get
into
this
any
further,
except
to
say
that
when
reading
the
literature,
be
aware
of
the
difference
of
meaning
of
terms.
It
is
fairly
easy
to
distinguish
them
under
very
different
context.
Let
f:
X
~
X
be
a
homeomorphism
generating
a
discrete
dynamical
system
~·
Let
-
be
the
equivalence
relation
defined
on
R x X
by
(u,x)
-
(v,y)
iff
u = v + m
for
some
m e
149

Z
andy=
fm(x).
Then
there
is
a
flow~=
R x Y
~Yon
Y
-=
(R
x
X)/-
defined
by
~(t,[u,x))
=
[u+t,x],
where
[u,x)
denotes
the
equivalence
class
of
(u,x)
e R x X.
The
flow
~
is
called
the
suspension
of
the
homeomorphism
f
(or
of~).
Clearly,
for
any
u e
R,
the
restriction
of
~
to
any
cross-section
[u,X)
with
the
obvious
identification
[u,X)
= X
coincides
with
f.
If
f:
R
~
R
is
defined
by
f(x)
=
-x,
then
the
suspension
is
a
flow
on
the
open
Mobius
band,
and
all
its
orbits
are
topologically
circles.
We
shall
discuss
rational
and
irrational
flows
on
a
torus
T
2
= s
1
x s
1
and
we
shall
show
that
all
rational
flows
are
essentially
the
same,
but
there
are
infinitely
many
different
types
of
irrational
flow.
It
should
also
be
pointed
out
here
that
the
phase
portrait
of
a
product
flow
is
not
uniquely
determined
by
the
phase
portraits
of
its
factors.
This
is
because
the
phase
portraits
of
rational
and
irrational
flows
are
completely
different
topologically,
but
they
both
come
from
factors
with
identical
phase
portraits.
We
shall
discuss
this
point
in
detail
later.
Let
~:
G x X
~
X
be
a
dynamical
system
on
X,
let
a:
G
~
G
be
a
continuous
automorphism
of
the
additive
group
G,
and
let
h:
X~
Y
be
a
homeomorphism.
Then
~=
G x Y
~
Y,
~
h·~·
(axh)"
1
,
is
a
dynamical
system
on
Y.
Then~
is
the
dynamical
system
induced
from
m
by
the
pair
(a.h)
(or
by
h,
if
a=
id).
The
simplest
example
is
that
if
h =
id:
X
~
X,
and
a
-id:
R
~
R,
we
then
obtain~-,
the
reverse
flow
of~'
by
150

~-
1
(t,x)
=
~(-t,x).
That
is,
in~-,
points
moving
along
the
orbits
of
~
at
the
same
speed
but
in
opposite
direction.
The
construction
of
induced
systems
is
not
particularly
interesting,
but
the
generalization
to
quotient
systems
does
produce
new
systems.
Let
~:
G x X
~
X
be
a
dynamical
system
on
X
and
let
-
be
an
equivalence
relation
on
X
such
that
for
all
t € G
and
all
x,
y € X,
~(t,x)
-
~(t,y)
iff
x -
y.
Then
~
induces
a
dynamical
system
~.
called
the
quotient
system
on
the
quotient
space
X/-
by
~(t,[x])
=
[~(t,x)]
where
t €
Rand
[x]
is
the
equivalence
class
of
x € X.
Iff
and
g
are
commuting
homeomorphisms
of
X,
i.e.,
fg
gf,
then
f
takes
orbits
of
g
onto
orbits
of
g,
thus
induces
a
homeomorphism
of
the
orbit
space
of
g.
An
example
of
a
quotient
system
~.
which
is
the
discrete
dynamical
system
generated
by
f
and
-
is
the
equivalence
relation
giving
orbits
of
g
as
equivalence
classes.
Similarly,
if
~
and
~
are
commuting
flow
on
X,
i.e.,
~s~t
=
~t~s
for
all
s,
t €
R,
then
~
induces
a
quotient
flow
on
the
orbit
space
X/~-
Let
us
define
an
equivalence
relation
-
on
R"
by
x - y
iff
x-
y e
z".
Then
the
quotient
space
R"/-
is
the
n-dim
torus
T" = s
1
xs
1
x
..•
xs
1
,
the
cartesian
product
of
n
copies
of
the
circle
s
1
•
Let
f
be
a
linear
automorphism
of
R"
whose
matrix
A,
with
respect
to
the
standard
basis
of
R",
is
in
GLn(Z).
That
is,
A
has
integer
entries
and
detA
=
±1.
Then
f
maps
z"
onto
itself
and
thus
f
and
f"
1
preserve
the
equivalence
relation.
Thus
f
induces
a
homeomorphism
(in
fact,
a
diffeomorphism)
of
T".
The
induced
homeomorphism
of
T"
is
called
a
hyperbolic
toral
automorphism.
For
any
hyperbolic
toral
automorphism
g:
T"
~
T",
its
periodic
and
non-periodic
point
sets
are
dense
in
T".
Note
that,
for
a
point
x €
T"
which
is
periodic
if
g•(x)
= x
for
some
r >
0.
We
shall
see
that
hyperbolic
toral
automorphisms
are
the
simplest
examples
of
Anosov
diffeomorphisms
on
compact
manifolds.
Originally,
the
hyperbolic
toral
automorphisms
were
counter-examples
to
the
conjecture
that
structurally
stable
diffeomorphisms
have
finite
periodic
sets.
151

Theorem
4.1.3
The
periodic
set
of
the
hyperbolic
toral
automorphism
g:
T"
-+ T"
is
precisely
Q"/-,
where
Q
is
the
rational
numbers.
Remark:
One
can
verify
that
induced
and
quotient
systems
are
dynamical
systems.
Let
¢
be
a
dynamical
system
on
a
topological
space
X.
For
each
x e X,
the
subset
Gx
=
{g
e G,
¢(g,x)
=
x}
is
a
subgroup
of
G
and
is
called
an
isotropy
subgroup
Cor
stabilizer)
of
x
Cor
of¢
at
x>.
Proposition
4.1.4
If
X
is
a T
1
space,
then
for
all
x e
X,
Gx
is
a
closed
subgroup
of
G.
Proposition
4.1.5
If
X
is
T
2
and
G/Gx
is
compact,
then
the
orbit
G·x
is
homeomorphic
to
G/Gx.
Proposition
4.1.6
Every
orbit
of
every
flow
is
connected.
The
definitions
and
propositions
in
this
section
are
common
to
the
theory
of
dynamical
systems
in
general.
The
next
section
will
introduce
various
equivalence
relations
and
conjugacy,
which
are
essential
to
the
introduction
of
limiting
sets.
4.2
Equivalence
relations
To
classify
dynamical
systems
is
one
of
the
center
themes
and
is
of
special
interest
to
the
subject.
One
begins
by
placing
certain
equivalence
relations
upon
the
set
of
all
dynamical
systems.
Such
equivalence
relations
should
be
natural
in
the
sense
that
the
systems
have
qualitative
resemblance.
Equipped
with
the
equivalence
relation,
one
can
form
the
equivalence
classes
and
be
able
to
distinguish
them
by
means
of
algebraic
or
topological
invariants
(quantities
that
are
associated
with
all
systems
and
are
equal
for
all
systems
in
the
same
equivalence
class).
A
good
classification
scheme
requires
a
careful
choice
of
"intrinsic"
equivalence
relations
with
tractable
invariants.
We
shall
consider
several
"obvious"
equivalence
relations.
In
order
to
appreciate
the
difficulties
involved
in
the
152

classification
problem,
one
only
has
to
look
at
the
situation
when
the
manifold
is
the
circle
s
1
•
See,
for
instance,
(Irwin
1980,
Section
2-II;
Nitecki
1971,
Chapter
1].
Let
f:
X
~
X,
g:
Y
~
Y
be
homeomorphisms
of
topological
spaces
X
andY.
A
topological
conjugacy
from
f
to
g
is
a
homeomorphism
h:
X~
Y
such
that
h·f
=
g·h.
That
is,
the
following
diagram
commutes:
x~x
h
~
y
-
g
~
h
y
If
such
a
homeomorphism
h
exists,
then
the
homeomorphisms
f
and
g
are
said
to
be
topologically
conjugate.
Clearly,
topological
conjugacy
is
an
equivalence
relation.
It
is
easy
to
show
that
a
topological
conjugacy
maps
orbits
onto
orbits,
periodic
points
to
periodic
points,
and
it
also
preserves
periods.
In
our
discussion,
we
are
mainly
concerned
with
differentiable
manifolds
M
and
N,
and
f
and
g
are
diffeomorphisms.
It
seems
natural
to
require
the
map
h
to
be
a
diffeomorphism.
This
gives
the
notion
of
differentiable
conjugacy.
It
is
a
stronger
relation
than
topological
conjugacy,
and
in
general,
there
are
many
more
equivalence
classes
with
respect
to
it.
Nonetheless,
with
differentiable
conjugacy,
we
do
find
stable
diffeomorphisms,
ones
which
stay
in
the
same
equivalence
class
when
slightly
perturbed,
which
are
very
rare.
Moreover,
we
also
have
to
classify
as
non-equivalent
diffeomorphisms
which
most
people
would
feel
are
qualitatively
the
same
such
as
the
contractions
x
~
xj2,
and
x
~
x/3
of
the
real
line.
For
these
reasons,
topological
conjugacy
remains
as
the
basic
equivalence
relation
even
when
we
are
dealing
with
a
differentiable
category.
Let
~
and
~
be
flows
on
topological
spaces
X
and
Y
respectively.
h:
X
~
Y
is
a
flow
map
from
~
to
u
if
it
is
continuous
and
if
there
exists
an
increasing
continuous
153
