Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.

homomorphism
a:
R
~
R
such
that
the
diagram
R X X
axh
.(.
R X Y
commutes.
Recall
that
a
is
just
multiplication
by
a
positive
constant.
If
h
is
a
homeomorphism,
we
then
call
(a,h)
or
h,
in
the
case
a =
id,
a
flow
equivalence
from
0
to
u.
We
say
that
m
is
flow
equivalent
to
u
if
such
a
pair
of
(a,h)
exists.
In
this
case,
~
is
the
flow
induced
on
Y
from
¢
by
(a,
h)
•
The
definition
of
flow
equivalence
seems
to
be
very
natural.
But
it
is
rather
too
strong
for
the
qualitative
theory
of
flows.
For
instance,
it
preserves
the
ratios
of
periods
of
closed
orbits,
but
flows
may
differ
in
this
respact
and
yet
have
a
very
similar
appearance.
We
now
define
a
weaker
equivalence
relation
which
is
regarded
as
a
basic
notion.
We
call
h:
X
~
Y a
topological
equivalence
from
0
to
u
if
it
is
a
homeomorphism,
which
maps
each
orbit
of
¢
onto
an
orbit
of
~.
and
it
preserves
the
orientation
of
orbits.
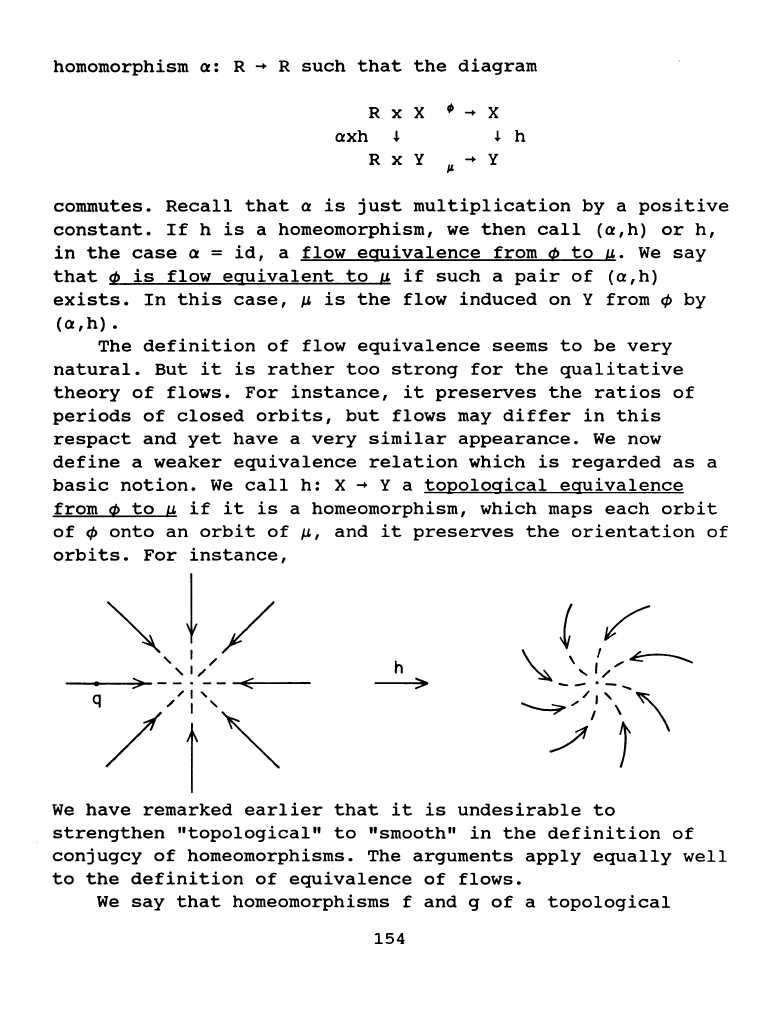
For
instance,
~t/
' I "
'I"
q
'I'
/'t'~
h
~
We
have
remarked
earlier
that
it
is
undesirable
to
strengthen
"topological"
to
"smooth"
in
the
definition
of
conjugcy
of
homeomorphisms.
The
arguments
apply
equally
well
to
the
definition
of
equivalence
of
flows.
We
say
that
homeomorphisms
f
and
g
of
a
topological
154

space
X
are
topologically
equivalent
if
there
is
a
homeomorphism
h
of
X
which
maps
orbits
of
f
onto
orbits
of
g.
This
relation
is
different
from
topological
conjugacy,
but
oftenly
they
are
the
same.
One
can
also
show
that
flow
equivalence
implies
topological
equivalence
and
both
are
equivalence
relations.
In
some
situations,
we
need
to
deal
with
topological
conjugacy,
topological
equivalence
and
flow
equivalence
in
a
local
form.
It
is
often
possible
to
modify
the
definitions
of
such
relations
by
paraphrasing
them
as
"in
some
neighborhood
of
a
given
point".
Even
if
the
"local"
definition
does
not
make
perfect
sense,
it
often
points
toward
a
sensible
concept.
Let
U
and
V
are
open
subsets
of
X
and
Y
respectively
and
let
f:
X
~
X
and
g:
Y
~
Y
be
homeomorphisms.
Somewhat
abusing
the
notation,
we
say
that
flu
is
topologically
conjugate
to
glv
if
there
is
a
homeomorphism
h:
U u
f(U)
~
v
u
g(V)
such
that
h(U)
= V
and,
for
all
x E
u,
hf(x)
=
gh(x).
If
p e X
and
g e Y, we
say
that
f
is
topologically
conjugate
at
p
to
g
at
g
if
there
exists
open
neighborhood
U
of
p
and
v
of
q
such
that
flU
is
topologically
conjugate
to
glv
and
by
a
conjugacy
h
which
takes
p
to
q.
Let
~:
D
~
X
be
a
continuous
map
where
X
is
a
topological
space
and
D
is
a
neighborhood
of
{0}
x X
in
R x
X.
We
write
t·x,
as
before,
for
~(t,x)
and
Dx
=
{t
E R:
(t,x)
e
D}.
We
say
that
is
a
partial
flow
on
X
if,
for
all
x
E
X,
(i)
Dx
is
an
interval,
(ii)
o·x
= x
(iii)
for
all
t E
Dx
with
s E
Dt·x'
(s+t)
· x =
s·
(t·
X),
(iv)
for
all
t E Dx, Dt·x =
{s-t
: s E
Dx}.
That
is,
~
is
a
flow
not
defined
for
all
time.
(iv)
implies
~
is
maximal
and
it
cannot
be
extended.
Proposition
4.2.1
Let
~:
D
~
X
be
a
partial
flow
on
X.
Then
(i)
D
is
open
in
R x X,
(ii)
if
Dx
=
(a,b)
with
b <
~
,
~cannot
be
extended
to
a
continuous
map
of
D U
{(b,x)}
into
X,
(iii)
if
x
is
a
fixed
point
or
periodic
point
of
~'
then
155

Dx
=
R.
It
is
clear
that
flow
equivalence
and
topological
equivalence
for
partial
flows
can
be
defined
in
a
straightforward
way.
If
~
is
a
partial
flow
on
a
topological
space
Y,
then
a
flow
equivalence
from
0
to
u
is
a
pair
(a,h)
where
h:
X
~
Y
is
a
homeomorphism,
a: R
~
R
is
a
multiplication
by
a
positive
constant,
and
h(t·x)
=a(t)·h(x)
for
all
(t,x)
E D.
And
a
topological
equivalence
from
0
to
u
is
a
homeomorphism
h:
X~
Y
that
maps
all
orbits
Dx·x
of¢
onto
orbits
of
~
and
preserves
their
orientation.
Proposition
4.2.2
Flow
equivalence
and
topological
equivalence
are
equivalence
relations
on
the
set
of
all
partial
flow
on
topological
spaces.
Let
¢
be
any
flow
(or
partial
flow)
on
X
and
let
U
be
an
open
subset
of
X.
Once
again,
we
are
abusing
the
notation
and
defining
a
map
¢IU:
D
~
U
by
(¢IU)
(t,x)
=
¢(t,x)
where
D
=
ux,uDxx{x}
of
R x
U,
and
call
¢I
U
the
restriction
of
the
flow
0
to
the
subset
u.
Notice
again,
this
is
an
abuse
of
notation,
since
U
is
not
even
in
the
domain
of
¢.
One
can
easily
check
that
¢IU
is
a
partial
flow
on
u.
If
~
is
a
flow
on
a
topological
space
Y
and
V
is
an
open
subspace
of
Y,
then
we
say
that
¢IU
is
flow
or
topologically
equilvalent
to
ulY
if
they
are
equivalent
as
partial
flows.
If
p E X
and
g
E Y,
we
say
that
¢
is
equivalent
at
p
to
~
at
q,
if
there
exists
open
neighborhoods
U
of
p
and
V
of
q
and
an
equivalence
from
¢IU
to
~IV
taking
p
to
q.
Proposition
4.2.3
Flow
equivalence
and
topological
equivalence
are
equivalent
relations
on
{(¢,p):
¢is
a
flow,
p E
phase
space
of¢}.
If
¢IP
is
flow
equivalent
to
~lq,
then
¢IP
is
topologically
equivalent
to
~lq.
4.3
Limit
sets
and
non-wandering
sets
Let¢
and~
be
flows
on
topological
spaces
X
andY.
Suppose
h
is
a
topological
equivalence
from
¢
to
~,
then
h
maps
the
closure
f
in
X
of
each
orbit
r
of
¢
onto
the
closure
h(I1
of
h(r)
in
Y.
Thus,
h
maps
the
set
f;r
onto
the
156

set
h(r)/h(r).
We
want
to
study
the
orbit
with
very
large
positive
and
negative
t.
Let
It=
[t,~).
Then-set
n(x)
of
x
eX
with
respect
to
the
flow
cp
is
defined
by
n(x)
=
nt£R
ct>x<It).
Intuitively,
n(x)
is
the
subset
of
X
that
t·x
approaches
as
t
~
~.
It
follows
from
the
definition
of
flow
that
for
all
t
e
R,
n(t,x)
=
n(x).
Thus
we
may
define
then-set,
n(r)
of
any
orbit
r
of
cp
by
n(r)
=
n(x)
for
any
x e
r.
Note
that
if
r
is
a
fixed
point
or
periodic
orbit
then
cpx(It) = r
for
all
x e r
and
t e R,
and
n(r)
=
r.
Thus
n(r)
is
not
necessarily
part
of
f/r.
Similarly,
the
a-set
of
a
point
x e X
is
defined
by
a(x)
=
ntER
cpx(Jt),
where
Jt
=
(-
~,t],
and
the
a-set
a(r)
of
'an
orbit
r
is
denoted
by
a(r)
=
a(x)
for
any
x
e
r.
Again,
intuitively,
the
a-set
is
the
subset
that
t·x
approaches
as
t
~
-~.
Since
the
n-set
and
a-set
are
''symmetric"
in
time,
we
shall
confine
our
attention
to
n-set,
and
the
corresponding
results
for
a-sets
are
exactly
analogous.
Let
a
vector
field
X e
X(M)
and
let
cp
be
the
flow
of
x.
The
orbit
of
X
through
p e M
is
the
set
r(p)
=
{cp(t,p)
I t e
R, p
eM}.
If
X(p) = o,
the
orbit
reduces
top,
then
we
say
that
p
is
a
singularity
of
X.
Otherwise,
the
map
a:
R
~
M,
a(t)
=
cp(t,p)
is
an
immersion.
If
a
is
not
injective,
there
exists
p > o
such
that
a(P)
=
a(O)
= p
and
a(t)
+ p
for
0 <
t < p.
In
this
case,
the
orbit
of
p
is
diffeomorphic
to
s
1
and
we
say
that
it
is
a
closed
orbit
with
period
p.
If
the
orbit
is
not
singular
or
closed,
it
is
called
regular.
Thus
a
regular
orbit
is
the
image
of
an
injective
immersion
of
the
line.
The
n-limit
set
of
p e
M,
n(p)
=
{q
e
M,
for
which
there
exists
a
sequence
tn
~~with
cp(tn,p)
~
q}.
Simiarly,
we
define
the
a-limit
set
of
p,
a(p)
=
{q
e
Ml
cp(tn,p)
~
q
for
some
sequence
tn
~
-~
}.
Thus,
a-limit
of
p
for
the
vector
field
X
is
n-limit
of
p
for
the
vector
field
-X.
Also,
n(p)
=
n(p')
if
p'
belongs
to
the
orbit
of
p.
Intuitively,
a(p)
is
where
the
orbit
of
p
is
"born"
and
n(p)
is
where
it
"dies".
157
Example
1.
Let
us
consider
the
system
of
differential
equations
defined
in
R
2
(in
polar
coordinates):
dr/dt
=
r(1-
r),
d8/dt
=
1.
It
can
easily
be
shown
that
the
solutions
are
unique
and
all
solutions
are
defined
on
R
2
•
Thus,
the
system
defines
a
dynamical
system.
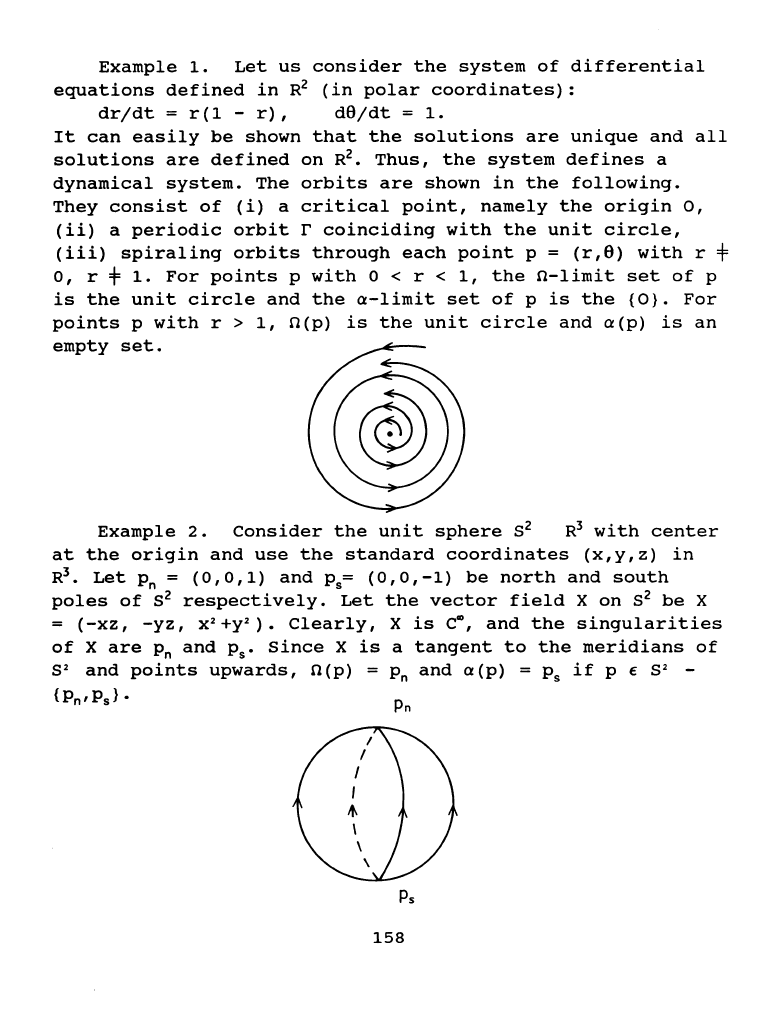
The
orbits
are
shown
in
the
following.
They
consist
of
(i)
a
critical
point,
namely
the
origin
o,
(ii)
a
periodic
orbit
r
coinciding
with
the
unit
circle,
(iii)
spiraling
orbits
through
each
point
p =
(r,8)
with
r +
o, r +
1.
For
points
p
with
0 < r <
1,
the
n-limit
set
of
p
is
the
unit
circle
and
the
a-limit
set
of
pis
the
{0}.
For
points
p
with
r >
1,
O(p)
is
the
unit
circle
and
a(p)
is
an
empty
set.
Example
2.
Consider
the
unit
sphere
s
2
R
3
with
center
at
the
origin
and
use
the
standard
coordinates
(x,y,z)
in
R
3
•
Let
Pn
=
(0,0,1)
and
p
8
=
(0,0,-1)
be
north
and
south
poles
of
s
2
respectively.
Let
the
vector
field
X
on
s
2
be
X
=
(-xz,
-yz,
x
2
+y
2
).
Clearly,
X
is~'
and
the
singularities
of
X
are
Pn
and
p
8
•
Since
X
is
a
tangent
to
the
meridians
of
s•
and
points
upwards,
n(p)
=
Pn
and
a(p)
= p
8
if
p £
s•
-
Pn
Ps
158
Theorem
4.3.1
(Poincare-Bendixson)
Let
X e
X(S
2
)
with
a
finite
number
of
singularities,
and
let
p e
s•
and
n(p)
be
the
n-
limit
set
of
p.
Then
one
of
the
following
possibilities
holds:
(i)
n(p)
is
a
singularity,
(ii)
n(p)
is
a
closed
orbit,
(iii)
n(p)
consists
of
singularities
p
1
,
•••
,pn
and
regular
orbits
such
that
if
r c
n(p),
then
a(r)
=
P;,
and
n(r)
=
Pr
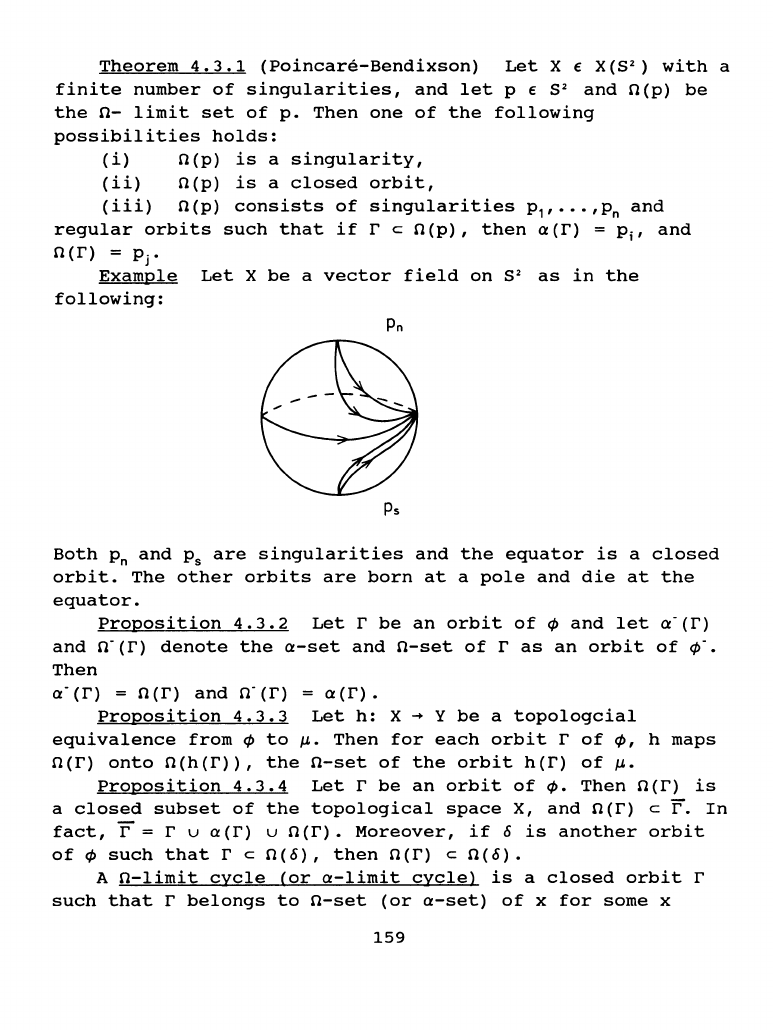
Example
Let
X
be
a
vector
field
on
S
2
as
in
the
following:
Pn
Ps
Both
Pn
and
p
8
are
singularities
and
the
equator
is
a
closed
orbit.
The
other
orbits
are
born
at
a
pole
and
die
at
the
equator.
Proposition
4.3.2
Let
r
be
an
orbit
of~
and
let
a-(r)
and
n-(r)
denote
the
a-set
and
n-set
of
r
as
an
orbit
of~-.
Then
a-
(r)
= n
(r)
and
n-
(r)
= a
(r)
•
Proposition
4.3.3
Let
h:
X~
Y
be
a
topologcial
equivalence
from
~
to
~·
Then
for
each
orbit
r
of
~,
h
maps
n(r)
onto
n(h(r)),
then-set
of
the
orbit
h(r)
of~·
Proposition
4.3.4
Let
r
be
an
orbit
of
~.
Then
n(r)
is
a
closed
subset
of
the
topological
space
X,
and
n(r)
c
r.
In
fact,
F=
r u
a(r)
u
n(r).
Moreover,
if
6
is
another
orbit
of~
such
that
r c
n(o),
then
n(r)
c
n(o).
A
n-limit
cycle
Cor
a-limit
cvclel
is
a
closed
orbit
r
such
that
r
belongs
to
n-set
(or
a-set)
of
x
for
some
x
159

which
does
not
belong
to
r.
Note
that,
limit
cycles
·enjoy
certain
properties
not
shared
by
other
closed
orbits,
namely,
if
r
is
an
n-limit
cycle,
then
there
exists
x
not
on
r
such
that
limt_
d(~t(x),r)
= o.
Geometrically,
this
means
that
some
trajectory
spirals
toward
r
as
t
~
~.
For
an
a-limit
cycle,
replace
~
by
-~.
We
list
some
other
results
of
limit
cycles
for
future
reference.
(A)
Let
r
be
ann-limit
cycle.
If
r =
n(x),
xis
not
on
r,
then
x
has
a
neighborhood
V
such
that
r =
n(y)
for
all
y e
V.
In
other
words,
the
set
{YI
r =
n(y)}-
r
is
open.
(B) A
nonempty
compact
set
K
that
is
positively
or
negatively
invariant
contains
either
a
limit
cycle
or
an
equilibrium.
(C)
Let
r
be
a
closed
orbit
and
suppose
that
the
domain
w
of
the
dynamical
system
includes
the
whole
open
region
U
enclosed
by
r.
Then
u
contains
either
an
equilibrium
or
a
limit
cycle.
Indeed,
one
can
state
a much
sharper
one.
(D)
Let
r
be
a
closed
orbit
enclosing
an
open
set
u
contained
in
the
domain
W
of
the
dynamical
system.
Then
U
contains
an
equilibrium.
(E)
Let
H
be
a
first
integral
of
a
planar
c
1
dynamical
system.
If
H
is
not
constant
on
any
open
set,
then
there
are
no
limit
cycle.
Any
union
of
orbits
of
a
dynamical
system
is
called
an
invariant
set
of
the
system.
We
then
have
the
following:
Proposition
4.3.5
Any
n-set
of
~
is
an
invariant
set
of
~-
Consequently,
if
n(r)
is
a
single
point
p,
then
p
is
a
fixed
point
of
~-
We
may
have
noticed
in
linear
examples
that
an
orbit
may
have
an
empty
n-set,
and
this
seems
to
be
associated
with
the
orbit
"going
to
infinity".
Thus,
it
is
reasonable
to
suppose
that
if
we
introduce
some
compactness
conditions,
we
can
ensure
non-
emptiness
of
the
n-sets.
Indeed,
we
have
the
following
propositions:
Proposition
4.3.6
If
X
is
compact
and
Hausdorff,
then,
160

for
any
orbit
r,
n(r)
is
non-empty,
compact
and
connected.
A
complimentary
statement
is
the
following
theorem:
If
X
is
locally
compact,
then
a
n-limit
set
is
connected
whenever
it
is
compact.
Furthermore,
whenever
a
n-limit
set
is
not
compact,
then
none
of
its
components
is
compact.
We
have
noticed
earlier
that
if
r
is
a
fixed
point
or
a
periodic
orbit,
then
n(r)
=
r.
In
fact,
one
can
show
that
under
very
general
conditions,
some
r
are
periodic.
We
now
want
to
investigate
this
relation
more
closely.
Proposition
4.3.7
Let
X
be
compact
and
Hausdorff,
then
an
orbit
r
of
~
is
closed
in
X
iff
n(r)
=
r.
(That
is,
closed
orbits
are
periodic
or
fixed
points).
Theorem
4.3.8
Let
X
be
Hausdorff.
Then
an
orbit
r
of
~
is
compact
iff
it
is
a
fixed
point
or
a
periodic
orbit.
Corollarv
4.3.9
Let
X
be
compact
and
Hausdorff,
then
the
following
three
statements
on
an
orbit
r
of
~
are
equivalent:
(i)
r
is
a
closed
subset
of
X,
(ii)
r
is
a
fixed
point
or
a
periodic
orbit,
(iii)
n(r)
r.
This
Corollary
can
be
generalized
to
non-compact
but
locally
compact
X.
A
minimal
set
of
a
dynamical
system
is
a
non-empty,
closed
invariant
set
that
does
not
contain
any
closed
invariant
proper
subset.
By
using
the
Zorn's
lemma,
one
can
prove
that
if,
for
any
orbit
r
of
~.
r
is
compact,
then
it
contains
a
minimal
set.
For
discrete
dynamical
systems,
the
theory
of
a-
and
n-limit
sets
can
also
be
developed
similarly.
If
f
is
a
homeomorphism
of
a
topological
space
X,
then
the
n-set
O(x)
of
x
eX
with
respect
to
f
is
defined
by
O(x)
=
nn,N
{fr(x):
r
~
n}.
Again,
the
a-set
a(x)
of
xis
then-set
of
x
with
respect
to
f-
1
•
All
results
of
a-
and
n-sets
have
analogues
form
except
that
n-sets
of
homeomorphisms
need
not
be
connected.
When
classification
of
dynamical
systems
becomes
difficult,
there
are
new
equivalence
relations
with
respect
161
to
which
classification
can
be
made
easier.
One
of
the
most
important
concepts
is
concerned
with
an
invariant
set
called
the
non-wandering
set
which
was
defined
by
Birkhoff
[1927].
The
intuitive
idea
is
the
following.
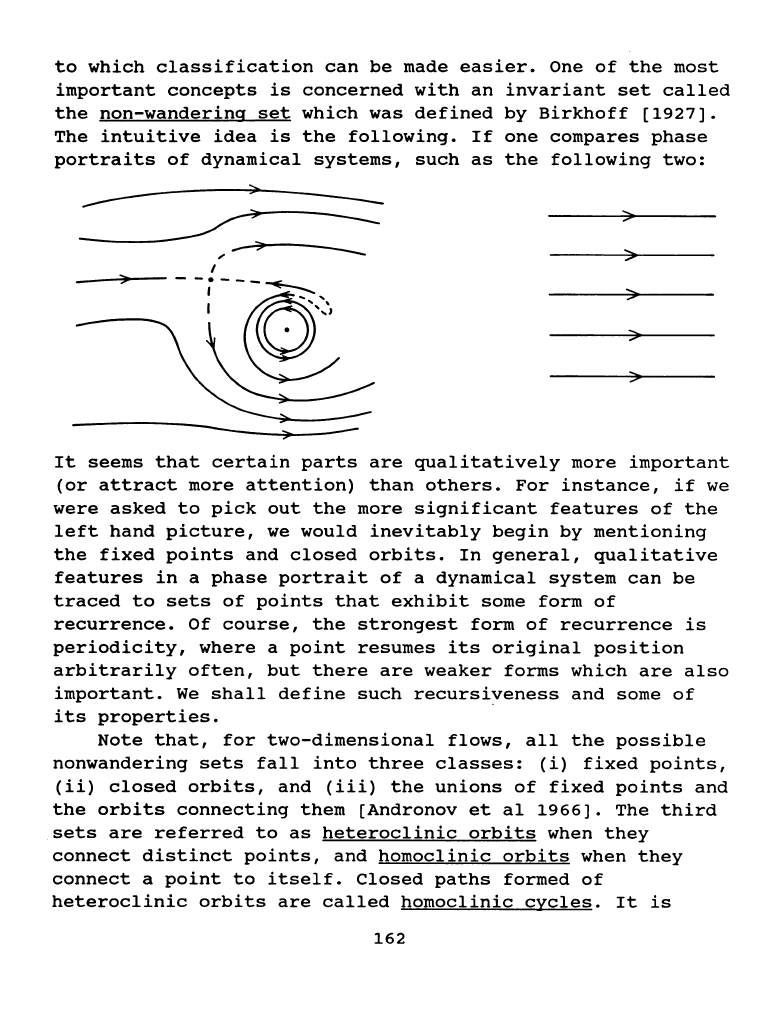
If
one
compares
phase
portraits
of
dynamical
systems,
such
as
the
following
two:
----
>
-
It
seems
that
certain
parts
are
qualitatively
more
important
(or
attract
more
attention)
than
others.
For
instance,
if
we
were
asked
to
pick
out
the
more
significant
features
of
the
left
hand
picture,
we
would
inevitably
begin
by
mentioning
the
fixed
points
and
closed
orbits.
In
general,
qualitative
features
in
a
phase
portrait
of
a
dynamical
system
can
be
traced
to
sets
of
points
that
exhibit
some
form
of
recurrence.
Of
course,
the
strongest
form
of
recurrence
is
periodicity,
where
a
point
resumes
its
original
position
arbitrarily
often,
but
there
are
weaker
forms
which
are
also
important.
We
shall
define
such
recursiveness
and
some
of
its
properties.
Note
that,
for
two-dimensional
flows,
all
the
possible
nonwandering
sets
fall
into
three
classes:
(i)
fixed
points,
(ii)
closed
orbits,
and
(iii)
the
unions
of
fixed
points
and
the
orbits
connecting
them
[Andronov
et
al
1966].
The
third
sets
are
referred
to
as
heteroclinic
orbits
when
they
connect
distinct
points,
and
homoclinic
orbits
when
they
connect
a
point
to
itself.
Closed
paths
formed
of
heteroclinic
orbits
are
called
homoclinic
cycles.
It
is
162

worthwhile
to
note
that
the
fixed
points
contained
in
such
cycles
must
all
be
saddle
points,
if
they
are
hyperbolic,
this
is
because
sinks
and
sources
necessarily
have
wandering
points
in
their
neighborhoods.
A
set
A c X
is
said
to
be
positively
recursive
with
respect
to
a
set
B c X
if
for
T E R
there
is
a t > T
and
an
x E B
such
that
t·x
EA.
Negative
recursiveness
can
be
defined
by
using
t <
T.
A
set
A
is
self
positively
recursive
if
it
is
positively
recursive
with
respect
to
itself.
A
point
x E X
is
positively
Poisson
stable
if
every
neighborhood
of
x
is
positively
recursive
with
respect
to
{X}
•
Theorem
4.3.10
Let
x E
X.
The
following
statements
are
equivalent:
(i)
x
is
positively
Poisson
stable,
(ii)
given
a
neighborhood
U
of
x
and
aT>
o,
t·x
E u
for
some t >
T,
(iii)
x e
n(x),
Civ>
r•cx> =
ncx>,
where
r•cx> =
<ct>Ct,x>
I o s t <
a},
(v)
r(x)
c
n(x),
(vi)
for
every
E > o,
there
is
at~
1
such
that
t·x
E
B(x,e),
where
B(x,e)
is
an
open
ball
centered
at
x
with
radius
e.
Note
also
that
if
x
is
positively
Poisson
stable,
then
so
is
t·x
for
every
t E R.
The
following
alternative
definition
of
Poisson
stability
is
customary
in
the
literature
and
is
clearly
suggested
by
the
above
theorem.
A
point
x E X
is
positively
or
negatively
Poisson
stable
whenever
x E
n(x)
or
x E
a(x)
respectively.
It
is
Poisson
stable
if
it
is
both
positively
and
negatively
Poisson
stable.
Theorem
4.3.11
r•(x)
=
n(x)
iff
s
is
a
periodic
point.
Remark:
Note
that,
if
r•(x)
=
n(x)
then
the
point
X
is
indeed
Poisson
stable.
The
following
example
shows
that
there
exist
points
which
are
Poisson
stable
but
not
periodic.
Example:
Consider
a
dynamical
system
defined
on
a
torus
163
