Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


a)
•
Theorem
2.3.3
If
X
is
pathwise
connected,
the
group
~,(X,x
0
)
is
independent
of
x
0
,
up
to
isomorphism.
It
is
denoted
by
~,(X)
-
the
fundamental
group
of
X.
A
space
X
is
simply-connected
if
it
is
pathwise
connected
and
~,(X)
= 0
(i.e.,
any
closed
loop
in
X
can
be
shrunk
to
a
point).
Theorem
2.3.4
~,(s
1
)
=
z,
~,cs")
= o, n
~
2.
Proposition
2.3.5
~,ex
x
Y)
=~,(X)
x
~,(Y).
In
Section
2.2
we
have
defined
covering
and
subcovering
to
define
the
concept
of
compact
space.
Here
we
shall
briefly
discuss
the
concept
of
covering
space
and
its
relation
to
homotopy.
E
P~
X
is
a
covering
space
of
X
if
every
x E X
has
an
open
neighborhood
U
such
that
p"
1
(U)
is
a
disjoint
union
of
open
sets
S;
in
E,
each
of
which
is
mapped
homeomorphically
onto
U
by
p.
S;
are
called
sheets
over
U.
If
X
has
a
covering
space
X-
~
X
such
that
X-
is
simply-connected,
then
X-
is
unique
up
to
equivalence,
and
it
is
called
the
universal
covering
space
of
X.
Theorem
2.3.6
Every
connected
space
(manifold)
has
a
universal
covering
space
(manifold).
Remark:
We
shall
discuss
the
covering
manifold
later.
Examples:
(i)
50(3),
group
of
rotations
of
R
3
,
~,(50(3))
Z/2.
The
universal
covering
space
of
50(3)
is
s
3
•
(ii)
Proper
Lorantz
group
Lt
~
p3
x R
3
its
universal
covering
group
is
S L (
2,
C)
~
s
3
x R
3
•
Higher
homotopy
groups
are
commutative
(~
1
(X)
may
not).
In
fact:
Theorem
2.3.7
(Hurewicz)
If
n
~
2
and
~q(X)=
0
for
all
q <
n,
then
~q(X)
~
Hq(X)
for
all
q S
n.
Theorem
2.3.8
If
X
is
pathwise
connected,
then
8 :
~,(X)
~
H
1
(X)
is
an
isomorphism
iff
~,(X)
is
commutative.
In
algebraic
topology,
in
order
to
calculate
a
particular
group
G,
one
often
proceeds
by
first
finding
an
exact
sequence
with
G
in
it,
then
evaluating
all
the
easier
groups
in
the
sequence
neighboring
G,
then
determining
G
by
44

using
the
properties
of
an
exact
sequence.
In
the
next
section,
we
shall
briefly
discuss
some
of
the
properties
of
a
differentiable
manifold
and
some
geometric
structures.
2.4
Elementary
differential
topology
and
differential
geometry
A
topological
manifold
of
dimension
m
is
a
topological
space
M,
(we
are
going
to
define
manifolds,
so
instead
of
denoting
the
topological
space
by
X, we
shall
use
M,
N,
...
to
denote
manifolds),
which
satisfies:
(i)
M
is
a
Hausdorff
space;
(ii)
if
p f
M,
then
there
is
an
open
set
U c
M,
p f U
such
that
U
is
homeomorphic
to
Rm;
(iii)
M
has
a
countable
basis
for
its
topology,
i.e.,
there
is
a
countable
family
of
open
sets
{Ua}
such
that
every
open
set
is
a
union
of
some
of
the
Ua's.
A
topological
manifold
M
of
dimension
m
has
some
set-
theoretical
and
topological
properties,
such
as:
(i)
M
is
locally
compact.
That
is,
if
p f
M,
any
open
neighborhood
U
of
p
which
is
homeomorphic
to
Rm,
then
centered
at
p
in
U we
may
choose
an
open
ball
of
finite
radius.
The
closure
of
such
an
open
ball
is
of
course
compact.
(ii)
(iii)
(iv)
(v)
M
is
separable.
M
is
regular.
M
is
a
normal
space
(Kelley
1955].
With
the
help
of
the
Urysohn's
metrization
theorem,
one
can
show
that
M
is
a
metric
space
(Kelley
1955].
A T
2
space
X
is
called
paracompact
if
for
each
covering
{Ua}a•A
of
X,
there
exists
a
locally
finite
covering
{V.s>.s.s'
which
is
a
refinement
of
{Ua>a•A
(i.e.,
each
V.s
is
contained
in
some
Ua)·
The
following
theorems
are
the
results
from
general
topology:
Theorem
2.4.1
A
paracompact
T
2
space
is
normal
(Kelley
1955].
Theorem
2.4.2
A
topological
space
is
paracompact
iff
45

every
open
cover
has
a
subordinate
partition
of
unity
[Kelley
1955].
Theorem
2.4.3
Every
metric
space
is
paracompact
[Kelley
1955].
Since
"metric"
notion
is
fundamental
to
any
world
model,
one
does
not
want
to
construct
a
world
model
which
is
non-
paracompact.
Recall
Theorem
2.2.16
and
the
subsequent
example,
an
arcwise
connected,
T
2
space
is
necessarily
to
be
connected.
But
the
strcuture
of
a
topological
manifold
is
rich
enough
such
that:
Theorem
2.4.4
A
topological
manifold
is
connected
iff
it
is
arcwise
connected.
Nonetheless,
a
topological
manifold
need
not
be
connected.
For
example,
the
group
space
of
O(n)
is
a
topological
manifold,
which
has
two
connected
components
corresponding
to
the
positive
and
negative
determinants.
Let
U
and
U'
be
open
subsets
of
Rm
and
R"
respectively,
and
let
f
be
a
mapping
of
u
into
u•.
The
map
f
is
differentiable
if
the
coordinates
yi(f(p}}
of
f(p}
are
differentiable
(sometimes
call
smooth,
i.e.,
infinitely
differentiable,
denoted
by
~)
functions
of
the
coordinates
xi(p},
p E
U.
A
differentiable
map
f:
U
~
U'
is
a
diffeomorphism
of
U
onto
U'
if
f(U}
=
U',
f
is
one-to-one,
and
the
inverse
map
f"
1
is
differentiable.
Let
M
be
a
Hausdorff
space.
An
open
chart
on
M
is
a
pair
(U,¢)
where
U c M
and
open,
and
¢
is
a
homeomorphism
of
u
onto
an
open
subset
of
Rm.
So,
the
dimension
of
M
is
m. A
differentiable
structure
on
M
of
dimension
m
is
a
collection
of
open
charts
cu
..
, ¢
..
)
ClfA
on
M
where
¢
..
cu
..
)
is
an
open
subset
of
Rm
such
that
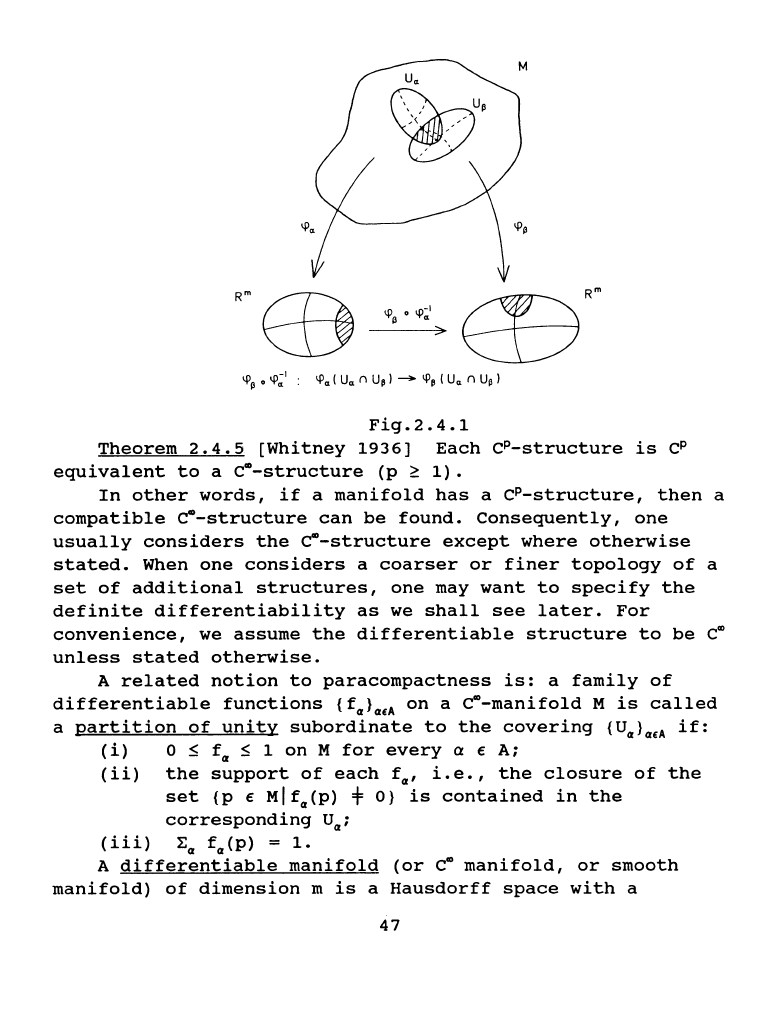
(i)
M = U u
..
;
(ii)
for
each
pair
a,~
E A
the
composite
map
¢p·¢
..
-
1
is
a
differentiable
map
of
¢"
(U"
n
Up)
onto
¢p(U" n
Up)
(see
the
following
figure);
(iii)
the
collection
(u
..
, ¢
..
)
is
a
maximal
family
of
open
charts
for
which
(i)
and
(ii)
hold.
Note:
(iii)
means
that
the
set
of
open
charts
{U"}
covers
M.
46
'l'p
•
'1'~
1
'1'. I u. n
u,
l -
'1',
I u. n
Up
l
Fig.2.4.1
Theorem
2.4.5
[Whitney
1936]
Each
CP-structure
is
CP
equivalent
to
a
c•-structure
(p
~
1).
In
other
words,
if
a
manifold
has
a
cP-structure,
then
a
compatible
c--structure
can
be
found.
Consequently,
one
usually
considers
the
c--structure
except
where
otherwise
stated.
When
one
considers
a
coarser
or
finer
topology
of
a
set
of
additional
structures,
one
may
want
to
specify
the
definite
differentiability
as
we
shall
see
later.
For
convenience,
we
assume
the
differentiable
structure
to
be
c•
unless
stated
otherwise.
A
related
notion
to
paracompactness
is:
a
family
of
differentiable
functions
{ f
11
}
111
A
on
a
c--manifold
M
is
called
a
partition
of
unity
subordinate
to
the
covering
{U
11
}
11
,A
if:
(i)
0 S f
11
S 1
on
M
for
every
a E
A~
(ii)
the
support
of
each
f
11
,
i.e.,
the
closure
of
the
set
{p
E
Mlf
11
(p)
+ 0}
is
contained
in
the
corresponding
U
11
~
(iii)
:Ell
fll(p)
=
1.
A
differentiable
manifold
(or
c-
manifold,
or
smooth
manifold)
of
dimension
m
is
a
Hausdorff
space
with
a
47

differentiable
structure
of
dimension
m.
In
other
words,
a
differentiable
manifold
of
dimension
m
is
a
topological
manifold
endowed
with
a
differentiable
structure.
Similarly,
an
analytic
manifold
is
defined
by
replacing
"differentiable"
by
"analytic".
The
essential
difference
between
a
topological
manifold
of
dimension
m
and
a
differentiable
manifold
of
dimension
m
is
that
the
differentiable
manifold
requires,
in
addition,
that
the
composite
map ¢
11
·q,a-
1
of
overlapping
region
Ua
n u
11
is
differentiable.
Clearly,
a
~-manifold
is
a
topological
manifold.
Not
surprising
from
a
logical
viewpoint,
but
remarkable
from
intuition,
is
that
the
reverse
assertion
is
false
[Kervaire
1961].
It
is
also
interesting
to
ask
whether
the
~-structure
on
a
em-
manifold
is
unique
or
not.
Milnor
[1956]
has
shown
that
s
7
has
more
than
one
distinct
~-structure.
Recall
from
earlier,
we
have
defined
a
differentiable
mapping
in
relation
to
open
sets
in
Rm
and
R".
Here
we
use
the
same
concept
to
define
a
differentiable
map
between
smooth
manifolds.
Let
M
and
N
are
smooth
manifolds
of
dimensions
m
and
n
respectively
and
f : M
~
N
be
continuous.
Then
f
is
a
differentiable
(or
smooth,
or
~)
map
if
for
every
p € M
and
every
coordinate
charts
¢a:
Ua
~
Rm
with
p €
Ua
and
TJ
11
: u
11
~
R"
with
f(p)
€ u
11
,
the
composite
map
T/
11
-f·q,a-
1
is
differentiable.
The
intuitive
outcome
of
this
definition
is
that
we
can
use
the
coordinate
charts
to
transfer
various
notions
from
manifolds
to
the
easily
understood
framework
in
Euclidean
spaces.
Let
M
1
and
M
2
be
two
~-manifolds.
They
are
diffeomorphic
if
there
are
~-maps
f:
M
1
~
M
2
and
g:
M
2
~
M
1
such
that
g·f
id
1
and
f·g
=
id
2
where
id
1
and
id
2
are
identity
maps
on
M
1
and
M
2
respectively.
The
maps f
and
g
are
diffeomorphisms.
If
the
manifolds
and
mappings
are
c·,
then
the
diffeomorphism
is
just
a
homeomorphism.
Furthermore,
we
do
not
need
to
explicitly
assume
that
the
manifolds
have
the
48

same
dimension.
This
follows
from
the
fact
that
if
two
manifolds
are
homeomorphic,
then they
have
the
same
dimension.
The
trivial
example
of
diffeomorphism
is
a
coordinate
transformation.
Moreover,
the
composition
of
two
diffeomorphisms
is
a
diffeomorphism,
thus
diffeomorphisms
form
a
group
called
the
group
of
diffeomorphisms.
We
shall
meet
it
again
later.
It
is
easy
to
convince
ourselves
that
equivalently
one
defines
a
diffeomorphism
of
M
1
onto
M
2
as
a
one-to-one
map
f:
M
1
-+
M
2
such
that
f
and
r
1
are
c"'.
Without
requiring
f-
1
be
also
c~,
f
is
just
a
c"'
homeomorphism.
But
c"'-homeomorphism
needs
not
be
a
diffeomorphism.
This
can
be
easily
seen
by
the
following
example.
For
n >
1,
let
u
2
n-
1
:
R
-+
R.
The
inverse
is
not
differentiable
at
the
origin.
This
also
shows
that
if
f
is
c~,
f-
1
needs
not
be
c~.
Since
our
goal
is
to
discuss
the
dynamical
systems,
differential
equations,
their
structural
stability
and
their
various
applications,
we
shall
deal
with
either
the
configuration
spaces
or
their
phase
spaces,
which
are
at
least
ck(k
~
1),
thus
without
any
confusion,
a
manifold
shall
mean
a
differentiable
manifold
hereafter
except
otherwise
stated.
Let
M
be
a
manifold,
p e M
and
denote
F(M,p)
be
the
set
of
all
C~
real
functions
with
domain
in
a
neighborhood
of
p.
A
ck
curve
in
M
is
a
map
of
a
closed
interval
[a,b]
into
M.
A
tangent
vector
at
pis
a
real
function
X
on
F(M,p)
(i.e.,
X
F(M,p)
-+
R)
having
the
following
properties:
(i)
X(f+g)
=Xf+Xg,
(ii)
X(af)
=
a(Xf),
(iii)
X(fg)
=
(Xf)
g(p)
+
f(p)
(Xg)
where
f,
g e
F(M,p)
and
a e
R.
The
above
rules
are
the
definition
of
a
derivation,
thus
a
tangent
vector
is
often
called
a
derivation
of
F(M,p).
All
the
tangents
at
p
form
a
linear
space
denoted
by
MP.
If~=
(x
1
,
••
,xm)
is
a
coordinate
syatem,
the
partial
49
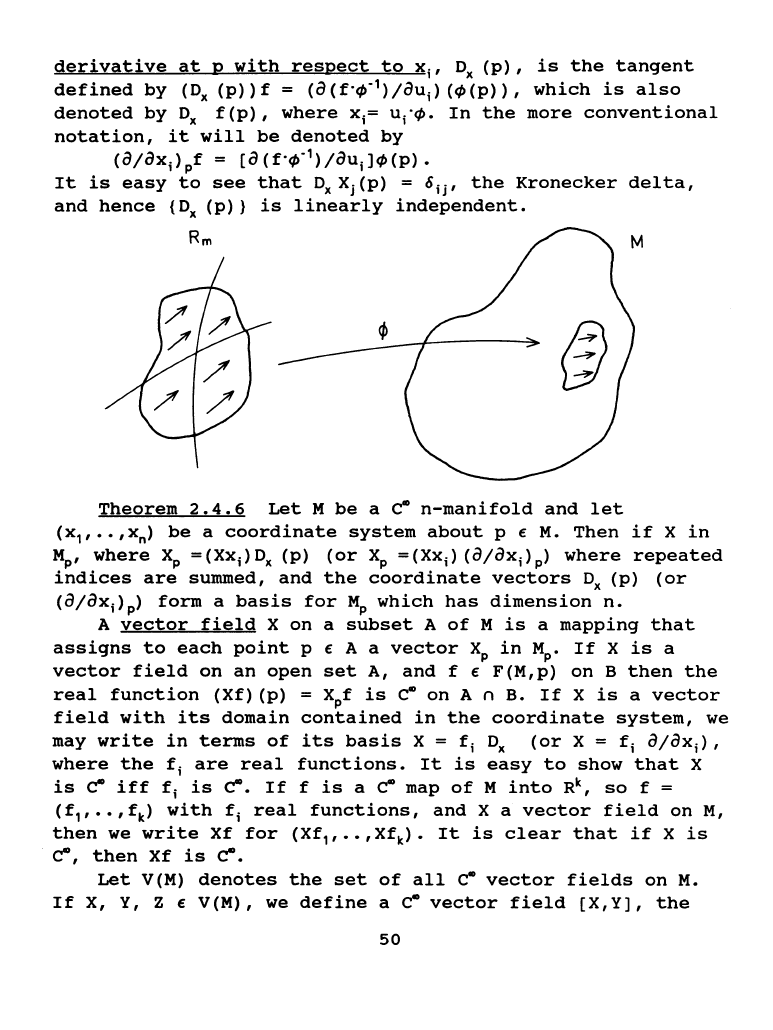
derivative
at
p
with
respect
to
xi,
Dx
(p),
is
the
tangent
defined
by
(Dx
(p))f
= cacf·q,"
1
)/aui)
(<fl(p)) I
which
is
also
denoted
by
Dx
f(p),
where
xi= ui·q,.
In
the
more
conventional
notation,
it
will
be
denoted
by
ca;axi)pf
= [a(f•cp"
1
);aui)<fl(p).
It
is
easy
to
see
that
Dx
Xi(p)
=
6ii'
the
Kronecker
delta,
and
hence
(Dx
(p)}
is
linearly
independent.
Rm
Theorem
2.4.6
Let
M
be
a
c-
n-manifold
and
let
(x
1
,
••
,xn)
be
a
coordinate
system
about
p
EM.
Then
if
X
in
~'
where
XP
=(Xxi)Dx
(p)
(or
XP
=(Xxi)
(a;axi)p)
where
repeated
indices
are
summed,
and
the
coordinate
vectors
Dx
(p)
(or
(a;axi)p)
form
a
basis
for
MP
which
has
dimension
n.
A
vector
field
X
on
a
subset
A
of
M
is
a
mapping
that
assigns
to
each
point
p E A a
vector
XP
in
MP.
If
X
is
a
vector
field
on
an
open
set
A,
and
f E
F(M,p)
on
B
then
the
real
function
(Xf)
(p)
XPf
is
c-
on
A n B.
If
X
is
a
vector
field
with
its
domain
contained
in
the
coordinate
system,
we
may
write
in
terms
of
its
basis
X =
fi
Dx
(or
X =
fi
a;axi)
I
where
the
fi
are
real
functions.
It
is
easy
to
show
that
X
is
c-
iff
fi
is
c-.
If
f
is
a
c-
map
of
M
into
Rk,
so
f =
(f
1
,
••
,fk)
with
f
1
real
functions,
and
X a
vector
field
on
M,
then
we
write
Xf
for
(Xf
1
,
••
,Xfk).
It
is
clear
that
if
X
is
c-,
then
Xf
is
c-.
Let
V(M)
denotes
the
set
of
all
c-
vector
fields
on
M.
If
X,
Y,
Z E V(M),
we
define
a
c-
vector
field
[X,Y],
the
50

bracket
of
X
and
Y,
on
the
intersection
of
their
domains
by
[X,Y] =
XY
-
YX.
Let
us
also
denote
F(M)
be
the
set
of
CU
real
valued
functions
on
M.
If
f,g
e
F(M),
it
is
trivial
that
[X,Y](f+g)
=
[X,Y]f
+
[X,Y]g
and
[X,Y](af)
=
a[X,Y]f
for
a e R. One
only
has
to
check
that
the
product
property
[X,Y](fg)
=
f[X,Y]g
+
g[X,Y]f
to
establish
[X,Y] E V(M).
It
is
clear
that
[X,Y]=
-[Y,X]
and
[X,X]=
o.
Moreover,
[fX,gY]
=
fg[X,Y]
+
f(Xg)Y
-
g(Yf)X.
The
bracket
operation
also
satisfies
the
Jacobi
identity,
[X,[Y,Z]]
+
[Z,[X,Y]]
+
[Y,[Z,X]]=
0.
Let
M
and
N
be
CO
manifolds
of
dimension
m
and
n
respectively.
We
have
defined
the
concept
of
a
CO
map f
from
M
into
N.
Such
a map
induces
a
linear
map
from
each
tangent
space
MP
into
the
tangent
space
Nf<p>.
This
linear
map
is
called
the
differential
of
f
and
it
is
defined
by:
if
t e
~'
~
e
F(N,f(p)),
then
df(t)(~)=
t(~·f).
By
selecting
a
coordinate
system
(x
1
,
••
,xm)
about
p
and
(y
1
,
••
,yn)
about
f(p),
we
can
determine
a
matrix
representation
for
df,
which
is
called
the
Jacobian
matrix
of
df,
with
respect
to
the
chosen
coordinate
systems
and
the
bases
{Dx
(p)}
and
{DY
(f(p))},
by
the
Jacobian
(Dx
(Y;"f)
(p))
for
1 S j S m
and
1 S
i S
n.
We
call
a
CU
map
f:M
~
N
non-
singular
at
p
if
df
at
pis
non-singular
i.e.,
the
Jacobian
at
pis
non-singular.
Let
f:M
~
N
be
CO
into
map.
(a)
f
is
an
immersion
if
dfP
is
non-singular
for
each
p
E
M.
(b)
The
pair
(M,f)
is
a
submanifold
of
N
if
f
is
an
one-to-one
immersion.
(c)
f
is
an
imbedding
if
f
is
an
one-to-one
immersion,
which
is
also
a
homeomorphism
into,
i.e.,
f
is
open
as
a map
into
f(M)
with
the
relative
topology.
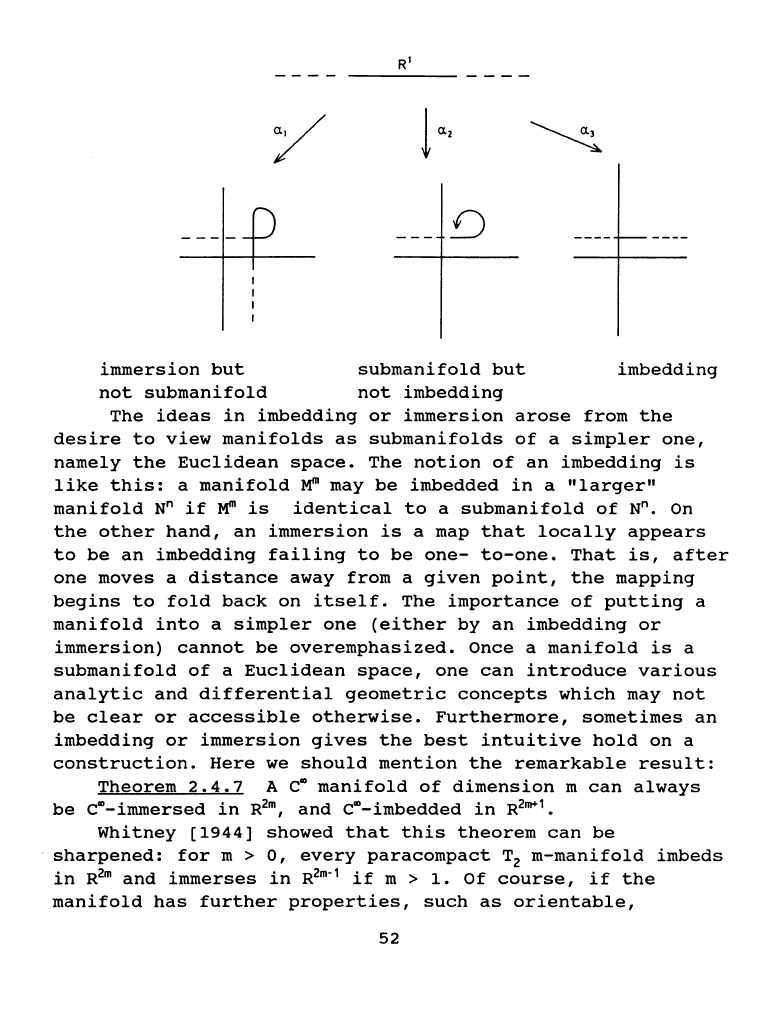
For
example,
one
can
immerse
the
real
line
R
into
the
Euclidean
plane
as
illustrated
in
the
following
figure.
Note
that
the
first
case
is
an
immersion
but
not
a
submanifold,
the
second
is
a
submanifold
but
not
an
imbedding,
and
the
third
is
an
imbedding.
51
R'
---------
--- D
immersion
but
submanifold
but
imbedding
not
submanifold
not
imbedding
The
ideas
in
imbedding
or
immersion
arose
from
the
desire
to
view
manifolds
as
submanifolds
of
a
simpler
one,
namely
the
Euclidean
space.
The
notion
of
an
imbedding
is
like
this:
a
manifold
M"'
may
be
imbedded
in
a
"larger"
manifold
N"
if
M"'
is
identical
to
a
submanifold
of
N". On
the
other
hand,
an
immersion
is
a
map
that
locally
appears
to
be
an
imbedding
failing
to
be
one-
to-one.
That
is,
after
one
moves
a
distance
away
from
a
given
point,
the
mapping
begins
to
fold
back
on
itself.
The
importance
of
putting
a
manifold
into
a
simpler
one
(either
by
an
imbedding
or
immersion)
cannot
be
overemphasized.
Once
a
manifold
is
a
submanifold
of
a
Euclidean
space,
one
can
introduce
various
analytic
and
differential
geometric
concepts
which
may
not
be
clear
or
accessible
otherwise.
Furthermore,
sometimes
an
imbedding
or
immersion
gives
the
best
intuitive
hold
on
a
construction.
Here
we
should
mention
the
remarkable
result:
Theorem
2.4.7
A
~manifold
of
dimension
m
can
always
be
~-immersed
in
R
2
m,
and
~-imbedded
in
R
2
"""
1
•
Whitney
(1944]
showed
that
this
theorem
can
be
sharpened:
for
m >
O,
every
paracompact
T
2
m-manifold
imbeds
in
R
2
m
and
immerses
in
R
2
m-l
if
m >
1.
Of
course,
if
the
manifold
has
further
properties,
such
as
orientable,
52
parallelizable,
(these
will
be
discussed
later),
then
the
imbedding
(or
immersion)
theory
can
be
improved
even
further.
E.g.,
a
theorem
due
to
Hirsch
[1959]
states
that
any
para1lelizable
manifold
can
be
immersed
in
an
Euclidean
space
of
one
higher
dimension.
For
a
nice
survey
of
work
on
imbeddings,
see
Lashof
[1965].
Inverse
Function
Theorem
2.4.8
Let
(x
1
,
••
,xm)
be
a
coordinate
system
at
p £
M,
f
1
,
••
,fm £
F(M,p).
Then~
(f
1
,
••
,fm)
restricts
to
a
coordinate
system
at
p
iff
det(Dx.fi(p))
=f
O,
i.e.,
d~
is
non-singular
on~·
wJ
can
restate
the
Inverse
Function
Theorem
in
a
more
familier
form:
Theorem
2.4.9
Let
f:
Rm-+
Rm
be
a
c"'
map.
For
p £
Rm
if
Dx;
fi
(p)
is
non-singular,
i.e.,
as
a
linear
transformation
Dxi
fi(p)
is
onto.
Then
there
are
open
subsets
U
and
V
of
Rm,
and
p £ U
and
q =
f(p)
£
v,
such
that
the
restriction
flU
is
a
diffeomorphism
from
U
onto
V;
i.e.,
there
is
a
c"'
map
g:V
-+ U
which
is
an
inverse
to
flu,
and
Dx.gi(q)
is
the
inverse
to
the
matrix
Dx.
fi
(p).
1
For
example,•
in
functions
of
several
variables,
say
when
we
deal
with
curvilinear
coordinates,
a
pair
of
functions
x
=
f(u,v),
y =
g(u,v)
can
be
regarded
as
mappings
from
the
uv-plane
to
the
xy-plane,
and
the
inverse
function
u =
~(x,y),
v = •
(x,y)
with
proper
domains.
v
y
E
X
The
well-known
result
[a(x,y)/a(u,v)]·
[a(u,v);acx,y)]
1,
53
