Kurfess T.R. Robotics and Automation Handbook
Подождите немного. Документ загружается.

10
-18 Robotics and Automation Handbook
Using
ˆ
a and c,wecandefine a local coordinate system on the tool defined by the set of mutually orthogonal
unit vectors
ˆ
e
1
,
ˆ
e
2
, and
ˆ
e
3
. The local coordinate system is defined as
ˆ
e
1
=
ˆ
a
if |
˙
ˆ
a|=0 then
ˆ
e
2
=
˙
ˆ
a
|
˙
ˆ
a|
and
ˆ
e
3
=
ˆ
e
1
×
ˆ
e
2
(10.29)
if |
˙
ˆ
a|=0 and
˙
ˆ
a ·
˙
c = 0 then
ˆ
e
3
=
ˆ
a ×
˙
c
|
˙
c|
and
ˆ
e
2
=
ˆ
e
3
×
ˆ
e
1
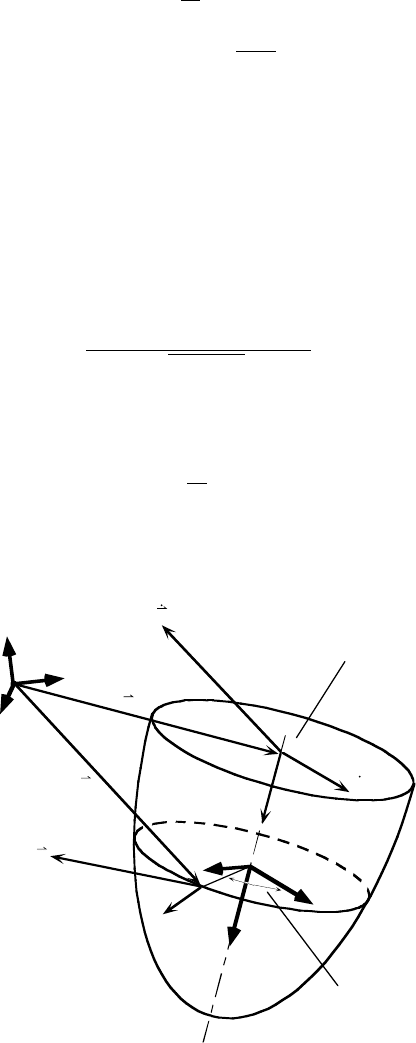
Consider, as depicted in Figure 10.15, the circle defined by the intersection of a plane normal to the
cutting tool’s axis of rotation
ˆ
a, offset from the origin of the tool coordinate system c, by the perpendicular
distance u. A point p lies in that plane at an angle θ measured from the axis
ˆ
e
2
. The velocity of point is
v =
˙
c + u|
˙
ˆ
a|
ˆ
e
2
−r (u)|
˙
ˆ
a|
ˆ
e
1
cos θ (10.30)
The unit normal vector to the surface of revolution at point p is
ˆ
n =
−r
(u)
ˆ
e
1
+
ˆ
e
2
cos θ +
ˆ
e
3
sin θ
√
1 +r
(u)
2
(10.31)
where
r
(u) =
dr
dz
z=u
(10.32)
n
^
a
^
e
1
^
e
3
^
e
2
^
Θ
Workpiece
Local Coordinate
System
Cutting Tool
Local Coordinate
System Origin
a
^
c
c
p
v
FIGURE 10.15 Notation for calculating points on the critical curve.
Copyright © 2005 by CRC Press LLC

Error Budgeting 10
-
19
Combining Equation (10.30) and Equation (10.31) into Equation (10.24) yields an equation of the form
A cos + B sin + C = 0
(10.33)
where
A =|
˙
ˆ
a|r
(u)r (u) +
˙
c ·
ˆ
e
2
+|
˙
ˆ
a|u
(10.34)
B =
˙
c ·
ˆ
e
3
(10.35)
C =−r
(u)
˙
c ·
ˆ
e
1
(10.36)
Equation (10.33) admits a closed form solution
D =
B
2
+ A
2
−C
2
(10.37)
cos θ =
C
2
− AC − B
2
+ BD
A
2
− AC + B
2
− BD
(10.38)
sin θ =
(A −C)(B − D)
A
2
− AC + B
2
− BD
(10.39)
The results of Equation (10.38) and Equation (10.39) can be used to compute the positions of the points
p(t, u) on the surface of the working envelope
p(t, u) = c + u
ˆ
e
1
+r (u)(
ˆ
e
2
cos θ ±
ˆ
e
3
sin θ ) (10.40)
The “±” symbol indicates that there are two solutions for p(t, u). If the tool is cutting a trough, then
both solutions are valid. If the tool is contouring counterclockwise as viewed in the positive
ˆ
e
3
direction,
then retain only the solution corresponding to the plus sign. If the tool is contouring clockwise as viewed
in the positive
ˆ
e
3
direction, then retain only the solution corresponding to the minus sign.
As is evident from Equation (10.39) above, if A is equal to −C then sin θ is zero, cosθ is one, and θ
equals zero. For any values of u such that A −C < 0, that cross section of the tool is not in contact with
the workpiece surface. Similarly, if the value of D is imaginary, the results of the calculation can be safely
disregarded because the cutting tool is again not in contact with the workpiece. This occurs only when the
tool is plunging at a steep angle so that every point cut by that section of the tool is immediately swept
away by the cross sections following behind it.
The closed form solution described abovecan be used in error budgeting byperforming threeoperations:
(1) compute the swept envelope of the cutting tool using a kinematic model with no error motions to
determine the nominal machined surface; (2) compute the swept envelope of the cutting tool including
error motions to determine the perturbed machined surface; (3) evaluate the accuracy of the machining
process by comparing the perturbed and nominal machined surfaces. The procedure described above has
proved useful for modeling form grinding, centerless grinding, and “chasing the pin” cylindrical grinding.
It should also be useful for ball end milling and flank milling with conical milling cutters. Due to the
assumption that the cutter is a perfect surface or revolution, it is most useful for evaluating tolerances of
form, profile, location, and size and is probably less useful for surface finish.
10.8 Summary
An error budget is a tool for predicting and managing variability in an engineering system. This chapter
has reviewed basic theory of probability, tolerances, and kinematics and described a framework for error
budgeting based upon those theoretical foundations. The framework presented here is particularly suited
to manufacturing systems including robots, machine tools, and coordinate measuring machines.
Copyright © 2005 by CRC Press LLC
10
-20 Robotics and Automation Handbook
Anerror budget must be developedwithgreat carebecause small mistakes in the underlyingassumptions
or the mathematical implementation can lead to erroneous results. For this reason, error budgets should
be kept as simple as possible, consistent with the needs of the task at hand. When error budgets are scoped
appropriately, developed rigorously, and consistent with theoretical foundations (e.g., engineering science,
mathematics, and probability), they are an indispensable tool for system design.
References
[1] Donaldson, R.R. (1980). Error Budgets. Technology of Machine Tools, Vol. 5, Machine Tool Task
Force, Robert J. Hocken, Chairman, Lawrence Livermore National Laboratory.
[2] Slocum, A.H. (1992). Precision Machine Design. Prentice Hall, Englewood Cliffs, NJ.
[3] Soons, J.A., Theuws, F.C., and Schellekens, P.H. (1992). Modeling the errors of multi-axis machines:
a general methodology. Precision Eng., vol. 14, no. 1, pp. 5–19.
[4] Chao,L.M. and Yang,J.C.S.(1987). Implementation of a scheme toimprovethe positioning accuracy
of an articulate robot by using laser distance-measuring interferometry, Precision Eng., vol. 9, no. 4,
pp. 210–217.
[5] Frey, D.D., Otto,K.N., and Pflager, W. (1997). Swept envelopes of cutting tools in integrated machine
and workpiece error budgeting. Ann. CIRP, vol. 46, no. 1, pp. 475–480.
[6] Frey, D.D., Otto, K.N., and Taketani, S. (2001). Manufacturing system block diagrams and optimal
adjustment procedures. ASME J. Manuf. Sci. Eng., vol. 123, no. 1, pp. 119–127.
[7] Frey, D.D. and Hykes, T. (1997). A Method for Virtual Machining. U.S. Patent #5,691,909.
[8] Treib, T. (1987). Error budgeting — applied to the calculation and optimization of the volumetric
error field of multiaxis systems. Ann. CIRP, vol. 36, no. 1, pp. 365–368.
[9] Portman, T. (1980). Error summation in the analytical calculation of lathe accuracy. Machines and
Tooling, vol. 51, no. 1, pp. 7–10.
[10] Narawa, L., Kowalski, M., and Sladek, J. (1989). The influence of kinematic errors on the profile
shapes by means of CMM. Ann. CIRP, vol. 38, no. 1, pp. 511–516.
[11] Whitney, D.E., Gilbert, O.L., and Jastrzebski, M. (1994). Representation of geometric variations
using matrix transforms for statistical tolerance analysis in assemblies. Res. Eng. Design, vol. 6,
pp. 191–210.
[12] Donmez, A. (1995). A General Methodology for Machine Tool Accuracy Enhancement: Theory, Appli-
cation, and Implementation, Ph.D. thesis, Purdue University.
[13] Ceglarek, D. and Shi, J. (1996). Fixture failure diagnosis for the autobody assembly using pattern
recognition. ASME J. Eng. Ind., vol. 118, no. 1, pp. 55–66.
[14] Kurfess, T.R., Banks, D.L., and Wolfson, J.J. (1996). A multivariate statistical approach to metrology.
ASME J. Manuf. Sci. Eng., vol. 118, no. 1, pp. 652–657.
[15] Drake, A.W. (1967). Fundamentals of Applied Probability Theory. McGraw-Hill, New York.
[16] ASME (1983). ANSI Y14.5M — Dimensioning and Tolerancing. American Society of Mechanical
Engineering, New York.
[17] Kane, V.E. (1986). Process capability indices. J. Qual. Technol., vol. 18, no. 1, pp. 41–52.
[18] Harry, M.J. and Lawson, J.R. (1992). Six Sigma Producibility Analysis and Process Characterization.
Addison-Wesley, Reading, MA.
[19] Phadke, M.S. (1989). Quality Engineering Using Robust Design. Prentice Hall, Englewood Cliffs, NJ.
[20] Denavit, J. and Hartenberg, R. (1955). A kinematic notation for lower pair mechanisms based on
matrices. J. Appl. Mech, vol. 1, pp. 215–221.
[21] Bryan, J.B. (1989). The Abb
´
e principle revisited — an updated interpretation. Precision Eng., vol. 1,
no. 3, pp. 129–132.
[22] Lin, P.D. and Ehmann, K.F. (1993). Direct volumetric error evaluation for multi-axis machines. Int.
J. Machine Tools Manuf., vol. 33, no. 5, pp. 675–693.
[23] CIRP (1978). A proposal for defining and specifying the dimensional uncertainty of multiaxis
measuring machines. Ann. CIRP, vol. 27, no. 2, pp. 623–630.
Copyright © 2005 by CRC Press LLC
Error Budgeting 10
-
21
[24] Shen, Y.L. and Duffie, N.A. (1993). Comparison of combinatorial rules for machine error budgets.
Ann. CIRP, vol. 42, no. 1, pp. 619–622.
[25] Hocken, R.J. and Machine Tool Task Force (1980). Technology of Machine Tools, UCRL-52960-5,
Lawrence Livermore National Laboratory, University of California, Livermore, CA.
[26] Wang, W.P. and Wang, K.K. (1986). Geometric modeling for swept volume of moving solids. IEEE
Comput. Graphics Appl., vol. 6, no. 12, pp. 8–17.
Copyright © 2005 by CRC Press LLC

11
Design of Robotic End
Effectors
Hodge Jenkins
Mercer University
11.1 Introduction
11.2 Process and Environment
System Design
•
Special Environments
11.3 Robot Attachment and Payload Capacity
Integrated End Effector Attachment
•
Attachment Precision
•
Special End Effector Locations
•
Wrist Compliance: Remote
Compliance Centers
•
Payloads
•
Payload Force Analysis
11.4 Power Sources
Compressed Air
•
Vacuum
•
Hydraulic Fluid Power
•
Electrical Power
•
Other Actuators
11.5 Gripper Kinematics
Parallel Axis/Linear Motion Jaws
•
Pivoting/Rotary Action
Jaws
•
Four-Bar Linkage Jaws
•
Multiple Jaw/Chuck Style
•
Articulating Fingers
•
Multi-Component End Effectors
11.6 Grasping Modes, Forces, and Stability
Grasping Stability
•
Friction and Grasping Forces
11.7 Design Guidelines for Grippers and Jaws
Gripper and Jaw Design Geometry
•
Gripper Design
Procedure
•
Gripper Design: Case Study
•
Gripper Jaw
Design Algorithms
•
Interchangeable End Effectors
•
Special Purpose End Effectors/Complementary Tools
11.8 Sensors and Control Considerations
Proximity Sensors
•
Collision Sensors
•
Tactile
Feedback/Force Sensing
•
Acceleration Control for Payload
Limits
•
Tactile Force Control
11.9 Conclusion
11.1 Introduction
Aside from the robot itself, the most critical device in a robotic automation system is the end effector.
Basic grasping end effector forms are referred to as grippers. Designs for end effectors are as numerous
as the applications employing robots. End effectors can be part of the robot’s integral design or added-on
to the base robot. The design depends on the particular robot being implemented, objects to be grasped,
tasks to be performed, and the robot work environment.
This chapter outlines many of the design and selection decisions of robotic end effectors. First, process
and environment considerations are discussed. Robot considerations including power, joint compliance,
payload capacity, and attachment are presented. Sections reviewing basic end effector styles and design
Copyright © 2005 by CRC Press LLC

11
-2 Robotics and Automation Handbook
guidelines follow this. Sensors and control issues are also presented. The chapter concludes with an end
effector design.
11.2 Process and Environment
Robots vary in size and payload capacities for many diverse operations. Some robots are designed for
specific, singular tasks such as materials handling, painting, welding, cutting, grinding, or deburing.
These robots use specific tools as end effectors. Primary considerations for end effector designs, in these
instances, are the tool, orientation, and control of the tool for effective processing, as well as the robot
payload capacity (to be discussed later). Other robots are designed for general purposes and material
handling. These robots require additional engineering detail in end effector design. In all cases the tasks
and the robot environment must be considered when selecting or designing the appropriate robot and
end effector. The end effector is seen as part of the overall system design subject to the same constraints
as the entire system.
11.2.1 System Design
The end effector is but part of a system. As with all system designs, it is important to have a process flow
diagram describing the tasks and how they are to be accomplished, before designing the end effector.
This will clarify the process in terms of the objects to be handled and what functions are necessary for
the end effector, as well as the entire system. A typical process flow chart defines the items to be han-
dled, where it is grasped, what is done to the item, where it is placed, and special orientations. A sample
chart is shown in Figure 11.1. This defines the roles of the robot, system controller, peripheral devices,
end effector, and specialized tools. From the activities necessary to complete the process, the end effector
design requirements and specifications are formed.
Take to dispense station
Place tube
Open thermal
cover
Pick up first test tube
from next tube rack position
Dispense solution
into sample
Pick up first cap
from next cap rack position
Place cap on tube at
dispense station
Pick up capped
tube and place
in next thermal
bath rack
position
···
···
FIGURE 11.1 Sample process flow chart.
Copyright © 2005 by CRC Press LLC

Design of Robotic End Effectors 11
-3
Many other aspects affect the design of the end effectors. Even with robot, process, and system require-
ments and specifications available, not enough necessary information may be provided about a process.
Many questions will need to be examined to complete the system and end effector design.
For example, answers to these questions and others will be necessary for designing end effectors.
1. What are the shape and weight of the grasped objects?
2. What is the uniformity or tolerance of handled objects?
3. Are the objects’ surfaces smooth? What is the coefficient of friction?
4. What is the spacing of objects?
5. How will they be oriented and presented?
6. What placement and spacing dimensions can be modified?
7. What are placement tolerances?
8. What are grasping forces or pressure limits?
9. Are complementary tools needed?
10. Is compliance necessary for assembling parts?
11. Are there special environmental considerations?
11.2.2 Special Environments
Many industrial situations have special system environmental requirements that impact the robot and end
effector design. A large area of operation for robots is semi-conductor manufacturing. Here robots must
operate in various clean room conditions. End effectors must be selected for compatibility to the class
of clean room in which the robot will operate. It is important that the end effector not generate many
particles in this situation. End effectors must be designed with all surfaces to be either stainless steel or
polymer or to be coated with a clean room acceptable material (such as an anodized aluminum surface
or a baked-on powder coat). Polymer (oil-less) washers and bearings should be used in the end effector
mechanisms to ensure that the surfaces do not contact, wear, or generate particles. In some circumstances,
mechanisms must be enclosed with seals or bellows. To meet the requirements for Class 1 clean room
operation, air bearings may be required. Many manufacturers sell end effectors designed for clean room
operation.
A different problemarises in hazardousenvironmentssuch as in the presenceof highlyreactivechemicals
(e.g., chlorides, fluorides). Here the robot and end effector must be protected. Robot systems must be
designed for chemical resistance. If using a custom end effector, component materials should be selected
to be as inert as possible based on the chemicals present. Nickel or aluminum alloys, for example, work
fairly well with oxidizers such as chlorine, fluorine, or high concentrations of oxygen. In many cases a
covering (including a glove for the end effector) with a pressurized gas purge system must be provided to
prevent gaseous vapors or aerosols from reacting with the end-effector or robot.
Another robotic environment requiring special treatments is food processing.In food handling tasks, the
end effectorand robot must be able to be cleaned and sterilized to kill germs and bacteria. High temperature
water and disinfectant sprays are commonly used to clean equipment. Non-corrosive materials and sealed
electrical connections for wash downs are required.
11.3 Robot Attachment and Payload Capacity
End effectors for general purpose robots are mounted at the end of the robot arm or wrist for articulating
arm robots, or at the end of the last affixed stage or motion device for SCARA and Cartesian coordinate
robots. Some robots have built-in grippers. Aside from these self-contained mechanisms, end effectors are
selected from standard components, a custom design, or most likely a combination of both. End effectors
can have multiple tools or grippers, but they must conform to the mounting attachment and payload
limits of the individual robot.
Copyright © 2005 by CRC Press LLC

11
-4 Robotics and Automation Handbook
FIGURE 11.2 Robot end effector integrated into arm controller.
11.3.1 Integrated End Effector Attachment
Several types of robots have their end effectors highly integrated into the robot. In specialized applications
such as welding, painting, gluing, and plasma cutting, the end effector is essentially a pre-engineered sub-
system of the robot. For example, Fanuc offers arc welders as end effectors for their robots, as a complete
system. Several smaller robots, such as those used in light manufacturing or bench-top applications, have
the basic motion of the end effector built into the robot itself. These integral end effectors are basic gripper
designs. The gripper integration feature is seen in robots such as the Microbot Alpha II (Questech, Inc.) and
the A465 (Thermo-CRS, Ltd.) (Figure 11.2). The Microbot uses two parallel beam mechanisms for each
side of the gripper, as seen in Figure 11.3. The control is provided by springs for opening the grippers and by
a motorspooling a lightweightcable to close the grippers. The A654 robot has a servo controlledparallel jaw
gripper. Control of the gripper opening is included in the robot controller. Even using the existing mech-
anisms, custom application jaws for the gripper can be designed and mounted to the gripper base. Note:
these robots allow the removal of the standard gripper and provide a mounting base for other end effectors.
11.3.2 Attachment Precision
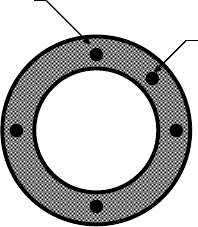
The attachment of the end effector to the robot must be secure and maintain the desired precision of the
robot system. Location and tolerance are extremely critical for maintenance and end effector replacement.
Precision dowel pins are used to locate and provide orientation for the end-effector while bolts secure it to
the robot. Figure 11.2 and Figure 11.4 show mounting configurations at the end of industrial robot arms.
Attachment means vary. Figure 11.2 shows an integral control attachment, while Figure 11.4 depicts an
internal passageway provided for power connections.
Itisstill possiblefor a replacementend effectortolose positional accuracy,even withpreviously described
design precautions. Thus, calibration of a newly placed end effector is necessary. This can be accomplished
Close Open
FIGURE 11.3 Four bar linkages gripper arms.
Copyright © 2005 by CRC Press LLC
Design of Robotic End Effectors 11
-5
End effector
power
connection
hole
Threaded hole
bolt circle: 4 holes
(attachment)
Precision hole for
dowel pin
(location &
Orientation)
FIGURE 11.4 Typical robot arm end.
in several ways. The most common techniques are to place the robotin teach mode to redefine key geometry
locations to hard stop locations, or to have specialized devices with proximity sensors to programmatically
locate a position or grasped object. Since all components of a robot and the grasped objects may have
varying tolerances, it is better to locate from a grasped artifact of calibrated dimensions, rather than a
sample of the actual grasped object, unless the object meets the desired tolerance specifications.
11.3.3 Special End Effector Locations
In grasping large objects such as metal sheets, special attention must be given to the location of grippers
(here multiple grippers are used in the end effector). Stability and preventing damage to the sheet are the
primary concerns. The reader is referred to Ceglarek et al. [1] for additional information.
11.3.4 Wrist Compliance: Remote Compliance Centers
Robots, workpieces, and fixturing have limited and defined tolerances. Some variation in workpieces
or orientation from fixturing may exist. In robotic or automation applications of part insertion into
another component or assembly, the use of a remote center compliance device (RCC) (typically wrist
mounted) is often required. The RCC allows an assembly robot or machine to compensate for positioning
errors (misalignments) during insertion. These position errors are due to machine or workpiece fixturing
inaccuracy, vibration, end effector path error, or tolerances.The RCCaccomplishesthis by lowering contact
forces (perpendicular to the axis of insertion) via lower horizontal and rotational stiffnesses while retaining
relatively higher axial insertion stiffness. This compliance can reduce or avoid the potential for part, robot,
or end effector damage.
A typical remote compliance center design is seen in Figure 11.5. When the remote compliance center is
near the contact point, the part will align with the hole automatically; correcting lateral and rotational mis-
alignment. RCC are typically passive mechanical devices with high axial stiffness but low lateral stiffness.
Many commercial designs use elastomer shear pads or springs or pneumatic pistons to create the compli-
ance. Other custom designs use a simple beam with greater horizontal than axial compliance (Ciblak [2]).
In all cases the RCC has limits on rotational or horizontal misalignments (typically less than +/ − 0.25
in. and +/ − 10
◦
[3]). Considerations for using an RCC are the added weight of the device and increased
moment caused by the additional length of end effector. However, if accounted for properly in design, the
RCC is a great aid for speedy part insertion.
11.3.5 Payloads
For all end effector designs the payload capacity of the robot must be carefully considered in the design.
Manufacturers specify the payload for each robot. It is important to consider acceleration forces and
moments as part of the load, if not already accounted for by the manufacturer.
Copyright © 2005 by CRC Press LLC
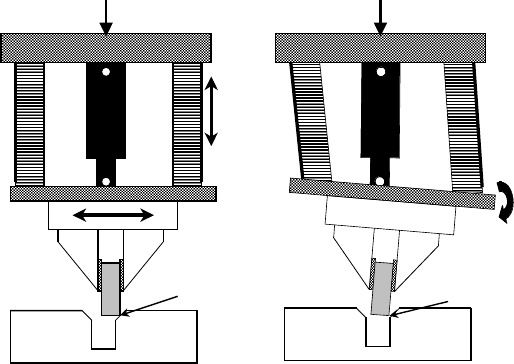
11
-6 Robotics and Automation Handbook
Axial Insertion Force
High
Axial
Stiffness
Low Lateral
Stiffness
Remote Center
Axial Insertion Force
Remote Center
FIGURE 11.5 Remote compliance center device.
Where the end effector is provided or integrated into the robot by the manufacturer, the payload is
specified as a weight or mass at a given distance on the gripper jaw of the end effector. These robots have
lighter allowable payloads (e.g., 2 kg for the CRS A465 [4]) with the manufacturer provided end effector. If
the standard end effector or rigid gripper attachment is replaced with a longer jaw, the allowable payload
will be less. This is because a higher torque will be generated at the robot joints.
Most general-purpose, articulating robots have specified the payload capacity in force and moment load
limits at the end of arm wrist attachment as well as other joint locations. The load and moment limits must
be adhered to when designing the end effector and gripper attachments. In these cases the acceleration of
the payload must also be included in addition to gravitational loading. For SCARA or Cartesian coordinate
style robots, payload capacity and maximum final joint inertia are sufficient, given the robot kinematics.
11.3.6 Payload Force Analysis
Force calculations for end effector payloads must be determined at all critical locations including the
gripper jaws and end of arm payload wrist reactions. Jaw designs and part-grasped orientation must be
known or have a bounding estimate. Figure 11.6 and Figure 11.7 depict typical force and moment locations
for end of arm geometry. The three-dimensional reaction force and moment at the end of arm location is
given in Cartesian coordinates by Equation (11.1) and Equation (11.2). Note that in developing dynamical
relationships for control design other coordinate systems, such as a polar or the generalized coordinate
methods developed by Kane [5] may be more advantageous.
F
R
= F
X
ˆ
i + F
Y
ˆ
j + F
Z
ˆ
k
(11.1)
M
R
= M
X
ˆ
i + M
Y
ˆ
j + M
Z
ˆ
k
(11.2)
The apparent force of an accelerated, grasped object is determined by application of Newton’s Second
Law, Equation (11.3). Note: In the configuration examined here, gravity is assumed to be present only in
the negative Y-direction. The subscript o denotes parameters associated with the object to be grasped,
while the g subscript indicates those parameters associated with the gripper/end effector.
F
R
= (m
g
+ m
o
)(A
X
ˆ
i +|A
Y
+ g
Y
|
ˆ
j + A
Z
ˆ
k)
(11.3)
Copyright © 2005 by CRC Press LLC
