Klyatskin V.I. Lectures on Dynamics of Stochastic Systems
Подождите немного. Документ загружается.

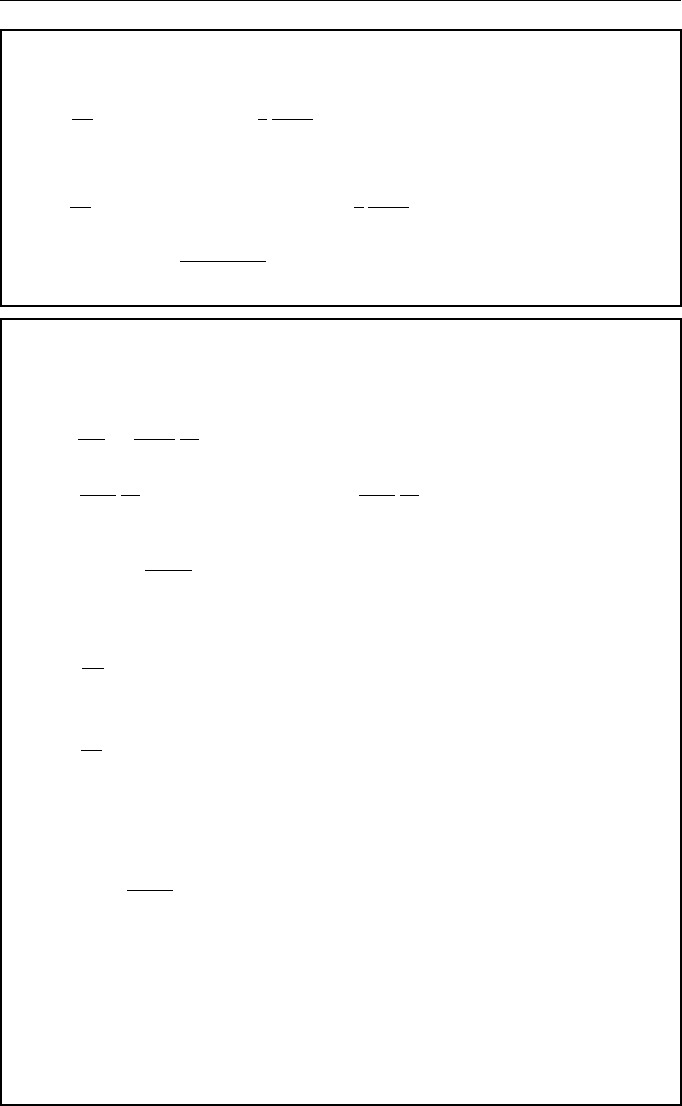
66 Lectures on Dynamics of Stochastic Systems
Solution
∂
∂L
u(x;L) =
ik(L) +
1
2
k
0
(L)
k(L)
[
2 − u(L;L)
]
u(x;L),
u(x;L)|
L=x
= u(x;x),
d
dL
u(L;L) = ik(L)
[
u(L;L) − 1
]
+
1
2
k
0
(L)
k(L)
[
2 − u(L;L)
]
u(L;L),
u(L
0
;L
0
) =
2k(L
0
)
k(L
0
) + k
1
.
Problem 2.3
Derive the imbedding equations for the boundary-value problem
d
2
dx
2
−
ρ
0
(x)
ρ(x)
d
dx
+ p
2
[
1 + ε(x)
]
u(x) = 0,
1
ρ(x)
d
dx
+ ip
u(x)
x=L
0
= 0,
1
ρ(x)
d
dx
− ip
u(x)
x=L
= −2ip,
where ρ
0
(x) =
dρ(x)
dx
Solution The imbedding equations with respect to parameter L have the form
∂
∂L
u(x;L) =
{
ipρ(L) + ϕ(L)u(L;L)
}
u(x;L),
u(x;L)|
L=x
= u(x;x);
d
dL
u(L;L) = 2ipρ(L)
[
u(L;L) − 1
]
+ ϕ(L)u
2
(L;L),
u(L;L)|
L=L
0
= 1,
where
ϕ(x) =
ip
2ρ(x)
h
1 + ε(x) − ρ
2
(x)
i
.
Remark The above boundary-value problem describes many physical processes,
such as acoustic waves in a medium with variable density and some types of
electromagnetic waves. In these cases, function ε(x) describes inhomogeneities
of the velocity of wave propagation (refractive index or dielectric permittivity)
and function ρ(x) appears only in the case of acoustic waves and characterize
medium density.

Solution Dependence on Problem Type, Medium Parameters, and Initial Data 67
Problem 2.4
Derive the imbedding equations for the matrix Helmholtz equation
d
2
dx
2
+ γ (x)
d
dx
+ K(x)
U(x) = 0,
d
dx
+ B
U(x)
x=L
= D,
d
dx
+C
U(x)
x=0
= 0,
where γ (x), K(x), and U(x) are the variable matrixes, while B, C, and D are the
constant matrixes.
Solution The imbedding equations with respect to parameter L have the form
∂
∂L
U(x;L) = U(x;L)3(L), U(x;L)|
L=x
= U(x;x),
d
dL
U(L;L) = D −
n
BU(L;L) + U(L;L)D
−1
BD
o
+ U(L;L)D
−1
γ (L)D
+ U(L;L)D
−1
n
K(L) − γ (L)B + B
2
o
U(L;L),
U(0;0) = (B − C)
−1
D,
where matrix 3(L) is given by the formula
3(L) = D
−1
[
γ (L) − B
]
D + D
−1
h
K(L) + B
2
− γ (L)B
i
U(L;L).
This page intentionally left blank

Lecture 3
Indicator Function and
Liouville Equation
Modern apparatus of the theory of random processes is able of constructing closed
descriptions of dynamic systems if these systems meet the condition of dynamic
causality and are formulated in terms of linear partial differential equations or certain
types of integral equations. One can use indicator functions to perform the transition
from the initial, generally nonlinear system to the equivalent description in terms of
the linear partial differential equations. However, this approach results in increasing
the dimension of the space of variables. Consider such a transition in the dynamic
systems described in the Lecture 1 as examples.
3.1 Ordinary Differential Equations
Let a stochastic problem is described by the system of equations (1.1), which we’ll
rewrite in the form
d
dt
r(t) = U
(
r(t), t
)
, r(t
0
) = r
0
. (3.1)
We introduce the scalar function
ϕ(r, t) = δ(r(t) − r), (3.2)
which is concentrated on the section of the random process r(t) by a given plane
r(t) = r and is usually called the indicator function.
Differentiating Eq. (3.2) with respect to time t, we obtain, using Eq. (3.1), the
equality
∂
∂t
ϕ(r, t) = −
dr(t)
dt
∂
∂r
δ(r(t) − r) = −U
(
r(t), t
)
∂
∂r
δ(r(t) − r)
= −
∂
∂r
[
U
(
r(t), t
)
δ(r(t) − r)
]
.
Using then the probing property of the delta-function
U
(
r(t), t
)
δ(r(t) − r) = U
(
r, t
)
δ(r(t) − r),
Lectures on Dynamics of Stochastic Systems. DOI: 10.1016/B978-0-12-384966-3.00003-9
Copyright
c
2011 Elsevier Inc. All rights reserved.

70 Lectures on Dynamics of Stochastic Systems
we obtain the linear partial differential equation
∂
∂t
+
∂
∂r
U(r, t)
ϕ(r, t) = 0, ϕ(r, t
0
) = δ(r
0
− r), (3.3)
equivalent to the initial system. This equation is called the Liouville equation. In terms
of phase points moving in phase space {r}, this equation corresponds to the equation
of continuity.
The above derivation procedure of the Liouville equation clearly shows the neces-
sity of distinguishing the function r(t) and the parameter r. In this connection, cor-
rect working with the delta-function assumes that the argument of function r(t) was
explicitly written in all intermediate calculations (cf. the forms of Eqs. (3.1) and (1.1),
page 3). Neglecting this rule usually results in errors.
The transition from system (3.1) to Liouville equation (3.3) entails enlargement
of the phase space (r, t), which, however, has a finite dimension. Note that Eq. (3.3)
coincides in form with the equation of tracer transfer by the velocity field U(r, t)
(1.39); the only difference consists in the initial values.
Equation (3.3) can be rewritten in the form of the linear integral equation
ϕ(r, t) = δ(r − r
0
) −
t
Z
t
0
dτ
∂
∂r
{
U(r, τ )ϕ(r, τ )
}
.
Varying this equation with respect to function U
i
(r
0
, t
0
) with the use of Eq. (2.6),
page 56, we obtain the integral equation
δϕ(r, t)
δU
j
(r
0
, t
0
)
= −
t
Z
t
0
dτ
∂
∂r
i
δU
i
(r, τ )
δU
j
(r
0
, t
0
)
ϕ(r, τ )
−
t
Z
t
0
dτ
∂
∂r
U(r, τ )
δϕ(r, τ )
δU
j
(r
0
, t
0
)
= −θ(t − t
0
)θ(t
0
− t
0
)
∂
∂r
j
δ
r − r
0
ϕ
r, t
0
−
t
Z
t
0
dτ
∂
∂r
U(r, τ )
δϕ(r, τ )
δU
j
(r
0
, t
0
)
, (3.4)
where θ(t) is, as earlier, the Heaviside step function. As a result, we have
δϕ(r, t)
δU
j
(r
0
, t
0
)
∼ θ(t − t
0
)θ(t
0
− t
0
),
and the variational derivative satisfies the condition
δϕ(r, t)
δU
j
(r
0
, t
0
)
= 0, if t
0
< t
0
or t
0
> t,
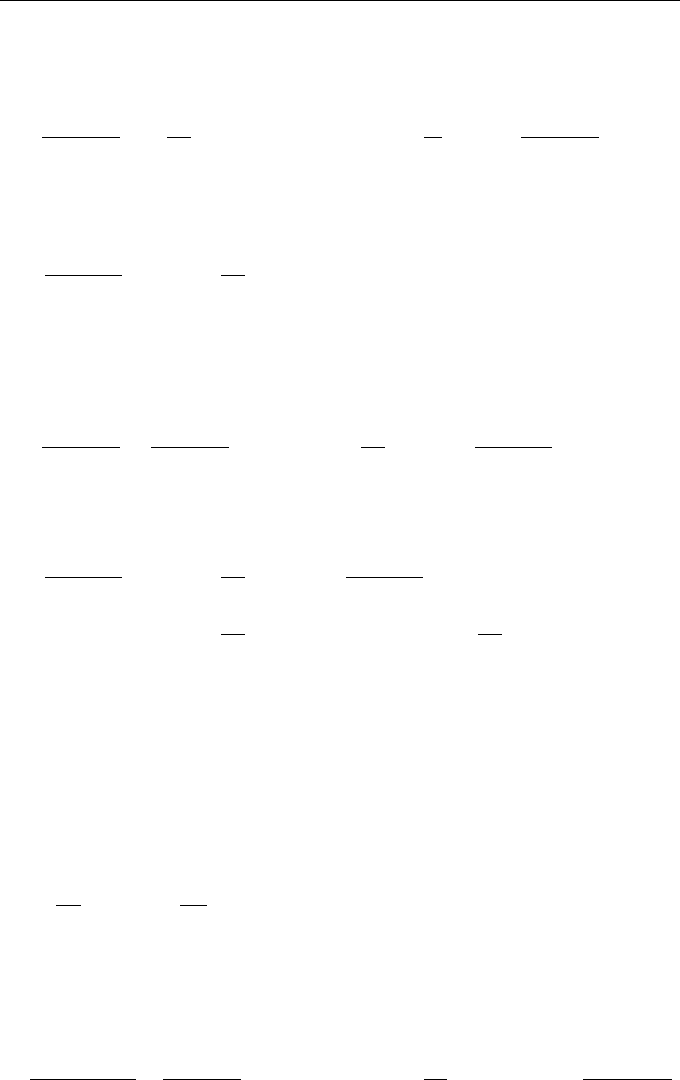
Indicator Function and Liouville Equation 71
which expresses the condition of dynamic causality for the Liouville equation (3.3).
For t
0
< t
0
< t, Eq. (3.4) can be written in the form
δϕ(r, t)
δU
j
(r
0
, t
0
)
= −
∂
∂r
j
δ
r − r
0
ϕ
r, t
0
−
t
Z
t
0
dτ
∂
∂r
U(r, τ )
δϕ(r, τ )
δU
j
(r
0
, t
0
)
, (3.5)
from which follows that
δϕ(r, t)
δU
i
(r
0
, t
0
)
t=t
0
+0
= −
∂
∂r
i
δ
r − r
0
ϕ(r, t
0
)
. (3.6)
Note that equality (3.6) can be derived immediately from Eq. (2.17), page 59.
Indeed, by definition of function ϕ(r, t), variational derivative δϕ(r, t)/δU
i
(r
0
, t
0
) has
the form
δϕ(r, t)
δU
j
(r
0
, t
0
)
=
δ
δU
j
(r
0
, t
0
)
δ(r(t) − r) = −
∂
∂r
i
δ(r(t) − r)
δr
i
(t)
δU
j
(r
0
, t
0
)
. (3.7)
As a consequence, for t = t
0
+ 0, we have the equality
δϕ(r, t)
δU
j
(r
0
, t
0
)
t=t
0
+0
= −
∂
∂r
i
δ(r(t
0
) − r)
δr
i
(t)
δU
j
(r
0
, t
0
)
t=t
0
+0
= −
∂
∂r
j
δ(r(t
0
) − r)δ
r
0
− r
= −
∂
∂r
j
ϕ(r, t
0
)δ
r
0
− r
.
The solution to Eq. (3.1) and, consequently, function (3.2) depends on the initial
values t
0
, r
0
. Indeed, function r(t) = r(t|r
0
, t
0
) as a function of variables r
0
and t
0
satis-
fies the linear first-order partial differential equation (1.105). The equations of such
type also allow the transition to the equations in the indicator function ϕ(r, t|r
0
, t
0
)
(see the next section); in the case under consideration, this equation is again the linear
first-order partial differential equation including the derivatives with respect to vari-
ables r
0
and t
0
∂
∂t
0
+ U(r
0
, t
0
)
∂
∂r
0
ϕ(r, t|r
0
, t
0
) = 0, ϕ(r, t|r
0
, t) = δ(r
0
− r). (3.8)
Equation (3.8) can be called the backward Liouville equation. At the same time, as
a consequence of the dynamic causality, the variational derivative of indicator function
ϕ(r, t|r
0
, t
0
),
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
=
δ
δU
j
(r
0
, t
0
)
δ(r(t|r
0
, t
0
) − r) = −
∂
∂r
i
δ(r(t|r
0
, t
0
) − r)
δr
i
(t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
,

72 Lectures on Dynamics of Stochastic Systems
can be expressed in view of Eq. (2.26), page 61, as follows
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
t
0
=t
0
+0
= −
∂
∂r
i
δ(r(t|r
0
, t
0
) − r)
δr
i
(t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
t
0
=t
0
+0
= −δ(r
0
− r
0
)
∂r
i
(t|r
0
, t
0
)
∂r
0j
∂ϕ(r, t|r
0
, t
0
)
∂r
i
. (3.9)
On the other hand,
∂ϕ(r, t|r
0
, t
0
)
∂r
0j
= −
∂r
i
(t|r
0
, t
0
)
∂r
0j
∂ϕ(r, t|r
0
, t
0
)
∂r
i
,
and Eq. (3.9) assumes the final closed form
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
t
0
=t
0
+0
= δ(r
0
− r
0
)
∂ϕ(r, t|r
0
, t
0
)
∂r
0j
. (3.10)
3.2 First-Order Partial Differential Equations
If the initial-value problem is formulated in terms of partial differential equations, we
always can convert it to the equivalent formulation in terms of the linear variational
differential equation in the infinite-dimensional space (the Hopf equation). For some
particular types of problems, this approach is simplified. Indeed, if the initial dynamic
system satisfies the first-order partial differential equation (either linear as Eq. (1.39),
page 24, or quasilinear as Eq. (1.65), page 33, or in the general case nonlinear as
Eq. (1.84), page 38), then the phase space of the corresponding indicator function
will be the finite-dimension space, which follows from the fact that first-order partial
differential equations are equivalent to systems of ordinary (characteristic) differential
equations. Consider these cases in more detail.
3.2.1 Linear Equations
Backward Liouville Equation
First of all, we consider the derivation of the backward Liouville equation in indicator
function
ϕ(r, t|r
0
, t
0
) = δ
(
r
(
t|r
0
, t
0
)
− r
)
considered as a function of parameters (r
0
, t
0
). Differentiating function ϕ(r, t|r
0
, t
0
)
with respect to t
0
and r
0j
and taking into account Eq. (2.21), page 61, we obtain the
expressions
∂ϕ(r, t|r
0
, t
0
)
∂t
0
=
∂ϕ(r, t|r
0
, t
0
)
∂r
i
U
(
r
0
, t
0
)
∂r
i
(
t|r
0
, t
0
)
∂r
0
,
∂ϕ(r, t|r
0
, t
0
)
∂r
0
= −
∂ϕ(r, t|r
0
, t
0
)
∂r
i
∂r
i
(t|r
0
, t
0
)
∂r
0
.
(3.11)
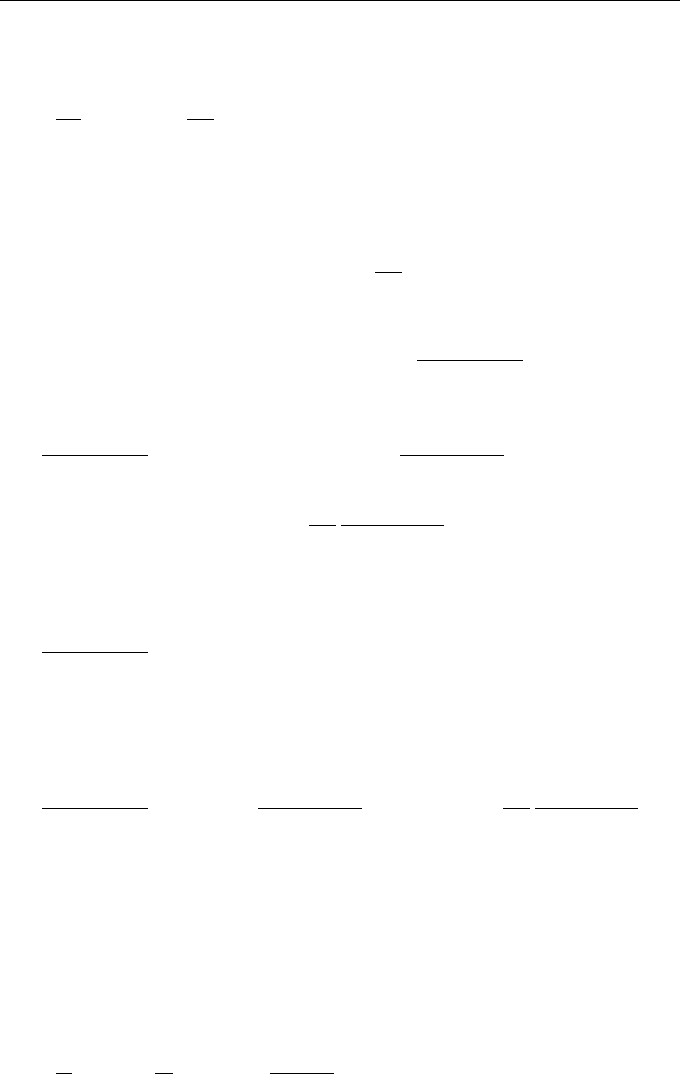
Indicator Function and Liouville Equation 73
Taking scalar product of the second equation in Eq. (3.11) with U
(
r
0
, t
0
)
and summing
the result with the first one, we arrive at the closed equation
∂
∂t
0
+ U
(
r
0
, t
0
)
∂
∂r
0
ϕ(r, t|r
0
, t
0
) = 0, ϕ(r, t|r
0
, t) = δ(r − r
0
), (3.12)
which is just the backward Liouville equation given earlier without derivation.
Rewrite Eq. (3.12) as the linear integral equation
ϕ(r, t|r
0
, t
0
) = δ(r − r
0
) +
t
Z
t
0
dτ U(r
0
, τ )
∂
∂r
0
ϕ(r, t|r
0
, τ ). (3.13)
According to this equation, variational derivative
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
satisfies the linear
integral equation
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
= δ(r
0
− r
0
)θ(t
0
− t
0
)θ(t − t
0
)
∂ϕ(r, t|r
0
, t
0
)
∂r
0j
+
t
Z
t
0
dτ U(r
0
, τ )
∂
∂r
0
δϕ(r, t|r
0
, τ )
δU
j
(r
0
, t
0
)
,
from which follows that
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
∼ θ(t
0
− t
0
)θ(t − t
0
),
i.e., the condition of dynamic causality holds. For t
0
< t
0
< t, this equation is simpli-
fied to the form
δϕ(r, t|r
0
, t
0
)
δU
j
(r
0
, t
0
)
= δ(r
0
− r
0
)
∂ϕ(r, t|r
0
, t
0
)
∂r
0j
+
t
0
Z
t
0
dτ U(r
0
, τ )
∂
∂r
0
δϕ(r, t|r
0
, τ )
δU
j
(r
0
, t
0
)
,
and we arrive, in the limit t
0
= t
0
+ 0, at Eq. (3.10) derived earlier in a different
way.
Density Field of Passive Tracer Under Random Velocity Field
Consider the problem on tracer transfer by random velocity field in more details. The
problem is formulated in terms of Eq. (1.39), page 24, that we rewrite in the form
∂
∂t
+ U(r, t )
∂
∂r
ρ(r, t) +
∂U (r, t)
∂r
ρ(r, t) = 0, ρ(r, 0) = ρ
0
(r). (3.14)
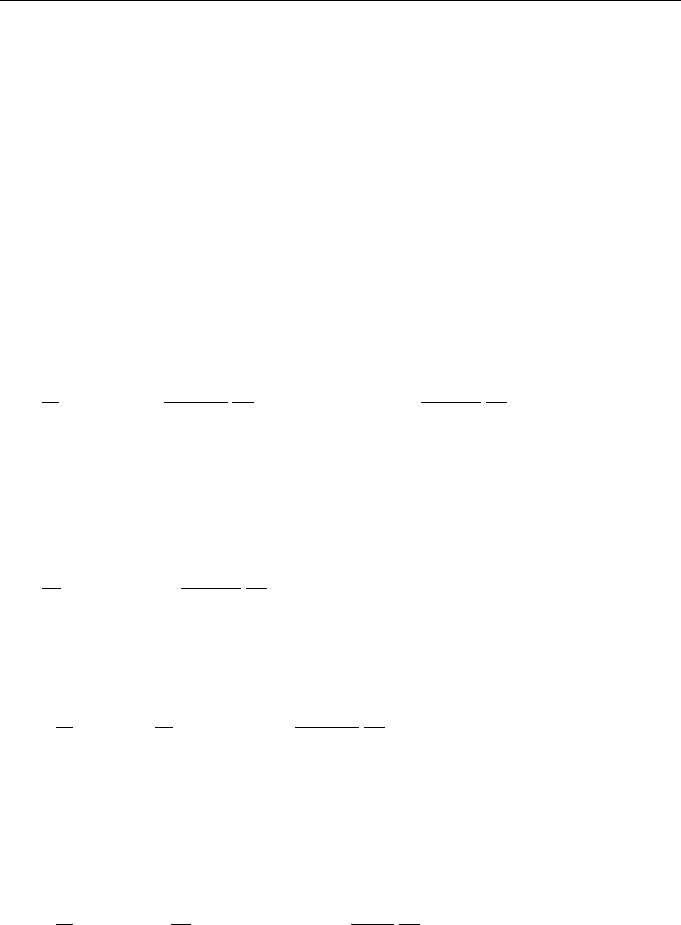
74 Lectures on Dynamics of Stochastic Systems
To describe the density field in the Eulerian description, we introduce the indicator
function
ϕ(r, t; ρ) = δ(ρ(t, r) − ρ), (3.15)
which is similar to function (3.2) and is localized on surface
ρ(r, t) = ρ = const,
in the three-dimensional case or on a contour in the two-dimensional case. An equation
for this function can be easily obtained either immediately from Eq. (3.14), or from
the Liouville equation in the Lagrangian description. Indeed, differentiating Eq. (3.15)
with respect to time t and using dynamic equation (3.14) and probing property of the
delta-function, we obtain the equation
∂
∂t
ϕ(r, t; ρ) =
∂U (r, t)
∂r
∂
∂ρ
ρϕ(r, t; ρ) + U(r, t)
∂ρ(r, t)
∂r
∂
∂ρ
ϕ(r, t; ρ). (3.16)
However, this equation is unclosed because the right-hand side includes term
∂ρ(r, t)/∂r that cannot be explicitly expressed through ρ(r, t).
On the other hand, differentiating function (3.15) with respect to r, we obtain the
equality
∂
∂r
ϕ(r, t; ρ) = −
∂ρ(r, t)
∂r
∂
∂ρ
ϕ(r, t; ρ). (3.17)
Eliminating now the last term in Eq. (3.16) with the use of (3.17), we obtain the closed
Liouville equation in the Eulerian description
∂
∂t
+ U(r, t )
∂
∂r
ϕ(r, t; ρ) =
∂U (r, t)
∂r
∂
∂ρ
[
ρϕ(r, t; ρ)
]
,
ϕ(r, 0; ρ) = δ(ρ
0
(r) − ρ).
(3.18)
Note that the Liouville equation (3.18) becomes the one-dimensional equation
in the case of velocity field described by the simplest model Eq. (1.15), page 10,
U(x, t) = v(t)f (x) (x is the dimensionless variable),
∂
∂t
+ v
x
(t)f (x)
∂
∂x
ϕ(x, t; ρ) = v
x
(t)
∂f (x)
∂x
∂
∂ρ
[
ρϕ(x, t; ρ)
]
,
ϕ(x, 0; ρ) = δ(ρ
0
(x) − ρ).
(3.19)
To obtain more complete description, we consider the extended indicator function
including both density field ρ(r, t) and its spatial gradient p(r, t) = ∇ρ(r, t)
ϕ(r, t; ρ, p) = δ
(
ρ(r, t) − ρ
)
δ
(
p(r, t) − p
)
. (3.20)
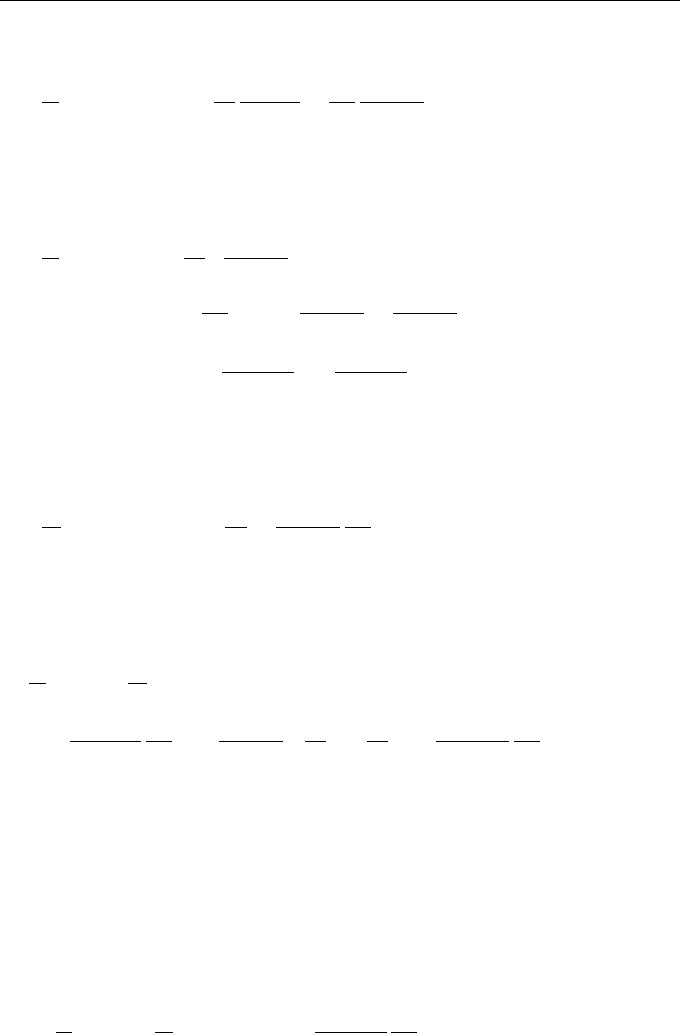
Indicator Function and Liouville Equation 75
Differentiating Eq. (3.20) with respect to time, we obtain the equality
∂
∂t
ϕ(r, t; ρ, p) = −
∂
∂ρ
∂ρ(r, t)
∂t
+
∂
∂p
i
∂p
i
(r, t)
∂t
ϕ(r, t; ρ, p). (3.21)
Using now dynamic equation (3.14) for density and Eq. (1.50), page 28 for the density
spatial gradient, we can rewrite Eq. (3.21) as the equation
∂
∂t
ϕ(r, t; ρ, p) =
∂
∂ρ
∂U (r, t)
∂r
ρ + U(r, t)p
ϕ(r, t; ρ, p)
+
∂
∂p
i
U(r, t)
∂p
i
(r, t)
∂r
+
∂U (r, t)
∂r
p
i
+ p
k
∂U
k
(r, t)
∂r
i
+ ρ
∂
2
U(r, t)
∂r
i
∂r
ϕ(r, t; ρ, p), (3.22)
which is unclosed because of the term ∂p
i
(r, t)/∂r in the right-hand side.
Differentiating function (3.20) with respect to r, we obtain the equality
∂
∂r
ϕ(r, t; ρ, p) = −
p
∂
∂ρ
+
∂p
i
(r, t)
∂r
∂
∂p
i
ϕ(r, t; ρ, p). (3.23)
Now, multiplying Eq. (3.23) by U(r, t) and adding the result to Eq. (3.22), we obtain
the closed Liouville equation for the extended indicator function
∂
∂t
+ U(r, t )
∂
∂r
ϕ(r, t; ρ, p)
=
∂U
k
(r, t)
∂r
i
∂
∂p
i
p
k
+
∂U (r, t)
∂r
∂
∂ρ
ρ +
∂
∂p
p
+
∂
2
U(r, t)
∂r
i
∂r
∂
∂p
i
ρ
× ϕ(r, t; ρ, p)
(3.24)
with the initial value
ϕ(r, 0; ρ, p) = δ
(
ρ
0
(r) − ρ
)
δ
p
0
(r) − p
.
In the case of nondivergent velocity field (div U(r, t) = 0), the Liouville equa-
tion (3.24) is simplified to the form
∂
∂t
+ U(r, t )
∂
∂r
ϕ(r, t; ρ, p) =
∂U
k
(r, t)
∂r
i
∂
∂p
i
p
k
ϕ(r, t; ρ, p),
ϕ(r, 0; ρ, p) = δ
(
ρ
0
(r) − ρ
)
δ
p
0
(r) − p
.
(3.25)
