Jackson M.J. Micro and Nanomanufacturing
Подождите немного. Документ загружается.


Diamond Nanogrinding 573
breakdown was reached. The initial trial wheels used were: angular
white alumina wheel with low temperature bonding system (Wheel
specification: 77A60JV); sol-gel and angular white abrasive mixture
wheel with low temperature bonding system (Wheel specification:
73A60JV); monocrystalline alumina grinding wheel with a low tem-
perature bonding system (Wheel specification: SA60JV); and laser
dressed chromium-doped alumina bonded with a high temperature
bonding system. All grinding wheels were manufactured with a vit-
rified bond, 60 mesh-size abrasive (approximately 220 |um in diame-
ter),
J- mechanical grade, and a fairly open structure. Experiments
were performed on a Jones and Shipman series 10 cylindrical grind-
ing machine using a 450 mm diameter grinding wheel rotating at 33
m/s surface speed. The mechanically-dressed wheels were dressed
using a D25 blade tool supplied by Unicorn-Van Moppes, and the
dressing conditions were: 3 passes at 0.15 mm/rev at a depth of
0.03mm plus one pass at 0.015 mm depth at the start of each batch.
Stock removal was 0.25 mm on diameter grinding AISI 52100 ball
bearing steel (59 HRC) using 2% concentration soluble oil at 0.5 bar
pressure and a flow rate of 15 liters/minute. Forty experimental test
pieces were ground per experiment. The laser dressed samples were
mounted in a modified grinding wheel that holds segments of sam-
ples that have been laser dressed. The segmented grinding wheel
acts in the same way as a conventional grinding wheel but has the
advantages of quick and easy replacement of laser-processed sam-
ples.
The most important measurements made during the grinding
experiments are grinding power, surface roughness of the work-
piece, and the wheel wear parameter, grinding ratio, which is the ra-
tio of workpiece machined to the amount of grinding wheel lost dur-
ing grinding.
10.7.2 Experimental Results and Discussion
Laser Dressing Temperatures
The rapid solidification of the laser dressed samples show that a re-
fined microstructure and reduced segregation of phases is possible.
Very high cooling rates are possible over a small region of the
workpiece where non-equilibrium structures are produced owing to

574 Micro-and Nanomanufacturing
rapid solidification of the material. High cooling rates can be
achieved using a laser beam that tends to diminish as the laser beam
moves along the track. Therefore, the microstructure will change at
the edges of the track toward the unaffected part of the grinding
wheel. Laser irradiation is considered to be a point source with heat
energy flowing radially away from the point. The system is as-
sumed to attain quasi-steady-state conditions when the melt velocity
at the melt/solid interface becomes constant [35]. Incident radiation
is partly reflected and absorbed by the abrasive material, whilst
some absorbed energy is lost by re-radiation and convection and
some is conducted into the material. The melt zone is heated from
the surface at the center of the track, which established convection
currents within the melt pool and transports heat away very quickly
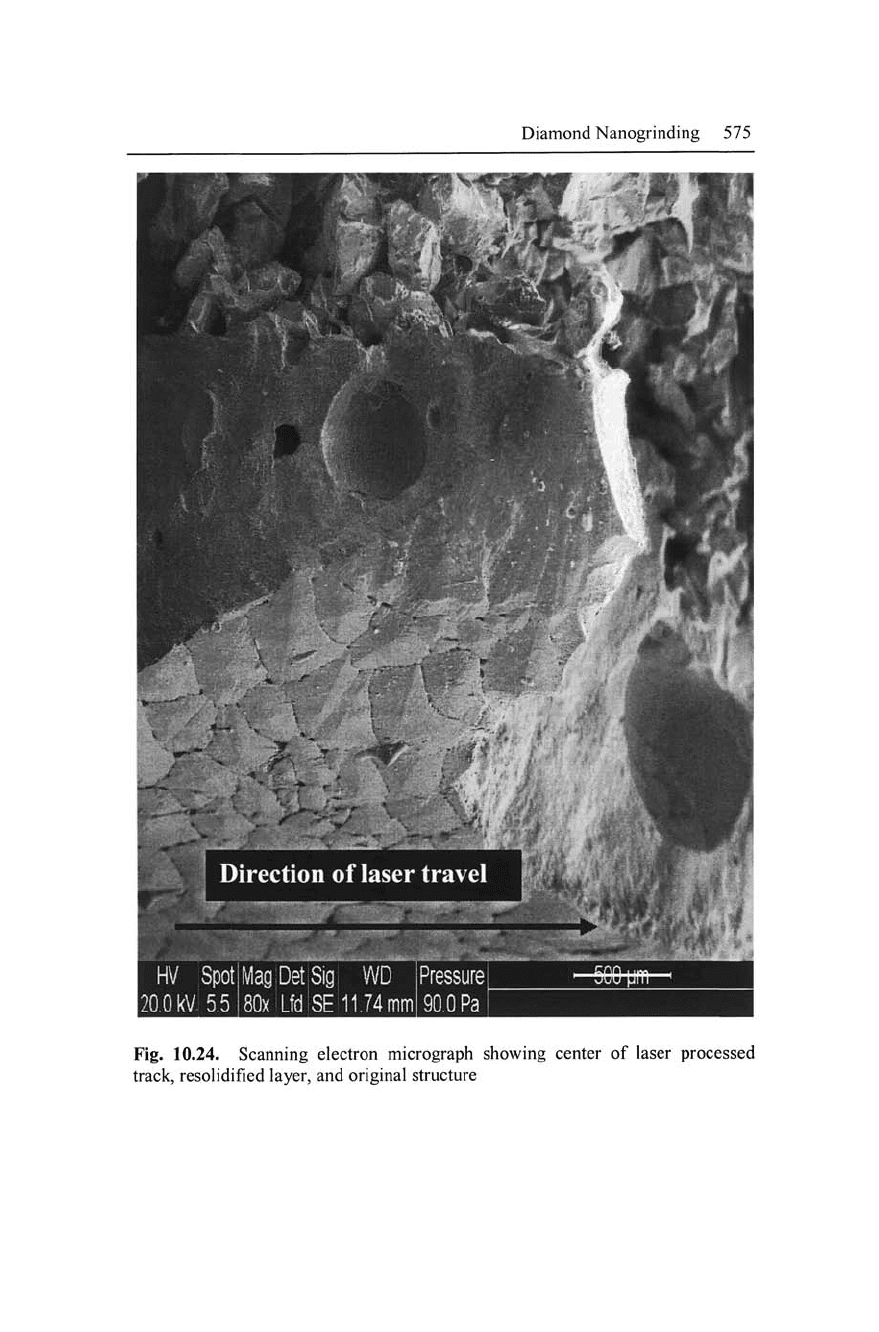
from the melt/solid interface (Figure 10.24).
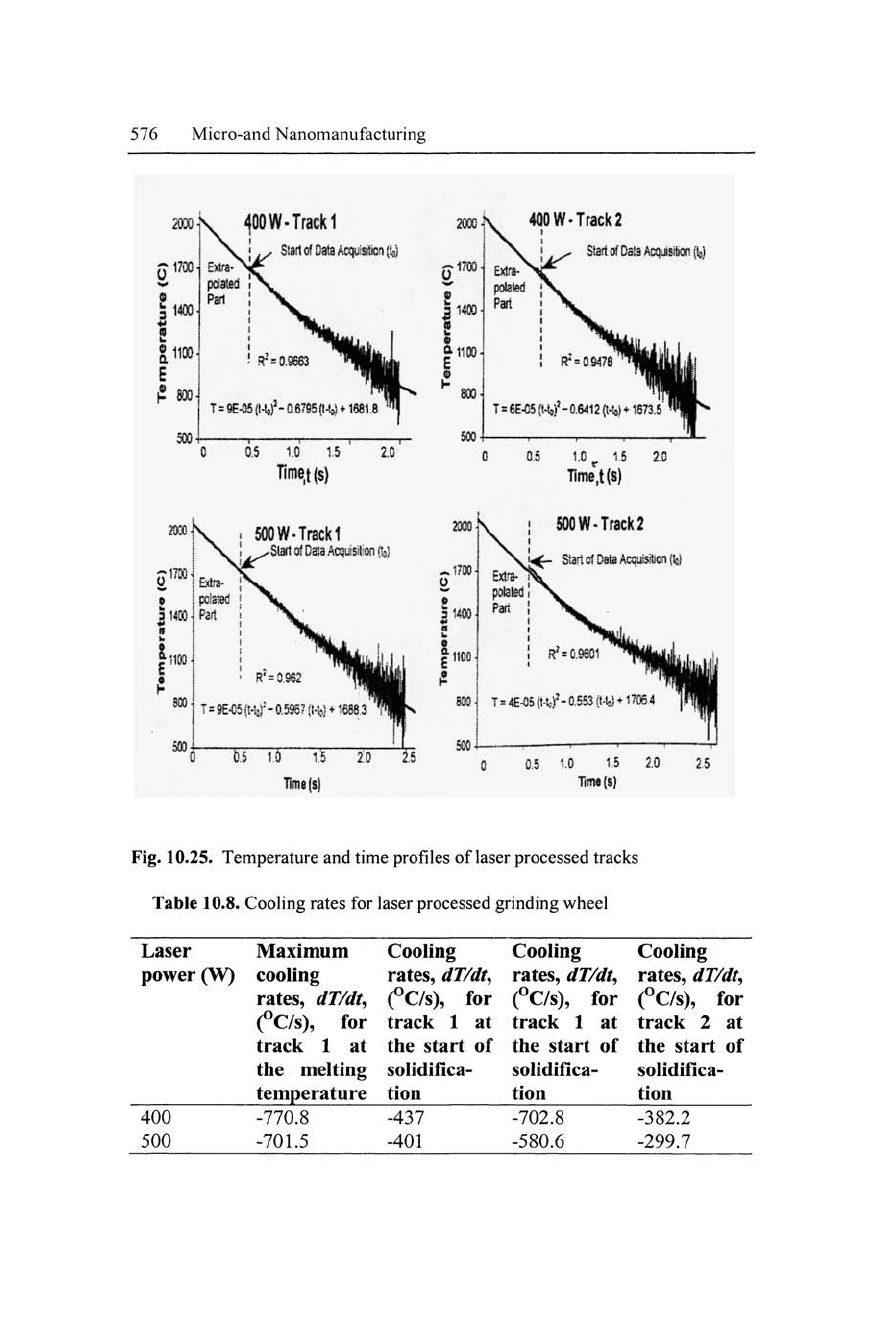
Experimentally determined cooling rates during laser proc-
essing for processing at two different power levels (400 W and 500
W) are shown in Table 10.8. The data are calculated from tempera-
ture and time data collected using the two-color pyrometer. The
data is obtained by curve fitting the time dependent equation for the
processing temperature so that cooling rates can be estimated close
to the melting point of the abrasive material. Experimental data is
provided for two tracks and cooling rates are calculated at the melt-
ing temperature of the abrasive material (2045 K), which is assumed
to be the maximum cooling rate (Figure 10.25). The cooling rates in
samples processed at higher laser power are lower compared to that
at lower laser power. This is explained by the fact that the abrasive
material is a poor conductor of heat compared to metals. Therefore,
an increase in laser beam power will provide a greater input of heat
to the material that will provide a larger melt zone and an increase in
the interaction volume. Thus, it takes more time to dissipate the heat
built-up and causes a drop in cooling rates. Therefore, for high laser
power the cooling rate is expected to be lower compared to those
calculated for 400 W and 500 W.
Diamond Nanogrinding 575
Direction of laser travel
HV Spot Mag DetSig
WD
Pressur.
20.0kVl 5.5
80x
Lid SE 11.74
mm
90.0 Pa
Fig. 10.24. Scanning electron micrograph showing center of laser processed
track, resolidified layer, and original structure
576 Micro-and Nanomanufacturing
£1700
400 W- Track 1
SlartofCataAcqiiisiSonlWi
4Q0W-Track2
I
Start of Dais Acquisition (to)
0.5
1.0 1.5
Timet (s)
0.5
tjD
r
15 2.0
Time,t(s)
!
M0W-Track2
i^- Start cf Data Acquisition tic)
Fig. 10.25. Temperature and time profiles of laser processed tracks
Table 10.8. Cooling rates for laser processed grinding wheel
Laser Maximum Cooling Cooling Cooling
power (W) cooling rates, dT/dt, rates, dT/dt, rates, dT/dt,
rates,
dT/dt, (°C/s), for (°C/s), for (°C/s), for
(°C/s),
for track 1 at track 1 at track 2 at
track 1 at the start of the start of the start of
the melting solidifica- solidifica- solidiflca-
temperature tion tion tion
400
500
-770.8
-701.5
-437
-401
-702.8
-580.6
-382.2
-299.7

Diamond Nanogrinding 577
The cooling rate in the second track is slightly lower than
track 1 for both 400 W and 500 W samples. As the laser passes the
over the first track it pre-heats the material adjacent to it that results
in a reduced cooling rate for the second track owing to the decrease
in the thermal gradient. Table 10.8 shows the cooling rate at 2.2 s
after solidification, which corresponds to the temperature of the
sample near to the lower calibrated temperature range of the py-
rometer. The trend observed shows that the cooling rate is similar to
that seen at the solidification temperature, but cooling rates are much
lower due to thermal gradients and heat loss from the sample. So-
lidification occurs in directions that are opposite to the direction of
heat extraction. It is also assumed that grain growth is equal in all
directions owing to the fact that the directed laser energy is a point
source of heat dissipation. Microstructural texture is assumed to
form in accordance with the model of heat extraction. A full range
of microstructures such as planar, cellular, and dendritic, are ob-
served in laser processed samples [36-37].
A planar solidification front is observed when the interface is
at the equilibrium liquidus temperature and when every point at the
front of the interface is above the liquidus temperature. Any insta-
bility dissolves back in the melt and if it is supercooled, then the per-
turbation formed on the interface will grow fast into the liquid melt.
Under the influence of a positive temperature gradient, constitutional
supercooling is solute driven. The type of solidification microstruc-
ture formed during constitutional supercooling depends on the G/R
ratio,
where G is the thermal gradient and R is the solidification rate
[36-37].
The solidification structure observed in the laser-processed
tracks of the abrsive material changes the surface morphology. Re-
ferring to Figure 10.26, grains are multifaceted at the center of the
laser track and are equiaxed possessing a dendritic structure. The
edges of the tracks show elongated columnar grains that grow away
from the point source in a direction normal to the laser beam. The
columnar structures are similar to those reported by other authors
[38-40] who have conducted laser based processing of alumina ce-
ramics.
The heat transfer equation for a moving heat source is given
by the following equation, where the substantial derivative of tem-
perature, i.e., T= T
(y,
t), and is given a partial derivative [41-44],

578 Micro-and Nanomanufacturing
d
r
, ,. dT dy dT d
2
T q
—[t(y
9
t)] = — + —.— = D—- + ~^—
dt dt dt dy dy
1
Co
(10.55)
For the time-independent situation, i.e., quasi-steady state heat
transfer for a moving boundary problem, the time derivative tends to
zero in Equation 10.55. Hence, the surface cooling rate dT/dt in the
direction opposite to the laser under the quasi-steady state (T/t=0)
can be estimated as [43],
dT _ dT dy
dt dy dt
G =
1
*L
v' dt
(10.56)
Where v is the velocity of the laser beam.
Direction of laser travel
HV ISpotlMagDetlSigl WD IPressurej
20.0 kVi 5.5 150x Lfd SE 11.08
mm
90.0
Pa
f
Fig. 10.26. SEM image showing equiaxed grains with dendritic structure in the
center of the track (A) and columnar growth of grains near the edge of the track
(B)

Diamond Nanogrinding
579
Thus,
the
thermal gradients
(G) are
calculated from
the
tempera-
ture and time diagrams assuming quasi-steady state conditions using
Eqn. 10.56. During solidification, the velocity
of
the liquid/solid
in-
terface
or
growth rate,
i?, is
provided
by the
Neumann solutions
to
the Stefan problem [44]. Assumptions made include: strong convec-
tive currents exist
in the
melt pool
and the
total energy absorbed
at
the free surface
of
the liquid
is
transported
to the
interface
by
con-
vection. The solution to the Stefan problem is,
V
iP
AH
m
-K
M
.2f-
=
V
iP
[*H
m
+C
p
(T
m
-T
0
)]
=
I
a
(10.57)
dy
Where
Vi is the
liquid/solid interface velocity,
and y is the
dis-
tance
in
the melt pool. This equation gives
the
average velocity
of
the interface during the time that the treated zone solidifies. The
so-
lidification front velocity
at
the start
of
solidification
is
greater than
Eqn. 10.57 estimates. Therefore,
at
the start
of
solidification the
lo-
cal temperature gradient
is
very large
and
this leads
to a
maximum
interface solidification velocity that is approximated
by
[45]:
V™
x
* d
^
\
T
U
(10.58)
Where
TI
is
the dwell time of the laser and
£
is provided by the so-
lution
of,
g[\
+
erf(g)]ex
V
(g
2
) =
Cp
j-
m
~J
o)
(10.59)
It can
be
shown from Eqn. 10.59 that
the
average
and
maximum
interface solidification rates
are
dependent
on
laser beam intensity
and velocity.
By
using Equations 10.57
and
10.58,
the
velocity
of
the solid/liquid interface,
or
growth rate (i?),
is
calculated
at
the cen-
ter
of the
track.
G/R
ratios
are
calculated
and are
given
in
Table
10.9.
The ratios
are
high near
to the
edge
of
the track where solidi-
fication starts and decreases toward the center of the track.

580 Micro-and Nanomanufacturing
Table 10.9. G/R ratios for laser
Track
tion
Center
Edge
3
posi-
G/R ratio for
laser power
of 400W:
Track 1
4.43 x 10
6
1.74 xlO
9
processing parameters
G/R ratio for
laser power
of 400W:
Track 2
4.04 xlO
6
1.74 xlO
9
G/R ratio for
laser power
of 500W:
Track 1
4.03 xlO
6
1.74 xlO
9
G/R ratio for
laser power
of 500W:
Track 2
3.34 xlO
6
1.74 xlO
9
a
G/R ratios are the same for all conditions at the edge of the track because
Eqn.
10.58 is used for calculating the growth rate, R, which is independent ofG.
The observed solidification structure varied from equiaxed grains
with dendritic structure within the track to columnar grains at the
edges of the track. Constitutional supercooling theory predicts that
increasing the G/R ratio causes a progressive change in solidification
structure from fully dendritic to cellular dendritic, then to cellular
and then finally to a planar morphology. A similar trend is observed
in the laser track. Therefore, the observed microstructure agrees
with the predictions made by theory.
Orientation Imaging Microscopy of Laser-Dressed Materials
Figure 10.30 shows the image quality (IQ) micrograph obtained
from the OIM. The area selected for analysis is of a cross-section of
the re-solidified layer of one of the tracks. IQ represents the sharp
character of the EBSD pattern and thus local crystal perfection.
Hence, the IQ represents the real microstructure as demonstrated in
an optical microscope.
The dark regions of the image are pores where the IQ intensity is
zero.
Grain boundaries are easily detected, which is in sharp con-
trast to a scanning electron microscopic image that does not resolve
grain boundaries at that particular magnification. The growth direc-
tion is radial from the surface of the center of the track where the
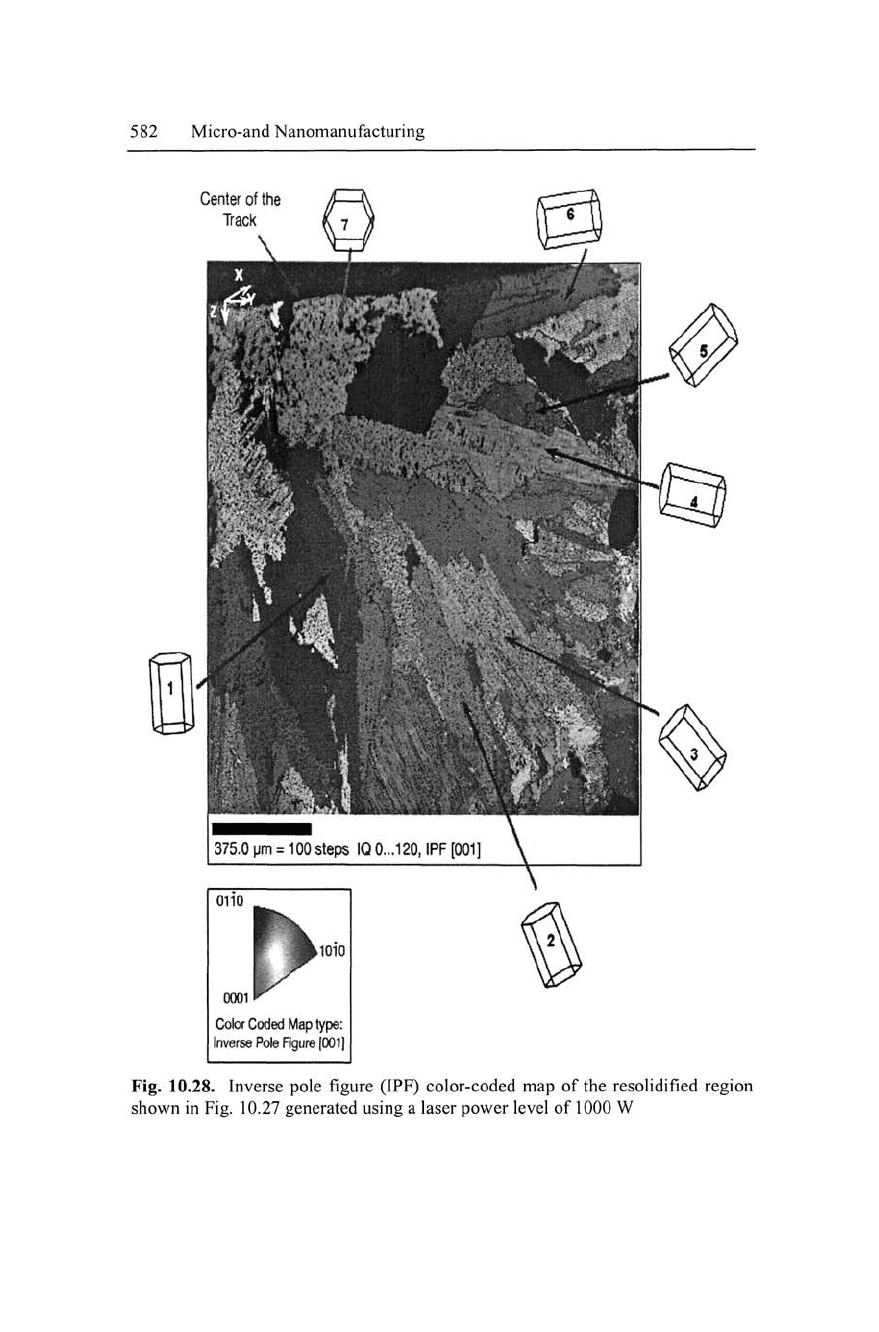
beam is focused. Figure 10.28 shows the inverse pole figure (IPF)
map of the area shown in Figure 10.27.

Diamond Nanogrinding 581
Fig. 10.27. Image quality cross section of the laser processed track showing the
re-solidified region laser dressed at 1000 W using OIM
The grains are color-coded that represents the crystallographic
orientation of the normal surface. The key used for color-coding is
provided in the stereographic triangle at the base of the figure. The
orientation of some of the grains are in the form of the hexagonal
cell for a-alumina. It is observed from the figure that growth takes
place along the c-axis in the hexagonal a-alumina lattice during so-
lidification of the molten abrasive bonding material. Grains num-
bered 1-4 possess an orientation that extends radially away from the
center of the track as shown by the heat extraction model.
These grains lie within the re-solidified layer, whilst the outer
grains lie parallel to the surface of the track. There are smaller
grains that have their c-axes oriented differently than other smaller
grains and not along the direction radially outward from the center
of the laser-processed track.
582 Micro-and Nanomanufacturing
00011
Color Coded Map type:
Inverse Pole Figure [0011
Fig. 10.28. Inverse pole figure (IPF) color-coded map of the resolidified region
shown in Fig. 10.27 generated using a laser power level of 1000 W
