Hungerford T.W., Shaw D.J. Contemporary Precalculus: A Graphing Approach
Подождите немного. Документ загружается.


(b) 3 log
5
(125x)
3 (log
5
125 log
5
x) [Product Law]
3 log
5
125 log
5
x
3 3 log
5
x [log
5
125 3 because 5
3
125]
log
5
x
log
5
x
1
log
5
1
x
[Power Law] ■
For graphic illustrations of the errors mentioned in the Caution, see Exercises 82
and 83.
EXAMPLE 8
Given that
log
7
2 .3562, log
7
3 .5646, and log
7
5 .8271,
find:
(a) log
7
10; (b) log
7
2.5; (c) log
7
48.
SOLUTION
(a) By the Product Law,
log
7
10 log
7
(2
5) log
7
2 log
7
5 .3562 .8271 1.1833.
(b) By the Quotient Law,
log
7
2.5 log
7
5
2
log
7
5 log
7
2 .8271 .3562 .4709.
(c) By the Product and Power Laws,
log
7
48 log
7
(3
16) log
7
3 log
7
16 log
7
3 log
7
2
4
log
7
3 4
log
7
2 .5646 4(.3562)
1.9894. ■
Example 8 worked because we were given several logarithms to base 7. But
there’s no log
7
key on the calculator, so how do you find logarithms to base 7 or
396 CHAPTER 5 Exponential and Logarithmic Functions
CAUTION
1. A common error in using the Product Law is to write something like log 6 log 7
log (6 7) log 13 instead of the correct statement log 6 log 7 log (6
7) log 42.
2. Do not confuse log
b
w
v
with the quotient
l
l
o
o
g
g
b
b
w
v
. They are different numbers. For example,
when b 10
log
4
4
8
log 12 1.0792 but
lo
lo
g
g
4
4
8
1
0
.
.
6
6
8
0
1
2
2
1
2.7922.

to any base other than e or 10? Answer: Use the LN key on the calculator and the
following formula.
Proof By Property 4 in the box on page 394, b
log
b
v
v. Take the natural log-
arithm of each side of this equation:
ln (b
log
b
v
) ln v.
Apply the Power Law for natural logarithms on the left side.
(log
b
v)(ln b) ln v.
Dividing both sides by ln b finishes the proof.
log
b
v
l
l
n
n
b
v
. ■
EXAMPLE 9
To find log
7
3, apply the change of base formula with b 7.
log
7
3
l
l
n
n
3
7
1
1
.
.
0
9
9
4
8
5
6
9
.5646. ■
SPECIAL TOPICS 5.4.A Logarithmic Functions to Other Bases 397
Change of
Base Formula
For any positive numbers b and v,
log
b
v
l
l
n
n
b
v
.
EXERCISES 5.4.A
Note: Unless stated otherwise, all letters represent positive
numbers and b 1.
In Exercises 1–8, fill in the missing entries in each table.
1.
2.
3.
4.
5.
6.
7.
8.
In Exercises 9–18, translate the given exponential statement
into an equivalent logarithmic one.
9. 10
2
.01 10. 10
4
10,000
11.
3
10 10
1/3
12. 10
0.6990
5
13. 10
7k
r 14. 10
(2ab)
c
15. 7
8
5,764,801 16. 5
3
1/125
17. 3
3
1/9 18. k
27
10,134
x 0124
f (x) log
4
x
x 1/e
2
1 ee
3
k(x) 3 ln x
x 01/7 7
49
f (x) 2 log
7
x
x .001 10
g(x) 2 log x 2 4
x 1/36 6 36 6
g(x) log
6
x
x 1/6 1 216
h(x) log
6
x 2
x 4 3
7
8
412
k(x) log
2
(x 4)
x 2.75 11 29
h(x) 3 log
2
(x 3)
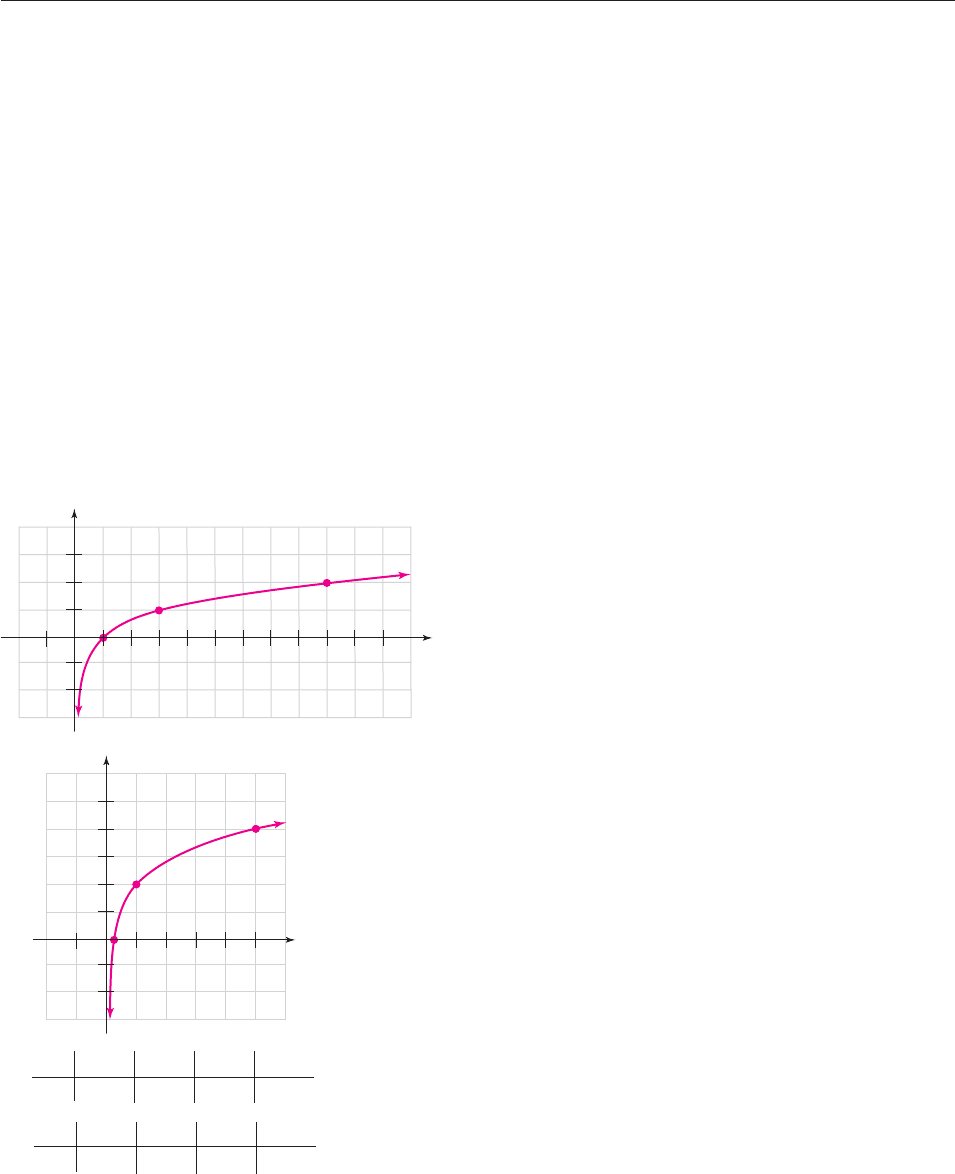
In Exercises 19–28, translate the given logarithmic statement
into an equivalent exponential one.
19. log 10,000 4 20. log .0001 4
21. log 750 2.88 22. log (.69) .1612
23. log
5
125 3 24. log
25
(1/125) 3/2
25. log
2
(1/4) 2 26. log
5
4
5
1/4
27. log (x
2
2y) z w 28. log (p q) r
In Exercises 29–36, evaluate the given expression without
using a calculator.
29. log 10
97
30. log
13
(13
2
)
31. log 10
x
2
y
2
32. log
2.7
[2.7
(3x
2
1)
]
33. log
16
4 34. log
3
81
35. log
3
(27) 36. log
3
2
(1/16)
In Exercises 37–40, a graph or a table of values for the func-
tion f(x) log
b
x is given. Find b.
37.
38.
39.
40.
In Exercises 41–46, find x.
41. log
3
243 x 42. log
243
81
43. log
27
x 1/3 44. log
2
x 6
2
5 10 15 20 25
1
3
4
5
−5
−2
−1
x
y
2
2
4
6 8 9 10 11
1
3
5 7
1
3
−1
−2
−1
x
y
398 CHAPTER 5 Exponential and Logarithmic Functions
45. log
x
64 3 46. log
x
(1/64) 3/4
In Exercises 47–60, write the given expression as the logarithm
of a single quantity, as in Example 7.
47. 2 log x 3 log y 6 log z
48. 2 log
5
x 7 log
5
y 3 log
5
(2z)
49. log x log (x 3) log (x
2
9)
50. log
4
(z
2
7z) log
4
(z 4) log
4
(2z)
51.
1
2
log
2
(25c
2
) 52.
1
4
log
6
(16p
12
)
53. 2 log
4
(7c) 54.
2
3
log
4
(3x 2)
55. 2 ln (x 1) ln (x 2)
56. 5 ln (2x 1) 2 ln (3x 5)
57. log
2
(2x) 1
58. 3 log
6
(36y)
59. 2 ln (e
2
e) 2
60. 3 2 log
4
(10)
In Exercises 61–68, use a calculator and the change of base
formula to find the logarithm.
61. log
2
10 62. log
3
18 63. log
7
5
64. log
16
27 65. log
500
1000 66. log
8
3
67. log
12
56 68. log
12
5
In Exercises 69–74, answer true or false and give reasons for
your answer.
69. log
b
(r/5) log
b
r log
b
5
70. log
2
(3p q) log
2
(3p) log
2
q
71. (log
b
r)/t log
b
(r
1/t
)
72. log
a
(3bc) log
a
(3b) log
a
c
73. log
5
(5x) 5(log
5
x)
74. 1 2 log t log 3 log (30t
2
)
THINKERS
75. Which is larger: 397
398
or 398
397
? [Hint: log 397 2.5988
and log 398 2.5999 and f (x) 10
x
is an increasing
function.]
76. If log
b
9.21 7.4 and log
b
359.62 19.61, then what is
log
b
359.62/log
b
9.21?
In Exercises 77–80, assume that a and b are positive, with
a 1 and b 1.
77. Express log
b
u in terms of logarithms to the base a.
78. Show that log
b
a 1/log
a
b.
79. How are log
10
u and log
100
u related?
x .05 1 400 2 5
f (x) 10 21/2
x 1/25 1 5 125
f (x) 40 2 6

80. Show that a
log b
b
log a
.
81. If log
b
x
1
2
log
b
v 3, show that x (b
3
)v
.
82. Graph the functions f (x) log x log 7 and g(x)
log (x 7) on the same screen. For what values of x is
it true that f (x) g(x)? What do you conclude about the
statement
log 6 log 7 log (6 7)?
83. Graph the functions f (x) log (x/4) and g(x)
(log x)/(log 4). Are they the same? What does this say
about a statement such as
log
4
4
8
l
l
o
o
g
g
4
4
8
?
SECTION 5.5 Algebraic Solutions of Exponential and Logarithmic Equations 399
In Exercises 84–86, sketch a complete graph of the function,
labeling any holes, asymptotes, or local extrema.
84. f (x) log
5
x 2 85. h(x) x log x
2
86. g(x) log
20
x
2
87. The number 10
100
(The number 1 followed by one-hundred
zeros) is called a googol.
(a) It is a fact that log
5
(10
100
) 143.0677. Is 5
150
greater or
smaller than one googol? How do you know?
(b) Which is bigger, 2
3322
or (googol)
10
(one googol to the
tenth power)?
88. Assume a and b are constants with a 1 and b 1. For a
particular positive number x we know that log
a
x log
b
x.
Is it possible to tell if a b or if b a? Why or why not?
5.5 Algebraic Solutions of Exponential and Logarithmic Equations
■ Solve exponential and logarithmic equations algebraically.
■ Use exponential and logarithmic equations to solve applied
problems.
Most of the exponential and logarithmic equations solved by graphical means
earlier in this chapter could also have been solved algebraically. The algebraic
techniques for solving such equations are based on the properties of logarithms.
EXPONENTIAL EQUATIONS
The easiest exponential equations to solve are those in which both sides are pow-
ers of the same base.
EXAMPLE 1
Solve 8
x
2
x1
.
SOLUTION Using the fact that 8 2
3
, we rewrite the equation as follows.
8
x
2
x1
(2
3
)
x
2
x1
2
3x
2
x1
Since the powers of 2 are equal, the exponents must be the same, that is,
3x x 1
2x 1
x
1
2
. ■
When different bases are involved in an exponential equation, a different
solution technique is needed.
Section Objectives
EXAMPLE 2
Solve 5
x
2.
SOLUTION
Take logarithms on each side:* ln 5
x
ln 2
Use the Power Law: x (ln 5) ln 2
Divide both sides by ln 5: x
l
l
n
n
2
5
1
.6
.6
9
0
3
9
1
4
.4307.
Remember:
l
l
n
n
2
5
is neither ln
2
5
nor ln 2 ln 5. ■
EXAMPLE 3
Solve 2
4x1
3
1x
,
SOLUTION
Take logarithms of each side: ln 2
4x1
ln 3
1x
Use the Power Law: (4x 1)(ln 2) (1 x)(ln 3)
Multiply out both sides: 4x(ln 2) ln 2 ln 3 x(ln 3)
Rearrange terms: 4x(ln 2) x(ln 3) ln 2 ln 3
Factor left side: (4
ln 2 ln 3)x ln 2 ln 3
Divide both sides by
(4
ln 2 ln 3): x .4628. ■
APPLICATIONS OF EXPONENTIAL EQUATIONS
As we saw in Section 5.2, the mass of a radioactive element at time x is given by
M(x) c(.5
x/h
),
where c is the initial mass and h is the half-life of the element.
EXAMPLE 4
After 43 years, a 20-milligram sample of strontium-90 (
90
Sr) decays to 6.071 mg.
What is the half-life of strontium-90?
SOLUTION The mass of the sample at time x is given by
f (x) 20(.5
x/h
),
ln 2 ln 3
4
ln 2 ln 3
400 CHAPTER 5 Exponential and Logarithmic Functions
*We shall use natural logarithms, but the same techniques are valid for logarithms to other bases
(Exercise 34).
where h is the half-life of strontium-90. We know that f (x) 6.071 when x 43,
that is, 6.071 20(.5
43/h
). We must solve this equation for h.
Divide both sides by 20:
6.
2
0
0
71
.5
43/h
Take logarithms on both sides: ln
6.
2
0
0
71
ln .5
43/h
Use the Power Law: ln
6.
2
0
0
71
4
h
3
ln .5
Multiply both sides by h: h ln
6.
2
0
0
71
43 ln .5
Divide both sides by ln
6.
2
0
0
71
: h
ln(6
4
.
3
07
ln
1/
.5
20)
25.
Therefore, strontium-90 has a half-life of 25 years. ■
EXAMPLE 5
When a living organism dies, its carbon-14 decays. The half-life of carbon-14 is
5730 years. If the skeleton of a mastodon has lost 58% of its original carbon-14,
when did the mastodon die?*
SOLUTION Time is measured from the death of the mastodon. The amount of
carbon-14 left in the skeleton at time x is given by
M(x) c(.5
x/5730
),
where c is the original mass of carbon-14. The skeleton has lost 58% of c, that is,
.58c. So the present value of M(x) is c .58c .42c, and we have
M(x) c(.5
x/5730
)
.42c c(.5
x/5730
)
.42 .5
x/5730
.
The solution of this equation is the time elapsed from the mastodon’s death to the
present. It can be solved as above.
ln .42 ln (.5)
x/5730
ln .42
57
x
30
(ln .5)
5730(ln .42) x(ln .5)
x
5730
ln
(ln
.5
.42)
7171.32.
Therefore, the mastodon died approximately 7200 years ago. ■
SECTION 5.5 Algebraic Solutions of Exponential and Logarithmic Equations 401
*Archeologists can determine how much carbon-14 has been lost by a technique that involves
measuring the ratio of carbon-14 to carbon-12 in the skeleton.

EXAMPLE 6
A certain bacteria is known to grow exponentially, with the population at time t
given by a function of the form g(t) Pe
kt
, where P is the original population and
k is the continuous growth rate. A culture shows 1000 bacteria present. Seven
hours later, there are 5000.
(a) Find the continuous growth rate k.
(b) Determine when the population will reach one billion.
SOLUTION
(a) The original population is P 1000, so the growth function is g(t) 1000e
kt
.
We know that g(7) 5000, that is,
1000e
k
7
5000.
To determine the growth rate, we solve this equation for k.
Divide both sides by 1000: e
7k
5
Take logarithms of both sides: ln e
7k
ln 5
Use the Power Law: 7k ln e ln 5.
Since ln e 1 (why?), this equation becomes
7k ln 5
Divide both sides by 7: k
ln
7
5
.22992.
Therefore, the growth function is g(t) 1000e
.22992t
.
(b) The population will reach one billion when g(t) 1,000,000,000, that is, when
1000e
.22992t
1,000,000,000.
So we solve this equation for t:
Divide both sides by 1000: e
.22992t
1,000,000
Take logarithms on both sides: ln e
.22992t
ln 1,000,000
Use the Power Law: .22992t ln e ln 1,000,000
Remember ln e 1: .22992t ln 1,000,000
Divide both sides by .22992: t
ln 1
.2
,0
2
0
9
0
9
,
2
000
60.09.
Therefore, it will take a bit more than 60 hours for the culture to grow to one
billion. ■
EXAMPLE 7
Inhibited Population Growth The population of fish in a lake at time t months
is given by the function
p(t)
1
20
2
,0
4
0
e
0
t/4
.
402 CHAPTER 5 Exponential and Logarithmic Functions
How long will it take for the population to reach 15,000?
SOLUTION We must solve this equation for t.
15,000
1
20
2
,0
4
0
e
0
t/4
15,000(1 24e
t/4
) 20,000
1 24e
t/4
2
1
0
5
,
,
0
0
0
0
0
0
4
3
24e
t/4
1
3
e
t/4
1
3
2
1
4
7
1
2
ln e
t/4
ln
7
1
2
4
t
(ln e) ln 1 ln 72
4
t
ln 72 [ln e 1 and ln 1 0]
t 4(ln 72) 17.1067.
So the population reaches 15,000 in a little over 17 months. ■
LOGARITHMIC EQUATIONS
Equations that involve only logarithmic terms may be solved by using the follow-
ing fact, which is proved in Exercise 33 (and is valid with log replaced by ln).
If log u log v, then u v.
EXAMPLE 8
Solve log (3x 2) log (x 2) log (7x 6).
SOLUTION First we write the left side as a single logarithm.
log (3x 2) log (x 2) log (7x 6)
Use the Product Law: log[(3x 2)(x 2)] log (7x 6)
Multiply out left side: log (3x
2
8x 4) log (7x 6).
Since the logarithms are equal, we must have
3x
2
8x 4 7x 6
Subtract 7x 6 from both sides: 3x
2
x 2 0
Factor: (3x 2)(x 1) 0
3x 2 0orx 1 0
3x 2 x 1
x
2
3
SECTION 5.5 Algebraic Solutions of Exponential and Logarithmic Equations 403
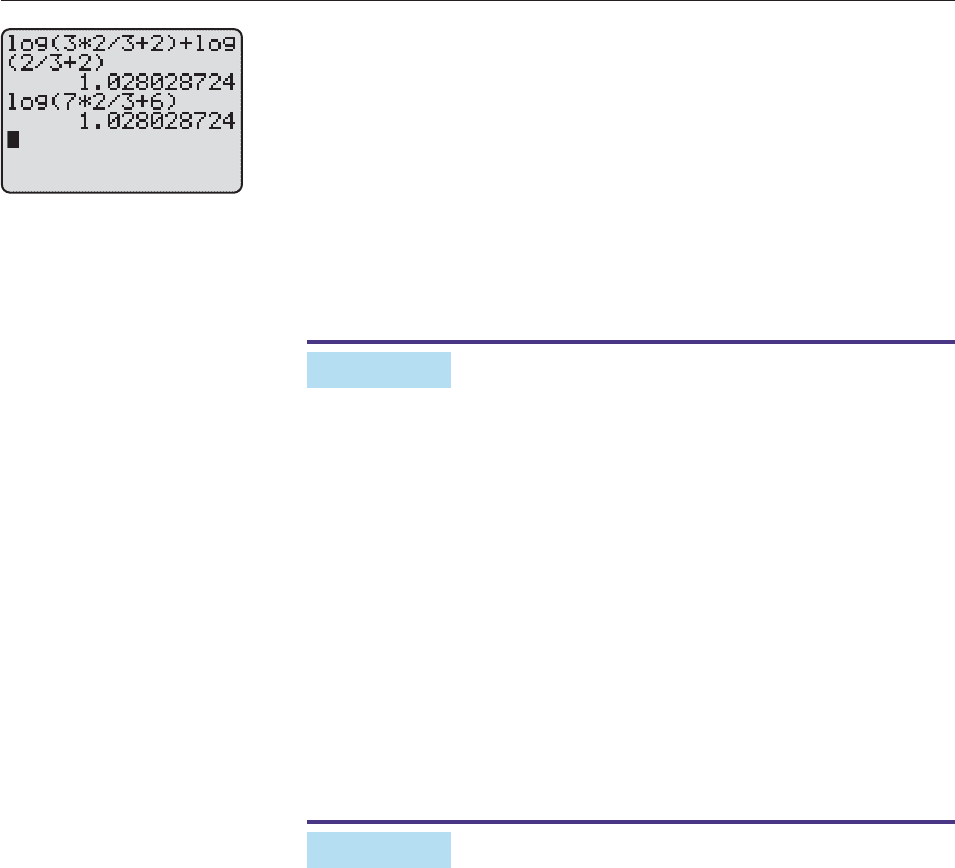
Thus, x 2/3 and x 1 are the possible solutions and must be checked in the
original equation. When x 2/3, both sides of the original equation have the
same value, as shown in Figure 5–28. So 2/3 is a solution. When x 1, how-
ever, the right side of the equation is
log (7x 6) log [7(1) 6] log (1),
which is not defined. So 1 is not a solution. ■
Equations that involve both logarithmic and constant terms may be solved by
using the basic property of logarithms (see page 380).
(
*
) 10
log v
v and e
ln v
v.
EXAMPLE 9
Solve 7 2 log 5x 11.
SOLUTION We start by getting all the logarithmic terms on one side and the
constant on the other.
Subtract 7 from both sides: 2 log 5x 4
Divide both sides by 2: log 5x 2.
We know that if two quantities are equal, say a b, then 10
a
10
b
. We use this
fact here, with the two sides of the preceding equation as a and b.
Exponentiate both sides: 10
log 5x
10
2
Use the basic logarithm property (
*
): 5x 100
Divide both sides by 5: x 20.
Verify that 20 is actually a solution of the original equation. ■
EXAMPLE 10
Solve ln (x 3) 5 ln (x 3).
SOLUTION We proceed as in Example 9, but since the base for these loga-
rithms is e, we use e rather than 10 when we exponentiate.
ln (x 3) 5 ln (x 3)
Add ln (x 3) to both sides: 2 ln (x 3) 5
Divide both sides by 2: ln (x 3)
5
2
Exponentiate both sides: e
ln(x3)
e
5/2
Use the basic property of logarithms (
*
): x 3 e
5/2
Add 3 to both sides: x e
5/2
3 15.1825.
This is the only possibility for a solution. A calculator shows that it actually is a
solution of the original equation. ■
404 CHAPTER 5 Exponential and Logarithmic Functions
Figure 5–28
EXAMPLE 11
Solve log (x 16) 2 log (x 1).
SOLUTION
log (x 16) 2 log (x 1)
Add log (x 1) to both sides: log (x 16) log (x 1) 2
Use the Product Law: log [(x 16)(x 1)] 2
Multiply out left side: log (x
2
17x 16) 2
Exponentiate both sides: 10
log (x
2
17x16)
10
2
Use the basic logarithm property (
*
): x
2
17x 16 100
Subtract 100 from both sides: x
2
17x 84 0
Factor: (x 4)(x 21) 0
x 4 0orx 21 0
x 4or x 21.
You can easily verify that 21 is a solution of the original equation, but 4 is not
[when x 4, then log (x 16) log (20), which is not defined]. ■
EXAMPLE 12
To solve log (x 5) 1 log (x 2),
Rearrange terms: log (x 5) log (x 2) 1
Use the Product Law: log [(x 5)(x 2)] 1
log (x
2
3x 10) 1
Exponentiate both sides: 10
log (x
2
3x10)
10
1
Use the basic logarithm property (
*
): x
2
3x 10 10
x
2
3x 20 0.
This equation can be solved with the quadratic formula.
x
3
2
89
.
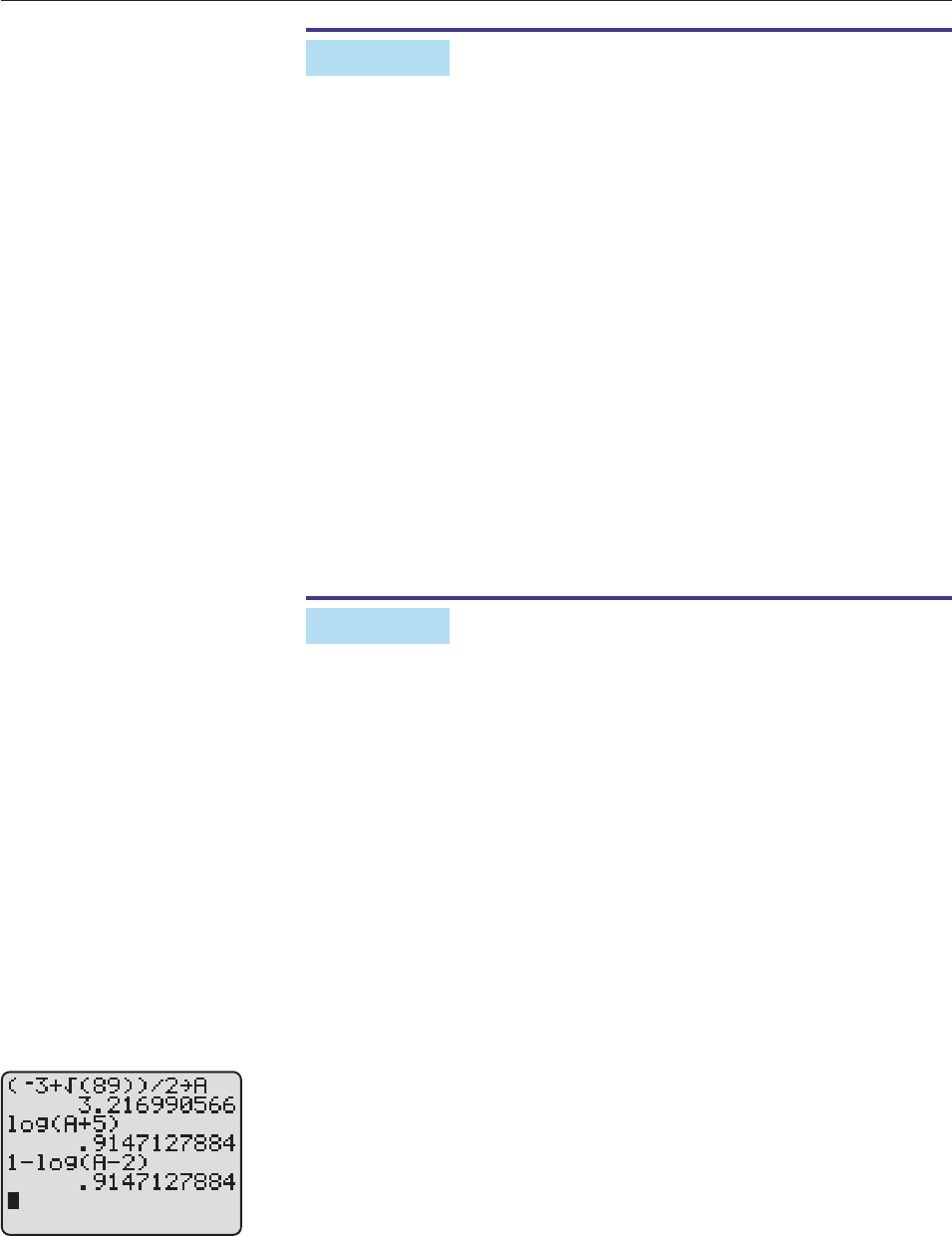
An easy way to verify that
x
3
2
89
is a solution is to store this number in your calculator as A and then evaluate both
sides of the original equation at x A, as shown in Figure 5–29. The other possi-
bility, however, is not a solution because
x
3
2
89
is negative, so log (x 2) is not defined. ■
3
3
2
4
1
(20)
2
1
SECTION 5.5 Algebraic Solutions of Exponential and Logarithmic Equations 405
Figure 5–29
