Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


28.1
VECTOR
FIELDS
AND
CURVATURE
883
28.1 Vector Fields and Curvature
A manifoldis, in general, not flat (flatness will be defined later). One way to "feel"
the curvatnre
of
a space intrinsically is to translate a vector parallel tn itselfalong
differentpaths and compare the final vectors. In a flat space the two vectors at the
end will be the same, but not in a general space.
An
illnstration is provided by the
surface
of
a sphere. Assume that we have a vector perpendicular to the equator.
To exaggerate the effect of curvatnre, we move the vector parallel to itself on the
equatora qnarter of the way around the sphere, then all the way to the north pole.
Alternatively, we start with the vector again perpendicular to the equatnr, but this
time we move it parallel to itself directly to the north pole. Clearly, the two fiual
vectors will uot be the same; in fact, they will be perpeudicular to oue another.
The above intnitive discussion should help to make it clear that to find the
curvatnre
of
space, we look at how vectors change.
In
analogy with the exterior
derivative and forms, we want to introduce a derivative that operates on vectors.
In fact, since we already have the exteriorderivative available, let us see
if
we can
extend
it to vectors.
Consider an arbitrary I-form w and an arbitrary vector field v. The pairing
(w, v) is a real-valued function f ou which we know how the exterior derivative
acts. A uatnral extension of
d (which we denote by the same symbol) is given as
follows:
df
= d
(w,
v) sa
(dw,
v)'
+(w,
dv)'
,
(28.1)
where we have used a prime to designate the new pairing.
In
general, this new
pairing may be different from the ordinary pairing, because for the participants in
the latter, no exterior differentiation is defined. As we shall see below, we indeed
have to change the old pairing slightly for the new pairing to make sense. The LHS
of Equation (28.1) is a I-form. The first term on the RHS is a 2-form coutracted
with avector, i.e., a
l-form,
For
the secoud term to be a l-form,
dv
mnstbe atensor
that contracts with a
l-form
to give a l-form, We say that
dv
is a vector-valued
vector-valued
I-form,
Horms Let us take a basis
lei)
and its dual
{€j)
and express Equation(28.1) in terms of
components
inthosebases.
Then,
ontheone
hand,
withw = Wi e
i
and
v = v
j
ei-
we have (w, v) = WiVi and
df
= d (w, v) = (dWi)Vi +
Widvi.
(28.2)
On the other hand, .
.
..,
..
. ,
df
= (dWi A
€'
+
Wid€',
vJej)
+(Wi€',
dvJej
+
vJdej)
.
.,.
. ,
..
I
..
,
=v
J
(dWi
A€',ej)
+VJWi
(d€',ej)
+Wi
(€',dvJej)
+WiVJ
(€',dej)
= v
j
(dWi A €i,
ej)'
+
Widvj
(E',
ej)
+VjWi
{(d€i,
ej)'
+(€i,
dej}'j,
'--:-'
(28.3)
.
ISj

884 28.
DIFFERENTIAL
GEOMETRY
where we have assumed that the primed pairing is equivalent to the old pairing
when no derivatives are inside.
If
Equations (28.2) and (28.3) are to hold for
arbitrary w and v, we must bave?
. I . ,
0=
ide",
ej)
+(E',
dej)
,
..
.,
(dWi)V'
= v
l
(dWi
A
E',
ej)
.
(28.4)
The first relation is simply the fact that the exterior derivative of
8~
= (E
i
,
e
j)
is
zero. The second relation defines the order
of
contraction
of
vectors and higher-
order forms in the new
pairing.
Since
dWj
is a I-form, we can write it as
dWj
=
Uikf.
k,
so
that
i k i
do» /\ € =
aik€.
/\
e ,
where
aik
=
-aki·
Then, the second equation
of
(28.4) gives
This equation demands that the new pairing
of
a vector with a wedge product of
l-forms be defined as
(
k i )' _ .
(k
i)
1
('
i , k)
€
Ae
,ej
=
-lej
e
Ae
=
-2
Okj€
-Oi}e
,
where i
ej
is the interiorproduct
of
Definition 26.5.8. We summarize the properties
of
the new pairing in the following equation:
. , . ,
(dE',ej)
+
(E',dej)
=0,
(
k
i
)'
.
(k
i)
1
('
i , k)
eAe,ej
=-lej€Ae
=-zUkje-Uij€.
(28.5)
Indifferential structures everything takes placelocally, and translations and move-
ments are all infinitesimal. Let us look at the exterior derivative from this point of
view. For a real-valuedfimction on M, we have
2 (
af
i)
I:
( a
2
f a
2
f)
i i
d f
=d
-.dx
=
-.-.-,
-
-.-.
dx
Adx.
ax'
..
axlax'
ax'ax
l
l<'
Thus, d
2
f = 0 means that the mixed partial derivatives are independent
of
the
order
of
differentiation-a
familiar result. Geometrically, this means that for small
displacements,
dx
i
and
dx
j
,the value of afunction is the same
if
one moves in two
(perpendicular) directions, once in a given order and then in reverse order. This is
2Theseconsistency
relations
applyto thefirst
application
of
exterior
derivative
to the
pairing.
Higher-order
applications,
or,
equivalently,
application
ofd toa
pairing
involving
wedge
products
of forms, may
require
newconsistency
relations.
Fortunately,
forour
purposes,
the
first-order
consistency
relations
will be sufficient.

28.1
VECTOR
FIELDS
AND
CURVATURE
885
true even if the space is cnrved.
For
flat spaces, we know thatthe same conclusion
holds for displacement (parallel to themselves)
of
vectors.
When
we interpret d
2v
as the change in a vector as it is displaced in two different directions, then the
example
of
the sphere above suggests that d
2v
must be related to curvature.
Let
us find this relation.
Starting with a basis [e,}and an arbitrary vectorv
= vier, operate on it with d
twice, keeping in mindthat its action on functions and differential forms is exactly
the same as the exterior derivative defined before:
dv
= dviei +videi and
d
2v
= d
2vi
ei +
(_I)ldv
i
A de, +dv
i
A de,
+v
id
2ei
= vid2ei'
'-.-'
.
=0
":0
(28.6)
This equation has a remarkable property:
It
leaves the components
of
v undiffer-
entiated!
In other words, regardless
of
how any given two vectors v and w vary
away from the point
P
of
the manifold, d
2
v = d
2
w as long as the two vectors are
equal at
P. More importantly,
if
we take the linearity
of
d (and therefore, d
2
)
into
account, we obtain
(28.7)
It
appears that d
2
v depends not on external objects (vectors),
but
on the intrinsic
property
of
the manifold, i.e., how it "curves"away from P. To find this curvature,
expand the vector-valued I-form
de, as
(28.8)
extracting
the
curvature
2-form
from
second
exterior
derivative
of
basis
veetors
As we shall see shortly, one has to be cautious to know which index is raised or
lowered.
Inthe formulas above, this cautionhas
been
observedby leavingblankthe
original position
of
the raised (or lowered) index. Differentiating Equation (28.8)
once more, we obtain the vector-valued 2-form
d2ei = de] A W
i
i
+ei
181
dW
i
i
= (ek
181
wki)
AW
i
i
+ei
181
dW
i
i
_",,(k
i)
""di_""(di+i
k)
=ek'<::JJ
Wj!\W
i
+ej'Ol
wi-ej'O'
Wi
WkAWi·
(28.9)
The
expression in parentheses is a 2-form, called the curvature two-form:
or
(28.10)
With this notation, Equation (28.6) becomes
d
2
id2 i
-o,
Oi
.o,
Oi i - "" e
v=v
ei=vej'Ol
i=ej'Ol
iV
=e'O'
v,
where the last expression is simply an abbreviation.
(28.11)

886 28.
DIFFERENTIAL
GEOMETRY
So far, we have been dealing with the exterior derivatives of the basis vectors
{e.},What about the exterior derivatives of the dual basis vectors {Ei}? They are
closely related to {de;}, as the following argument shows. The first relation of
(28.5), as well as Equation (28.8) and the fact that Ei pairs up with ek yield
. , . , k . I k . j
(ei,
de')
= - (dei, E
l)
= - (ek
I8i
W i' E
l)
=
-w
i
(eko
E
l)
=
-w
i
(28.12)
On the other hand, since dEi is a 2-form, it can be written as
and
where
Y
i _
yi
km - - mk
where we used the second equation in (28.5). Comparing (28.13) with Equation
(28.12) yields
a{
=
-W
i
i
.
We therefore have
(28.14)
Equations (28.8) and (28.14) show that the
{wij}
give all the information about
how the bases {ei} and {Ei} change with infinitesimalmovementaway from a point
P.
28.1.1. Box.
If
we can find the
{wij},
we will know the (local) geometry
of
the manifold.
connection
From the definition of r
i
ik
in Equation (28.8), we have wii
= riikEk. The
coefficients
functions
riik
are called the connection coefficients. Because of (28.8), these
coefficients are antisymmetric in their first two indices. On the otherhand, (28.14)
gives
(28.15)
If
coordinate frames are used for basis vectors, so that E
k
= dx"; the LHS
of
(28.15) will be zero and the coefficients on the RHS of Equation (28.15) must
vanish,
i.e., theconnection coefficients
are
synunetric
in
their
lasttwo
indices:
in coordinateframes. I (28.16)

28.2
RIEMANNIAN
MANIFOLDS
887
Equation (28.15) shows further that by calculating
de
k
we merely determine the
antisymmetriccombination
rijrn
-
rimj.
However, the antisymmetry orrijm in its
first two indices
can
be used to determine it completely.
Let
Cijm ea
rijm
- rim}
be the coefficients that
can
be read off from (28.15). Then
one
can show that
(28.17)
Riemannian
and
pseudo-Riemannian
manifolds
defined
orthonormal
frames
Once the
P's
are determined by this relation, they can be used to write W}i'S as a
linearcombination
of
the dual basis vectors.
28.2 Riemannian Manifolds
As mentioned before, manifolds that possess a metric are important.
The
general
theory
of
relativity, for example, is entirely
based
on the existence
of
a metric.
In
fact, it is the
job
of
that theoryto determine the metric
of
4-dimensionalspace-time
from a knowledge
of
the distribution
of
matter.
28.2.1. Definition.
A Riemannian manifold is a differentioble manifold M with
a symmetric tensor field
9 E
T~(M),
called the metric, such that at each point
P
E M,
gjp
is apositive definite innerproduct. A manifoldwith an indefinite inner
product at each point is called a pseudo-Riemannian manifold.
With 9 defined on
M,
we can obtain orthonormal vectors at each point
of
M.
That
is, we can constroct
orthonormal
frames
{ei} such that
at each point P
EM.
28.2.1 Curvature via Connection
We can find a relation between the metric tensor
and
Wi} by taking the exterior
derivative
of
both sides
of
gil = ei . e} and using Equation (28.8):
dgi} =
(dei)
. e} +e, .
(de})
=(ek ® wk;J. e) +ei . (ek ® w
k)
sa
w\
®
(ek'
e}) +
J}
® (e, . ek) = wkigk} +Wk}gik
'"
W}i +
wij.
In
patticniar, if we
work
in an orthonormal basis, then gil =
±8ij,
and
the LHS
will be
zero'
In
such a case, we obtain the following antisymmetry condition for
the I-forms
Wi}:
structure
equations,
integrability
condltlon,
and
curvature
matrix
Wij+Wji
=0.
(28.18)
30rthonormality is not really required. All that is necessary is for
gjj
to be constant.
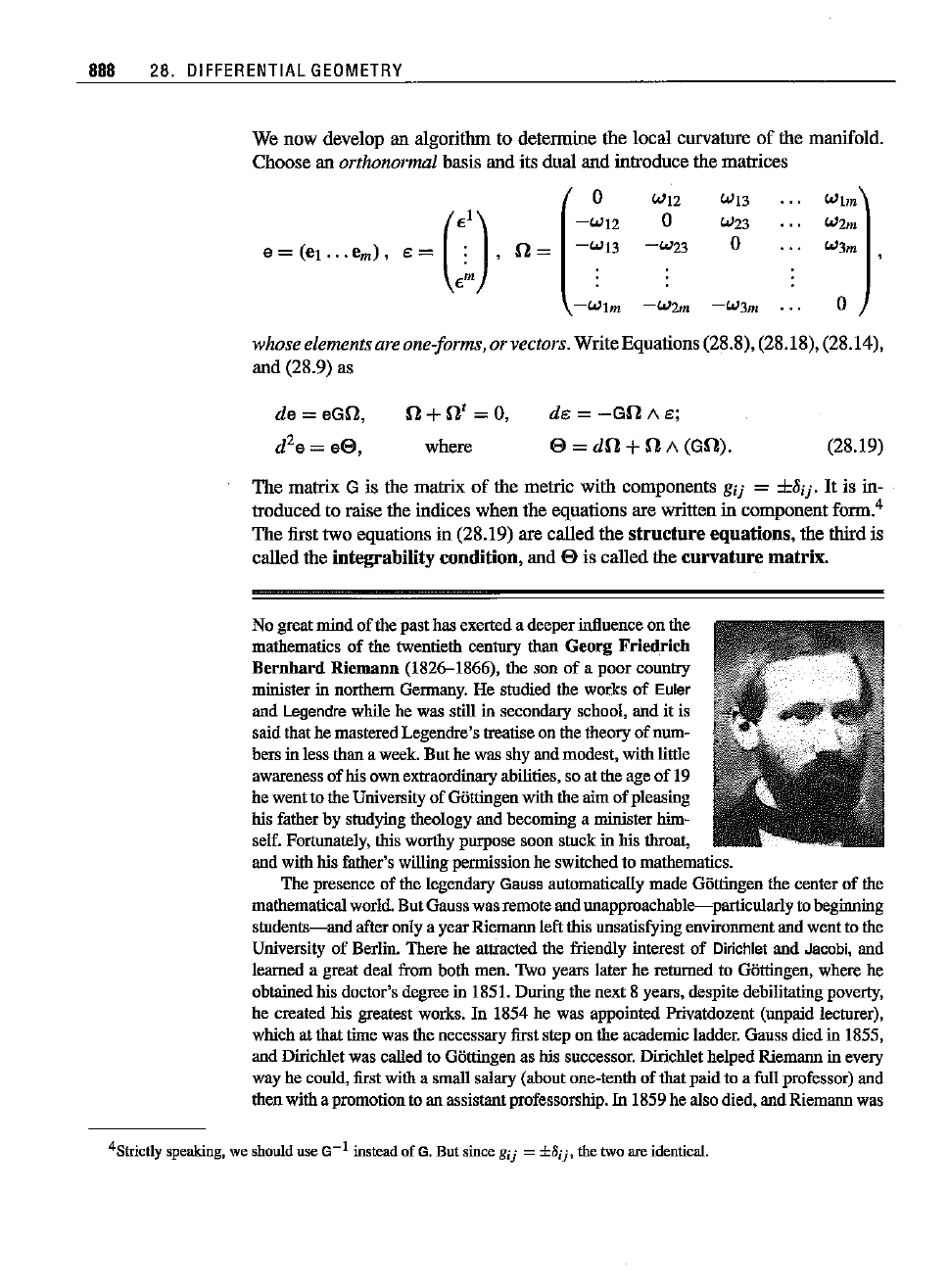
888 28. DIFFERENTIAL
GEDMETRY
We now develop an algoritlnn to determine the local curvature
of
the manifold.
Choose an orthonormal basis and its dual and introduce the matrices
0
W12 W13
Wl
m
e=C),
-W12
0
W23 W2m
e = (el
...
em),
0=
-w13
-W23
0
W3m
-Wl
m
-W2m
-W3m
0
whoseelementsare one-forms, or vectors. Write Equations (28.8), (28.18), (28.14),
and (28.9) as
de=
eGO,
d
2
e=ee,
0+0'
=0,
where
de
=
-GOAe;
e
=dO
+
OA
(GO).
(28.19)
The matrix G is the matrix
of
the metric with components
gij
= ±8ij.
It
is in-
troduced to raise the indices when the equations are wrilten in component form,"
The first two equations in (28.19) are called the
structure
equations, the third is
called the integrability condition, and
e is called the
curvature
matrix.
No
great
mindof thepast
has
exerted
adeeperinfluence onthe
mathematics
of the
twentieth
century
than
Georg Friedrich
Bernhard Riemann (1826-1866), the son of a poor country
minister
in
northern
Germany.
He
studied
the
works
of
Euler
and
Legendre
whilehe wasstill in
secondary
school, andit is
said
that
he
mastered
Legendre's
treatise
onthe
theory
of num-
bers
inless
than
aweek.Buthe wasshyandmodest,withlittle
awareness
ofhisown
extraordinary
abilities, so attheageof 19
hewenttothe
University
of
G5ttingen
withtheaimofpleasing
his
father
by
studying
theologyandbecominga
minister
him-
self.
Fortunately,
this
worthy
purpose
soonstuckin his
throat,
andwithhis
father's
willingpermissionhe switchedto
mathematics.
The
presence
of the
legendary
Gauss
automatically
madeGdttingen the
center
of the
mathematical
world.
But
Gauss
was
remote
andunapproachable-particularly to
beginning
students-and
after
onlyayear
Riemann
leftthisunsatisfying
environment
andwenttothe
University
of
Berlin.
There
he
attracted
the
friendly
interest
of
Dirichlet
and
Jacobi,
and
learned
a
great
deal
from
bothmen.Tho yearslaterhe
returned
to Gottingen,
where
he
obtained
his
doctor's
degree
in 1851.
During
thenext8
years,
despite
debilitating
poverty,
he
created
his
greatest
works. In 1854 he was
appointed
Privatdozent
(unpaid
lecturer),
whichatthattimewasthe
necessary
first
steponthe
academic
ladder.
Gauss
diedin 1855,
and
Dirichlet wascalledto Gottingen ashis successor. Dirichlet helped
Riemann
inevery
wayhe could,firstwitha smallsalary(aboutone-tenth ofthatpaidto a fullprofessor) and
thenwitha
promotion
toan
assistant
professorship.
In 1859healsodied,and
Riemann
was
"Stnctty
speaking,
we shoulduse G-
1
instead
of G.Butsincegjj =
±8ij,
thetwoare
identical.

28.2 RIEMANNIAN MANIFOLDS 889
appointed
asafull
professor
to
replace
him.
Riemann's
yearsof
poverty
were
over,
buthis
health
was
broken.
Attheageof 39 he diedof
tuberculosis
in
Italy,
onthelastof
several
trips
he
undertook
in
order
to escape thecold, wet climateof
northern
Germany.
Riemann
had a short life andpoblishedcomparatively little, but his workspermanently alteredthe
courseof
mathematics
in analysis,geometry, and
number
theory.
Itis said
that
the
three
greatest
mathematicians
of modemtimes
are
Euler,
Gauss,
and
Riemann.
It is a
curiosity
of
nature
thatthese
three
names
are
among
themost
frequently
mentioned
namesinthephysics
literature
aswell.Asidefromthe
indirect
use
of
his namein
the
application
ofcomplexanalysis inphysics,Riemanniangeometryhasbecomethemost
essential
building
blockof all
theories
of
fundamental
interactions,
starting
with
gravity,
which
Einstein
formulated
in this
language
in 1916.As
part
of the
requirement
tobecome
a
Privatdozent,
Riemann
had
to
write
a
probationary
essay andto
present
a trial lecture
to the
faculty.
Itwas the
custom
forthe
candidate
to offer
three
titles,andtheheadof his
department
usually
accepted
the
first.
However,
Riemann
rashly listed as his
third
topic
the
foundations
of
geometry.
Gauss,
who hadbeen
turning
this
subject
overin his mind
for60
years,
was
naturally
curious
toseehowthis
particular
candidate's
"gloriously
fertile
originality"
wouldcopewithsuchachallenge, andto
Riemann's
dismayhe
designated
this
asthe
subject
of the
lecture.
Riemann
quickly
torehimselfaway
from
hisother
interests
at
thetime-"my
investigations
of theconnection betweenelectricity,
magnetism,
light,and
gravitation"-and
wrote
his
lecture
inthenexttwo
months.
Theresultwasoneof the
great
classical
masterpieces
of
mathematics,
and
probably
themost
important
scientific
lecture
evergiven.It is
recorded
thateven
Gauss
was
surprised
and
enthusiastic.
We
can
derive further integrability conditions. For instance, applying d to the
Bianchi
identity
third equation
of
(28.19) gives
0=
d
2e
=
-d(GO)
/\ e +GO /\ de =
-GdO
/\ e - (GO) /\ (GO) /\ e.
Multiplying both sides on the left by G =
G-
1,
we obtain
Riemann
curvature
tensor
0=
-[dO
+0 /\ (GO)] /\ e =
-El
/\ e.
Similarly, the reader may show that
dEl =
0/\
(GEl) - El /\ (GO).
This is called the
Bianchi
identity.
The
i
jth
element
of
the matrix
of
El
can
be written as
(Jij = !
Rijkl,}
/\
e
l
,
(28.20)
(28.21)
(28.22)
which defines the components
Riju
of
the
Riemann
curvature
tensor. The an-
tisyrnrnetry
of
the matrix El (showing this is left as a problem for the reader) and
Equation (28.22) give
Rijkl
+R
jikl
= 0,
Rijkl
+
Rijlk
=
O.
(28.23)
890 28. DIFFERENTIAL
GEOMETRY
Similarly, the relation El
Ae
= 0
ofEqnation
(28.20)can be shownto be eqnivalent
to
Square
brackets
mean
anti
symmetrization.
Ruu
+Riklj +Riljk = 0
where
and
Ri[jkl]
= 0,
(28.24)
28.2.2. Box.
The enclosure
of
indices in square brackets means complete
antisymmetrization
of
those indices.
When coordinate bases are used, Wij is no longer antisymmetric, but we still
have
dgij
= Wij +W
ji
es
rijkdxk
+F
jikdxk.
Since
dgij
=
(agij/axk)dx
k,
we get
agij
glj,k
'"
ax
k
=
rijk
+F
jik-
Christoffel
symbol
Using Eqnations (28.16) and (28.25), we
can
readily show that
1 I
(agij
agik
agkj)
rijk
= 'J.(gij,k +gik,j - gkj,i)
'"
2:
axk + ax
j
-
axi
.
(28.25)
(28.26)
connection
between
infinitesimal
displacement,
arc
length,
and
metric
tensor
This is the
Christoffel
symbol
nsedin classical tensoranalysis. Now we consider
the connectionbetweenan infinitesimaldisplacementand ametric. Let
P be a point
of
M. Let y be acurve through P suchthat y (c) = P.
For
an infinitesimalnumber
Su,let
pI
=
y(c
+8u) be a point on y close to P. Since the
xi
are well-behaved
functions,
xi
(PI)
_xi
(P)
are infinitesimal
real
numbers.
Let;i
=
xi
(PI)
_xi
(P),
and construct the vector v =
;i
ai, where
{ai}
consists
of
tangent vectors at P. We
call v the infinitesimal
displacement
at P.
The
length
of
this vector, g(v, v), is
shown to
be
gij;i;j.
This is called the
arc
length
from P to
r,
and is naturally
written as
ds
2
=
gij;i;
j .
It
is customary to write
dx
i
(not a
I-foun!)
in place
of
;i:
(28.27)
where the
dx'
are infinitesimal real numbers.
Elwin Bruno Christoffel (1829--1900) came from a famityin the clothtrade. He attended
anelementary schoolin Montjoie (whichwasrenamed
Monschau
in 1918)butthenspenta
number
of
years being tutoredat home in languages, mathematics, and classics. He attended
secondary schools from 1844 unti11849. At first he studied at the Jestdt gymnasium in

28.2 RIEMANNIAN
MANIFOLDS
891
Cologne
hutmovedtothe Friedrich-Wilhelms Gymnasiumin thesametownforatleastthe
three final years of his schooleducation. He was
awarded
the
final school certificate
with
a
distinction
in 1849.
The
next
year
he
went
to the University
of
Berlin
and
studied
under
a
number
of
distinguished mathematicians, including Dirichlet.
After
one
year
of
military service in the Guards Artillery Brigade,he
returned
to Berlin
to study for his doctorate,
which
was awarded in
1856
with a dissertation on
the
motion
of
electricity in homogeneous bodies. His examiners
included
mathematicians
and
physicists,
Kummer
being
one
of
the mathematics examiners.
At this point Christoffel spent three years outside the aca-
demic world. He returned to Montjoie, where his mother was
in poor health, but read widely from the works of Dirichlet.
Riemann,
and
Cauchy. It has
been
suggested
that
this
period
of
academic isolation
had
a
major
effect on his personality
and
on
his independent approach towards mathematics.
It
was during
this
time
that
he published his first
two
papers
on
numerical
in-
tegration, in 1858, in which he generalized
Gauss's
method
of
quadrature
and
expressed the polynomials
that
are involved as
a determinant,
This
is
now
called Christoffel's theorem.
In
1859Christoffel took the qualifying examinatiouto be-
come
a university teacher
and
was appointed a lecturer at the University
of
Berlin.
Four
years later, he was appointed to a
chair
at the
Polytechnicum
in Zurich, filling the
post
left
vacant
when
Dedekind
went
to Brunswick. Christoffel was to have a
huge
infiuence on
mathematics at the Polytechnicum, setting up an institute for mathematics
and
the natural
sciences there.
In 1868 Christoffel was offered
the
chair
of
mathematics at the Gewerbsakademie in
Berlin,
which
is
now
the University
of
Technology
of
Berlin. However, after
three
years at
the
Gewerbsakademie in Berlin, Christoffel
moved
to the University
of
Strasbourg as the
chair
of
mathematics, a
post
he
held
until he was forced to retire
due
to
ill
health
in 1892.
Some
of
Christoffel's
early
work
was on
conformal
mappings
of
a simply connected
region
bounded
by polygons
onto
a circle. He also wrote
important
papers
that
contributed
to the development
of
the tensor calculus
of
Gregorio Ricci-Curbastro
and
Tullio
Levi-Clvlta.
The
Christoffel symbols
that
he introduced are
fundamental
in the study
of
tensor analysis.
The
Christoffelreductiontheorem, so
named
by Klein,solves the localequivalence
problem
for two quadratic differential forms.
The
procedure Christoffel
employed
in his solution
of
the equivalence
problem
is
what
Ricci
later
called
covariant differentiation; Christoffel
also
used
the latter concept to define the basic
Riemann-Christoffel
curvature
tensor.
His
approach allowed Ricci
and
Levi-Civita
to develop a coordinate-free differential calculus
which Einstein,
with
the
help
of
Grossmann,
turned
intothe tensoranalysis,the mathematical
foundation
of
general relativity.
In
applications, it is common to start with the metric tensor 9 given in terms
of
coordinate differential forms:
(28.28)
Then the orthonormal bases {eil and
{e
i
}
are constructed in terms
of
{ail
and
{dx
i
}, respectively, and are utilized as illustrated in the following examples.
The

892
28.
DIFFERENTIAL
GEDMETRY
equivalence
of
the arc leugth [Equation(28.27)] and the metric [Equation (28.28)]
is the reason
why it is the arc length that is given in most practical problems.
Once the arc length is known, the metric
gij
can
be read off, and all the relevant
geometric quantities can be calculated from it.
28.2.3.
Example.
Let us look at a few examplesof arc lengthsand the corresponding
metrics.
(a)For ds
2
=
dx
2
+
di
+ dz
2,
9 is the Euclideanmetric
of]R3,
with gij = 8ij'
(b)For ds
2
=
-dx
2
- d
y2
- dz
2
+
dt
2,
9 is the Minkowski (or Lorentz)metricof
]R4,
with
gij
= 'fJij,where
7Jxx
=
'fJyy
=
'11zz
=
-YIn
=
-1
and
TJij
= 0 for i
=1=
j.
(c) For ds
2
= dr
2
+ r
2(d02
+ sin
20dq>2),
the metric is the Euclideanmetric given in
spherical
coordinates
in
IR3
with
grr
= I, gee = r
2
,
g(/l({J
= r
2sin
2
e.andall
other
components
zero.
(d) For ds
2
= a
2d02
+ a
2
sin
2
Odq>2,
the metric is that of a two-dimensional spberical
surface,
with
see
= a
2
, gtptp = a
2
sin
2
e,
andall
other
components
zero.
Friedmann
metric
(e)For
the
metric
is theFrledmann metricusedin cosmology.
Here
gtt = 1,sx« =
-[a(t)]2,
g99 =
-[a(t)]2
sin
2
x.
g~~
= _[a(t)]2 sin
2
Xsin
2
0, and all othercomponents are zero.
Schwarzschild
metric
(f)
For
the metricis
the Sehwarzsehild metric with Btt =
1-
2M/r,
grr =
-(I
-
2M/r)-t,
gfJf)
=
_r
2
,
g(fl'P
=
_r
2
8in
2
9, andall
other
components
zero.
For
eachof the
arc
lengths
above,
we
have
an
orthonormal
basisof
one-forms:
(a) 9
=.1
®.I
+.2
®.2
+.3
®.3
with.
1
=
dx,.2
=
dy,.3
= dz:
(b) 9 =
_.1
®.I
_ .2
®.2
_ .3
®.3
+.0
®.O
with.
1
=
dx,
e2
= dy, .3 = dz,
.0
= dt;
(c) 9 = t!'
®.'
+.9
®.9
+.~
®.~
with e" =
dr,.9
=
rdO,.~
=
rsinOdq>;
(d)9 = .9
®.9
+."
®.~
with.e
=
adO,.~
= a
sinOdq>;
(e) 9 =
.'
®.'
_.x
®.x
_.9
®.9
_.~
®.~
with.'
=
dt,.X
=
a(t)dx,.9
=
a(t)
sinXdO,.~
= a(t) sinX
sinOdq>;
(f)
9 =
.'
®
.'
-
.r
®
.r
_
.e
® .9 _
.~
®.~
with.'
= (I -
2M/r)I/2dt,
.'
=
(1-
2M/r)-1/2dr,.9
=
rdO,.~
=
rsinOdq>.
III
28.2.4. Example. In this
example,
we
examine
the
curvilinear
coordinates
usedin
vector
analysis.
Recall
that
in terms of these
coordinates
the
displacement
is givenby ds
2
=
hr(dql)2
+
h~(dq2)2
+
h~(dq3)2.
Therefore,theorthonormalone-forms
are.
1
=hI
dqj,
.2
= h2
dq2,
.3 = h3
dq3.
Wealsonote (seeProblem26.24)that
(28.29)
