Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


irreducible
representations
of
the
rotation
group
and
spherical
harmonics
Connection
between
Casimir
operators
and
the
PDEs
of
mathematical
physics
27.5
REPRESENTATION
OF
LIE
ALGEBRAS
863
is a
Casimir
operator.
Butthisis preciselythe
operator
discussed in detailin
Chapter
12.
We
found
there
that
the
eigenvalues
of L
2-
were
labeled
by
j,
where
j was
either
an
integer
orahalfodd
integer.
Inthecontextof our
present
discussion, wenotethattheLie
algebra
50(3)has
rank
one,because
there
isno
higher
dimensional
subalgebra
ofso(3) allofwhose
vectors
commute
with one
another.
It follows from
Theorem
27.5.5
that
L
2
is the only
Casimir
operator,
and
that
all
irreducible
representations
T(j)
of so(3)
are
distinguished
by
their
label
j.
Furthermore,
the
construction
of
Chapter
12 showedexplicitlythatthe
dimension of
T(J) is
2j
+ 1.
Theconnectionbetweenthe
representation
of
Lie
algebras
andLie
groups
permits
usto
conclude thatthe
irreducible
representations
of the
rotation
group
are
labeledbythe(half)
integers
i. andthejth
irreducible
representation
hasdimension
2j
+1.Whenj isan
integer
1andthe
carrier
spaceis
/:.;2(S2),
the
square-integrable
functions
ontheunit
sphere,
thenL
2
becomesa
differential operator, andthe
spherical
harmonics Yl
m
«(),
rp),
withafixedvalue
of
l,
provide
abasisforthelth
irreducible
invariant
subspace.
II
The last sentence of Example 27.5.6 is at the heart of the connection between
symmetry, Lie groups, and the equations of mathematical physics. A symmetry
operation of mathematical physics is expressed in terms of the action of a Lie
group on an underlying manifold
M,
i.e., as a group of transformations of M.
The Lie algebra of such a Lie group consists of the infinitesimal generators
of
the
corresponding transformation. These generators can be expressed as first-order
differential operators as in Equation (27.20).
It
is therefore naturalto chooseas the
carrier space of a representation the Hilbert space
£,2
(M)
of
the square-integrable
functions on
M,
which, through the local identification
of
M with jRm (m =
dim M), can be identified with functions on jRm•
Then
the infinitesimal generators
act directly on the functions
of
£,2(M)
as first-order differential operators.
The Casimir operators
(Ca}~~l'
where r is the rank
of
the Lie algebra, are
polynomials in the infinitesimal generators, i.e., differential operators of higher
order. On the irreducible invariant subspaces of
£,2(M),
each C
a
acts as a multiple
of the identity, so
if
f(r)
belongs to snch an invariant subspace, we have
Caf(r)
= )..(OI)f(r),
ex.
=
1,2,
...
, r. (27.62)
This is a set of differential equations that are invariant under the symmetry of the
physical system, i.e., its solutions transform among themselves under the action
of the group
of
symmetries.
It
is a stunning reality and a fact of profound significance that many
of
the
differential equations
of
mathematical physics are, as in Equation (27.62), expres-
sions of the invariance of the Casimir operators
of
some Lie algebra in a particular
representation. Moreover, all the standardfunctions of mathematicalphysics, such
as Bessel, hypergeometric, and confluent hypergeometric functions, are related to
matrix elements in the representations of a few of the simplest Lie gronps (see
[Mill68) for a thorough discussion of this topic).
Claude Chevalley(1909-t984) was the onlysonof AbelandMarguerite Chevalley who
werethe
authors
of the
Oxford
Concise
French
Dictionary.
He
studied
under
Emile
Picard

864 27. LIE
GROUPS
ANO
LIE
ALGEBRAS
at
theEcole NormaleSuperieurin Paris, graduatingin 1929and becomingthe youngestof
the mathematiciansof the
Bourbaki
school.
After graduation, Chevalley went to Germany to continue
his studiesunder
Artin
at Hamburgduring the session 1931-32.
He then went to the Universityof Marburg
to work with Hasse,
who had been appointed to fill Hensel's chair there
in 1930.
He was awardedhis doctorate in 1937.A year later Chevalley
went to theInstitutefor AdvancedStudy at Princeton, where he
also served on the faculty
of
Princeton University. From July
1949
until June 1957 he served as professor
of
mathematics at
Columbia University, afterwardsreturning to the University of
Paris.
Chevalleyhad a major influenceon the development of several areas of mathematics.
Hispapersof 1936aod 1941ledto major advaocesinclassfieldtheoryaodalsoinalgebraic
geometry.He didpioneeringworkin the theory of local rings in 1943,developingthe ideas
of
Krull intoa theorembearinghisname.Chevalley'stheoremwasimportantin applications
madein 1954toquasi-algebraically closedfieldsaodthefollowingyeartoalgebraicgroups.
Chevalleygroupsplayacentralrole intheclassificationof finitesimplegroups.Hisnameis
alsoattachedto Chevalleydecompositionsand
to aChevalleytypeof semisimplealgebraic
group.
Manyofhistextshavebecomeclassics.HewroteTheory
of
Lie Groups inthreevolumes
whichappearedin 1946,1951, aod 1955.Healso publishedTheory
of
Distributions (1951),
Introduction to the Theory
of
Algebraic Functions
of
one Variable (1951),The Algebraic
Theory
of
Spinors (1954), Class Field Theory (1954), Fundamental Concepts
of
Algebra
(1956),aodFoundations
of
Algebraic Geometry (1958).
Chevalleywasawardedmanyhonors for his work. Among these was the ColePrize of
theAmericanMathematicalSociety.Hewaselected amemberoftheLondonMathematical
Societyin 1967.
27.5.3 Representation of .50(3) and .50(3,1)
Because
of
theirimportance in physical applications, we studythe representations
Of50(3),
the
rotation,
and50(3,
I),
the Lorentz, algebras.
For
rotations, we define
Jl
'"
-iM23, J2
'"
iMB, and J3
'"
-iM12Y
and
note
that
the
J, 's satisfy exactly
the samecommutationrelationsas
the
angular
momentum
operators
of
Chapter12.
Therefore, the irreducible representations
of
50(3) are labeled by
j,
which
can
be
an integer or a balf-odd integer (see also
Example
27.5.6).
These
representations
are finite-dimensional because
80(3)
is a
compact
gronp (Example 27.3.3 and
Theorem27.3.9). The dimension
of
the irreducible representation
of
50(3) labeled
by
j is
2j
+1.
Because
of
local isomorphism
of
Lie
groups
and
their Lie algebras, the same
irreducible spaces found for
Lie
algebras
can
be
used
to represent
the
Lie groups.
13Sometimes we use J
x•
J
y,
and J
z
instead of
Jl,
J2, and J3.

27.5
REPRESENTATION
OF
LIE
ALGEBRAS
865
In particular, the states
(Ijm)
}~,=_j'
where m is the eigenvalue of J" can also be
used as a basis of the j -th irreducible representation.
The flow of each infinitesimal generator
of
50(3) is a one-parameter subgroup
of
S
0(3).
For example, exp(M12rp)is arotation of angle 'I' aboutthe z-axis. Using
Euler angles, we can write a general rotation as
R(1f!,
e,
'1') = exp(M121f!) exp(M31e) exp(M12rp).
The corresponding rotation operator acting on the vectors of the carrier space is
R(
1f!,
e, '1') =
exp(M121f!)
exp(M31
e)
exp(M12rp)
=
eiJ,Y,
eiJ,e
eiJ,~.
rotation
matrix
The
rotation
matrix
corresponding to the above operator is obtained by sand-
wiching
R(1f!,
e,
'1')
between basis vectors of a given irreducible representation:
D~,~(1f!,
e, '1')
'"
(jm'l
R(1f!,
e, '1')Ijm) =
(jm'l
eiJ,Y,
e
iJye
eiJ,~
Ijm)
=
eim'Y,
eim~
(jm'l
eiJ,e Ijm) =
ei(m'y,+m.)d;;;'~(e).
(27.63)
Thus, the calculation of rotation matrices is reduced to finding
d;;;'~
(e). These are
Wigner
formula
for given by the
Wigner
formula
(see [Hame 89, pp. 348-357]):
rotafion
matrices
. (
e)2(j-JL)+m-m
1
(
e)2
JL
+m
l
- m
d~,~(e)
=
Lq,(j,m,m';!-')
cos2"
sin-
" 2 (27.64)
where
. m m': =
-1"
[(j
+
m)!(j
-
m)!(j
+
m')!(j
- m')!]1/2
q,(j, ,
,!-,) - ( )
(.
+
)1
1('
I
)'(
I
+)1
j m
-!-'
.!-'. j - m
-!-'
. m - m
!-'.
and the summation extends over all integral values of !-' for which the factorials
have a meaning. The number of terms in the summation is equal to 1
+
r,
where
r is the smallest
of
the four integers j ± m, j ± mi.
From the rotation matrices, we can obtain the characters of the rotation group.
However, an easier way is to use Euler's theorem (Theorem 4.7.7), Example
23.2.19, and Box 24.2.7 to conclude that the character of a rotation depends only
on the angle of rotation, and not on the direction of the rotation axis. Choosing the
z-axisasouronlyaxis of
rotation,
we
obtain
j j j
X(j)(rp) = L
(jml
eiJ,~
Ijm) = L
eim~
=
e-
iN
L
ei(j+m)~
m=-j
m=-
j
m=-
i
..
2j.
..
ei(2j+l)~
_ 1
= e-lJrp '"'" e
lk
rp
=
e-1j'P"---,-;:.
,----,---
L..,
e1f/J
- 1
k=O
ei(j+l)~
_
e-ij~
sin(j
+
~)
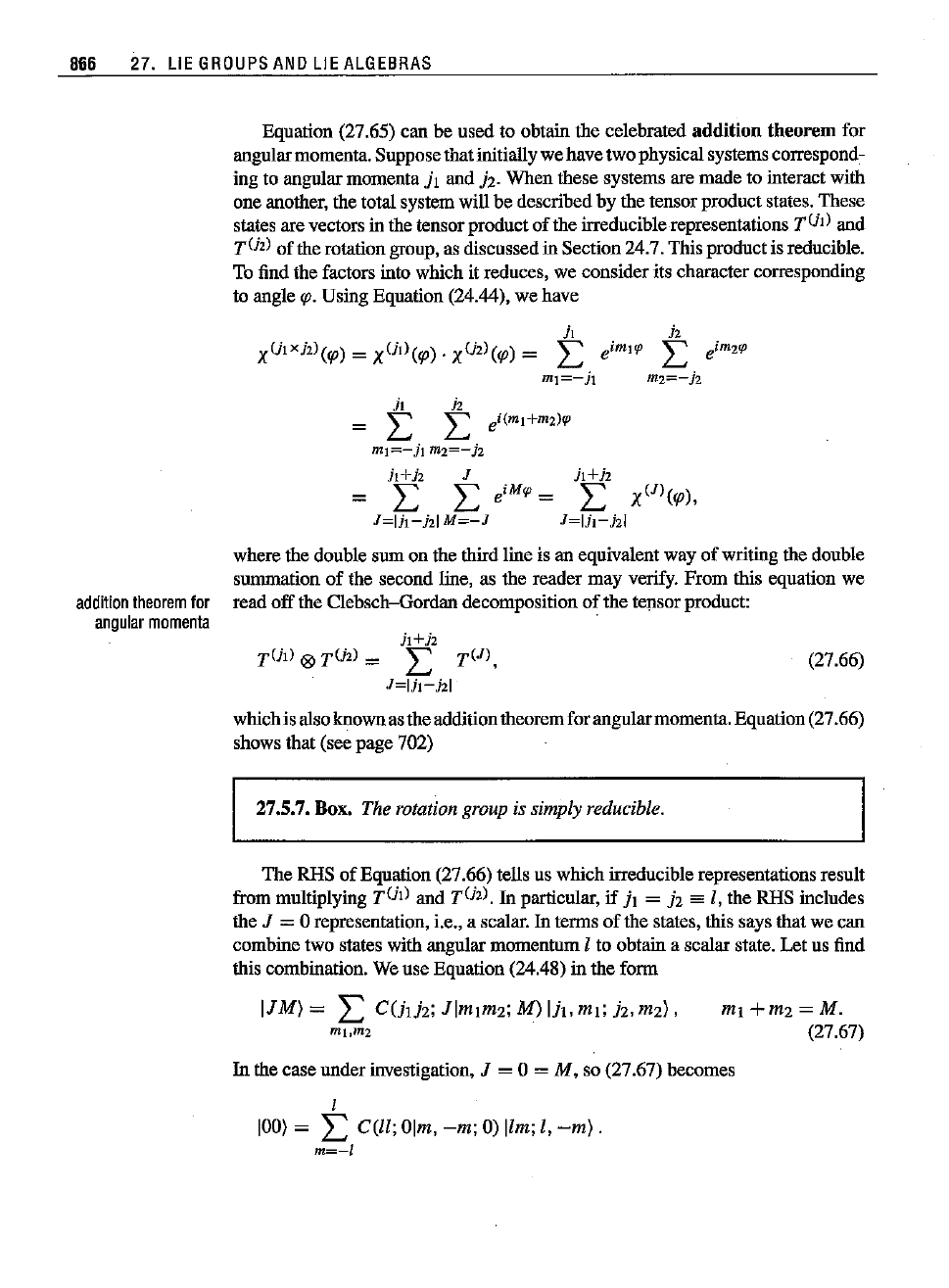
= = (27.65)
ei~
- 1 sin(rpj2)
866 27. LIE
GROUPS
ANO
LIE
ALGEBRAS
Equation (27.65) can be used to obtain the celebrated
addition
theorem
for
angularmomenta. Supposethatinitiallywe bavetwo physical systemscorrespond-
ing to angular momenta
l: and h- When these systems are made to interact with
one another, the total systemwill be described by the tensor product states. These
states are vectors in the tensorproduct
of
the irreducible representations T
Ch)
and
TCh)
of
the rotation group, as discussed in Section 24.7. This productis reducible.
To find the factors into which it reduces, we consider its character corresponding
to angle
tp, Using Equation (24.44), we have
it
12
XUtxh)(rp) =X
Cill
(rp). X
(
2)(rp)
= L
eimt~
L
eimz~
mt=-it
m2=-h
h
= L
h:
L e
i
(m I+m2)rp
(27.66)
addition
theorem
for
angular
momenta
iI+h
J
h+h
= L L
eiM~
= L XCI)(rp),
1=lh-hIM=-1
1~lh-hl
where the double sum on the third line is an equivalent way
of
writing the double
summation
of
the second line, as the reader
may
verify. From this equation we
read
off
the Clebsch-Gordan decomposition
of
the tensor product:
h+h
TUt>
e TUz) = L TCI),
1=lh-hl
whichis also knownasthe additiontheoremfor angularmomenta. Equation(27.66)
shows that (see page 702)
27.5.7. Box. The rotation group is simply reducible.
The
RHS
of
Equation (27.66) tells us which irreducible representations result
from multiplying TUt) and TUZ).
In particular,
if
h = iz
==
I, the RHS includes
the
J = 0 representation, i.e., a scalar. In terms
of
the states, this says that we can
combine two states with angular momentum I to obtain a scalar state. Let us find
this combination. We use Equation (24.48) in the form
IJM)
= L
C(hh
Jlmj
m2;
M)
Ih,
mj;
h,
m2),
ml,m2
mj
+m2
=M.
(27.67)
In the case underinvestigation, J = 0 =
M,
so (27.67) becomes
I
100)
= L C(ll;
Dim,
-m;
0)
11m;
I,
-m)
.
m=-l

27.5
REPRESENTATION·
OF
LIE
ALGEBRAS
867
Problem 27.33 shows that C(ll;
Dim,
-m;
0) =
(_I)I-m
/ ,J21+I, so that
I
(_l)l-m
100)
= L
,J2TTI
11m;
I,
-m)
.
.
m~-l
21+1
Take the "inner product"
of
this with
(IJ,
tp;IJ',
",'I
to obtaio
I
(_l)l-m
(IJ,tp;IJ',",'I 00) = L
,J2TTI
(IJ,
tp; IJ',
",'11m;
I,
-m)
m~-l
21+I
I
(_l)l-m
, ,
= L
,J2TTI(IJ,,,,llm)(IJ,,,,II,-m),
I 21+I
'"---vo-''-v-'
m=-
Ylm(e,~)
Yl,-m«()',ql)
(27.68)
where we have used
(IJ,
ip; IJ',
",'I
=
(IJ,
",I
(IJ',
",'I
and contracted each
bra
with
a ket. We can evaluate the LHS
of
(27.68) by noting that since it is a scalar, the
choice
of
orientation of coordinates is immaterial. So, let
IJ
=0to get IJ'=y, the
angle between the two directions. Then using the facts
fii+l
YlO(lJ,
",)
= V
~PI(coslJ)
and
r:
Ylm(O,",) =
SmO
--
4".
on the RHS
of
(27.68), we obtaio
(-Ii
(IJ,
tp;
IJ',
",'I
00) =
--,J21
+I PI(COSY).
4".
Substituting this in the LHS
of
Equation (27.68), we get
I
41l '"'" m
.,
!
PI(COS
y)
= 21+I
m~}
-I)
Ylm(lJ, "')YI,-m(1J ,
'"
),
which is the addition theorem for spherical harmonics discussed in Chapter 12.
Let us now turn to
.0(3,
1). We collect the generators in two categories
M sa
(Ml,
M2, M3) sa (M23, M31, M12),
N
es
(Nl,
N2, N3) es (MOl, M02, M03),
and verify that
[Mi,
Mj]
=
-€ijkMk,
[NI,
Nj]
= €ijkMk, [MI,
Nj]
=
-€ijkNk,
and that there are two Casimir operators: M
2
-
N
2
and M . N.
It
follows that the
irreducible representations
of
.0(3,
1) are labeled by two numbers. To find these
numbers,
define
the
generators
1
J
==
2i
(M
+
iN),
1 .
K==
-(M-IN)
2i '

8&8
27. LIE
GROUPS
AND
LIE
ALGEBRAS
and show that
It follows that the
J's
and
the
K's
generate two completely independent
Lie
al-
gebras isomorphic to the angular
momentum
algebras and that
so(3,
I) is a direct
sum
of
thesealgebras. Since each
one
requires a (half-odd) integer to designate its
irreducible
representations,
we canchoosethesetwo
numbers
as the eigenvalues
of
the Casimiroperators needed to labelthe irreduciblerepresentations
of
so (3,
I).
Thus, the irreducible representations
of
so(3,
I) are
of
the form
TW'),
where j
and
i'
can
each be an integer or a half-odd integer.
27.5.4 Representationof the Poincare Algebra
The Poincare algebra p(p, n -
p),
introduced in Section 27.2.1, is the generaliza-
tion
of
the
Lie
algebra
of
the invariance group
of
the special theory
of
relativity.
It contains the Lorentz, the rotation, and the translation groups as its proper sub-
groups. Its irreducible representations are
of
direct physical significance, and we
shall study themhere.
As the first step inthe construction
of
representations
of
p(p,
n-
p),
we shalltry
to find its Casimir operators. Equation (27.61) suggests one, but it works only for
semisimple
Lie
algebras, and the Poincare algebrais
not
semisimple. Nevertheless,
let us try to find an operator
based
on that construction.
From
the commutation
relations for
P(p, n -
p),
as given in Equation (27.40),
and
the double-indexed
structure constants defined by,14
we obtain
mn
,m,n ,m,n
+
,m,n
,moo
Cjj,kl = OJ 01
tlik
- OJ 0kTJa Vi ukTJjl - Uj 01
Tljt,
CV,k
=
8j11ik
-
8'('1}jk.
(27.69)
From
these
structure
constants, we can
construct
adoubleindexed
"metric"
.. _ rs
...mn
+ r m
glj,kl
- Cij,mn(;kl,rs Cij,mCkl,T'
which the reader may verify to be equal to
14Pleasemakesuretodifferentiate betweenthepair(Mij, Pk) (which acts onp) andthepair(Mij,Pk),whichactsonthestate
vectors
in the
Hilbert
spaceof
representation.

27.5
REPRESENTATION
OF
LIE
ALGEBRAS
869
There is no natnral way of constructing a single-indexedmetric. Therefore, we can
only contract the M's.
In
doing so, it is understood that the indices are raised and
lowered by ryij' So, the first candidate for a Casimir operator is
2
""kl
""kl""
M sa gij.klM'JM =
2(n
- l)(ryjkryil - ryikryjl)M'JM =
-4(n
- I)M'JMij
The reader may verify that M
2
commntes with all the Mij 's but not with the pi,s.
This is to be expected because M
2
,
the total "angular momentnm" operator'P is
a scalar and should commute with all its components. But commutation with the
pi'8
is not
guaranteed.
The construction above, although a failure, gives us a clue for a successful
construction. We can make another scalar out of the P's. The reader may check
that p
2
es
ryijpiPj
indeed commutes with all elements
of
the Poincare algebra.
We have thus found one Casimir operator. Can we find more? We have exhausted
the polynomials of degree two. The only third-degree polynomials that we can
construct are
Mij PiPj and ryilMijMjkMkl. The first one is identically zero (why?),
and the second one will not commute with the P's.
To find higher-orderpolynomials in the infinitesimal geuerators, we build new
tensors out
of
them and contract these tensors with one another. For example,
consider
thevector
(27.70)
Then
c'Ci, a fourth-degree polynomial in the generators, is a scalar, and therefore,
it commuteswiththe
Mij
's, butunfortunately, not with
Pi'S.
Another common way to construct tensors is to contract various numbers
of
the generators with the Levi-Civita teusor. For example,
(27.71)
is a contravariant tensor
of
rank n - 3. Let us contract W with itselfto find a scalar
(which we expect to commute with all the Mij's):
W
2
= W
it
...i
n
-
3
W· .
- lJ···
1
n- 3
- E
il
...i
1l-3jkI
M·
P E"
M"p
t
- [k: I 1I···ln_3rst
(
l)n_"
,it
,i2 8
i.-
3
8
j
8
k
8
1
M P
M"P'
= -
L..J
Ejfu
JT(it)u
n(i2)
...
1l"(in-3) nCr)
n(s)
net)
jk
I
n
=
(-I)P(n
- 3)! L
E,,8£(r)8~(,)8;(t)MjkPIM"pt,
-n
where we used Equation (25.21). The sum above can be carriedout, with the final
result
W
2
=
2(-I)P(n
- 3)!
(MijM
ijp
2
-
2CiCi)
=
2(-I)P(n
- 3)!
(M
2p
2
-
2C
2
) ,
15This
"angular
momentum"
includes
ordinary
rotations
aswell asthe
Lorentz
boosts.
(27.72)

870
27. LIE
GROUPS
AND
LIE
ALGEBRAS
where Ci was defined in Equation (27.70). We have already seen that M
2
,
p
2
,
and
C
2
all commute with the Mjk 's. The reader may check that W
2
commutes with the
Pj 's aswell. In fact,W
it
...i
n
- 3 itselfcommutes withall thePj
'so
Other
tensors
and
Casimir
operators
canbe
constructed
in a similar
fashion.
We now want to construct the irreducible vector spaces that are labeled by
the eigenvalues of the Casimir operators. We take advantage of the fact that the
poincare algebra has a commutative subalgebra,the translation generators. Since
the
Pk'S commute among themselves and with p
2
and W
2,
we can choose simulta-
neous eigenvectors of
{PklZ~I'
p
2
,
and W
2.
In particular,we can label the vectors of
an irreducible invariant subspaceby the eigenvalues of these operators. The p
2
and
w
2
labels will be the same for all vectors in each irreducible invariant subspace,
while the Pk'S will label different vectors of the same invariant subspace.
Let
us concentrate on the momentum labels and lei I
1frt}
be a vector in an
irreducible representation of
p(p,
n -
p),
where p labels momenta and I-' distin-
guishes among all different vectors that have the same momentum label. We thus
have
for k = 1,2,
....
n,
(27.73)
where
Pk is the eigenvalue
of
Pk. We also need to know how the "rotation" op-
erators act on
l1frt).
Instead of the full operator
eMijO'j,
we apply its small-angle
approximation 1
+
Mijeij.
Since all states are labeled by momentum, we expect
the rotated state to have a new momentum label, i.e., to be an eigenstate
of
Pk. We
want to show that (1 +
Mije
i
j)
l1frt}
is an eignevector of Pk. Let the eigenvalue
be
p',
which should be slightly different from p. Then, the problem reduces to
determining 8p' es
p'
- p. Ignoring the index I-' for a moment, we have
Pk
l1fr
p'}
= PI.
l1fr
p')
= (Pk +8Pk) (1 +
Mijeij)
l1fr
p)
.
Using the commutation relations between Pk and Mij, we can write the LHS as
LHS
= Pk (1 +
Mijeij)
l1fr
p}
= [Pk
+eij
(MijPk +
~jkPi
-
~ikPj)]
l1fr
p).
The RHS, to first order in infinitesimal quantities, can be expressed as
RH
S = (Pk +
8Pk
+Pkeij
Mij)
l1frp}
.
Comparison of the last two equations shows that
.. ..
I"
I
8Pk=
e'}
(~jkPi
-
~ikPj)
=
e'}
(~jk~il
-
~ik~jl)
P =
e'}
(Mij)kl P ,
where we used Equation (27.37).
It
follows that
p' = p +8p = (1 +
eijMij)p,
stating that the rotationoperatorof the carrierHilbert space rotates the momentum
label of the state. Note that since "rotations" do not change the length (induced by
'T/),
p'
and p have the same length.
•

construction
and
properties
oftheIiltle
group
Iiltle
group
and
Iiltle
algebra
induced
representations
27.5
REPRESENTATION
OF
LIE
ALGEBRAS
871
To obtain all the vectors
of
an irreducible representation
of
p(p, n -
p),
we
must apply the rotation operators to vectors snch as
Itt).
But
not all rotations
will change the label p; for example, in three dimensions, the vector p will not be
affected16by a rotation about p. Tltis motivates the following definition.
27.5.8.Definition.
Let
Po
be any given eigenvalue
of
the translation generators.
The set
9<po
of
all rotations
APO
that do not change Po,is a subgroup
of
the rotation
group 0
(p,
n -
p),
calledthe little
group
corresponding to Po. The little algebra
consists
of
the generators
M~o
satisfying
M
PO
-
0
ijPo-
.
The significance
of
the little group resides in the fact that a representation
of
9<po
induces a represeutation
of
the whole Poincare group. We shall only sketch
the
proof
in the following and refer the reader to Mackey [Mack 68] for a full and
rigorous discussion
of
induced
representations.
Suppose we have found an irreducible representation
of
9<po
with operators
APo.
Let
APPO
be the rotation that carrtes'? Po to p,i.e., p =
APPopo.
Considerany
rotation A and let pi be the momentum obtained when A acts on
p, i.e., Ap sa p'.
Then
Tltis shows that
(AP'PO)
-I
AAPPo
belongs to the little group. So,
T(A)
Itt)
sa A
Itt)
=
AP'poAPO
(APPtJ)-1
Itt)
=
AP'poAPO
Itto)
=
AP'PtJ
L:
TV/L(APO)
It;o)
=
L:
TV/L(APO)AP'PO
It;:')
v v
=
L:
TV/L(APO)
It;,)
=
L:
TV/L(APO)
ItX
p)'
v v
Note how the matrix elements
of
the representation
of
the little group alone have
entered in the lastline. We therefore consider
16The
reader
shouldbe warnedthat
although
such a
rotation
does not changep. the
rotation
operator maychangethe state
I'iflpf'
However,
the
resulting
statewillbean
eigenstate
ofthePk'swith
eigenvalue
p.
Weareusing thefact
that
0 (p, n - p) is
transitive
(see Problem27.39).

872 27. LIE
GROUPS
AND LIE
ALGEBRAS
To avoid confusion, we have used R for the representation of the little group. We
claim that Equation (27.74) defines a (matrix) representation of the whole group.
In fact,
T(Aj)T(Az)
Ilfr~}
= T(Aj) L
RVl'(A~)
IlfrX
2
P}
v
= L
Rvl'(~O)
L Rpv(A\",)
Ilfrf,A2P}
v p
=
~
(~Rpv(A~O)RVl'(~)).llfrf'A2P)'
=Rpp;(Aro~Po)
sinceR is arep.
ThereadermaycheckthatA~OA~O
es (AjAz)Po. Therefore,
T(Aj)T(Az)
Ilfr~}
= L Rpl'
((AjAz)PO)
Ilfrf,A2P}
es T(AjAz)
Ilfr~}
,
p
and T is indeed a representation. It turns out that if R is irreducible, then so is T.
The discussion above shows that the irreducible representations
of
the Poincare
group are entirely determined by those
of
the little group and Equation (27.73).
The recipe for the construction
of
the irreducible representations
ofp(p,
n -
p)
is
now clear:
27.5.9.
Theorem.
Choose any simultaneous eigenvector Po
of
the Pk'S. Find the
little algebra
!R
po
at
Po by finding all Mij's satisfying
MijPO
=
O.
Find all irre-
ducible representations
of
!R
po
' The same eigenvalues that label the irreducible
representations
of!RPlJ
can be used, in addition to those
ofP
z
and
W
Z,
to label the
irreducible representations
ofp(p,
n -
pl.
We are particularly interested in p(3,
I),
the synnnetry group of the special
theory of relativity.
In applying the formalism developed above, we need to make
contact with the physical world. This always involves interpretations. Borrow-
ing from the angolar momentnm theory, in which a physical systemwas given the
attribote
of
angolarmomentum, the labelof the irreduciblerepresentation
of
thero-
tationgroup, we attribote the labels of anirreduciblerepresentationof the Poincare
group, i.e., the eigenvalues
of
the four translation generators and the two Casintir
operators, to a physical system. Sincethe four translation generators are identified
as the three components of momentnm and energy, and their specification implies
their
constancy
over
time,
we havetocometotheconclusion
that
27.5.10. Box.
An
irreducible representation
of
the Poincare group specifies
afree
relativistic particle.
