Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


27.2 AN OUTLINE
OF
LIE
ALGEBRA
THEORY
833
Note that this is consistent with the geometric definition of the invariant measure
given in Proposition 27.1.31 because
L.-1*
= L
g
;
and the matrix of
L;
is
the
inverse of the matrix of L
g
*. The volume element at g, which is invariant on
G'---and therefore has the same value as at the
identity-and
which we denote by
d/1-(g), will be given by
(
ami
(g,
X))
I
d/1-(g) =
d/1-(e)
ea
d'
x =
deC!
i
d'
g,
ax
x=o
(27.26)
density
functions
associated
with
Haar
measure
where we have replaced y with the more suggestive g. The volume element
d'
g is
the ordinary Euclidean volume element
of
ll~.'
evaluated at the parameters corre-
sponding to g. The quantity multiplying
d'
g is called the
density
function. Note
that since we are interested in the derivatives
of
m!
at small values
of
X, we can
take the components of
X to be small, and retain them only up to the first order.
This will sometimes simplify the calculation
of
the invariant Haar measure.
27.1.32.
Example.
Fromthemultiplicationrulefortheone-dimensionalprojectivegronp
given
in Example
27.1.8,
weeasilyfind
Thus the density function is
(al
a4 -
aza3)-2,
and the invariant Haar measure is
d/1-(a)
=
(a!a4
-
a2a3)-2~a.
27.2 An Outline of Lie Algebra Theory
ill
The notion
of
a Lie algebra has appeared on a number
of
occasions both in our
study of vector fields on manifolds and, more recently, in the study of Lie gronps
in the vicinity of their identity elements. Lie algebras play an important role in
the representation theory of Lie groups as well.
It
is therefore worth our effort to
spend some time getting acquainted with the formal structure and properties of
these algebras. We shall restrict our discussion to finite-dimensional Lie algebras.
27.2.1. Definition.
A finite-dimensional vector space V over
JR
(or iC)is called a
Lie
algebra
defined
Lie
algebra over
JR(
or iC)ifthere is a binary operation, called
Lie
muUiplication,
[".J
: V x V-->
Von
V,satisfying
1. [X, Y] =
-[Y,
Xl/or
all X, Y E V (antisymmetry).
2.
[aX
+fJY, Z] =
a[X,
Z] +fJ[y,
ZJ/or
a,
fJ
E
JR
(or iC) (linearity).
3. [X, [Y, Z]] +[Z, [X, Y]] +[Y, [Z, X]] = 0 (Jacobi identity).

834
27. LIE
GROUPS
AND
LIE
ALGEBRAS
subalgebra,
ideal,
and
center
ota
Lie
algebra
Knowing
the
structure
constants,
one
can
reconstruct
the
Lie
aigebra!
The concepts
of
a homomorphism, its kernel, its range, etc. are the same as before.
TodistinguishLie algebras from vector spaces, we shall denote the former by
lowercaseGermanletters as we have donefor the Lie algebras of Lie groups.
27.2.2.Example.
Recall
from
Chapter
t thatan
algebra
isa
vector
space
with
a
product.
If
this
product
is associative, thenone can
construct
a Lie
algebra
out of the associative
algebra
by
defining
[a,b]
ea
ab - ba. In
particular,
the
matrix
algebra
under
commntation
of
matrices
becomes
aLie
algebra,
which
we
denote
by
gl(n,lit)
[orgl(n, iC)]. III
27.2.3. Definition.
Let
Ubea Lie algebra. A subspaceu
of»
is calleda subalgebra
if [X, Y] E u whenever X, Y E u. The subspace u is calledan ideal if [X, Y]
E.U
whenever either X E U or Y E u. The center3
of
U is the collection
of
all X E U
whose Lie multiplication with all vectors
of
U vanishes. A Lie algebra is abelian,
or commutative,
if3 = n.
If
we choose a basis in the Lie algebra n,and express the Lie multiplication
of basis
vectors
as a
linear
combination of basis vectors, we end upwith basis-
dependentstructureconstantsthatsatisfyEquation (27.13).Thestructnreconstants
completely determine the Lie algebra: Given these constants, one can choose a
vectorspace V of
correct
dimension, a basis in
that
space, andimpose the Lie
multiplication law among the basis vectors suggested by the structure constants.
OncetheLiemultiplicationlawforbasisvectorsisestablished,the lawforarbitrary
vectors follows from linearity of Lie multiplication. This procedure induces a
binary operation on
V and turns it into a Lie algebra n.Any other algebra so
constructedwill be isomorphicto
n,
27.2.4.Example.
We
can
classify
all
two-dimensional
Lie
algebras
by
analyzing
their
structure
constants.
LetXl andX2 be anytwo
linearly
independent
vectors
of the two-
dimensional
Lie
algebra
n,
Write
theonly
nonzero
Lie
bracket
as
[Xl,
X2] =
CjXj
+C2X2·
There
are
twocasesto
consider:
Either
C1
= 0 = C2oroneof the
constants
is
nonzero.
The
first
case
corresponds
toa2-dimensional
abelian
Lie
algebra:
fori,j
= 1,2.
Forthesecondcase,
suppose
C1
':f::.
0 and
define
the
vectors
Y es
X2/Cj.
Thenthe
nonzero
Lie
bracket
becomes
[X,Y] = X.
The result of Example 27.2.4 is summarizedas follows:
27.2.5. Box.
There are only two 2-dimensionalLie algebras given by either
one
of
thefollowing nonzero Lie bracket relations:
iii
or
27.2
AN
OUTLINE
OF
LIE
ALGEBRA
THEORY
835
27.2.6.
Example.
The Pauli spiu matrices
(
0
-i)
cz
> i 0 '
fcrm
a Lie algebra under the commutationrelation given by
Thus,
c~k
= 2iE
iki-
Pauli spin matrices are a basis for
su(2).
III
Wayl
basis
for
gl(n,lll.)
27.2.7.
Example.
TheLie group
GL(n,
lll.)
hasgl(n, R), the setofallrealn
xn
matrices,
asitsLiealgebra.ThestandardbasisofthisLiealgebra,alsocalledtheWeylbasis, consists
of
matrices
8ij
that have zeros everywhereexcept at
the
ijth
position. We therefore have
(27.27)
We can readily find the Lie multiplication (commutation relations) for these matrices. We
simply
need
to look at the elements
of
the matrix
of
the
commutator:
([Sij,
eklD
mn
=
(8ijekl)mn
-
(ekleij}mn
=(eij)mr (ekllrn - (ekl)mr
(eij),n
=~~r~~-~~~r~n=~m~~-~~~n
=(eU}mn
8jk
-
(ekj)mn
8Jj.
or
(27.28)
The structure constants, which are naturally
double-indexed,can
be read off from Equation
(27.28):
(27.29)
where
we have
used
a superscript
for
some
of
the Kroneckerdeltasto
conform
tothe position
of
the corresponding index on the LHS.
III
27.2.8.
Example.
Animportantdatum is the
dimension
of
the Lie group(or its associated
Lie
algebra, sincetheyare the same). This datum is
not
apparentin
most
cases
of
interestin
whichthe groupis definedin terms
of
somegeometricproperty.
Forexample,
the
symplectic
group is defined as all linear transfonnations A
that
leave a certain antisymmetric bilinear
fonn
invariant (Example 23.2.2). In
tenns
of
matrices, we have
J=(O
-1
It follows
that
the symplectic group consists
of
all matrices A such that
A'JA=J.
(27.30)

836 27. LIE
GROUPS
AND
LIE
ALGEBRAS
If
we write Ain block
form,
A =
(At!
\.A
21
whereAij aren x n matrices, then,
Equation
(27.30) becomes
or
1)
(At!
o A21
A12)
( 0
A22
=
-1
~)
,
(27.31)
Forthesymplectic
algebra
sp(2n,lR.), we areinterested inthe
matrix
Awhenitis close
to the
identity.
Thismeans
that
Substitutingthese in Equation(27.31) and keeping terms linearin E, we obtainthe following
relations
among
Xij:
(27.32)
It follows
that
weneedn
2
parameters
to
describe
then x n
matrices
XIIand
X22
simultane-
ously.
For the
symmetric
matrices
X12
andX2I. we need
n(n
+1)/2independentparameters
each.
Therefore,
thetotal
number
of
independent
parameters
neededfor(orthe
dimension
of) the symplectic algebrasp(2n,lR) is
III
Althoughour attemptis to give a formal discussionof the Lie algebras and their
structure in this section, we shall do this with an eye to the eventual utility
of
this
discussion in a betterunderstanding of the Lie algebras of Lie groups. To make the
connection between the present formalism and the Lie algebras arising from Lie
groups, we shall make heavy use of matrix groups, i.e.,
GL(n,IR)
[or
GL(n,
iC)]
and its subgroups. Equation
(27.8)
gives a method
of
finding the matrices of the
algebra if those of the group are known:
27.2.9. Box.
Differentiate the matrix with respect to a parameter at the
identity (where all parameters are set equal to zero) tofind the matrix "in
the direction"
of
thatparameter.

27.2 AN
OUTLINE
OF
LIE
ALGEBRA
THEORY
837
27.2.1 The Lie Algebras o(p,n -
p)
and
p(p,n -
p)
Many
of
the
Lie
groups encountered in physical applications are special cases
of
the (pseudo) orthogonal group 0
(p,
n -
p)
and
its associated Poincare group
p
(p,
n -
p).
It
is therefore worthwhile to study their
Lie
algebras in some detail.
Introduce the diagonal matrix
..,=
diag(-I,
-I,
...
,
-1,
1, 1,
...
,1)
.
''-.,.--'
p times n - p times
and note thatthe (pseudo) orthogonal group 0
(p,
n -
p)
consists
of
n x n matrices
that leave the bilinear form x . x
==
x'..,x invariant for x E En. This means that the
matrices Awill have to satisfy
A'..,A
=..,
=}
(detA)2 =1.
(27.33)
worthogonal
matrices
Such
matrices are called ..,-orthogonal.
The
fact that 0
(p,
n -
p)
is a group
and
that
..,-1
= ..,can be used to show that
(27.34)
ormoehronous
and
proper
ortnocnronous
Lorentz
transformations
Timelike,
spacelike,
and
null
vectors:
JR'
as
the
set
of
events
future
light
Gone
27.2.10.
Example.
TIlE
LORENTZ
GROUP
The groupof the specialtheory of relativityis the full Lorentz group
0(3,
1). This isthe
group
of
transformations
that
leave the invariant
length
6
T/ij
XiXj
=
-xr
-
xi
-
x~
+xt
ee
x6
-xr
-
xi
-
xi
of a 4-vector (XI, X2, X3, Xo =
ct)
iovatiant.The (0,
OJ-components
of Equations(27.33)
and (27.34)yield
2 2 2 2 1
aOO
- alQ -
llZo
- a
30
= ,
a50
-
a51
-
a52
-
a53
= 1. (27.35)
Either one
of
these equations implies
that
aOO
2: 1 or
aOO
~
-1.
Lorentz transformations
forwhichcoo
~
1arecalledorthuchronons. Since
detl
= +1and100= +1,theidentity
belongs to the subset consisting
of
transformations with det A
==
+1
and
aoo ::: 1.
Such
transformations fonn a snbgroup of
0(3,1)
called the proper urthochronuns Lorentz
transformations, and have the property that they can be reached continuously from the
identity.
Depending on whether x . x
> 0, x . x < 0, or x . x = 0, the vectorx is called timelike,
spacelike, or
null,
respectively. In
the
special theory
of
relativity IR
4
becomes the set
of
events.
At
every event x the set ]R4 is divided
into
5 regions:
I.
All eventsy = (Ylo Y2, Y3, YO)to whichonecangofromxby materialobjects,with
speed less than
c.Iie
to the future of x, i.e.,
YO
-
xo
c- 0,
and
are timelike:
(YO
-xO)2>
(YI
_XI)2
+(Y2
-X2)2
+(Y3
-X3)2.
They
form
a 4-dimensional subset of IR
4
and
are said to lie
inside
the
future
light
cone.
6n is common to label the time coordinate with index 0 rather than 4. We shall use this convention.
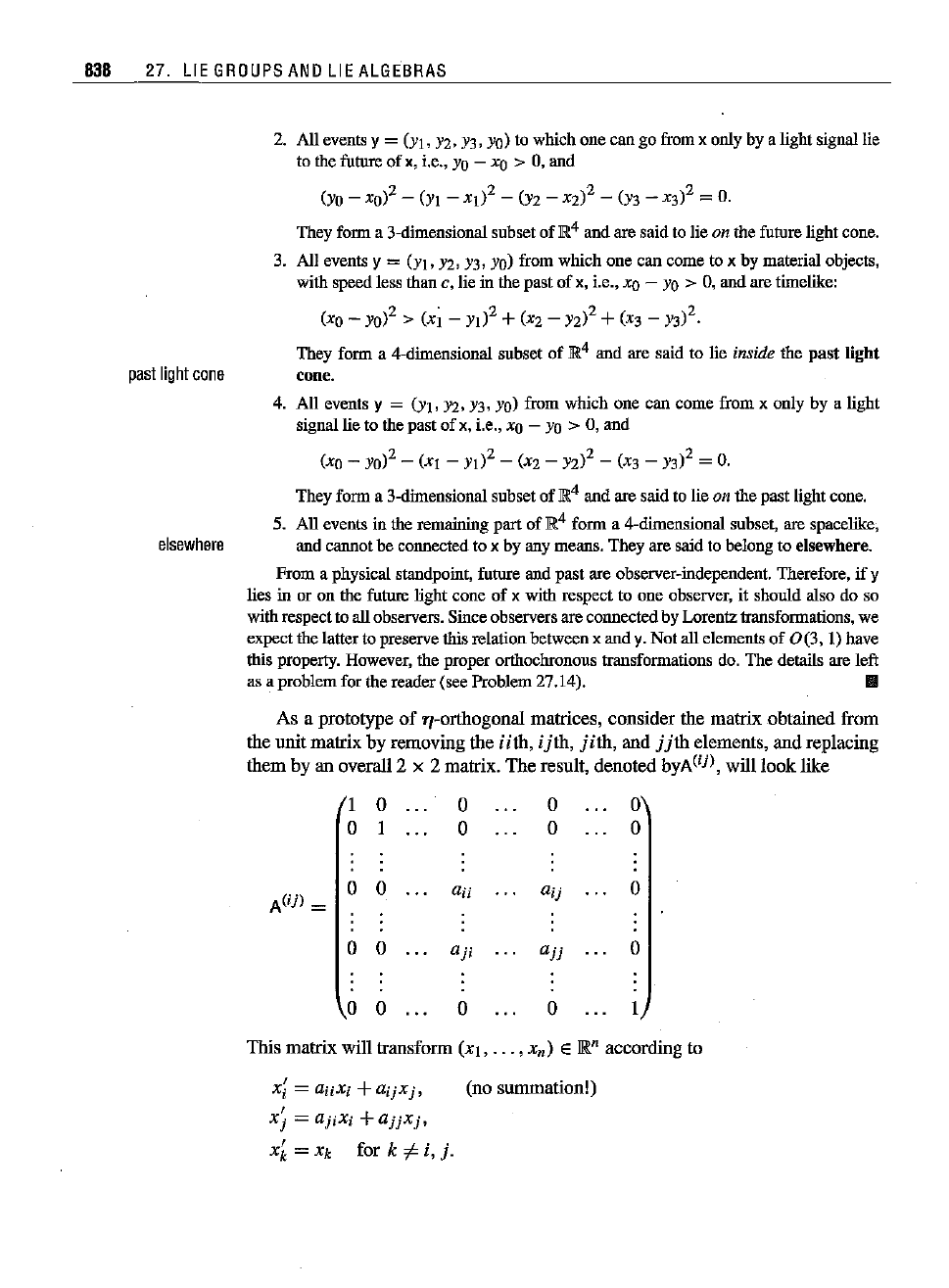
past
light
cone
838 27. LIE
GROUPS
ANO
LIE
ALGEBRAS
2. All eventsY= (YI, Y2, Y3,
YO)
to which one can go from x onlyhy a light signallie
to the
future
of x, i.e.,
YO
-
xo
> 0, and
(YO
- xO)2 - (YI -
XI)2
- (Y2 - X2)2 - (Y3 - X3)2 =
o.
They
form
a 3-dimensional subset
of]R.4
andaresaidtolie on the
future
lightcone.
3. All
eventsy = (Yl, Y2, Y3,
YO)
fromwhichone cancometo x by
material
objects,
withspeedless
than
c. lie in thepastof x, i.e., XQ -
YO
> 0, andare
timelike:
(XO
- YO)2 > (xi - YI)2 +(X2 - Y2)2 +(X3 - Y3)2.
They
form
a 4-dimensional subsetof
lR
4
andaresaidto lie
inside the past light
cone.
4. All events y
= (Ylo
Y2,
Y3,
YO)
from which one can come from x only hy a light
signal lie to the past
of
x, i.e., XQ -
YO
> 0, and
(xo -
YO)2
- (Xl - YI)2 - (X2 - Y2)2 - (X3 - Y3)2 =
O.
They fonn a 3-dimensionalsubset of
Jll.4
and are said to lie onthe past light coue.
5.
All
eventsinthe
remaining
part
of
lR.
4
forma 4-dimensional subset, are spacelike,
elsewhere
and
cannot
be
connected
tox by any
means.
Theyaresaidto
belong
toelsewhere.
From
aphysical
standpoint,
future
andpastare
observer-independent.
Therefore,
if y
lies
in oron the
future
lightcone of x withrespectto one
observer,
it shouldalso do so
with
respect
to all
observers.
Since
observers
areconnected by
Lorentz
transformations,
we
expectthe
latterto
preserve
this
relation
betweenxandy. Notall
elements
of
0(3,1)
have
this
property.
However,
the
proper
orthochronous
transformations
do. The
details
are
left
as a problem for the reader (see Problem 27.14).
III
As a prototype
of
"I-orthogonal matrices,
consider
the
matrix obtained from
the
unit
matrix by removing the
iith,
ijth,
jith,
and
jjth
elements,
and
replacing
them
by an overall 2 x 2 matrix.
The
result,
denoted
byA
(ij),
will
look
like
I 0
0
0 0
0
I
0
0 0
A(ij) =
0 0
au
aij
0
0
0
an
ajj
0
0
0 0 0
I
This matrix will
traasform
(Xl,
...
,X
n
)
E
~n
according to
,
(no summation!)
Xi =
aiiXi
+aijXj,
t
Xj
=
ajiXi
+ajjXj,
,
forkt=i,j.
Xk
=Xk

27.2 AN
OUTLINE
OF
LIE
ALGEBRA
THEORY
839
In
order for
A(ij)
to leave the bilinear form
X'T/X
invariant, the 2 x 2 submatrix
(:~~
;~~)
mustbe
either
a
rotation
(corresponding
to thecase
where
i, j
::::
p or
i, j >
p),
or a Lorentz boost? (corresponding to the case where i ::; P and j >
p).
In
the first case, we have
-
Sine)
CDSe
'
and in the second case
- sinh
g)
coshs ,
where gea
tanh-I
(v/c)
is the "rapidity."
The matrices of the algebraare obtainedby differentiation
ate
=0 (or g=0).
Denoting these matrices by
Mij,
we readily find that for the case
of
rotations, Mij
has
-I
at the
ijth
position, +I at the
jith
position, and 0 everywhere else. For
the case of boosts,
Mij
has
-I
at the
ijth
and the
jith
position, and 0 everywhere
else. Both cases can be described by the single relation
(M
..
)m _
""
,m
I)
1 - TJilUj
-1}jl
o
i I
Mij
=
-Mji.
It
is convenientto have allindices in the lower position. So, we multiplyboth sides
by rymk to obtain
(27.36)
Lie
brackets
forthe
algebra
alp,n- p)
Poincare
group
as
(n+1)
x
(n+1)
matrices
We can use Equation (27.36) to find the Lie multiplication (in this case, matrix
commutation relations) for the algebra 0(p, n -
p):
(27.37)
The Lie group 0
(p,
n -
p)
includes rotations and Lorentz transformation.
Another group with considerable significance in physics is the Poincare
group
p (p, n -
p),
which includes translations'' in space and time as well. An element
of
pep,
n - p) transforms x E
JR"
to x' = Ax +u, where U is a columo vector
representing the translationpart of the group.
It
is convenient to introducematrices
to represent these group operations. This is possible
if
we represent an elementof
JR"
as an (n +I)-column whose last elementis an insignificant
I.
then, the reader
may check that a Poincare transformation can be written as
(27.38)
7The
elementary
Lorentz
transfonnationsinvolving onlyone space
dimension.
80
ne
can
think
of
the
Poincare
group
asa
subgroup
of the
group
of
affine
motionsinwhichthe
matrices
belongto 0 (p, n- p)
rather
than
GL(n,
JR.).

840
27. LIE
GROUPS
ANO
LIE
ALGEBRAS
where A is the n x n matrix
of
O(p, n - p),
and
u is an n-dimensional
column.
vector.
The
Lie algebra
of
the Poincare group is obtained by differentiating the (n +
I) x (n +1) matrix
of
Equation
(27.38).
The
differentiation
of
the matrix A will
give
o(p, n - p)
of
Equation (27.37).
The
translation part will
lead
to matrices Pi
with matrix elements given by
(pd
z
=
8f8
z+!
'* (Pi)kZ =
~ik8Z+!·
These matrices satisfy the following Lie multiplicationrules:
(27.39)
It
then
follows that the full
Poincare
algebra
p
(p,
n -
p)
is described by the
following
Lie
brackets:
Lie
brackets
forthe
Poincare
algebra
p(p,
n- p)
[Mij,
MkzJ
=
~ikMjZ
-
~ilMjk
+
~jlMik
-
~jkMil,
[Mij, Pk] =
~ikPj
-
~jkPi,
[Pi, Pj] =
o.
(27.40)
derivation
algebra
of
a
Lie
algebra
adjoint
algebra
ofa
Lie
aigebra
Illustration
of
homomorphism
of
su(2)
andits
adjoint
using
Pauli
spin
matrices
27.2.2 Operations on Lie Algebras
27.2.11. Definition. Letu beaLie
algebra.
Alinearoperator0 : U--> Usatisfying
O[X, Y] = [OX, Y] +[X, OY]
iscalleda derivation of n,
Although
the
product
of
two derivations is
not
a derivation, their commutator
is. Therefore, the set
of
derivations
of
a
Lie
algebra Uthemselvesform a
Lie
algebra
:D.
under
commutations,
which
is called the
derivation
algebra.
Recall that the infinitesimal generators
of
the
adjoint action
of
a
Lie
group
on
its algebra were given by
a~e
[Equation (27.14)]. We
can
apply this to a general
Lie
algebra Uby fixing a vector X E U
and
defining the
map
adx
: U--> Ugiven
by
adx(Y)
= [X, Y]. The reader
may
verify that
adx
is a derivation
of
Uand that
ad[X,Y]
=
[OOx,
adv], Therefore, the set
a~.
==
{OOx
IX E n] is a
Lie
algebra, a
subalgebra
of
the derivation algebra
D;
of
U,
and is called the
adjoint
algebra
of
U.
There
is a natoral homomorphism
1/r
: U-->
a~.
given by 1/r(X) =
adx
whose
kernel is the center
of
e. Furthermore,
a~.
is an ideal
of
:D
e-
27.2.12. Example. We constructthe matrixrepresentation of the operators in the adjoint
algebra
of
5u(2)
with Pauli spin matrices as a basis.
From
we conclude that the first
column
of
the
matrix
of
ad
a l
is zero.
From

27.2 AN
OUTLINE
OF
LIE
ALGEBRA
THEORY
841
we
conclude
that
thesecond
column
ofthe
matrix
ofada
1
haszerosforthe
first
two
elements
and 2i for the last.Similarly, from
we conclude
that
the
third
columnof the
matrix
of
adu
l
has zerosfor the
first
and
third
elements
and- 2i forthe
second.
Thus,
the
matrix
representation
of ad
rr1
is
(
0 0
ad
ot
= 0 0
o 2i
Likewise,we can
obtain
theothertwo
matrix
representations;
they
are
adu2=(~
-2i
o
2i)
o 0 ,
o 0
-2i
o
o
~)
.
The readermay readilyverifythat [ada
j
, ad
uk
] =
2iEjkladq.
If
1jf
is an automorphism
of
n, i.e., an isomorphism
of
n onto itself, then
III
ady,(X)
=
1/J
a
adx
a
1/J-l
VXE~.
(27.41)
Since
adx
is an operator on the vector space e,
one
can define the trace
of
adg,
However, the notion
of
trace attains a far greater sigoificance
when
it is combined
with the notion
of
composition
of
operators.
For
X, Y E e,define
(X
[Y)
sa
tr(adx
0
ady).
(27.42)
Thenone can showthat (.[.) is bilinear
and
symmetric.
It
becomes an innerproduct
if the
"vectors"
of theLie
algebra
arehermitian
operators
on somevector
space
V,
or
if
the underlying vector space is over ~ (see Proposition 3.6.6 in Chapter 3).
Furthenmore, (.[.) satisfies
([X,Yll Z) +([X, Z] IY) =
O.
(27.43)
Killing
lorm
01
a
Lie
algebra
27.2.13. Definition. The symmetric bilinearform (.[.) :
~
x
~
--> n defined by
(27.42) is called the Killing form
of»,
It
is an inunediate consequence
of
this definition and Equation (27.41) that the
Killing fonm
of
~
is invariant
under
all automorphisms
of
n.
Most
mathematicians
seemtohavelittleorno interestin
history,
so
that
oftenthename
attached
to akey
result
is
that
of thefollow-up
person
who exploited anideaor
theorem
rather
than
its
originator
(the
Jordan
form isdueto
Weierstrass,
Wedderburn
theory
to
Cartan
and
Malien).
No
one.
has
suffered
fromthis
ahistoricism
more
than
Killing.For
example,
the
so-called"Carlansub-algebra"and"Carlanmatrix"were definedandexploitedby Killing.

842 27. LIE GROUPS AND LIE ALGEBRAS
He exhibitedthe characteristicequation of an arbitrary element of the Weylgroup when
Weyl
was 3 years old and listed the orders
of
the Coxeter transformation 19 years before
Coxeter was bern!
Wilhelm
Karl
Joseph
Killing
(1847-1923)
began
university studyin Munster in 1865
but quickly moved to Berlin and came under the influence of Kummer and Weierstrass.
From 1868 to 1882 much
of
Killing's energy was devoted to teaching at the gymnasium
levelin Berlin and Brilon (southof Miinster). At one stage,whenWeierstrass wasurging
him to write up his research on space structures, he was spending as
much
as 36 hours
per
week
in the classroom or tutoring. (Now
many
mathematicians consider 6 hours a
week
an
intolerableburden!)On
the
recommendation
of
Weierstrass,Killingwas appointedprofessor
of
mathematics at the Lyzeum Hosianum in Braunsberg,
in
EastPrussia (now Braniewo in
the region
of
OIsztyn, in Poland). This was a college
fouuded
in 1565by Bishop Stanislaus
Hosius,whosetreatiseon the Christianfaithranto 39 editions! The
main
object
of
the college
was the training
of
Roman Catholic clergy, so Killing
had
to teach a wide range
of
topics,
including the reconciliation
of
faith
and
science.
Although
he was isolated mathematically
during his
ten
years in Braunsberg, this was the
most
creative
period
in his mathematical
life. Killing produced his brilliant
work
despite worries about the health..!
of
his wife and
seven children, demanding administrative duties as
rector
of
the college and as a member
and chairman
of
the City Council,
and
his active role in the Church
of
St. Catherine.
What
we now callLie algebras wereinventedby
the
Norwe-
gian
mathematician
SOphU5
Lie about 1870 and independently
by Killing about 1880. Lie was seeking to develop an approach
to the solution of differential equations analogous to the Galois
theory
of
algebraic equations. Killing's consuming
passion
was
non-Euclidean geometries and their generalizations, so he was
led to
the
problem
of
classifyinginfinitesimal motions
of
a rigid
body
in any type
of
space
(or Raumformen, as he calledthem).
In 1892he was called
back
to his native Westphalia aspro-
fessor
of
mathematics at the University
of
Miinster,
where
he
was quickly submerged in teaching, administration,
and
charitable activities. He was Rec-
tor Magnificus for some
period
and
president
of
the St. Vincent de Paul charitable society
for
ten
years. Killing's
work
was neglected partly
because
he was a
modest
man
with high
standards; he vastly underratedhis own achievement. His interest was geometry,
and
for this
he
needed
all real Lie algebras. To obtainmerely the simple Lie algebras over the complex
numbers did not appear to
him
to be very significant. Another reason was due to Lie,
who
was quite negative aboutKilling's work. At the top
of
page
770
of
a three-volume
joint
work
of
Lie and Engel we find the following less than generous comment
about
Killing:
"With
the exception
of
the preceding unproved
theorem.
..
all the theorems
that
are correct are
due to Lie
and
all the false ones are
due
to Killing!"
Killing was conservative in his political views
and
vigorously opposed the attempt to
reform the examinationrequirements for graduate students at the University
of
Miinsterby
deleting the compulsory study
of
philosophy. Engel conunents "Killing could uot see
that
for
most
candidates the
test
in philosophy was completely worthless," He
had
a profound
patriotic love
of
his country, so that his last years (1918-1923) were deeply
pained
by the
collapse
of
social cohesion in Germany after the War
of
1914-18.
