Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


27.3
REPRESENTATION
OF
COMPACT
LIE
GROUPS
853
Characters satisfysimilar relations: Equation (24.11) becomes
fa
X(a)(g)X
(P)*
(g)
dJ.L
g
= 8
a
p,
(27.55)
and the useful Equation(24.16) turns into
l
IX(g),2
dJ.Lg
=
L>~'
(27.56)
G a
Thisformulacanbeusedtotest forirreducibilityofarepresentation:If theintegral
is unity,the representation is irreducible; otherwise, it is reducible.
Finally,we statethecelebrated
Peter-
Weyltheorem(foraproof,see [Barn 86,
pp.I72-173])
Peter-Weyllheorem
27.3.12. Theorem. (Peter-Weyltheorem) The functions
,In;
1';;a)
(g),
Va
and I s t. j s n
a,
form
a complete
set
of
functions in
.c
2
(G),
the Hilbert space
of
square-integrable
functions on
G.
If
u E
.c
2
(G), we can write
where
b5
= n
a
1u(g)1';;a)*(g) d/Lg.
G (27.57)
y=e+e'.
The
Peter-Weyl
theorem
isthe
generelization
ofthe
Fourier
series
expansion
of
periodic
functions.
27.3.13. Example.
Equation
(27.57)
isthe
generalization
ofthe
Fourier
series
expansion
of
functions. The connectionwith Fourier series becomes
more
transparent if we consider
a particular compact.group. The unit circle 8
1
is a one-dimensional abelian compact 1-
parameterLiegroup.
In
fact,fixingan"origin" onthecircle,anyotherpointcanbedescribed
by the parameter8. the angulardistancefrom
the
point
to
the
origin. 8
1
is obviouslyabelian;
it is also compact, because it is a bounded closed region of
IR.2
(BWHB theorem). By
Theorem 24.2.3, which holds for all
Lie
groups, all irreducible representations
of
8
1
are
I-dimensional.
So
T(~)(g)
-+
T(a)(e).
Furthermore,
T(a)(e)T(a)(e') = T(a)(e + e').
IJ
Differentiating
both
sides withrespect to
8'
at 0' = 0 yields the differential equation
(a) dT(a) I
dr(a)
I _dT(a)
T (e)
-,-
=
--
=--,
de
0'=0
dy y=O de
The
solution to this DE is
Ae
aB.
Since
T(a)
are unitary, and since a I-dimensional unitary
matrix must looklike i
fIJ
•we must have A =1.Furthermore, eand()+21fareidentifiedon
the
unit circle; therefore, we
must
conclude
that
a is i times an integern,
which
determines
the
irreducible representation. We label the irreducible representation by n
and
write
T(n)(e) = e
inO
,
n = 0 ± 1± 2
....
ThePeter-Weyl theoremnowbecomestheruleofFourierseriesexpansionofperiodicfunc-
tions. This
last
property follows from the fact
that
any function u : SI
-+
JR
is necessarily
periodic.
II

854
27. LIE
GROUPS
ANO
LIE
ALGEBRAS
There are many occasions in physics where the state functions describing
physical quantities transform irreducibly
under
the action
of
a
Lie
group (which
we assume to be compact). Oftenthis
Lie
group also acts on the underlying space-
time manifold. So we have a situation in which a
Lie
group G acts on a Euclidean
space
R
n
as well as on the space
of
(square-integrable)functions L
<R
n
).
Therefore,
the functions
(¢;(a)
(xj],
belonging to the
ath
irreducible representation transform
amongthemselvesnot only because
of
the indexi, but also because
of
the argument
xER
n.
To see the connection between physics
and
representation theory, consider
the transformation
of
the simplest case, a scalar function. As a concrete example,
choosetemperature. Toobserver
0 at the
comer
of
a room 8 meters long, 6 meters
wide,
and
3 meters high, the temperature
of
the center
of
the
room
is given by
0(4,3,
1.5) where O(x, y, z) is a function that gives 0 the temperature
of
various
points
of
the room. Observer
0'
is sitting in the middle
of
the floor, so that the
center
of
the
room
has coordinates
(0,0,
1.5).
0'
also has a function that gives
her the the temperature at various points.
But
this function
must
necessarily be
different from
0 because
of
the different coordinates the same points have for
o and
0'.
Calling this function 0', we have 0'(0,
0,1.5)
=
0(4,3,1.5),
and
in
general,
O'(x',
y',
z')
= O(x, y, z),
where (x', y', z') describes the same point for
0'
that (x, y, z) describes for O.
In the context
of
representation theory, we
can
think
of
(x', y', z')as the trans-
formed coordinates obtained as a result
of
the action
of
some group: (x',
s'.
z') =
g . (x, y, z), or x' = g . x. So, the equation above
can
be written as
O'(x') = O(x) =
O(g-t.
x)
or
O'(x)
=O(g-l.x).
It
is natural to call 0' the transform
of
0
under
the action
of
g and write 0' = T
gO.
This is one way
of
constructing a represeutatiou [see the comments afterEquation
(24.1)]. Instead
of
g-l
on the left, one
could
act with g on the right.
When
the physical quantity is
not
a scalar, it is natural to group together the
smallest set
of
functions that transform into
one
another. This leads to the set
of
functions that transform according to a row
of
an irreducible representation
of
the
group. In some sense, this situation is a combination
of
(24.1)
and
(24.37).
The
reader may verify that
n.
Tg¢;(a)(x) =
LTj~a)(g)¢;a)(x.g-l)
j=l
(27.58)
defines a representation
of
G.
We now use Box 27.1.26
to construct an irreducible representation
of
the
Lie
algebra
of
G from Equation (27.58). By the definition
of
the infinitesimal action,

27.3
REPRESENTATION
OF
COMPACT
LIE
GROUPS
855
we let g = exp(et) and differentiate both sides with respect to t at t =
O.
This
yields
fr
TexP(Et):i
a)
(x)
1,=0,
=
~
:t
(TJa\exp(et))¢t)(x . exp(
-em}
1,=0
=Lj
~
ji(~,)¢~OI)(X)
where we have defined the matrices
:l)
ji
(e) for the LHS. The derivative in the first
sum is simply
'.rJ~)
(e) the representation
of
the generator e of the l-parameter
gronp
of
transformations in the space of functions
{¢i
a
)}.
The derivative in the
second sum can be found by writing x'
(t) = x . exp(-et) and differentiating as
follows:
where we used Equation (27.18) and defined
Xk(x;
e) by the last equality. We also
changed the coordinate index to Greek to avoid confusing it with the index of the
functions. Collecting everything together, we obtain
(27.59)
where
X" (x; e) is the vth component of the infinitesimal generator
of
the actiou
induced by e
E g. We shall put Equation (27.59) to good use when we discuss
symmetries and conservationlaws in Chapter30. The derivative with respectto the
functions, although meaningless at this point, will be necessary when we discuss
conservation laws.

856
27. LIE
GROUPS
AND
LIE
ALGEBRAS
27.4 Representation of the General Linear Group
GL(V) is not a compact group, but we cau use the experience we gained in the
aualysis
of
the symmetric group to find the irreducible representations of GL(V).
The key is to construct tensor product spaces
of
V-which,
as the reader may
verify, is a carrier space
of
GL
(V)-aud
look for its irreducible subspaces. In fact,
if r is au arbitrary positive integer,
T : G --* GL (V) is a representation, aud
V®,
==V®·
..
®V.
~
T
times
Then T®' : G --*
GL(v®r),
given by
[T®r(g)](Vt,
...
,v
r
) sa
T~r
(VI,
...
, v
r
)
= Tg(VI) ®
...
® Tg(v,),
is also a representation. In particular, considering V as the (natural) carrier space
for
GL(V),
we conclude that T®r :
GL(V)
--*
GL(v®r)
is a representation.
This tensor product representation is reducible, because as is evident from
its definition,
T~'
preserves auy symmetry
of
the tensor it acts on. For example,
the subspace of the full
n
r
-dimensional tensor product space consisting of the
completely symmetric tensors of the type
t, sa L
VnCI)
® V
n
(2) ®
...
®
vnC')
1rES
r
is invariaut. Similarly, the subspace consisting of the completely autisymmetric
tensor
products-the
r-fold wedge
products-is
invariant
To reduce V®', we choose a basis
{eklk=1
forV.
Then the collection of n
r
tensor products {ek,®
...
®ek,},
where each k
i
runs from 1to n, is a basis for V®'.
An invariaut subspace of v®r is a spau of linear combinations of certain of these
basis vectors. Since the only thing that distinguishes among {ek, ®
...
® ek,} is a
perroutation of the r labels, we star! to see the connection between the reduction
of
v®r aud S,. This conoection becomes more evident
if
we recall that the left
multiplication of the group algebra of S; by its elements provides the regular
representation, which isreducible. The irreduciblerepresentations are the minimal
ideals of the algebra generated by the Young operators.
The same idea works here as well: Certain linear combination of the basis
vectors of v®r obtained by perroutations cau serve as the basis vectors for irre-
ducible representations of
GL(V).
Let us elaborate on this. Recall that a Young
operatorof
S; is written in the forro Y = QP where Qaud P are linearcombina-
tions
of
perroutations in Sr. Y has the property that
if
one operates on it (via left
multiplication) with all perroutations of
S"
one generates a minimal ideal, i.e., au
irreducible representation
of
S,. Now let Y be a Young operator that acts on the
indices
(kl'
...
,
k,),
giving linear combinations of the basis vectors of V®'. From
the minimality
of
the ideal generated by Y aud the fact that operators in GL(V)

connection
between
the
Young
tableaux
and
irreducible
representations
of
GL(V)
27.4
REPRESENTATION
OF
THE
GENERAL
LINEAR
GROUP
857
permutethe factors in ek
j
181·
. ·l8Iek, in allpossibleways,it shouldnow be clearthat
if
we chooseany single basis vectorek
j
l8l
" •
181
ek,,thenY(ek
j
l8l
" 'l8Iek,) generates
an irreducible representation
of
GL (V). We therefore have the following:
27.4.1.
Theorem.
Let
{eklk~1
be any basis
for
V. Let Y = QP be the Young
operator
of
S; that permutes (and takes linear combinations of) the basis vectors
{ekj
181
...
181
ek,.}. Then
for
any given such basis vector, the vectors
{T~rY(ekl
181···181
ek,) Ig E
GL(V)}
span an irreducible subspace
ofVe
r.
A basis
of
such an irreducible representation can be obtained by taking into
accountall the Young tableaux associated withthe irreduciblerepresentation.
But
which
of
the symmetry types will be realized
for
given values
of
n and r? Clearly,
the Young tableau should not contain more than
n rows, because then one
of
the
symbols will be repeated in a column, and the Young operator will vanish due to
the antisymmetry in its column indices. We
can
therefore restrict the partition (A)
to
(A) =
0,1,
A2,
...
, An), Al +...
+A
n
= r,
Let
us consider an example for clarification.
27.4.2. Example. First,letn = r = 2. Thetensor
product
spacehas2
2
= 4
dimensions.
To
reduce
it, we
consider
the
Young
operators,
which
correspond
toe +
(kl,
kl)
ande -
(kt.
k2)· Letusdenotethese
operators
by VIandVl. respectively. By
applying
eachone to
a
generic
basis
vector
ekl ® ek2' we can
generate
all the
irreducible
representations.
The
first
operator
gives
VI(ekj e
ek,)
= ekj e ek, + ek, ® ek!'
where kl and k2 can be 1 or 2. For kl = ka = 1, we get
2el
®
ei.
For kl = 1, k2 = 2,
or kl
= 2, k2 = 1, we get
el
® e2 +
ez
® ej , Finally, for kl = k2 = 2, we get 2e2 ® e2.
Altogether,
we
obtain
3
linearly
independent
vectors
that
are
completely
symmetric.
When
the
second
operator
actsona
generic
basis
vector,
itgives
Y2(ekl
® ek2) = ekl ®
ek2
-
ek2
® ekl'
Theonlytime
that
this
is notzerois whenkl andk2 are
different.
hi
either
case.we get
±(el
®
ez
-
C2
® el)·
This
subspace
is
therefore
one-dimensional.
The
reduction
ofthe
tensor
product
spacecan
therefore
be
written
as
3D
symmetric
subspace
Next,letus
consider
thecaseof n = 2. r = 3. The
tensor
product
space
has2
3
= 8
dimensions,
To
reduce
it,weneedto
consider
all
Young
operators
of 83.
There
are
four
of
these.
corresponding
tothefollowing
tableaux:

858 27. LIE
GROUPS
ANO
LIE
ALGEBRAS
Let us denote these operatorsby
Vb
Y2,Y3.and Y4,respectively.By applying each one to
a generic basis vector ekl ®
ek2
® ek3' we can generate all the irreducible representations.
The firstoperatorgives
VI (ekl ® ek2 ® ek3) =ekl ® ek2 ® ek3 + ekl ® ek3 ® ekz +ek2 ® ekl ® ek3
+~®~®~+~®~®~+~®~®~,
where
zj
, kz,
andkz
can be 1 or 2.
Forkl
= kZ = k3 = I, we get Sej
®el
®el·Forthe
case where two of the ki'S are 1 and the thirdis 2, we get
For thecase where two of the
ki'S
are 2 andthe third is I, we get
Finally, for
kl
= kz = k3 = 2, we get 6ez ® ez ® ez. Altogether, we obtain 4 linearly
independent vectors that are completely symmetric.
When the second operator acts on a generic basis vector,
it gives
lO
Yz(ek, ®
ek, e ek,) = [e -
(kl,
k3)][e +
(kl,
kz)] (ek, e ek, ® ek,)
= ekl ®
ek2
®
ek3
+ ekz ® ekl ® ek3
-ek3
®ekz
®ekl
-ek2
®ek3
®ekt"
If all threeindices are the same, we get zero. Suppose
kl
= 1. Then kz can be 1or 2. For
k2 = 1, we must set k3 = 2 to get e2 ® ei ®
ei
- ej ® e2 ®
ej
. For k2 =2, we must
set
k3 = 1 to obtain el ® ez @ e2 -
ez
® ei ®
ez.
If
we start with
kl
= 2, we will not
produce any new vectors, as the reader is urged to verify. Therefore, the dimension of the
irreducible subspace spanned by the second Young tableau is 2.
The action of the third operator on a generic basis vector yields
Y3(ek,
e ek, ® ek,) = [e -
(kl.
kz)][e +(klo k3)](ek, ® ek, ® ek,)
= ekt ® ek2®
ek3
+ek2 ® ekl ®
ek3
-ek3
® ek2® ekl -
ek3
® ekl ® ek2'
The readermay checkthat we obtain a two-dimensionalirreduciblerepresentation spanned
by ej ® ej ®
ez
- ez ®
el
® el and
"I
® eZ®
ez
- eZ®
ez
®
ej.
The fourth Young operator gives zero because it is completely antisymmetric in three
slots and we have only two indices. The reduction of the tensor product space can therefore
lOWhen a symmetric group is considered as an abstract
group-as
opposed to a group
of
transformations-e-we may multiply
permutations (keep track
of
how each numberis repeatedly transformed) from left to right. However, since the permutations here
act on vectors on their right, it is more natural to calculate their products from right to left.
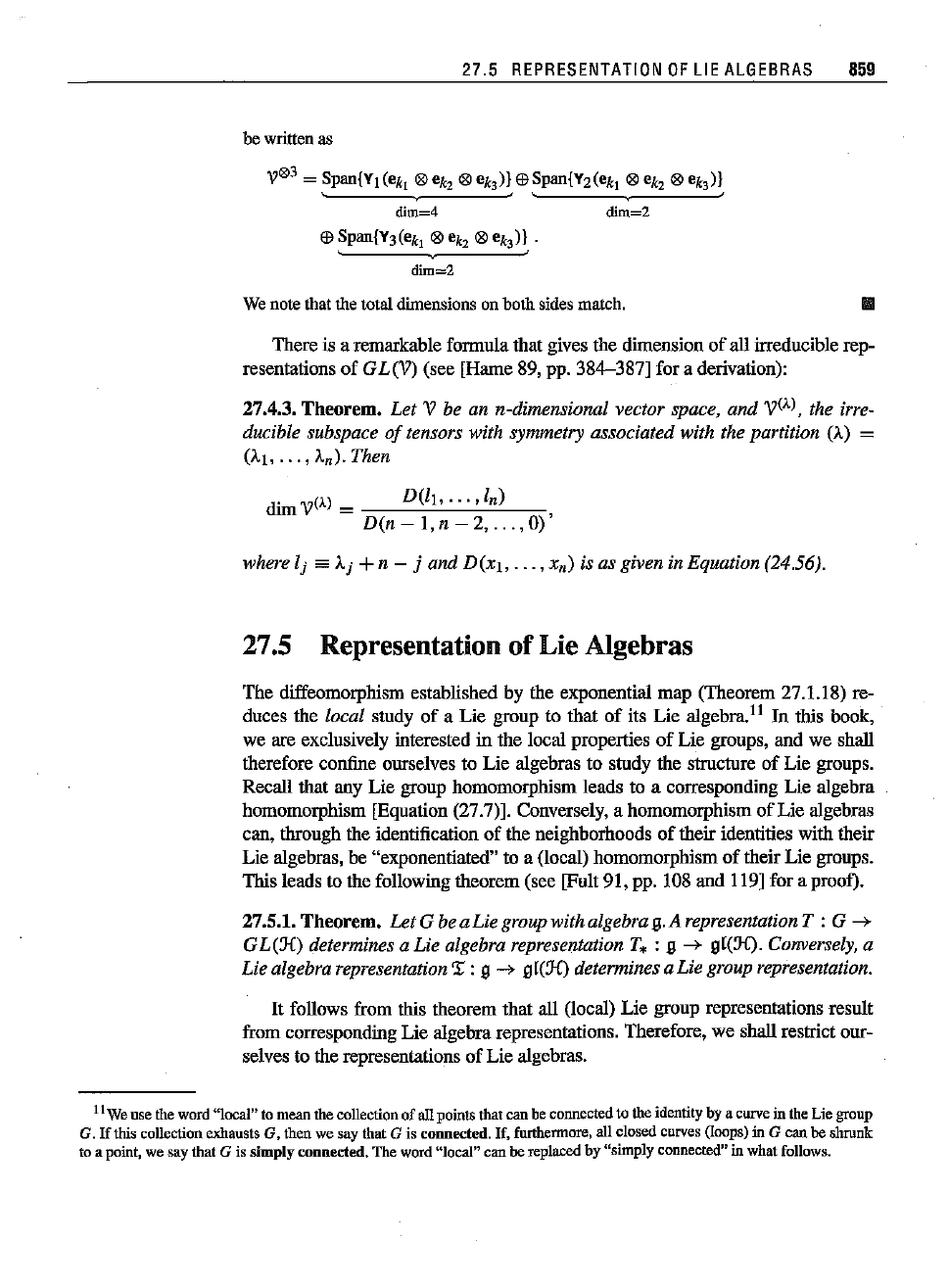
27.5
REPRESENTATION
OF
LIE
ALGEBRAS
859
be
written
as
dim=4
Ell
Span{Y3(ek,
<8l
ek,
<8l
ek,)} .
dim=2
dim=2
Wenote
that
the
total
dimensions
on
both
sides
match.
III
There is a remarkable formula that gives the dimension
of
all irreducible rep-
resentations of
GL(V)
(see [Harne 89, pp. 384-387] for a derivation):
27.4.3.
Theorem.
Let V be an n-dimensional vector space, and
V(A),
the irre-
ducible subspace
of
tensors with symmetry associated with the partition (A) =
(AI,
...
, An). Then
dim V(A) =
D(l[,
...
,In) ,
D(n - 1, n - 2,
...
, 0)
where
Ij sa Aj +n - j and
D(x[,
...
,x
n)
is as given in Equation (24.56).
27.5 Representation of Lie Algebras
The diffeomorphism established by the exponential map (Theorem 27.1.18) re-
duces the
local study
of
a Lie group to that
of
its Lie algebra.'! In this book,
we are exclusively interested in the local properties
of
Lie groups, and we shall
therefore confine ourselves to Lie algebras to stody the structure
of
Lie groups.
Recall that any Lie group homomorpbism leads to a corresponding Lie algebra
homomorphism [Equation (27.7)]. Conversely, a homomorphism
of
Lie algebras
can, through the identification of the neighborhoods
of
their identities with their
Lie algebras, be "exponentiated" to a (local) homomorphism
of
their Lie groups.
This leads to the following theorem (see [Fult 91, pp. 108 and 119] for a proof).
27.5.1.
Theorem.
LetGbeaLiegroupwithalgebrag.ArepresentotionT:
G-->
GL(Jf)
determines a Lie algebra representation T. : g --> gl(Jf). Conversely, a
Lie algebra representation
X : g
-->
gl(Jf)
determines a Lie group representation.
It
follows from this theorem that all (local) Lie group representations result
from corresponding Lie algebra representations. Therefore, we shall restrict our-
selves to the representations of Lie algebras.
11
Weusetheword
"local"
tomeanthecollectionof allpoints
that
canbe
connected
to the
identity
bya
curve
intheLie
group
G.lfthis collection
exhausts
G. thenwe saythatGis connected.If,furthermore, all closed
curves
(loops)in G canbe
shrunk
toa
point,
wesay
that
G is simplyconnected.The
word
"local"
canbe
replaced
by
"simply
connected"
in
what
follows.

860 27. LIE
GROUPS
ANO
LIE
ALGEBRAS
27.5.1 Representation of Subgroups of
GL(V)
Let 9 be any Lie algebra with basis vectors {X;}. Let a representation T map
these vectors to
{Ti} E
gl(~
for some carrier space Je. Then, a general element
X
=
Li
aiXi
of
9 will be mapped to T =
Li
aiTi. Now snppose that
~
is a
snbalgebra
of
g. Thenthe restriction
of
T to
~
provides a representation
of~.
This
restriction
may
be reducible.
If
it is, then there is an iuvariant subspace Jet
of
Je.
It follows that
(blTx
10)
= 0
vX E
~
whenever
10)
E Jet and Ib) E
Jet,
where
Tx
'"
T(X).
If
we write
Tx
=
Li
a;X)Ti, then iu terms
ofTi,
the equation
above
can
be written as
dUng dUng
L a;X) (blr,
10)
sa L a;X\(ba) = 0
i=1
;=1
VXE
~,
(27.60)
where
,?a)
sa (blTi
10)
are complex nmnbers. Equation (27.60) states that
27.5.2. Box.
If
T, as a representation
of
~
(a Lie subalgebra
of
g), is re-
ducible, then there exist a number
of
equations that a;X) must satisfy when-
ever X. E
~.
If
T, as a representation
of
g, is irreducible, then no relation
such as given in (27.60) will exist when X runs over all
of
g.
This laststatement will
be used to analyze certain subgroups
of
GL(V).
Let
us first identify
GL(V)
with
GL(n,
iC).Next, consider
GL(n,
R),
which
is a subgroup
of
GL(n, iC),and transferthe discussionto theirrespective algebras.
If
{Xi} is a basis
of
g[(n, iC),then an arbitrary elementcan be written as
Li
aiXi.
The difference between g[(n,
iC)
and gl(n, R) is that the
ai's
are real iu the latter
case; i.e., for
all real values
of
{ai}, the sum belongs to g[(n,
R).
Now suppose
that
T is an irreducible representation
of
g[(n,
iC)
thatis reducible when restricted
to
gI(n,
R).
Equation (27.60) states thatthe function
n
2
f(Zt,
...
,
zn2)
sa
LZi,,lba)
;=1
vanishes for all real values
of
the Zi'S. Siuce this function is obviously entire, it
must vanish for all
complex values
of
Zi'Sby analytic continuation (see Theorem
11.3.1).
But
this is impossible because T is irreducible for g[(n, iC).We have to
conclude that
T is irreducible as a representation
of
g[(n,
R).
The
next subalgebra
of
gI(n,
iC)
we consider is the Lie algebra
.[(n,
iC)
of
the
specialliuear
group. The only restriction on the elements
of
.[(n,
C) is for them
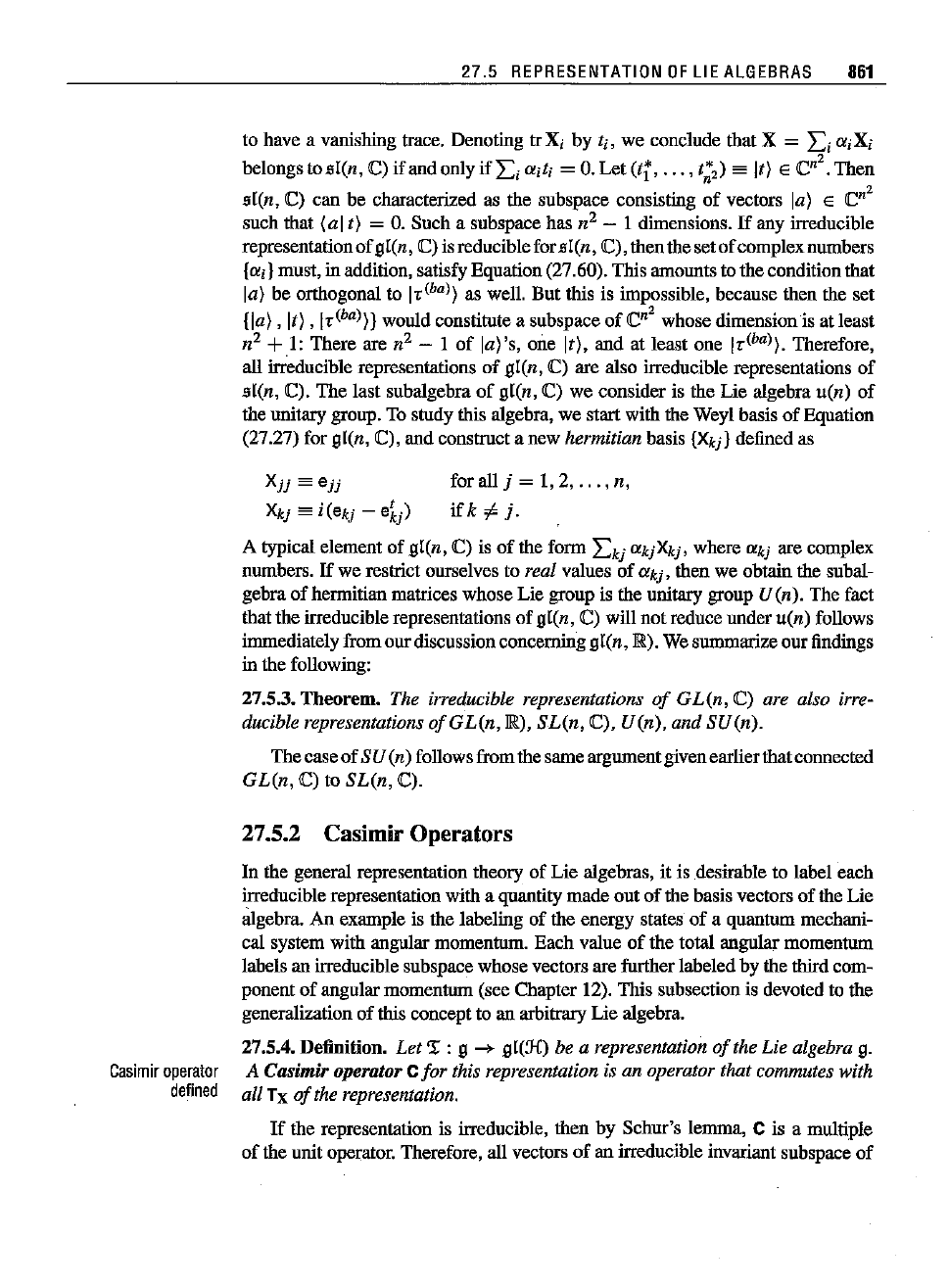
27.5
REPRESENTATION
OF
LIE
ALGEBRAS
861
to have a vanishing trace. Denoting
tr
X, by ti, we conclude that X =
Li
O!iXi
belongs
toal(n,
C) if and only
ifLi
O!iti
=
O.
Let
(tt,
...
,
t:,)
es It} E
en'.
Then
a[(n, C) can be characterized as the subspace consisting
of
vectors la} E
en'
such that (al t) =
O.
Such a subspace has n
2
-
I dimensions.
If
any irreducible
representationofgl(n,
C)
isreducible
foral(n,
C),
thenthe set
of
complexnumbers
100i}
must, in addition, satisfyEquation (27.60). This amounts to the conditionthat
la} be orthogonal to Ir(ba
l}
as well.
But
this is impossible, because then the set
lla}
,
It}
, Ir(bal}} would constitute a subspace
of
en'
whose dimension is at least
n
2
+
I:
There are n
2
-
I
of
la),s, one It}, and at least one Ir(ba
l}.
Therefore,
all irreducible representations
of
glen, C)
are
also irreducible representations
of
a[(n,
iC).
The
last subalgebra
of
glen, C) we consider is the Lie algebra u(n)
of
the unitary group. To study this algebra, we star! with the Weyl basis
of
Equation
(27.27) for
glen,
C),
and construct a new hermitian basis IXkj} defined as
Xjj ==ejj
forallj
= 1,2,
...
,n,
Xkj
==
i(ekj -
ei)
if
k
01
j.
A typical element
of
glen, C) is
of
the form
Lkj
O!kjXkj,
where
O!kj
are complex
numbers.
If
we restrict ourselves to real values
of
O!kj,
then we obtain the subal-
gebra
of
hermitian matrices whose Lie group is the unitary group U(n). The fact
that the irreducible representations
of
glen,
iC)
will not reduce underu(n) follows
immediatelyfrom our discussionconcerning
glen, R). We summarize our findings
in the following:
27.5.3.
Theorem.
The irreducible representations
of
GL(n,
C) are also irre-
ducible representations
ofGL(n,
R),
SL(n,
ci
U(n), and
SU(n).
The
case
of
SU
(n) follows from the same argumentgiven earlierthatconnected
GL(n,
iC)
to
SL(n,
iC).
27.5.2 CasimirOperators
Inthe general representation theory
of
Lie algebras, it is desirable to label each
irreducible representation with a quantity made
out
of
the basis vectors
of
the Lie
algebra,
An example is the labeling
of
the energy states
of
a quantum mechani-
cal system with angular momentum.
Each
value
of
the total angular momentum
labels an irreducible subspace whose vectors
are further labeled by the third com-
ponent
of
angular momentum (see Chapter 12). This subsection is devoted to the
generalization
of
this concept to an arbitrary Lie algebra.
27.5.4. Definition.
Let
X:
g
-+
g[(1f) be a representation
of
the Lie algebra g.
Casimir
operator
A Casimir operator C for this representation is an operator that commutes with
defined
all TX
of
the representation.
If
the representation is irreducible, then by Schur's lemma, C is a multiple
of
the unit operator. Therefore, all vectors
of
an irreducible invariant subspace
of

862
27. LIE
GROUPS
AND
LIE
ALGEBRAS
the carrier space
J{
are eigenvectors of C corresponding to the same eigenvalue.
That Casimir operators actually determine the irreducible representations of a
semisimple Lie algebra is the content of the following theorem (for a proof, see
Chevalley's
theorem
[Vara84, pp. 333-337]).
27.5.5. Theorem. (Chevalley)For every semisimpleLie algebralJ
ofrank
12
r with
a basis
{Xi}, there exists a
set
of
r Casimir operators in the form
of
polynomials
in
TXi whose eigenvalues characterize the irreducible representations
of
g.
From
now on, we shalluse the
notation
Xi forT
Xi'
Itfollows
from
Theorem
27.5.5 that all irreducible invariant vector subspaces of the carrier space can be
labeled by the eigenvalues of the
r Casimir operators. This means that each in-
variant irreducible subspace has a basis all
of
whose vectors carry a set of r labels
corresponding to the eigenvalues of the
r Casimir operators.
One Casimir
operator-in
the form of a polynomial of degree
two-which
works only for semisimple Lie algebras, is obtained easily:
C =
LgijXiXj,
(27.61)
i,j
where
gij
is the inverse of the Cartan metric tensor. In fact, with the summation
convention in place, we have
[C, Xkl = gij[XiXj, Xkl = gij {Xi[Xj, Xk] +[Xi, Xk]Xj}
= gij Icjk
XiXr
+erkXrXj}
= gij crk(XjX
r
+XrXj) (because
gij
is symmetric)
=
gij
g"
Oiks (XjX, +X,Xj)
=
O.
(because gij gsrCiks is antisymmetric in
j,
r)
The last equality follows from the fact that
gij
and
g"
are symmetric, Ciks is
completely antisymmetric [see the discussion following Equation
(27.45)], and
there is a som over the dummy index
s.
27.5.6. Example. Therotation groupSO(3)
in]R3
is a
compact
3-parameter Lie
group.
The infinitesimal
generators
arethe
three
components of the
angular
momentum
operator
(see
Example
27.1.29).
From
the
commutation
relations
oftheangularmomentum
operators
developedin
Chapter
12, we conclude that
c~
=
iEijk.
It follows thatthe
Caftan
metric
tensoris
gij
=
ciscjr
= (iEisr
)(iE
jrs)
=
+EisrE
jsr
=2liij.
Ignoring
thefactorof 2 anddenotingthe
angular
momentum
operators
by Lj,we conclude
that
L
2
==
LI
+L~ +L~
12Recall
that
the
rank
offI isthe
dimension
of the
Cartan
subalgebra
offl.
