Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

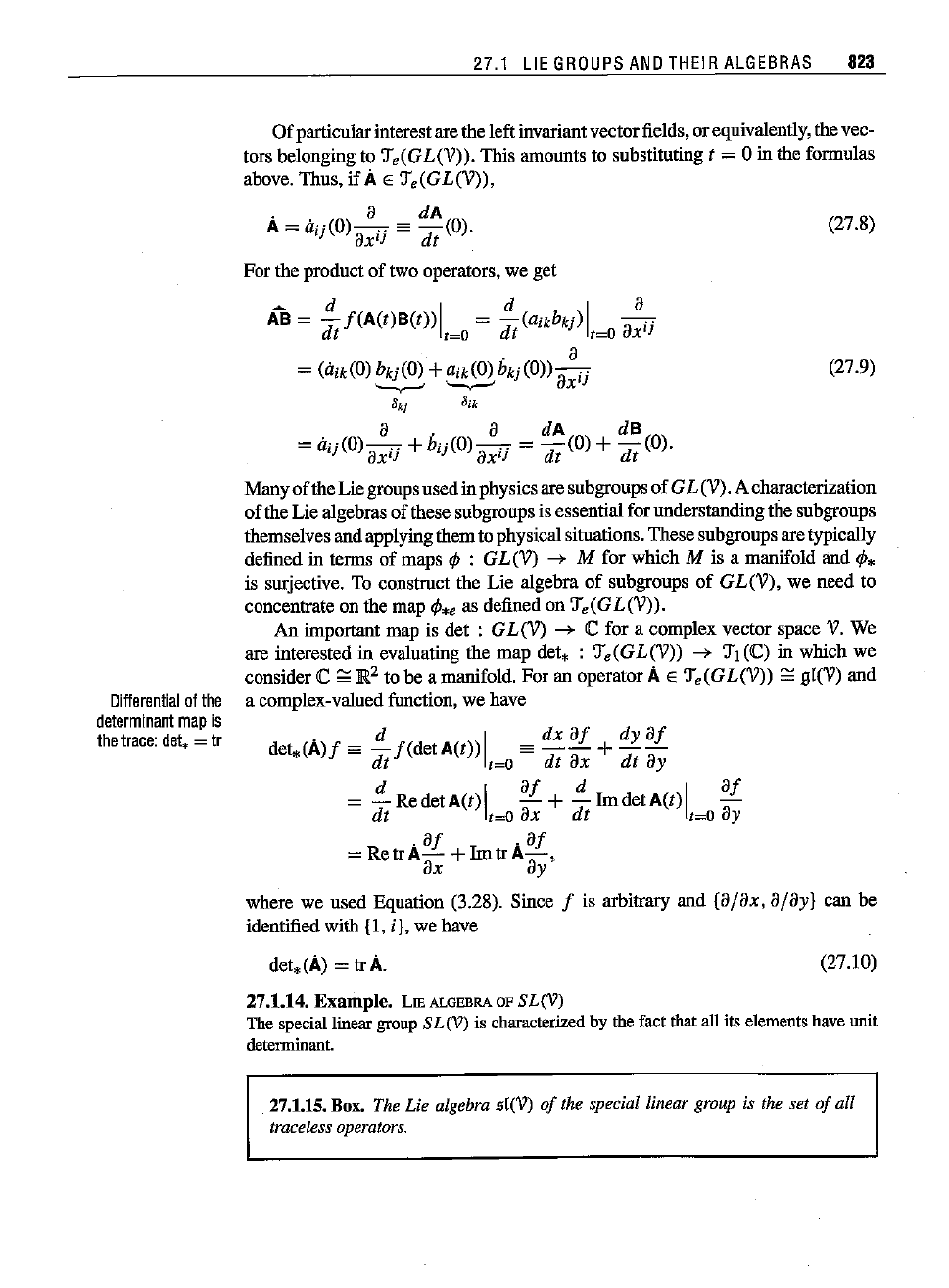
(27.9)
Differential
of
the
determinant
map
is
the
trace:
det,= tr
27.1 LIE
GROUPS
ANO
THEIR
ALGEBRAS
823
Of particularinterestare the left iovariant vectorfields, or equivalently,the vec-
tors belonging to
T,(GL(V)).
This amounts to substituting t =
010
the formulas
above. Thus, if
A E T,(GL(V)),
. a dA
A =
tiij(O)-
.. es
-(0).
(27.8)
ax'] dt
For the product of two operators, we get
Ail
=
!!-
f(A(t)B(t))[
= !!-(aikbkj)[
~
dt
,~O
dt 1=0 ax']
. a
=
(tiik(O)bkj(O)+aik(O)bkj(O))-a
.,
'--v--'
'-.,.-..'
x'!
t3kj ()ik
a . a dA dB
=
tiij(O)-
.. +
bij(O)-
.. =
-d
(0) +
-d
(0).
axl]
ax']
t t
Many
of
the Lie groups used 10physicsare subgroups of GL(V).Acharacterization
of the Lie algebras of these subgroups is essentialfor understandingthe subgroups
themselves and applyiogthem to physicalsituations. Thesesubgroups are typically
defined 10terms of maps
t/J
: GL (V) --> M for which M is a manifold and
t/J.
is surjective. To construct the Lie algebra
of
subgroups
of
G
L(V),
we need to
concentrate on the map
t/J"
as defined on T,(GL(V)).
An important map is det :
GL(V)
-->
iC
for a complex vector space V.We
are interested
10evaluating the map
det,
:
T,(GL(V))
--> Tj(iC) 10which we
consider
iC
~]R2
to be a manifold. For an operator A E
T,(GL(V))
~
gl(V) and
a complex-valued function, we have
. d
[dX
af
dy
af
det.(A)f
sa -
f(detA(t))
es
--
+--
dt
,~O
dt ax dt ay
d
I
af
d
[a
f
= -
RedetA(t)
- + -
ImdetA(t)
-
dt
1=0
ax dt 1=0 ay
.
af
.
af
=
RetrA-
+ImtrA-,
ax ay
where we used Equation (3.28). Sioce f is arbitrary and
{ajax,
ajay}
can be
identified with
(I,
i), we have
det,
(A) = tr A.
(27.10)
27.1.14.
Example.
LIEALGEBRA OF
SL(V)
The special linear group
SL(V)
is characterized by the fact that all its elements have unit
determinant.
27.1.15. Box. The Lie
algebra
sl(V) of the
special
linear
group
is the set of all
traceless operators.

824 27. LIE GROUPS AND LIE ALGEBRAS
Thisisbecauseif weuse(27.10) and (26.12) andthefact that
SL(V)
=
det-
l
(l)
, we
canconcludethatdet.(A)
= tr A= 0for all AE sl(V). III
27.1.16.
Example.
LIE
ALGEBRAS OF UNITARY AND RELATED GROUPS
Letus
first
show
that
thesetof
unitary
operators
on V,denotedby U(V). is aLie
subgroup
unilary
group
of
GL(V),
calledthe unitary group ofV. Considerthe map
1/!
:
GL(V)
--+
1HI,
where
IHI
is theset ofHermitian
operators
considered as avectorspace
(therefore,
amanifold) over
the
reals,
defined
by
1/!(A)
= AAt.UsingEquation(27.9), thereadermayverifythat1/!.is
surjective
and
(27.11)
It
follows
from
Theorem
26.3.7 that
U(V)
_ 1/!-1(1) is a subgroupof
GL(V).
Using
Equation
(26.12), we
conclude
that1/!.(A) = A+ At = 0for allAE u(V), i.e.,
27.1.17.Box. The Lie
algebra
u(V) of the unitary
group
is the set of all anti-
hermitian operators.
special
unitary
group
orthogonal
and
special
orthogonal
groups
exponenlial
map
ofa
Lie
algebra
By counting the
number
of
independent
real
parameters
of a
matrix
representing
a
hermitian
operator.
wecanconcludethatdim
JH[
=n
2
. It followsfrom
Theorem
26.3.7
that
dimU(V)
=n
2.
Theintersection of
SL(V)
and
U(V),
denotedby
SU(V),
is calledthespecial unitary
group. ItsLie
algebra
su(V) consistsof anti-hermitian traceless
operators.
The
reader
may
checkthatdim
SU(V)
=n
2
-
I.
Whenthevectorspaceis C", we write
U(n)
and
SU(n)
insteadof
U(C
n)
and
SU(C
n).
If
we
restrict
ourselvesto realvectorspaces,then
unitary
and.special
unitary
groups
becometheorthogonal group
O(V)
andspecialorthogonal group
SO(V),
respectively.
Their
algebras
consistof
antisymmetric
and
traceless
antisymmetric
operators,
respectively.
WhenV =
JRn,
weuse theootation
O(n)
andSO(n). III
Let X be a vectorfield OnG. We know from our discussion
of
flows that X has
aflow
F, sa exp(tX) at every pointg
ofG
with
-E
< t < E. Now, since
F,(g)
i'
g
is in G, it follows from the group property
of
G that (F,)n (g) = F
n,
(g) E G for
all n. This shows that the flow
of
every vector field on a Lie group is defined for
all t E
JR,
i.e., all vector fields Ona Lie group are complete. Now consider 9 as
a vector space
and manifold and define a map exp : 9
~
G that is simply the
flow evaluated at
t = 1.
It
can be shown that the following result holds ([Warn 83,
pp. 103-104]):
27.1.18.
Theorem.
exp : 9
~
G, called the exponential map, is a diffeomor-
phism
of
a neighborhood
of
the origin
of
9 with a neighborhood
of
the identity
element
of
G.
This theorem states that in aneighborhood
of
the identityelement,a Lie group,
as a manifold, "looks like" its tangent space there.
In particular,
inner
automorphism
ofa
Lie
group
adjoint
map
ofa
Lie
algebra
27.1 LIE
GROUPS
AND
THEIR
ALGEBRAS
825
27.1.19. Box. Two Lie groups that have identical Lie algebras are locally
diffeomorphic.
27.1.20.
Example.
WHYexpIS CALLEDTHE
EXPONENTIAL
MAP
LetV be a finite-dimensional vectorspace and A E
£1[('\7).
Define, as in Chapter 2,
and note that
Furthennore,
00
k k
00
nAn
00 00
k n
etAe
sA
=
L~
E-
S
- =
LL~Ak+n
k=O k! n=O n! k=On=O k!n!
DOGm
m-nn)
= E L t S Am = eCt+s)A.
m=Q =0 (m -
n)!n!
=(t+s)mjm!
It follows that
etA
has all the properties expected of the flowof the vector fieldA.
IlIII
The exponentialmap has someimportantpropertiesthat we shall have occasion
to use later. The first of theseproperties is the content of the following proposition,
whose proofis left as an exercise for the reader.
27.1.21. Proposition.
Let
t/J
: H
--+
G be a
Lie
group homomorphism.
Then,for
all
'1/
E
~,we
have t/J(exPH
'1/)
=
eXPG(t/J.'1/)·
For every g E G, let I
g
es R
g
1
0
L
g
•
The reader may readily verify that
I
g,
which takes x E G to
sxs:"
E G, is an isomorphism
of
G, i.e., Ig(xy) =
Ig(x) Ig(y) and Ig is bijective.
It
is called the
inner
automorphism
associated
with
g.
27.1.22. Definition. The Lie algebra isomorphism I
g•
= R
g
! 0 L
g•
: 9
--+
9 is
denoted by
Ad
g
and is called the adjoint
map
associated with g.
Using Proposition 27.1.21, we have the following corollary.
27.1.23. Corollary.
exp(Adge) =
Igexpe
=
gexpeg-1!orall
eE
gandg
E
G.

826
27. LIE
GROUPS
AND
LIE
ALGEBRAS
Let
{ed
be a basis for the (finite-dimensional) Lie algebra of the Lie group G.
The Lie bracket of two basis vectors, being itself a left-invariant vector field, can
be written as a linear combination of
(ed:
"
lei'
ej]
=
:~:>tek'
k~!
On a general manifold,
c~.
will depend on the point at which the fields are be-
ing evaluated. However,
o~
Lie groups, they are independent
of
the point, as the
following manipulation shows:
n n n
= L
g•
I:>~/e)ek(e)
=
LC~j(e)Lg.ek(e)
=
Lc~/e)ek(g).
k~l
k=! k=!
Therefore, the value of
C~j
at any pointg
EGis
the sanneasits value at the identity,
i.e.,
ct
is a constant. This statement is called
Lie's
second
theorem.
27.1.24. Definition. Let
(ed?~!
be a basisfor the Lie algebra g
of
the Lie group
G. Then
Lie's
second
theorem
structure
constants
ofe
Lie
algebra
n
[ei(g),
ej(g)]
=
LC~jek(g),
k=!
(27.12)
where 0
j
, which are independent
of
g, are called the structure constants
of
G.
The structure constants satisfy certain relations that are immediate conse-
quences
of
the commutation relations. The antisymmetry
of
the Lie bracket and
Lie's
third
theorem
the Jacobi identity lead directly to
(27.13)
The fact that
{~p}
obey Equation (27.l3)is the content of
Lie's
third
theorem.
27.1.3 InfinitesimalAction
The action
ep
: G x M
--+
M of a Lie group on a manifold M induces a homo-
morphism of its algebra with X(M).
If
e E g, then exp(te) E G can act on M at
a point
P to produce a curve
y(t)
=
exp(te)
. P going through P. The tangentto
this curve at
P is defined to be the image
of
this homomorphism.
infinitesimal
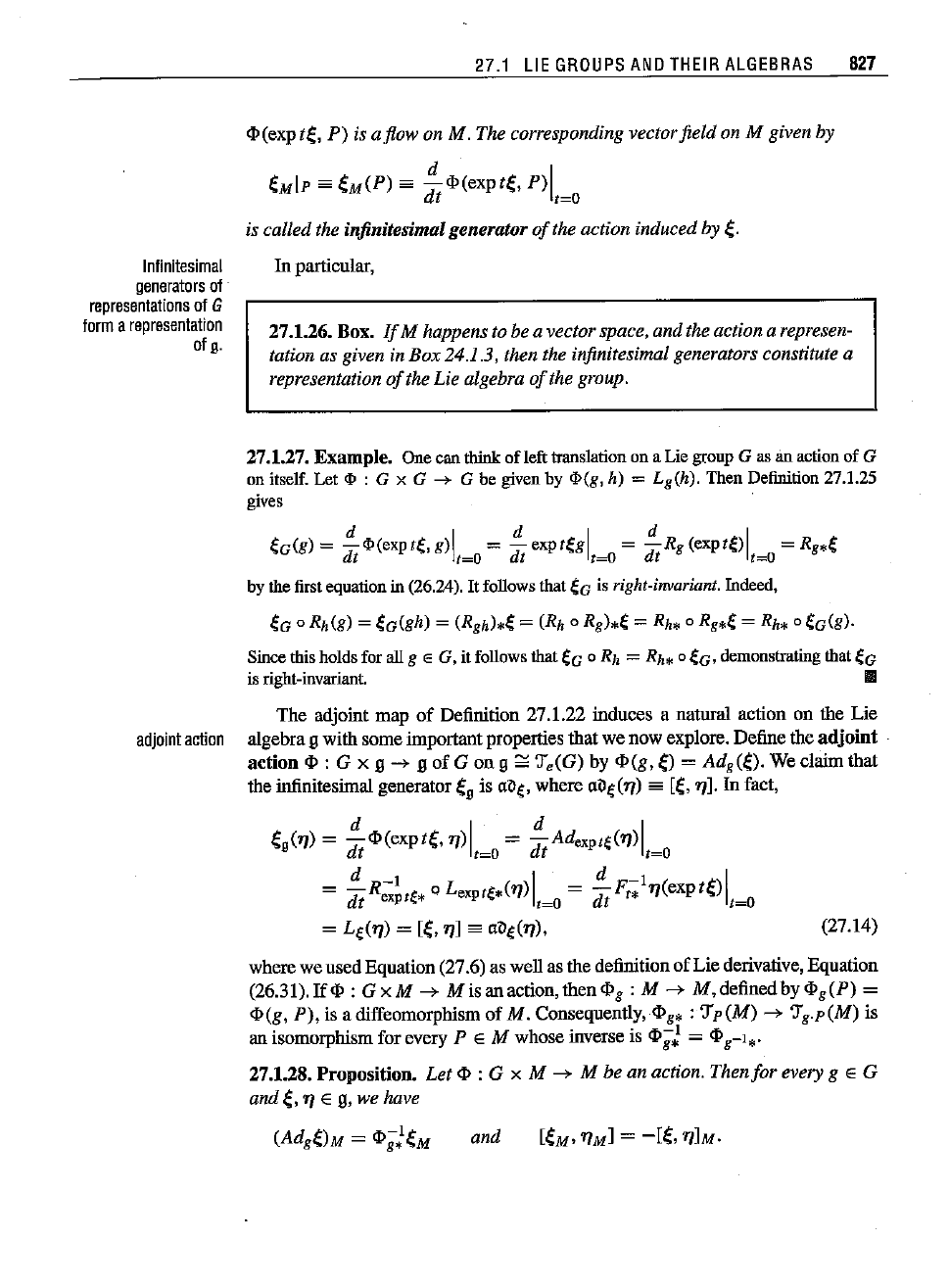
27.1.25. Definition. Let
ep
: G x M
--+
M be an action.
If
e E g, then
generators
ofan
action
Infinitesimal
generators
of
representations
ofG
form
a
representation
ofg.
27.1 LIE
GROUPS
AND
THEIR
ALGEBRAS
827
<I>(exptl;,
P) is ajlow on M. The corresponding vectorfield on M given by
I;M[P
sa I;M(P) sa
~<I>(exptl;,
P)[
dt
t=O
is called the infinitesimalgenerator
of
the action induced by
1;.
In
particular,
27.1.26. Box.
If
M happens to beavector space, and the action a represen-
tation as given in Box 24.1.3, then the infinitesimal generators constitute a
representation
of
the Lie algebra
of
the group.
27.1.27. Example. Onecanthinkofleft
transtation
onaLie
gronp
G asan
action
ofG
on
itself.
Let
<I>
: G x G -+ G be
given
by
<I>(g,
h) = Lg(h). Then
Definition
27.1.25
gives
eG(g) =
dd
<I>(expte,
g)1
=!!.-
exptegl
=
dd
R
g
(expte)1
=
Rg.e
t t=O
dt
t=O t t=O
bythe
first
equation
in(26.24).
It
follows
thateG is
right-invariant.
Indeed,
eG 0 Rh(g) = eG(gh) = (RghJ.e =(Rh 0
Rg).e
=
Rh.
0
Rg.1f.
=
Rh.
0 If.G(g).
Since
this
hotds
forall g E G, it
follows
thateG 0 Rh =
Rh.
0 If.G,
demonstrating
that
If.G
is
right-invariant.
11III
The adjoint map of Definition 27.1.22 induces a natural action on the Lie
adjoint
action
algebrag with someimportantpropertiesthat wenow explore. Definethe adjoint
action
<I>
: G x g -+ g of G on g ~
~)"e(G)
by
<I>(g,
1;)
= Adg(I;). We claim that
the infinitesimalgenerator
1;9
is
nDe,
where
nDe(1/)
==
[1;,1/].
In
fact,
I;g(1/)
=
dd
<I>(exptl;,
1/)[ =
dd
Adexpte(1/)[
t t=O t 1=0
d
-t
I d
-1
I
=
dt
Rexpte. 0 L
expte.(1/)
t~O
= dt Ft. 1/(exptl;)
t~O
= Le(1/) =
[1;,1/]
sa
nDe(1/),
(27.14)
whereweusedEquation (27.6) aswell as the definitionofLie derivative,Equation
(26.31).
If
<I>
:
GxM
-+
Mis
anaction,then
<l>g
: M
-+
M,definedby<l>g(P) =
<I>(g,
P), is a diffeomorphismof M. Consequently,
<l>g.
: 'J'p(M) -+ 'J'g.p(M) is
anisomorphism forevery P E M whose inverseis
ct>g;
=
ep
g-l*.
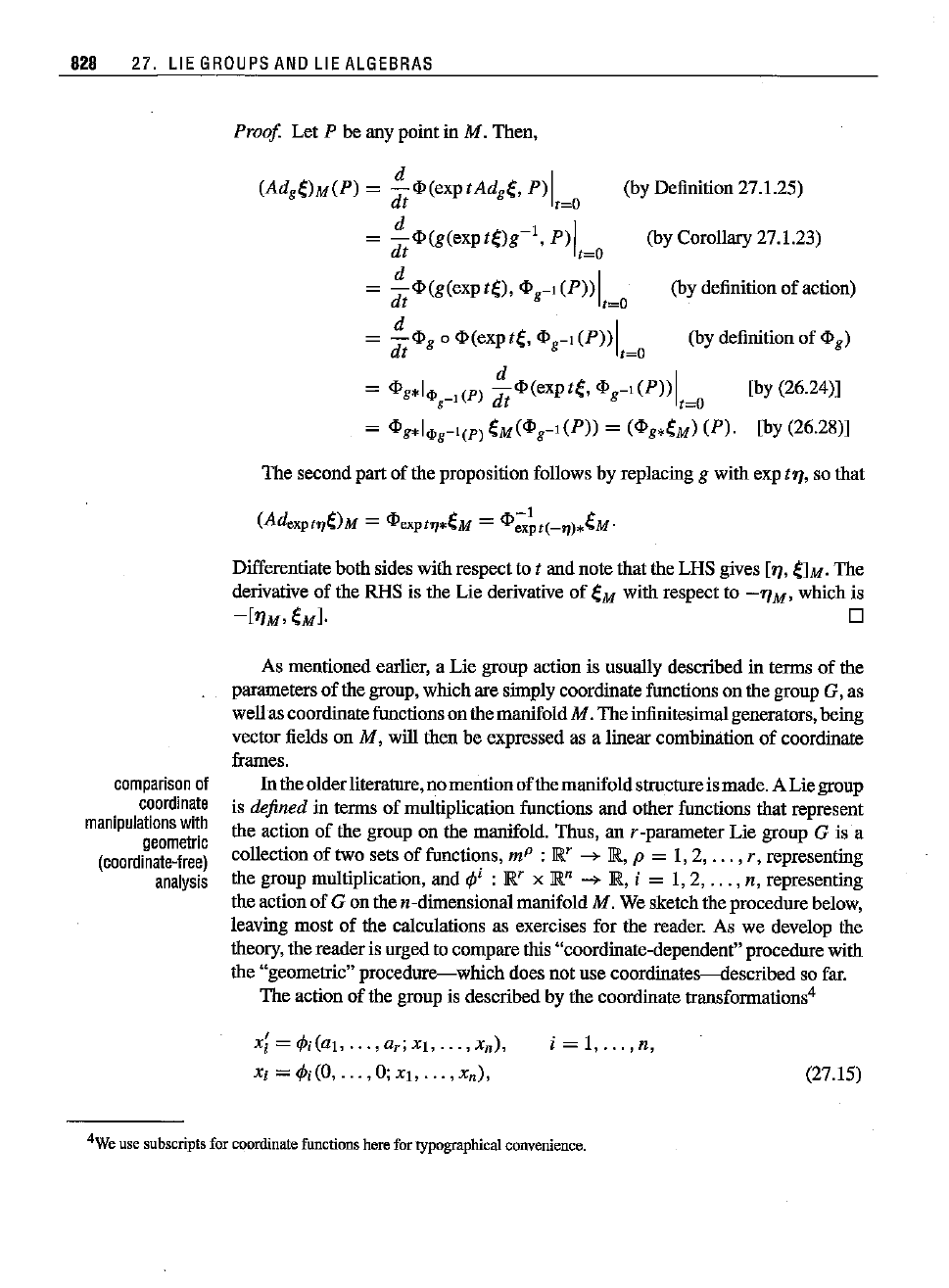
27.1.28. Proposition. Let
<I>
: G x M
-+
M be an action. Thenfor every g E G
and
1;,1/
E g, we have
and
828
27. LIE
GROUPS
ANO
LIE
ALGEBRAS
Proof
Let P be any point in M. Then.
(by Corollary 27.1.23)
(by Definition 27.1.25)
(by definition
of
action)
[by (26.24)]
[by (26.28)]
(Adge)M(p)
=
~lP(exPtAdge.
p)1
dt
t=O
=
~lP(g(expte)g-l.
p)1
dt
t~O
=
~lP(g(expte).
lPrl(pnl
dt
t=O
=
~lPg
0
lP(expte.
lPrl
(pnl
(by definition of lP
g)
dt
t~O
=
lPg*I<I>
(P)
dd
lP(expte.
lPrl(pnl
g-l
t
(=0
= lPg*I<I>r1(p)eM
(lPrl
(P))
= (lPg.eM)
(P).
The second part of the proposition follows by replacing g with exp
t'TJ.
so that
Differentiate both sides with respect to t and note that the LHS gives
['TJ.
elM.
The
derivative of the RHS is the Lie derivative
of
eM with respect to -'TJM. which is
-~M.e~.
D
comparison
of
coordinate
manipulations
with
geometric
(coordinate-free)
analysis
As mentioned earlier. a Lie group action is usually described in terms
of
the
parameters
of
the group. which are simply coordinate functions on the group G. as
wellascoordinatefunctions on the manifold
M.
The infinitesimalgenerators. being
vector fields on
M.
will then be expressed as a linear combination
of
coordinate
frames.
In
the olderliterature. no mentionofthemanifoldstructureismade. ALie group
is
defined in terms of multiplication functions and other functions that represent
the action
of
the group on the manifold. Thus. an r-parameter Lie group G is a
collection of twosetsof
functions,
m!'
:
IR
r
---+
JR,
p = 1,2,
...
,
r,
representing
the group multiplication. and
cf>i
:
E'
x En
->
E.
i = I. 2
•...•
n, representing
the action of G on the n-dimensionalmanifold M. We sketch the procedure below.
leaving most of the calculations as exercises for the reader. As we develop the
theory. the readeris urged to compare this "coordinate-dependent"procedure with
the "geometric"
procedure-which
does not use coordinates--<iescribed so far.
The action of the group is described by the coordinate transformations"
x; = tPi(al.
.,.,
ar;
Xl,
, X
n
),
Xi =
tPi(O,
...
,0;
Xl,
, X
n
) ,
i = 1,
...
,n,
(27.15)
4Weuse
subscripts
for
coordinate
functions
herefor
typographical
convenience.

27.1 LIE
GROUPS
ANO
THEIR
ALGEBRAS
829
as well as the group multiplication properties
Cp =
mp(al,
, a
r;
bI, ,b
r
),
P = 1,
...
, r,
a
p
= mp(O, , 0;a1, , a
r)
= rnp(al.
...
, ar; 0,
...
,0),
mp(a;
m(b;
c)) = mp(m(a; b); c).
(27.16)
Equation(27.15) is tobe interpretedas arule that takes the secondset of arguments
and transforms them
via
the first set into the LHS. Now suppose that we translate
from
x; to a neighboring point x; +dX; via a set of group parameters
{~ap}~=l'
Wecanalsoget to
XI~
+
dX;
fromXi via a new set of parameters.l whichhaveto
be slightly different from
{ap}~=l'
say lap +
dap}~=l'
We then have
xI
+dX; =
41i(8al,
...
,8a,;
x~,
...
,
x~),
xI +
dX;
=
4Ji(al
+
dai,
...
,a; + dar;
xt
,
...
, x
n),
a
p
+da
p
=
mp(al,
...
, a
r;
8a1o
...
, 8a,),
and, with summation over repeated indices understood,
dX;
=
(27.17)
(27.18)
Inverting the secondequationand substitutingthe resulting
~a's
in the firstequation
yields
or
where in the last equation, we changed the free coordinate variable on both sides.
It
then follows that
(27.19)
Lie's
first
theorem
Equation (27.19) and establishing that
UiK
is e
oo
is the content
of
Lie's
first
theorem.
The change
of
an arbitraryfunction
f(x)
due to an infinitesimaltransformation
is
SHere
we are
assuming
that
theactionofthe
group
is transitive,i.e.,
that
everypointof the
manifold
canbe
connected
toany
other
pointviaa
transformation.

830 27. LIE
GROUPS
AND LIE
ALGEBRAS
This suggests calling
n a
X
K
= L
UiK
(x)
ij:"
i=l
X,
(27.20)
(27.21)
infinitesimal
generators
as
vector
fields
onM
the infinitesimal generators
of
the Lie group.
The
commutator
of
two
of
these
generators
is
[
aU
[o
au
jp]
a
[Xp,X
n
] = UiP-a--Uin-
a-
-a
.
Xi Xi Xi
This commutator does not appear to be similar to the one in Definition 27.1.24,
which is necessary
if
the generators are to form a Lie algebra. However, through
a long aod tortuous manipulation, outlined in Problem 27.9,
one
cao show that
(27.22)
where
c~u
areconstants.
One
cao also obtain this sarne result by the
much
simpler method
of
applying
Proposition 27.1.28 to both sides
of
Equation (27.12):
[(OM,
(ej)Ml
=
-[e,
'7]M
= - (tcfjek) = -
tct(ek)M.
k=1
M
k=l
This equation is equivalent to (27.22) if we identify the X
p
's withthe
(ei)
M'S
aod
ignore
the
irrelevant
minus
sign.
The
reader has hopefully
been
able to appreciate the power aod elegaoce
of
the geometric approach to Lie groups aod Lie algebras.
The
above illustration
(Problem 27.9) brings out the tedium aod the error-prone procedure
of
obtaining
group-theoretic results throughcoordinate maoipulations, a procedure used
in the
old literatureincludingthe work
of
SophusLie himself. Althoughsuch calculations
areinevitable in
practice,
wheremostLie
groups
aregiveninterms of
parameters,
they are not suitable for obtaining formal results.
27.1.29.
Example.
The two-dimensional rotation group
80(2)
is a I-parameter Lie
group definedby
xi
==
cPl(Xl,XZ; B)
=Xl
cos s
-X2sinB,
x2
ee
¢2(XI,
xz:
0) = XI sinO +
Xz
cosO.
Using Equation (27.20).we findthe (only) generator of this group:
X =
Uj
a~i
where Ui =
aa~
IlbO·
Explicitly,
wehave
"I
=
at/>ll
=
(-Xl
sinO -
Xz
cosO)lo=o = -xz,
ao
0=0
"Z =
at/>zl
= (XI cosO -
Xz
sinO)lo-o =
XI.
ao
0=0
-

27.1 LIE
GROUPS
AND
THEIR
ALGEBRAS
831
and
a a a a a a
X=UI-+U2-
=-X2-+xI-
=-y-+x-.
aXI
aX2
aXI
aX2
ax ay
Thereaderrecognizes
this,
within
afactorofi, asthe
z-component
ofthe
angular
momentum
operator.
In
general,
27.1.30.Box. Angular
momentum
operatorsare the infinitesimal generatorsofro-
tation.
Inclusion
of the
other
two
rotations
about
thez-axis andthey-axis
completes
theset
of
infinitesimal
generators
of the
rotation
group
in
three
dimensions.
II
The action of a Lie group on M can be reconstructed from its infinitesimal
action. The flow of
X
K
is the solution of the DE
dx; ,
dt
= UiK(X),
X;(O)
=Xi.
(27.23)
Once the solution is obtained, one can replace
t with
UK
for each K. In some
applications,
uiK(x) will be given implicitly in terms
of
certain parameters
of
integration of some DEs [unrelated to (27.23)]. The solution of these DEs are
typically generators of coordinate transformations that can
be wtitten linearly in
terms of the parameters. To be more precise, suppose that after solving some DEs,
we obtain
r
Xi =
I>iKfji)(Xl'
...
,X
n),
1C=1
(27.24)
where
{eiK]are the parameters of integration, and Xi are components
of
the vec-
tor field that generates the coordinate transformation. This means that for small
parameters,
onecanwrite
r
xI = Xi +
LCiKf;i)(Xl,'."
x
n)
K=l
and read off
UiK
(x) =
fji)
(x). In that case, we have
X[(O)
=Xi.
(27.25)
We shall have occasion to use tltis formula later.

832 27. LIE
GROUPS
ANO
LIE
ALGEBRAS
27.1.4 Integrationon Lie Groups
As any other manifold, one can define integration on Lie groups; i.e., one can
construct nonvanishing n-forms and use Equation (26.46) to define integrals on a
Lie group G. Because
of
the left-invariant property
of
objects on G, it would be
helpfnl if the integration process were also left-invariant.
For
this to happen, the
n-form would have to be left-invariant.
It
turns out that this can be accomplished
more or less urtiquely:
27.1.31.
Proposition.
Let
G be a Lie group
of
dimension n. Then there exists a
left-invariantnonvanishing n-form
1L
thatis uniqueup to a nonzeromultiplicative
constant.
If
G is compact, then
110
is also right-invariant
and
the multiplicative
constant can be chosen to be
I.
Proof
Let
lJoe
be any nonzero n-form on 'Je(G).
The
desired n-form is the left
translation
of
this form, i.e., L;-llJoe' Indeed, let {Xili'=, be left invariant. Then
IJo
g
(X, l
g
, ·
..
, Xnl
g)
= L;_llJoe(X,l
g,
, Xnl
g)
= lJoe(Lg-l*X,l
g
, ,
Lg-l*X
n
Ig)
= lJoe(Xilg-1g,
...
, Xnlg-l
g)
= lJoe(X,le,
...
, Xnl
e).
This shows that
110
is left-invariant. Now note that any other n-form
1Jo~
on 'Je(G)
is a constant multiple
of
lJoe'
Therefore, the corresponding n-form
1Jo~
will be a
constant multiple
of
IJo
g
•
Let x E G and consider
110'
'"
RilJo.
We have
L
* ' L* R* R* L* R*
I
gIL = g 0 xl! = x 0
glt
=
xJL
=
JL
•
where we used the fact that L
g
and R
x
commute and that
110
is left invariant. The
equation above shows that lJo'is also left-invariant. Therefore,
110'
=
CIJo.
If
G is
compact, we can integrate both sides and note that
f
G
110
= f
G
110'
because
110'
is
related to
110
by a change
of
variable. Therefore, c = I and
Ri
110
=
110.
D
The
left-invariant volume element (nonvanishing n-form) guaranteed by the
Haar
measure
proposition above is called Haar
measure.
Since all calculations are done using
some coordinate system, we give an explicit expression
of
the Haar measure in
terms
of
coordinates (parameters)
of
a general Lie group. Let y =
(y',
...
, yr)
be the coordinates
of
the translation
of
x = (x
',
...
,
x")
by g E G. Then we can
write y
=meg, x), so that
dy!
=
(ayi
jaxi)dx
i
=
(ami
jaxi)dx'-
Therefore,
d
'
r
(ami(g,X»)
i r
y
A···
A dy = det .
dx
A···
A
dx
.
ax'
In particnlar,
if
x = 0, the coordinates
of
the identity, theny will be the coordinates
of
g. So, the volume element at g, denoted by
dry,
will be given by
d
r
y = det
(ami
(~'
X»)
I d
r
x.
ax
x=o
