Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


28.3
COVARIANT
DERIVATIVE
AND
GEODESICS
903
divergence
ofa 28.3.6.Definition. Given a tensor field T, define its divergence V . T to be the
tensor
field
tensor obtainedfrom VTby contracting the last upper index with the covariant
derivative index. In components,
The
covariant derivative replaces the ordinary derivative
when
functions are
generalized to tensor fields, and in many respects it is very similar to ordinary
derivatives. The aspect
of
the covariantderivative thatis in contrastto the ordinary
derivative, namely, its lack
of
commutativity,is relatedto an innportant geometrical
object that we encountered before: curvature.
Let
us find this relation. Start with
an
ordinary
l-form w =
(Vie;,
and note
that
(dw, u A v) = (dwi A e
i
+Widei, u A v)
= (dwi A e
i,
u A v) +(Widei, U A v) ,
(28.49)
where u
and
v are arbitrary vectors. For the first term
of
Equation (28.49), we
obtain
(dwi A
e,
U A v) =ido», u) vi - ido», v) u
i
=ViU(Wi) - UiV(Wi)'
Now we use
U«(v,
w))
= U(ViWi) = WiU(Vi) +ViU(Wi),
v«(u,w» =V(uiWi) =WiV(Ui) +UiV(Wi),
to get
idto; A e
i,
U A v) =
uf
(v, w)
-V«U,
w» +Wi[V(U
i)
-
u(v
i)].
(28.50)
With
dei =
_w
i
j
A e
j
=
-rijke
k
A e
j,
the second term
of
(28.49) becomes
(widei,
U A v) =
-Wirijk
(e
k
A e
j,
U A v) =
-Wirijk
(ukv
j
- u
j
vk)
=
-Wiukvj
(r
i
jk
- r
i
k
j)
=
_ukv
j
(w, [ek,
ej]).
~
(£i,[ek,ejD
We also note that
[U, v] =[ukek,
viej]
=
ukek(vjej)
-
vjej(ukek)
=
[u(vj)]ej
+
ukvjekej
- [v(uk)]ek -
ukVjejek
= [u(v
j)
-
v(uj)]ej
+
ukvj[ek,
ej].

904 28. DIFFERENTIAL
GEOMETRY
It
follows that
aod the second term of (28.49) becomes
(Widei, u A v) = - (w, [u, v]) +Wj[u(v
j)
- v(u
j)].
Combining Equatious (28.49), (28.50), aod (28.52), we obtain
(dw, u A v) =
u«v,
w)) -
v«u,
w)) - (w, [u, v]) .
(28.51)
(28.52)
(28.53)
Equation (28.53) is the basis of our generalization involving the covariant
derivative: We replace w with a vector-valued l-formT, aod the derivatives
u(
..
·)
aod
v(···)
with V
u("')
aod V
v("')'
The result will be
(dT, u A v) = Vu«v, T)) - Vv«u, T)) - (T, [u,
v]).
Choose a vector w aod replace T with
dw
to obtain
(d
2w,
U A v) = V
u
«v.
dw))
-V
v
«u,
dw))
- (dw, [u, v])
'-v-'"
"
;;:=Vvw
="Vuw
=V[u,vjW
=
vuvvw
-
vvvuw
-
Vlu,vlW
es R(u, v)w,
(28.54)
(28.55)
where we have iotroduced the linear operator R(u, v) :
X(M)
->
X(M)
in the last
Iine, We rewrite the definition of this operator as
lI(u, v) = VuV
v
- VvV
u
- Vlu,vl'
(28.56)
Equations (28.55) aod (28.56) cao be used to derive the following identity (see
Problem 28.11):
R(u, v)w
+R(w,
u)v
+R(v,
w)u
=
O.
Define the map R : X*(M) x
X(M)
x
X(M)
x
X(M)
->
R by
R(w, w,
u, v) sa (w, R(u, v)w) .
(28.57)
(28.58)
Riemann
curvature
"tensor'ts
indeed
a
tensor
in
the
sense
of
Box
26.4.11
!
The covariaot derivatives in the definition
of
R(u, v) may give the impression that
R will differentiate whatever appears to its right. It is a remarkable property
of
R that this will not happen: That R is a tensor
of
type
(1,3)
[see Box 26.4.11],
the
Riemann
curvature
tensor, follows from Equations (28.7) aod (28.55). The
readermay verify that the components of this tensor are precisely those iotroduced
in Equation (28.22).
In
fact, it is now more appropriate to change Oii to Rij aod
write
(28.59)

28.3 COVARIANT OERIVATIVE
AND
GEODESICS
905
From Equation (28.57) follows the cyclic property of the lower indices
of
the
components of R as given in Equation (28.24).
The components of R can be conveniently evaluated in terms
of
connection
coefficients:
R
i
jkl
ea
R(.i,
ej,
ek,el) = (.1, R(ek, e/)ej)
= (e
i
,
VekVe1ej - Ve/Vekej - V[eb
el1
e
j )
=
<.1,
V'
k
V./ej) -
<.i,
V./V.kej) -
<.i,
V['ko./lej) .
We calculate each pairing separately. The vector in the first paiting is
V'
k
V./ej
= V'
k
(rjle,.)
= [ek
(rjl)]e,.
+
r"jlv.ke,.
=
rjt,kern
+
rjlrnmken.
Similarly, the second vector can be expressed as
(28.60)
VelVekej =
rjk,le
m
+rjkrnmlen.
For the third vector, we use the definition
of
the structure constants and write
Substituting these in Equation (28.60), we obtain
R
i
j k1
=
fi
j 1
.
k
-
fijk,l
+
rjtrimk
-
fjkriml
-
C11
ri
j
m'
If
we use
coordinate
frames,
the
structure
constants
arezero,
and
we get
(28.61)
(28.62)
.
ar
i
jl
ar
i
j
k·
.
R'jkl
=
-k-
-
--1-
+
r1mkfjl
-
r1m1fjk'
ax ax
If
the manifold has a metric (Riemannian or pseudo-Riemannian manifold), then
a combination of (28.26) and (28.62) gives the curvature in terms of the mettic
tensor.
28.3.2 Geodesics
The reader may recall from elementary physics courses that in a flat space, one
is allowed? to move a vector about as long as it is kept parallel to itself.
In any
kind
of
parallel displacement
of
a vector, one moves the (tail
of
the) vector along
some
curve.
This
curve
is in our subconscious in
flat
space,
becauseit playsno
role in such a
displacement-all
curves give the same end result. However, in
curved spaces, different curves "paralleltransport" a vector differently, so that the
"Except in
situations
wherethepositionof thevectoris
important,
asin
torques
and
angular
momenta.

906 28.
DIFFERENTIAL
GEOMETRY
parallel
transport
of
vectors
and
tensors
along
a
vector
end result will be different. Each curve honestly "thinks" that it is indeed parallel
transporting the vector, i.e., that it is not changing the direction of the vector as
the
latter
movesalongthe
former.
No
change
meanszero
derivative;
and
sincethe
concept of derivative is local,
let
us replace the curve with its Iangent u:
28.3.7. Definition.
A vectorvis
said
to be
paralleltransported
along
u
ifV
u
v =
O.
Similarly, a tensor T is
said
to be parallel transported along u
ifV
u
T =
O.
If
the manifold M has a metric g,then it is desirablefor the parallel transporta-
tion not to affect the metric, i.e., that the angle between any two vectors remain
the same aftertransportation. Therefore, we demand that
9 be constant for parallel
transportation along any vector,
i.e.,
Vug =0 \I u
=}
Vg =0, or
g;j;k
= 0 \I i,
j,
k.
A
statement equivalent to this equation is that
(28.63)
28.3.8. Box. The operation
of
raising
and
lowering
of
indices commutes
with the operation
of
covariantdifferentiation.
Considertwo vectors v and w.
If
9 satisfies Equation (28.63), then
Vu(v'
w) = Vu[g(v, w)] ea V
u
(g, v
<81
w)
= (Vug, v
<81
w) +(g, (Vuv)
<81
w) +(g, v
<81
(Vuw))
= g(Vuv, w) +g(v, Vuw),
or
Vu(v·
w) = (Vuv) . w +v (Vuw). (28.64)
In
particular,
if
v and w are parallel transported along u, their dot productwill not
change.
In
the flat space of a large sheet of paper, construction of a straight line
in a given direction starting at a given point
Po
is done by laying down the end
of
a vector (a straight edge) at
Po
pointing in the given direction, connecting
Po
to a neighboring point PI aloog the vector, moving the vectorparallel to
itself
to
PI, connecting PI to a neighboring point Pz, and continuing the process.
In
the
language of the machinery of the covariant derivative, we mightsay that a straight
line is constructed by transporting the tangent vector parallel to itself.
28.3.9. Definition.
Let
M be a manifold
and
y : [a, b] --* M a curve. Then y
geodesics
defined
is
called
a geodesic
of
M
if
the tangent
vector
u
at
every
point
of
y is parallel
transported along itself:
Vuu =
O.
geodesic
equation
It
follows from Equation (28.48}--with v
k
= uk = dx
k
/
dt-that
a geodesic

28.3
COVARIANT
DERIVATIVE
AND
GEODESICS
907
curve satisfies the following DE:
d
2xk
dx
i
dx
j
-+~.--=O
~~
dt
2
J'
dt
dt
.
This second-order DE, called the geodesic equation, will have a unique solution
if
xi(a) and xi
Cal,
i.e., the initial point and the initial direction, are given. Thus,
28.3.10. Box.
Through a given
point
and
in a given direction passes only
one geodesic curve.
28.3.11. Example.
Let usdetermine the geodesics of the space whose arc length is given
hyds
2
= (dx
2
+d
y2)/y2 (see Example
28.2.5).
With x =x
t
andy
=x
2,
we recognize
the
metric
tensor
as
1
su =
g22
=
2'
Y
gtl
= g22=
y'2
g12 =
g21
= 0,
Using
Equation
(28.26),we can
readily
calculate the
nonzero
connection coefficients:
I
J'nz
=
f12l
=
-f2tl
=
f222
=
-3"'
y
Thegeodesic
equation
forthe
first
coordinate
is
d
2x
dx
i
dx
i
_+f1.
__
=0
dt
2
J'
dt dt '
or
e. 1
(dx)2
1 dx dy 1
(d
y)2
dt
2
+
I'
tl
dt +
2f
12 dt dt +
I'
22 dt . =
O.
Tofindthe
connection
coefficients with
raised
indices,we
multiply
thosewithallindices
lowered
by the
inverse
of the
metric
tensor.
Forinstance,
f\2
=
gli
f
i12
=
gtl
f
tl
2
+
gI2
f212
= y2
(-~)
=
_.!:..
~
y y
~O
Similarly,
fl
tl
=0 =
fI
22
,
and the geodesic equation for thefirst coordinate hecomes
d
2x
1 dx dy
--2---
=0.
(28.66)
dt
2
y dt dt
Forthesecond
coordinate,
weneedr
2
I1
•
r
2
12
•and
r
2
22
·Thesecanbereadily
evaluated
as
above,
withtheresult
2 2 1
f
tl
=-f
22
= - ,
Y

908 28.
DIFFERENTIAL
GEDMETRY
yieldingthe geodesicequationfor the second coordinate
d
2y
+
.!.
(dx)2
_.!.
(d
y)2
=
o.
dt
2
Y dt Y dt
With x'"
dxldt,
Equation (28.66) canbe writtenas
dx _ 2X
dy/dt
= 0 '*
dx/dt
= 2
d
y/
dt
'* x =
ci.
dt y x Y
Usingthe chain rule andthe notation y'
==
dyjdx,
we obtain
dy t , C 2 /
dt = Y x = Y
y,
d
2
d
dy'
-2
Y
= C(2y..2'.y' +
i-x)
= C
2(
2y3yl2
+y4y").
dt dt dx
Substituting inEquation(28.67) yields
y3y'2 +y4
y"
+y3 = 0 '* (y')2 +
yy"
+I = 0 '* ..'£(yy') +
1=
O.
dx
(28.67)
isometry
defined
Isometries
ofa
manifold
form
a
subgroup
of
Diff(M).
Killing
vector
field
It
follows that ss'=
-x
+A and x
2
+y2 =2Ax +B. Thus, the geodesics are circles with
arbitrary radii whose centers lie on the x-axis. II
28.4 Isometrles and Killing Vector Fields
Whenever a structure is defined on a given family
of
sets, mappings between snch
sets that preserve that structure become the "natural" mappings. Thns, the natural
maps among vector spaces are
linear maps, among groups are homomorphisms,
among algebras are algebra homomorphisms, and among manifolds are smooth
maps. The introdnction of a metric on amanifold makes certain maps more privi-
leged than others.
28.4.1. Definition.
Let
M
and
N be Riemannian manifolds with metrics 9M
and
9N, respectively. The smooth
map
1/1
:M
->
N is called isometric
at
P E M
if
9M(X,
Y) = 9N(1/I.X,
1/IS)for
all X, Y E
Tp(M).
An
isometry
of
M to N is a
bijective smooth
map
that is isometric at every
point
of
M, in which case we have
1/I.9M
= 9N'
Of
special interest are isometries
1/1
: M -e- M of a single manifold. These
happen to be a subgroup
of
Diff(M), the group
of
diffeomorphisms
of
M. 10the
subgroup of isometries, we concentrate on the one-parameter groups of transfor-
mations. These define (and are defined by) certainvector fields:
28.4.2. Definition.
Let
X E
X(M)
be a vectorfield with integral curve F,. Then
X is calleda Killing vector
field
if
F
,
is an isometry
of
M.

28.4
ISOMETRIES
ANO
KILLING
VECTOR
FIELDS
909
The
following proposition follows innnediately from the definition
of
the Lie
derivative [Eqnation (26.29)].
28.4.3.
Proposition.
A vectorfield X E
X(M)
is a Killing vectorfield if
and
only
if
Lxg
=
O.
Choosing a coordinate system
{xi},
we write 9 =
gijdx
i
<81
dx!
and conclude
that
Xl
aj is a Killing vector field if and only
if
0=
Lx(gijdx
i
<81
dx
j)
=
X(gij)dx
i
<81
dx!
+
gij(LXdxi)
<81
dx
j
+
gijdx
i
<81
(Lxdxj).
Killing
equation
Using Equation (26.33) for the I-form
dx
k,
we obtain the Killing
equation
(28.68)
If
in Equation (28.45) we replace T with 9 and u with X, where X is a Killing
vector field, we obtain
where we have assumed thatthe covariant derivative is compatible with the metric
tensor. The reader may check thatEquation (28.69) leads to
Killing
equation
in
termsof
covariant
derivative
Xj;k
+
Xk;j
=
O.
This is another form
of
Killing equation.
(28.69)
(28.70)
28.4.4.
Proposition.
IIX
is a Killing vector field, then its inner product with the
tangenttoanygeodesicis constantalongthatgeodesic,i.e.,
ifu
is
such
a
tangent,
then Vu[g(u, X)] =
O.
Proof
We write the desired covariant derivative in component form,
k
i-o
i k
t-o
i k
i."
k
ji
u (gijU
Xl);k
= U gij;k U
Xl
+U
gij
U ;k
Xl
+U su» X ;k
'-,-'
'-,-'
=0 =0
1 i k
=
2:
(Xi;k
+
Xk;i)
U U ,
'-,---'
=0
by (28.70)
where the first term vanishes by assumption
of
the compatibility of the metric and
the covariant derivative, and the second term by the geodesic equation. D
28.4.5. Example. In a
flat
m-dimensional
manifold
wecanchoosean
orthonormal
coor-
dinateframe(Theorem28.2.9),sothattheKillingequation
becomes
(28.71)
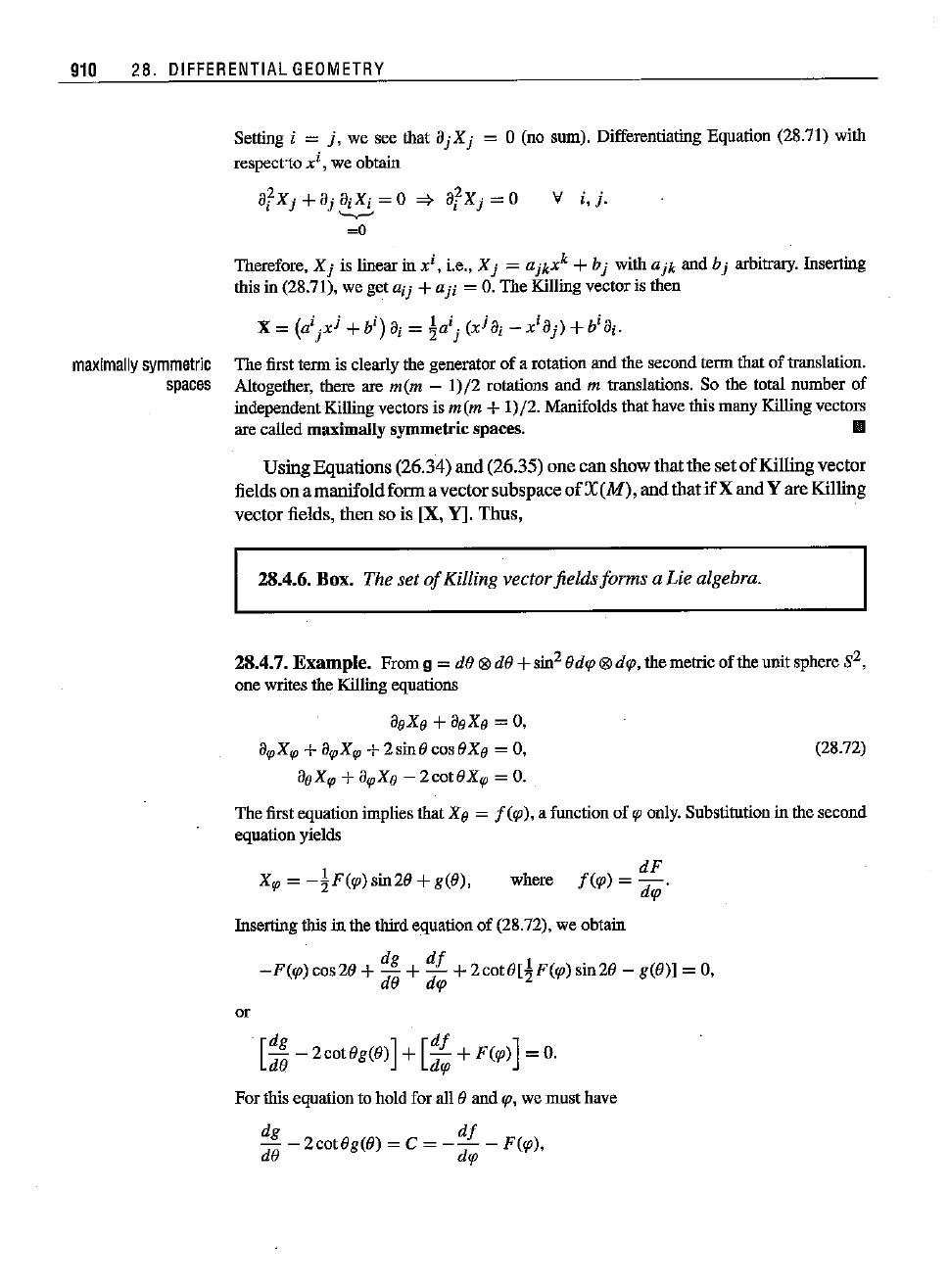
V t, j.
910 28.
DIFFERENTIAL
GEOMETRY
Setting i =
j,
we see that
OjXj
= 0 (no sum). Differentiating Equatioo (28.71) with
respect-to xi , we
obtain
2 2
0iXj+OjOiXi
=0
=}
0iXj
=0
-
~O
Therefore,
Xj is
linear
in
xi,
i.e., Xj = ajkXk + bj withajk andbj
arbitrary.
Inserting
this in (28.71), we get
aij
+
aji
=
O.
The Killing vector is thee
maximally
symmetric
spaces
x= (aijx
i
+hi)
aj
=
!a
i
j
(xi
aj
-
Xiaj)
+biaj.
The
first
term
is clearlythe
generator
of a
rotation
andthesecond
term
that
of
translation.
Altogether,
there
are
m(m - 1)/2
rotations
andm
translations.
So the total
number
of
independent
Killing
vectors
is m(m + 1)/2.
Manifolds
thathavethismanyKilling
vectors
arecalled maximally symmetric spaces.
IIlII
Using
Equations (26.34)
and
(26.35)
one
can
showthat
the
set
of
Killing vector
fields Ona
manifold
forma vectorsubspace
ofX(M),
and
that
if
X
and
Yare Killing
vector fields,
then
so is [X, V]. Thus,
28.4.6. Box. The set
of
Killing vector fields forms a Lie algebra.
28.4.7. Example. From 9 =dB
(i!)dB
+sin
2
Bdcp
(i!)dcp,
the metric ofthe unit sphere S2,
one writestheKilling
equations
iJeXe
+iJeXe= 0,
o~X~
+
O~X.
+ 2sinBcosBXe = 0,
oeX~
+
o~Xe
-
2cotBX~
=
O.
(28.72)
The
first
equation
impliesthat
X()
= !(rp), a
function
of ip only.
Substitution
in thesecond
equation
yields
X~
=
-iF(cp)
sin2B + g(B),
where
dF
f(cp) = dcp'
Insertingthis in the thirdequation of (28.72),we obtain
dg
df
1
-F(cp)cos2B+
dB +
dcp
+2cotB[ZF(cp)sin2B-g(B)]
=0,
or
. [d
g
df
dB - 2cotBg(B)] +
[dCP
+
F(CP)]
=
O.
Forthis
equation
toholdforall
()
andtp, wemusthave
dg
df
dB - 2cotBg(B) = C = -
dcp
- F(cp),

28.4
ISOMETRIES
AND
KILLING
VECTOR
FIELDS
911
where
C is a
constant.
Thisgives
g(O) =(Cl - C cot 0) sin
z
0
with
and
/('1') = Xe = A sin 'I' +B cos 'I'
x'"
=(A cos'I' - B sin
'1')
sinO
cose
+ Ct sin
z
O.
A
general
Killing
vector
fieldis
thus
givenby
where
Lx = -coscpoe +cot
61
sinrporp.
L
y
=sinqJoe
+cotBcoscp(}cp,
L
z
=
a",
are the generators of SO(3).
..
discussion
of
conformal
transformations
and
conformal
Killing
vector
fields
Sometimes it is useful to relax the complete invariance of the metric tensor
under the diffeomorphism
of
a manifold induced by a vector field and allow a
change
of
scale in the metric. More precisely, we consider vector fields X whose
flow
F
,
changes the metric
of
M. So
F,.g
= e¢(I)g, where e is a a real-valued
function on
M that is also dependent on the parameter t. Such a transformation
keeps angles unchanged but rescales all lengths.
In analogy with those of the
complex plane with the same property, we call such transformations conformal
transformations. A vector field that generates a conformal transformation will
satisfy
k k
k·
X aUij +aiX gkj +
ajX
gki = -1/Jgij,
a"'j
1/J='-
,
at
1=0
(28.73)
and is called a conformal Killing vector field.
Wenow specialize to a flatm-dimensionalmanifoldandchoose anorthonormal
coordinate frame (Theorem 28.2.9). Then Equation (28.73) becomes
aiXj
+ajXi = -1/Jgij.
Multiply both sides by gij and sum over i to obtain
Remember
Einstein's
summation
convention!
. . m
za'
Xi = -m1/J
=}
a'Xi = -21/J,
m=dimM.
(28.74)
Apply
a
i
to both sides
of
Equation (28.74) and sum over i. This yields
(28.75)

912
28.
DIFFERENTIAL
GEDMETRY
Differentiate both sides of the second equation in (28.75) with respect to x
k
and
symmetrize the result in j and k to obtain
(m - 2)aiak1ft =
aiai(akXi
+
aiXk)
=
-gikaiai1ft.
Raising the index j and contracting it with k gives a
i
ad
= 0 if m
t=
I.
It
follows
that
(28.76)
Equations (28.74), (28.75), and (28.76) detemnine both
1ft
and
Xi
if
m
t=
2.
It
follows from Equation (28.76) that
1ft
is linear in
x,
and, consequently [from
(28.74)], that
Xi is at most quadratic in x. The most general solution to Equation
(28.74) satisfying (28.75) and (28.76) is
(28.77)
conformal
group
dilitation
inversion,
or
special
conformal
transformation
where
aij
=
-a
ji
andindices
of
theconstants areraisedandloweredas usual.
Equation(28.77) givesthe generators
of
an [(m+1)(m+2)/2]-parametergroup
of
transformations on R
m
,
m
t=
2 calledthe
conformal
group. The reader should
note that translation (representedby the parameters b
i
)
and rotations (represented
by the parameters aij) are included in this group. The other finite (as opposed to
infinitesimal) transfomnations
of
coordinates can be obtained by using Equation
(27.25). For example, the finite transfomnation generated by the parameter 8 is
given by the solution to the DE
dx'!
[dt
=
x'l
, which is
x'i
=
e'x
i,
or
x'i
=
e"
xi,
and is called a dilitation
of
coordinates. Similarly, the finite transfomnation
generated by the parameter ci is given by the solution to the DE
dx'i
[de,
=
8
ij
x'k
x' -
2X'i
x'j or
k '
which is called inversion, or the special
conformal
transformation.
Equations
(28.75), and (28.76) place no resttiction on
1ft
(and therefore on
Xi)
when m = 2.
This means that
.
28.4.8. Box. The conformal group is infinite-dimensional
for
R
2
.
In
fact, we encountered the confomnal transfomnations
of
R
2
in the context
of
complex analysis, where we showed that any (therefore, infinitely many) analytic
function is a confomnal traosfomnation of C
~
R
2
•
The confomnal group of R
2
has important applications in string theory and statistical mechanics, but we shall
not pursue them here.
