Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


25.3
EXTERIOR
ALGEBRA
743
Weconcludethat thethreevectorsarelinearlydependent.
III
As an application
of
Proposition 25.3.8, let us prove the following.
Cartan's
lemma
25.3.10.
Theorem.
(Cartan's lemma) Suppose that lei
};~1'
P ::: dim V,form a
linearly independentset
of
vectors in V
and
that
IVilf~1
are also vectors in Vsuch
that
L;~1
ei
/\
Vi
=
O.
Then all
Vi
are linear combinations
of
onlythe set lei
};~1'
Furthermore, ifVi =
L}=1
Aijej,
then
Aij
=
Aji.
Proof
Multiplying both sides
of
L;=1 ei /\
Vi
= 0 by ez/\
...
/\
e
p
gives
-VI
/\ er /\ e2 /\
...
/\ e
p
= O.
By Proposition 25.3.8, VI and the
ei are linearly dependent. Similarly, by multi-
plying
L;~1
ei /\
Vi
= 0 by the wedge product with ek missing, we show that Vk
and the ei are linearly dependent. Thus, Vk =
L;=1
Akiei,
for all k. Furthermore,
wehave
p p p
o=
I:ek
/\
Vk
= L
I:ek
/\
(Akiei) =
L(Aki
-
Aik)ek
/\
er,
k=l
k=l
i=l
k-ci
where the lastsum is over both k and i with k < i, Clearly, Iek
/\
ei}with k < i are
linearly independent (show this!). Therefore, their coefficients must vanish. D
Elie Joseph Carlan
(1869-1951), born in Dolomieu (near
Chambery), Savoie,Rhone-Alpes,
France,
becamea
student
at theEcole
Normale
in 1888 and
obtained
his
doctorate
in
1894. He lecturedat Montpellier (1894-1896), Lyon
(1896-
1903), Nancy (1903-1909), and Paris (1909-1940). He had
fourchildren, oneof whom,
Henri
Cartan,
wastoproducebril-
liantwork
in
mathematics.
Twoothersdiedtragically. Jean,a
composer,
diedattheageof25, whileLouis,a physicist, was
arrested
bythe
Germans
in 1942and
executed
after
15
months
in
captivity.
Cartan
added
greatly
to the
theory
of
continuous
groups,
which hadbeen
initiated
by
Lie.
His thesis (1894)
contains
a
major
contribution
to Lie
algebras
wherein
he
completed
theclassification ofthe
semisimple
algebras
that
Killing
had
essentially
found.
He
then
turned
tothe
theory
of
associative
algebras
and
investigated
the
structure
forthese
algebras
overtherealand complex
fields.
Wedderburn
would
complete
Cartan's
work
inthis
area.
He
then
turned
to
representations
of
semisimple
Lie
groups.
His
work
is a
striking
synthesis
ofLie
theory,
classical
geometry,
differential
geometry,
and
topology,
whichwas
tobe
found
inallCartan's
work.
Healso
applied
Grassmann
algebra
tothe
theory
of
exterior
differential
forms,
By 1904
Cartan
was
turning
to
papers
on
differential
equations,
and
from
1916 on
he
published
mainly
on
differential
geometry.
Klein's
Erlanger Program was seento be

744 25.
ALGEBRA
OF
TENSORS
inadequateasageueraldescriptionofgeometryby
Weyl
and
Veblen,
andCarlanwastoplay
a
major
role. He examineda spaceactedon by an
arbitrary
Lie
group
of
transformations,
developing
a
theory
ofmoving
frames
that
generalizes the
kinematical
theory
of
Darboux.
Cartan
further
contributed
to
geometry
withhis
theory
of
symmetric
spaces,
whichhave
their
origins
in
papers
he
wrote
in 1926.Itdevelops ideas
first
studied
by
Clifford
and
Cayley
and used topologicalmethodsdevelopedby Weylin 1925.This work was completedby
1932.
Cartan
thenwenton to examine
problems
on a topic
first
studiedby
Poincare.
By this
stage
hisson,HenriCartan,was
making
major
contributions
to
mathematics,
and
Elie
Cartan
wasabletobuildon
theorems
proved
byhisson.
Cartan
also
published
work
on
relativity
andthe
theory
of
spinors.
Heis
certainly
one
of themost
important
mathematicians
ofthe
first
half of the
twentieth
century.
25.3.1 The Determinant
One
of
the most beautiful applications
of
exterioralgebra is in the theory
of
deter-
minants. We have already considered determinants in detail in Chapter 3, where
we noted how messy it was to prove some
of
the theorems. With the machinery
of
exterior algebra at our disposal, we will see how elegant this theory becomes and
how trivial some
of
the proofs will
tum
out to be.
First, let us recall that
dim
AN
(V) = I when N = dim V. This means that if
{ed!:!
is abasis
of
V,thenej
/\ez/\'
.
·/\eN
is the onlyvectorin the corresponding
basis
of
AN (V). On the otherhand, if
{Vi
I!:!
is any set
of
N vectors, the product
vi
/\
Vz
/\
...
/\
VN
is either zero
(if
the Vi'Sare linearly dependent)
or
a nonzero
product belonging
to
AN
(V). Since e! /\ ez /\ /\ eN is a basis
of
fIN
(V), we
conclude that for any set
of
N vectors,
vi.
vz. ,
VN
E V, the product vi /\
Vz
/\
...
/\
VN
is a multiple (possibly zero)
of
e! /\ ez /\
...
/\ eN.
Now let A be a linear operator on V.
The
set
of
vectors Ae!, Aez,
...
, AeN
all belong to V. By the above remarks (Ae!) /\
...
/\ (AeN) is proportional to
e! /\ ez /\
...
/\ eN. We now show that the proportionalityconstant is simplydet A.
determinant
in
terms 25.3.11. Theorem. Let
A:
V --> V be
linear.
Let
{ed!:!
bea basisfor V. Then
of
wedge
products
(Ae!) /\
...
/\ (AeN) = (detA)e! /\
...
/\ eN.
Proof LetAe
r
=
A~eir'
forr = 1,2,
...
, N. Then
It
is straightforward to show that
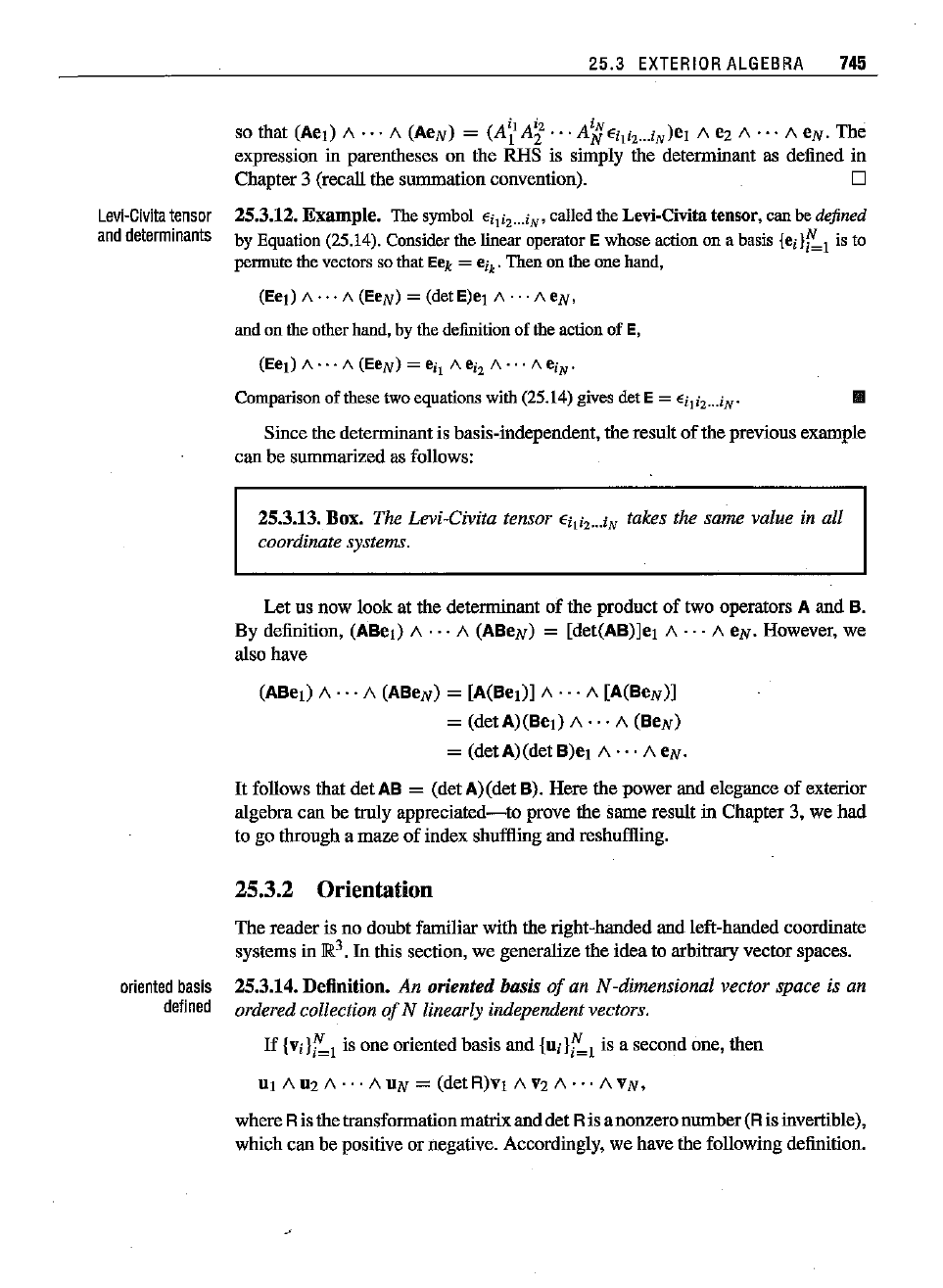
(25.13)
(25.14)
25.3
EXTERIOR
ALGEBRA
745
so that (Ael) /\
...
/\ (AeN) = (A\l
A~
...
A~
Eili2
...iN)ej /\
ez
/\
... /\eN. The
expression in parentheses on the RHS is simply the determinant as defined in
Chapter 3 (recall the summation convention). D
Levi-Civita
tensor
25.3.12. Example. The
symbol
Eili2
...iN'calledtheLevi-Civitatensor, canbe
defined
and
determinants
by
Equation
(25.14).
Consider
the
linear
operator
Ewhose actionon a basis
{ed~l
is to
permute the
vectors
so
that
Eek = eik'
Then
ontheone
hand,
andonthe
other
hand,
bythe
definition
of the
action
of E,
(Eel) A
...
A (EeN) = eit 1\ ei2 A
...
1\ eiN'
Comparison
of these two
equations
with(25.14)gives detE=
filiz
...iN"
III
Since the determinant is hasis-independent, the result
of
the previous example
can be summarized as follows:
25.3.13. Box. The Levi-Civita tensor
Eili2
...
iN
takes the same value in all
coordinate systems.
Let us now look at the determinant of the product of two operators A and B.
By definition, (ABel) /\
...
/\ (ABeN) = [det(AB)]ej /\
...
/\ eN. However, we
also have
(ABel) /\
...
/\ (ABeN) =
[A(Bel)]/\'"
/\ [A(BeN)]
= (det A)(Bej) /\
...
/\ (BeN)
= (detA)(det B)el /\
...
/\ eN.
It
follows that detAB = (det A)(det B). Here the power and elegance of exterior
algehra can be truly
appreciated-to
prove the same result in Chapter 3, we had
to go through a maze
of
index shuffling and reshuffling.
25.3.2 Orientation
The reader is no doubt familiar with the right-handed and left-handed coordinate
systems in
~3.
In
this section, we generalize the ideato arbitrary vector spaces.
oriented
basis
25.3.14. Definition. An oriented basis
of
an Nsdimensional vector space is an
defined
ordered collection
of
N linearly independentvectors.
If
{Vi
l;;"j is one oriented basis and {uil;;"1 is a second one, then
UI/\
U2/\'"
/\
UN
= (det
Rjvj
A
V2/\"'/\
VN,
where Ris the transformationmatrix and det Ris anonzeronumber(Ris invertible),
which can be positive or negative. Accordingly, we have the following definition.

746 25.
ALGEBRA
OF
TENSORS
oriented
vector
spaces
defined
volume
element
ofa
vector
space
25.3.15. Definition. An orientation is the collection
of
all oriented bases related
byatransformation matrixhavingapositive determinant.Avector spacefor which
anorientationis specified is called an oriented vector space.
Clearly, thereare onlytwo orientations in any vector space. Eachorientedbasis
is positively related to any oriented basis belonging to the same orientation and
negatively related to any oriented basis belonging to the other orientation. For ex-
ample, inJR
3
,
the bases {ex, e
y,
e,} and {e
y,
ex, e
z}
belongto differentorientations
because
The
first basis is (by convention) called a
right-handed
coordinate system, and
the second is called a
left-handed
coordinate system. Any
other
basis is either
right-handed or left-handed. There is no third altemative!
25.3.16. Definition.
Let V be a vector space. Let V* have the oriented basis
(e
i
}~I'
The oriented volume elementI-' E
AN
(V*)
of
V is defined as
positive
orientation
Note that if
[e.]
is ordered as {e
j
}, then I-'(e" e2,
...
,
ev)
= +
liN!,
and we
say that {e.} is
positively oriented with respect to
1-'.
In general, {v;}is positively
oriented with respect to I-'if I-'(VI,
V2,
...
,
VN»
O.
The
volume element
of
V is defined in terms
of
a basis for V*.
The
reason for
this will become apparentlater, whenwe shall see that
dx,
dy,
and dz form a basis
for
(ffi.3)*,
and dx dy
dz
sa
dx
A dy
Adz.
25.3.3 SymplecticVector Spaces
Mechanics was a great contributor to the development
of
tensor analysis.
It
pro-
vided examples
of
manifolds that went beyond
mere
subspaces
of
JRn.
The phase
space
of
Hamiltonianmechanicsis a paradigm
of
manifolds thatare not "hypersur-
faces"
of
some Euclidean space. We shall have more to say about suchmanifolds
in Chapter 26. Here, we shall be content with the algebraic strocture underlying
classical mechanics.
symplectic
form,
symplectic
vector
space,and
sympiectic
transformation
25.3.17. Definition. A 2-form w E A
2
(V*) is called nondegenerate if
w(v],
V2)
= 0 for all VI E V implies
V2
=
O.
A symplectic form on V is a
nondegenerate2-form
WE
A
2(V*).
The pair (V, w) is called a symplectk vector
space.
If
(V, w) and (W, p) are symplectic vector spaces, a linear transforma-
tion
T : V
--+
W is called a symplectic transformation or a symplectic map if
T*p=w.
Any 2-form (degenerate or nondegenerate) leads to other quantities that are
also
of
interest.
For
instance, given any basis
{Vi}
in V,one defines the
matrix
of
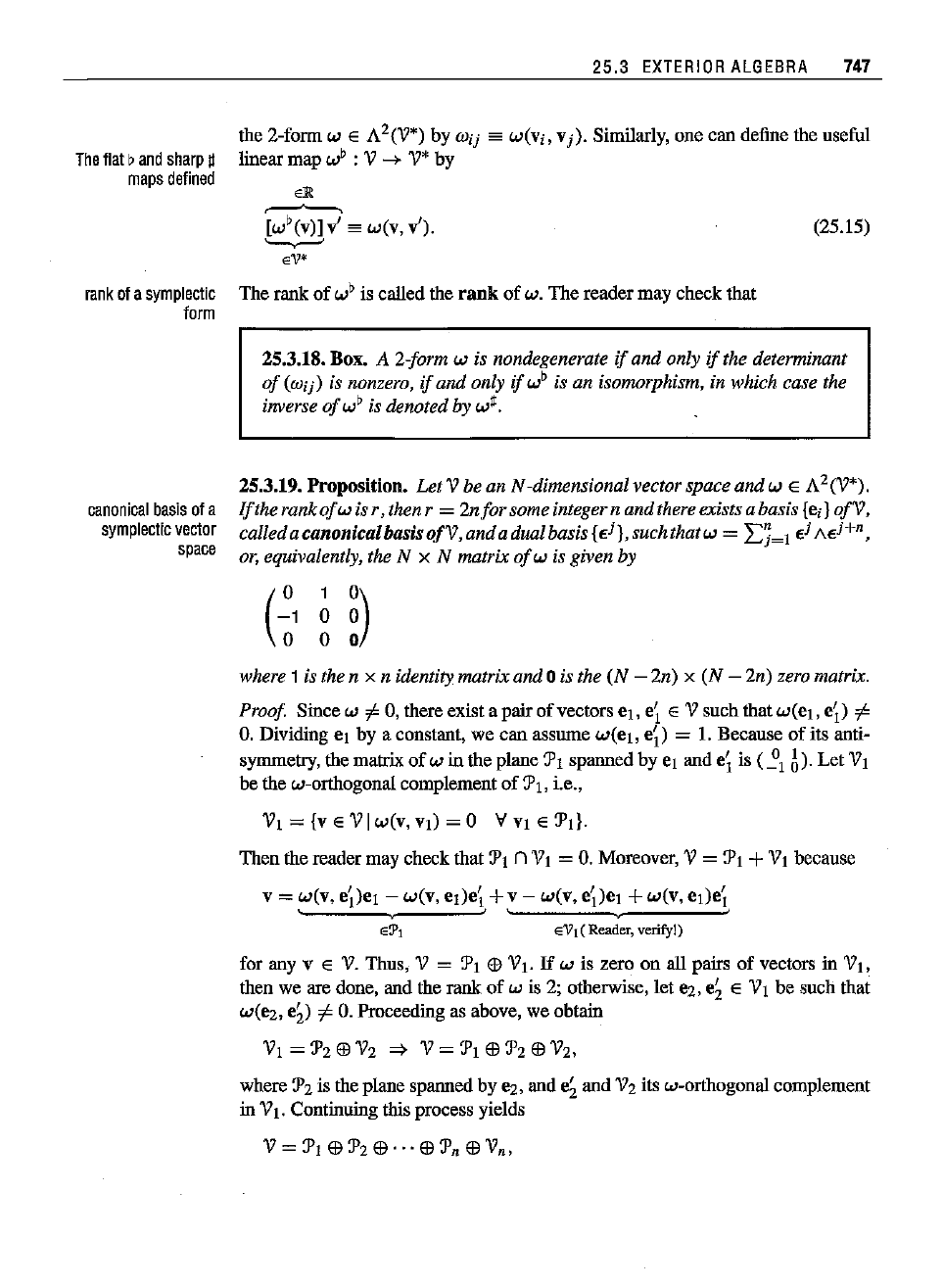
The
flat, andsharpd
maps
defined
25.3
EXTERIOR
ALGEBRA
747
the 2-form
WE
A
2(V*)
by wii sa W(Vi,
vi)'
Similarly, one can define the nseful
linear map
w'
:V
--->
V* by
eR
'[W'(v)]Vi es w(v, Vi).
~
e11*
(25.15)
rank
ofa
symplectic
The
rank
of
w' is called the
rank
of
w.
The
readermay check that
form
25.3.18. Box. A 2-form W is nondegenerate if and only if the determinant
of
(wi}) is nonzero, ifand only if
w'
is an isomorphism, in which case the
inverse
of
w'
is denoted by
w~.
canonical
basis
ofa
symplectic
vector
space
25.3.19. Proposition.
Let
V be an N-dimensional vector space
and
W E A
2
(V*).
If
the
rankofw
isr,
thenr
=
2nfor
some integer n and thereexists a basis [e.}
of
V,
calledacanonicalbasis
of
V,
anda
dual basis {Ei},suchthatw =
L'i~1
Ei/\EJ+n,
or,equivalently, the N x N matrix
of
w is given by
1
0)
o 0
o 0
where 1 is the n x n identity matrixand 0 is the
(N
- 2n) x
(N
- 2n) zero matrix.
Proof
Since w
01
0, there exist a pair
of
vectors
ei,
e; E V snch that
w(
el,
e;)
01
O.
Dividing el by a constant, we
can
assume
w(el,
e;)
= 1. Because
of
its anti-
symmetry, the matrix
of
w in the plane
Pj
spannedby er and e; is
C~\
A).
Let
VI
be the w-orthogonal complement
of
:PI, i.e.,
VI
=(v E V Iw(v, VI) =0 V VI E :PJl.
Then the reader may check that
P,
nVI =
O.
Moreover, V =:PI +VI because
v
= ,w(v,
e;)el
- w(v,
el)e;,
+":'
-
w(v,
e;)el
+w(v,
el)e;,
eVr (Reader, verify!)
for any v E V. Thus, V = :PI
E9
VI.
If
w is zero on all pairs
of
vectors in VI,
then we are done, and the
rank
of
w is 2; otherwise, let e2,
e;
E VI be such that
w(e2,
e;)
01
O.
Proceeding as above, we obtain
VI
=:P2
E9
V2
=}
V = :PI
E9:P2
E9
V2,
where :P2is the plane spanned by 02, and
e;
and V2 its w-orthogonal complement
in VI. Continuing this process yields
V
= :PI
E9:P2
E9
...
E9:P
n
E9
V
n,

748 25.
ALGEBRA
OF
TENSORS
where V.. is the subspace
of
V ou which w is zero. Thisshows that the rank
of
w is
2n. By
reordering
thebasisvectorssuchthat
e~
==
en+k, we construct anew basis
lei
1f:,1
in whichw has the desired matrix.
To concludethe proposition, it is sufficientto show
that
L:
J
= l e
i
A e
i
+
n,
in
which
{eilf=,
is dual 10 lei
1f:,1
,has
the samematrix
asw.
This is left as an exercise
furthereoo~
D
We note that in the canonical basis,
if
i, j
::::
n,
if
j = n +k, k :::n,
ifi
::0:
2n or j
::0:
2n.
If
we write v E Vas v =
L7=,
(Xiei +
Yie
..+i) +Lf:,j2 .. ziC2..+i in the
canonical basis
of
V,with a corresponding expansion for v', then the reader may
verify that
..
w(v, v') =
~)xiyi
- XiYi).
i=l
The following proposition gives a useful criterion for nondegeneracy
of
w:
25.3.20.
Proposition.
Let w be a 2-form in thefinite-dimensional vector space V.
Then w is nondegenerate iffVhas even dimension, say 2n,
and
w" es w
A··
. A w
is a volume element
ofV.
Proof
Suppose w is nondegenerate. Then,
w'
is an isomorphism. Therefore, the
rank
of
w, an eveu
number
by Proposition 25.3.19,
must
equal
dim
17*
=
dim
V.
Moreover, by taking successive powers
of
w and using mathematical induction,
one can show that
w" is proportional to e
l
A
...
A e
2
",
Conversely,
if
w
n
ex:
e
1
1\
...
/\
e
2n
.isa volumeelement, thenbyProposition
25.3.8, the
{eil
are linearly independent. Furthermore,
dim
17*
must
equal the
number
of
linearlyindependent factors in the
wedge
product
of
a volumeelement.
Thus,dim
17*
=
2n.But2n
is also the
rank
ofw.
Itfollows
thatw'
is onto.SinceVis
finite-dimeusioual, the dimension theorem implies that
w'
is an isomorphism. D
25.3.21.
Example.
Let Vbe a vectorspaceand
17*
its dual.The directsum V
Ell
'.1*
can
be turnedinto a symplecticvectorspace
if
wedefineW E A
2(17
Ell
'.1*)
by
W(v +1",v' +1"')
==
1'"
(v) -
I"(v),
wherev, v' E V and 1",
1'"
E
'.1*.
The readermay verify that (V
Ell
'.1*,
w) is a symplectic
vector
space.
This
construction
ofsymplecticvectorspacesisclosely
related
to
Hamiltonian
dynamics,to whichweshallreturn in Chapter26.
III

(25.16)
25.4
INNER
PROOUCT
REVISITEO
749
Suppose (V, w) and (W,
p)
are 2n-dimensional symplectic vector spaces.
Then, by Proposition25.3.7, any symplectic
mapT
: (V, w) --+
(W,
p)
is volume-
preserving, i.e., (T*
p)n is a volume element
of
W.
It
follows lbat lbe rank
of
T*
is
2n, and by Proposition 1.3.18, so is lbe
rank
ofT.
The
dimension lbeorem now
implies lbat T is an isomorphism. Symplectic transformations on a single vector
space have an interesting property:
25.3.22.
Proposition.
Let
(V, w) be a symplectic vector space. Then the set
of
symplectic
group
symplectic mappings T : (V, w) --+ (V,
w)
forms a group under composition,
called the symplectic group and denoted by
Sp(V,
w).
Proof Clearly, Sp(V, w) is
asubsetofGL(V).
One
needonlyshow lbatlbeinverse
of
a symplectic transformation is also such a transformation and lbat lbe product
of
two symplectic transformations is a symplectic transformation.
The
details are
left for lbe reader.
0
symplectic
matrices
A matrixis called symplectic
if
it is lbe representation
of
a symplectictransfor-
mation in a canonical basis
of
lbe underlying symplectic vector space. The reader
may check lbatlbe condition for a matrix
A to be symplectic is AtJA = J, where J
is therepresentation
of
w in thecanonicalbasis:
J =
(0
1)
-1
0 '
where 1 and 0 are lbe n x n identity and zero matrices, respectively.
25.4 Inner Product Revisited
The inner product was defined in Chapter 1 in terms
of
a metric function lbat took
two vectors as inpnt and manufactored a number. We now know what kind
of
machine this is in lbe language
of
tensors.
symmetric
bilinear
25.4.1.
Definition.
A symmetric bilinear
form
9 on V is a symmetric tensor
of
form
type (0, 2).
If
{ej
}r~l
is a basis
of
V and
{.i}~l
is its dual basis, lben 9 =
gij.i.
j
(recall
Einstein's
summation
convention),becausee
i
e
J
= e
i
@ e
j
+e
j
® e
i
formabasis
of
82 (V). For any vector v E V, we can write
9(V) =
gij.i.
j
(v) =
gij.i.
j
(vkekl
=
vkgij.i
.j
(ek) = gijV
j•
i.
'-,,-'
=!if
Thus, 9(V) E V*. This shows that 9 can be thought
of
as a mapping, 9 : V
--+
V*, given by Equation (25.16).
For
lbis equation to make sense, it should not
matter which factor in lbe symmetric product v contracts wilb.
But
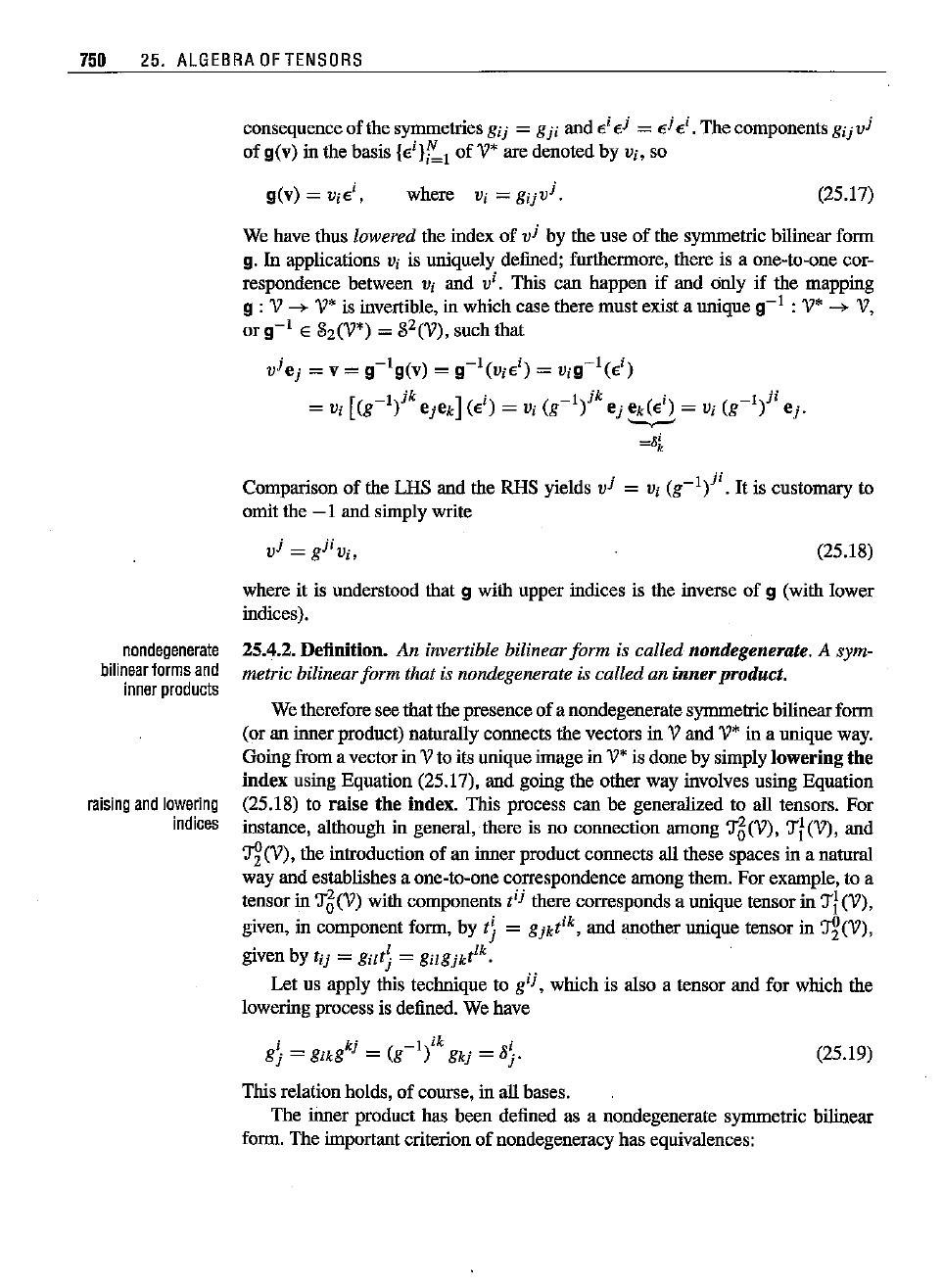
this is a trivial
750
25.
ALGEBRA
OF
TENSORS
consequence
of
the
symmetries
gij
=
gji
and
f;.i
f)
=€i e', The
components
gijV
i
of g(v) in the basis
lei}!:1
ofV'
are denoted by Vi, so
g(v) =
Viei,
(25.17)
We have thus lowered the index
of
vj
by the use
of
the synunetric bilinear form
g. Iu applications Vi is uniquely defined; furthermore, there is a one-to-one cor-
respondence between
Vi and
vi.
This can happen
if
and only if the mapping
9 : V
--+
V'
is invertible, in which case there must exist a unique
g-1
: V* --+V,
or
g-I
E S2(V*) = S2(V), such that
vjej
= v =
g-Ig(v)
=
g-I(Viei)
=
vig-I(e
i)
= Vi
[(g_l/k
ejek]
(e')
= Vi
(g_l/
k
ej
ek(e
i)
= Vi
(g-l/,
ej.
---
=8~
Comparison of the LHS and the RHS yields
vj
= Vi
(g-1
/i.
It
is customary to
omit the
-I
and simply write
(25.18)
nondegenerate
bilinear
forms
and
inner
products
raising
and
lowering
indices
where it is understood that 9 with upper indices is the inverse of 9 (with lower
indices).
25.4.2. Definition.
An invertible bilinear form is called nondegenerate. A sym-
metric bilinearform that is nondegenerate is
called
an
inner
product.
We therefore see that the presence of a nondegeneratesymmetric bilinearform
(or an inner product) naturally connects the vectors in V and V* in a unique way.
Going from a vector in Vto its unique image in
V'
is done by simply lowering
the
index using Equation (25.17), and going the other way involves using Equation
(25.18) to raise
the
index. This process can be generalized to all tensors. For
instance, although in general, there is no connection among 'J'5(V), 'J'!(V), and
':!1(V), the introduction of an inner product connects all these spaces in a natural
way and establishes a one-to-one correspondence among them. For example, to a
tensor in 'J'5(V) with components
tij
there corresponds a unique tensor in 'J'!(V),
given, in component form, by
tj
=
gjktik,
and another unique tensor in ':!1(V),
given by
tij
=
giltj
=
gilgjkt1k.
Let us apply this technique to
gij,
which is also a tensor and for which the
lowering process is defined. We have
(25.19)
This relation holds, of course, in all bases.
The inner product has been defined as a nondegenerate synunetric bilinear
form. The important criterion
of
nondegeneracy has eqnivalences:

g-orlhogonal
and
null
vectors
25.4
INNER
PRODUCT
REVISITED
751
25.4.3.
Proposition.
A symmetric bilinear
form
9 is nondegenerate ifand only if
1. the matrix
of
components gij has a nonvanishing determinant, or
2.
for
every nonzero v E V,there exists
WE
Vsuch that g(v, w)
t=
o.
Proof
The
first part is a direct consequence
of
the definition
of
nondegeneracy.
The second part follows from the fact that
9 : V -->
V'
is invertible
iff
the nullity
of
9 is zero.
It
follows that if v E V is nonzero, then g(v)
t=
0, i.e., g(v) is not the
zero functional. Thus, there must exist a vector w
E Vsuch that [g(v)](w)
t=
O.
The proposition is proved once we note that [g(v)](w) sa g(v, w). 0
25.4.4. Definition. A general(not necessarily nondegenerate) symmetricbilinear
form
9 can be categorized as follows:
1. positive (negative) definite:
g(v, v) > 0 [g(v, v) < 0]
for
every nonzero
vectorv;
2. definite: 9 is eitherpositive definite or negative definite;
3. positive (negative) semidefinite:
g(v, v)
'"
0 [g(v, v)
:s
0]
for
every
v;
4. semidefinite: 9 is eitherpositive semidefinite or negative semidefinite;
5. indefinite:
9 is not definite.
25.4.5. Example.
Some
of
the
categories
of
the definition above
can
be illustratedin
:[R2
with
vi
=
(xj
,
Yd,
v2 = (X2, Y2), and v = (x, y).
(a) Positive definite: g(Vl, Vl) =
XIX2
+YIY2
because if v
'f:.
0, thenone
of
its components
is nonzero,
and
g(v,
v) =x
2
+y2 > O.
(b) Negative
definite:
g(vl. V2) =
!(XjY2
+ X2Y1) - XjX2 - YIY2 because g(v, v) =
xy
- x
2
-
y2 = -!(x - y)2 _ !x
2
- !y2. which is negative for nonzero v.
(c) Indefinite: g(vl. V2) = XjX2 - YIY2. For v = (x,
x),
g(v, v) =
O.
However,
9 is
nondegenerate, because it hasthe invertible matrix 9 =
(6
~1)
in the standard basis
of~2.
(d)Positive semidefinite:
g(Vl.
V2) =
XIX2
::::} g(v, v) = x
2
andg(v, v) isnever negative.
However,9 is degenerate because its matrix in the standard basis oflR.
2
is 9
=
(6
g),
which
is not invertible. II
Two vectors u, v E Vare said to be
g-orthogonal
if
g(u,
v) =
O.
A
null
vector
of
9 is avectorthat is g-orthogonalto itself.
If
9 is definite, then the onlynull vector
is the zero vector. The converse is also true, as the following proposition shows.
25.4.6.
Proposition.
If
9 is not definite, then there exists a nonzero null vector.
Proof
That 9 is not positive definite implies that there exists a nonzero vector
v
E V such that g(v, v)
:s
o.
Similarly, that 9 is not negative definite implies that
there exists a nonzero vector w
E V such that g(w, w) '"
O.
Construct the vector
u
=
av
+(1 -
a)w
and note that
g(u,
u) is a continuous function
of
a.
For
a = 0
this function has the value g(w, w) '"
O,andfora
=
lit
has the value
g(v,
v):S
O.
Thus, there must be some a for which
g(u,
u) =
O.
0

752 25.
ALGEBRA
OF
TENSORS
25.4.7.
Example.
In the specialtheoryofrelativity, the innerprodoetof two"position"
four-vectors,
rt
= (xj ,
Yl,
zj ,
efl)
and rz = (X2. Y2, Z2,et2), where c is the speed of light,
is defined as
This is clearly an indefinite symmetric bilinear form. Proposition 25.4.6 tells us that there
must exist a nonzero null vector. Such a vector r satisfies
g(r, r) = e
2
t
2
-
x
2
- i - z2 = 0,
x
2
+y2 +z2
,;
x2
+y2 +Z2 distance
or c
2
= 2
::::}
c = ± =
±-.--.
This corresponds to a
t t time
particle moving withthe speed
of
light. Thus, light rays are the null vectors in the special
theoryofrelativity.
III
g-orthonormal
vectors,
diagonal
components
ofg,
index
and
signature
a!9
polarization
identity
Whenever there is an inner product on a vector space, there is the possibility
of orthogonal basis vectors. However, since g(v, v) is allowed to be negative or
zero, it is impossible to demand normality for some vectors.
25.4.8. Definition.
A basis
(eil~t
of
V is g-orthonormal if g(ei,
ej)
= 0 for
i
i'
i.and g(ei,
e.)
(nosumf) is+
I,
-I,
orO. The g(ei,
ei)
are called thediagonal
components
ofg.
We use n+,
n_,
and no to denote the number
of
vectors ei for
which
g(ei, ei) is, respectively, +I,
-I,
or
O.
The integer n.: is called the index
of
g, and s = n+ -
n_
is called the signature
of
g.
The existence of orthonormal bases was established for positive definite g
by the Gram-Schmidt orthonormalization process in Chapter I. One of the steps
in this process is division by (the square
root
01) g(v, v), which could be zero
in the present context. A slight modification
of
the Gram-Schmidt process, in
conjunction with the procedure used in the symplectic case, can help construct an
orthonormal basis for a general (possibly indefinite) g. To be specific, start with
the
polarization
identity,
g(v,
v') =
![g(v
+v', v +v') - g(v - v', v - v')],
and use it to convince yourselfthat 9 is identically zero unless there exists a vector
e) such that
g(e),
ej)
i'
O.
Rescaling, we
can
assume that
g(et,
et)
==
~)
= ±1.
Let V) be the span
of
et and V2 the g-orthogonal complement of Vt. Clearly,
Vt
nV2 =
(OJ;
moreover, VI +V2 = V,
forifv
E V, we can write
v
=
~tg(v,
eirer
+v -
mg(v,
et)et
.
~.
eV
1
eV2
Now,
if
9 = 0 on V2, we are done; otherwise, there is a vector 02 such that
g(e2, e2) sa
~2
= ±1. We continue indnctively to complete the construction. We
therefore have the following:
