Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

24.9
PROBLEMS
723
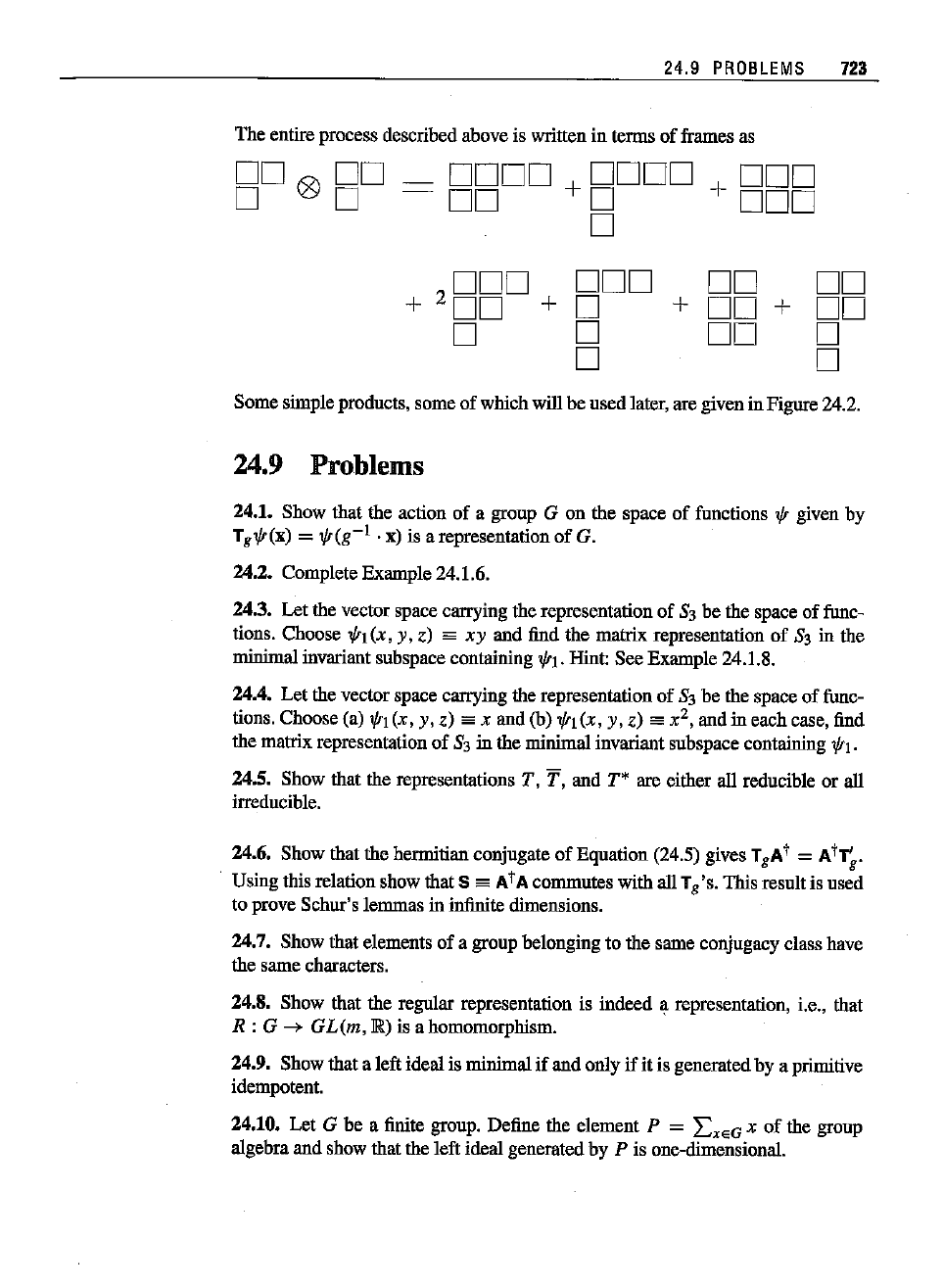
The entire process describedabove is written in terms of frames as
DO
Q9
DO
DODD
+OODO
+
DOD
D D
-DO
0
DOD
0
2
0 0 D
DOD
DO
DO
+
DO
+
0
+
OD
+
DD
0
0
OD
D
0
D
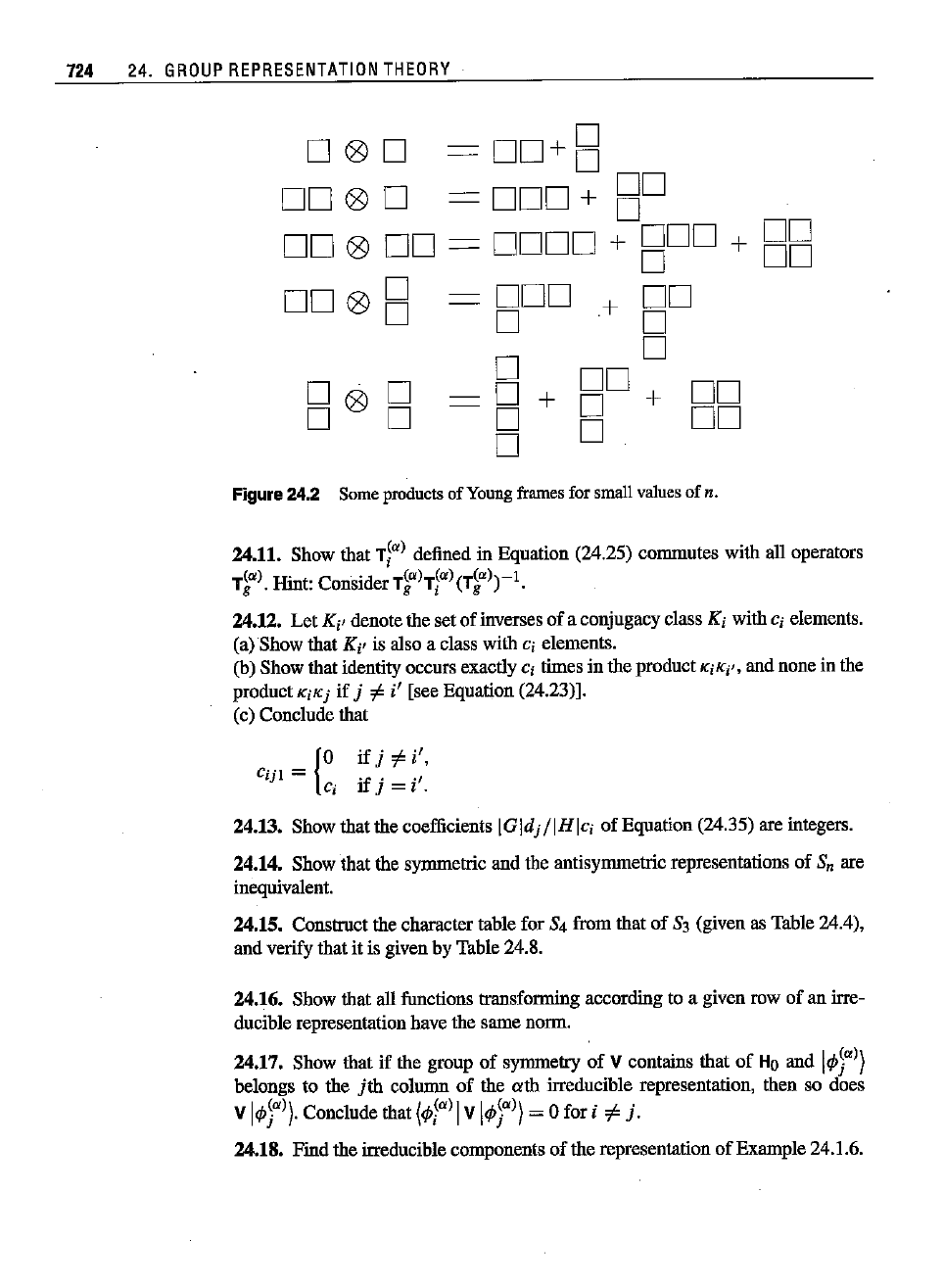
Some simpleproducts,someof whichwill be used later,are givenin Figure 24.2.
24.9 Problems
24.1. Show that the action of a group G on the space of functions
1/1
given by
T
g1/1(x)
=
1/1(g-1
. x) is a representationof G.
24.2. CompleteExample 24.1.6.
24.3. Let the vectorspacecarrying the representation of 83 be the space of func-
tions. Choose
1/11
(x, y, z) sa
xy
and find the matrix representation of 83 in the
minimal invariantsubspacecontaining
1/11.
Hint: See Example 24.1.8.
24.4. Let the vector space carrying the representation of 83be the space of func-
tions. Choose(a)
1/11
(x,
y, z)
==
x and (b) 1/1t(x, y, z)
==
x
2
,
andin each case, find
the matrix representationof 83in the minimal invariant subspacecontaining
1/11.
24.S. Show that the represeutations T, T, and T* are either all reducible or all
irreducible.
24.6. Show that the hermitian conjugate of Equation (24.5) gives TgAt
=
AtT'g'
Usingthis relation showthat S sa AtAcommutes with all Tg's. This result is used
to prove Schur's lemmas in infinitedimensions.
24.7. Showthat elements of a group belonging to the same conjugacyclass have
the same characters.
24.8. Show that the regular representation is indeed a representation, i.e., that
R:
G
-+
GL(m,
IR)
is a homomorphism.
24.9. Showthat a left ideal is minimalif and only
if
it is generatedby a primitive
idempotent.
24.10. Let G be a finite group. Define the element
P = LXEGx of the group
algebra and showthat the left ideal generated by
P is one-dimensional.
724
24.
GROUP
REPRESENTATION
THEORY
000
DD+B
0000
000+
B
D
000
DO
DODD
+
DOD
+
DO
0
DO
000
B
DOD
+
DO
0
0
0
0
DO
00
0
0
+
0
+
DO
o 0
0
0
DO
0
Figure24.2 Someproducts of
Young
frames
for smallvalues
ofn.
24.11. Show that
Ti·)
defined in Equation (24.25) commutes with all operators
T~·).
Hint: Consider
T~·)Ti·)
(T~·»)-l.
24.12.
Let
Ki'
denote the set of inverses
of
a conjugacyclass K, with Ci elements.
(a)
Show
that K
i
,
is also a class with
Ci
elements.
(b) Show that identity occurs exactly Ci times in the product
KiKi',
and none in the
product
KiK
j if j
oF
if [see Equation (24.23)].
(c) Conclude that
{
o
if
j
oF
if,
c·· -
lJl
-
"f..f
Ci 1
J=Z.
24.13. Show that the coefficients IGldj IIHlCi of Equation (24.35) are integers.
24.14. Show that the symmetric and the antisymmetric representations of 8
n
are
inequivalent.
24.15. Construct the character table for 84 from that of 83 (given as Table 24.4),
and verify that
it
is given by Table 24.8.
24.16. Show that all functions transforming according to a given row of an
itre-
ducible representation have the same norm.
24.17. Show that if the group
of
symmetry of V contains that
of
Ho
and
ItI>;·»)
belongs to the
jth
column
of
the ",th irreducible representation, then so does
V
ItI>;·»).
Conclude that
(tI>;.)
IV
ItI>;·»)
= 0 for i
oF
j.
24.18. Find the irreducible components of the representation
of
Example 24.1.6.

24.9
PROBLEMS
725
I
Kl
6Kz
3K3 8K4
6Ks
T(l)
1 1 1
1
1
T(Z)
1
-1
1
1
-1
T(3)
2 0 2
-1
0
T(4)
3 1
-1
0
-1
T(S)
3
-1
-1
0 1
Table 24.8
Character
table
for
84.
24.19. Showthat p(3)
11fr3}
of Example24.6.3is alinearcombinationof p(3)
11fr1)
and p(3)
l1frz).
24.20. Showthat the tensorproductof twounitary representations is unitary.
24.21. Switchthe dummyindicesofthedoublesumin (24.45),add(subtract)the
two sums, and use
(T
®
T)ia.jb(g)
=
(T
®
T)ai.bj(g)
to showthat the double
sumcanbe writtenas a sum overthe
symmetric (antisymmetric) vectorsalone.
24.22. Show that the characters XS(g) and
XA(g)
of the symmetrizedand anti-
symmetrized productrepresentationsare giveu,respectively, by
and
24.23. Supposethat
Ai
a
)
transformsaccordingto
T(a)
, andA
7)
accordingto
T(P)
.
Showthat
At)
A7)
transformsaccordingto
T(axp).
24.24. Showthat
Onecaninterpretthisasthestatementthatthesquareofthereducedmatrixelement
isproportionaltotheaverage(over
i and
j)
ofthesquareofthefullmatrixelements.
24.25. Constructthecharactertableof84usingtheanalyticalmethodandEquation
(24.57).
24.26. Findallthestandard
Young
tableauxfor8s.Thus,determinethedimension
of each irreduciblerepreseutations of 8s. Check that the dimensions satisfy the
secondequationof (24.18).
24.27.
Verify
theremainingentriesof Table24.6.
24.28. Constructthe charactertableof 8s.

726 24.
GROUP
REPRESENTATION
THEORY
24.29. Suppose that Q,an element of the group algebra
of
5
n
,
is given by
n1
Q = L
f
n j
1t
i l
i=1
Show that
and
Q
2 _ 'Q
-no
.
24.30. Show that
y(n)y(l")
=
O.
Hint: There are as many even permutations in
5
n
as there are odd permutations.
24.31. Show that that the product
of
any two Young operators of 53 corresponding
to different Young tableaux is zero and that
Y
(2,I)y (2.1) _
3y(2.1)
1 1 - 1 '
Y
(2,I)y(2,1) _ 3y(2,1)
2 2 - 2 .
24.32. Construct the ideal
.c~2,1)
generated by
y?,I)
and verify that it is two
dimensional.
24.33. Using the ideal.c\2,1) generated by
y?,I),
find the matrices of the irre-
ducible representation
T(2,1).
Fromthese matrices calculate the simple characters
of
53and compareyour resultwith Table 24.4. Show that the
ideal.c~2,
I) generated
by
yJ
2
,1) gives the same set
of
characters.
24.34.
Find all the Young operators for 54 corresponding to the first entry of
each row of Figure 24.1. Find the ideals .c\3,1) and .c\2
2
)
generated by the Young
operators
y?,
I) and y?2) corresponding to the secondand third rows
of
the table.
Show that .c\3,I) and .c\2
2
)
have 3 and 2 dimensions, respectively.
24.35. Verify the products of the Young frames of Figure 24.2.
Additional Reading
1, Barut, A.andRaczka, R.Theory
of
Group RepresentationsandApplications,
World Scientific, 1986. A comprehensive treatise on group theory and its
application to physics written in a formal,
but
readable, language.
2. Boerner, H.
Representation
of
Groups, North-Holland, 1963. A detailed
introduction to group representation using group algebra techniques.
3. Elliott,
J. and Dawber, P.Symmetry in Physics (2 volumes), Oxford Univer-
sity Press, 1979. Anotherinformal bookwritten for physicists.

24.9
PROBLEMS
727
4. Fulton,
W.
and Harris, J. Representation Theory, Springer-Verlag, 1991. A
formal introduction to representation theory designed for graduate students
in mathematics, but also usefulfor physicists with a solid math background.
5. Hamermesh, M.
Group Theory
and
its Application to Physical Problems,
Dover, 1989. A detailed and very readable account of the representation
of
syrmuetric groups written for physicists.

25 _
Algebra
of
Tensors
Einstein's
summation
convention
Up until a mere two decades ago, tensors were almost completely synonymous
with (general) relativity--except for a minor use in hydrodynamics. Students of
physics did not need to study tensors until they took a course in the general theory
of
relativity. Thenthey would read the introductory chapter on tensor algebra and
analysis, solve a few problems to condition themselves for index "gymnastics,"
read through the book, learn some basic facts about relativity, and finally abandon
it (unless they became relativists).
Today, with the advent of gauge theories
of
fundamental particles, the realiza-
tion that gauge fields are to be thoughtof as geometricalobjects, and the widespread
belief that all fundamental interactions (including gravity) are different manifes-
tations of the same superforce, the picture has changed drastically.
Two importantdevelopments have taken place as a consequence: Tensors have
crept into other interactions besides gravity (such as the weak and strong nuclear
interactions), and the geometrical (coordinate-independent)aspects of tensors have
becomemore and more significant in the study of all interactions. The coordinate-
independent study of tensors is the focus
of
the fascinating field
of
differential
geometry and Lie groups, the subject of the remainder of the book. This chapter
covers tensor algebra, while the next is devoted to tensor analysis.
As is customary, we will consider only real vector spaces and abandon the
Dirac bra and ket notation, whose implementationis mostadvantageous in unitary
(complex) spaces. From here on, the basis vectors!
of
a vector space V will be
distinguishedby a subscriptand those of its dualspaceby a superscript.
If
{ei
}~1
is
a basis in
V,then
{€j}f~!
is abasis in
V'.
Also,
Einstein's
summation
convention
will be used:
1Wedenotevectorsby
roman
boldface,and
tensors
of higher
rank:
by sansserifboldletters.

25.1 MULTILINEAR MAPPINGS 729
25.0.1.Box.
Repeated
indices,
of
which
one
is an upper
and
the other a
k . N
k'
lower index, are assumed to be summed over: a
j
bj
means Li=1 a
j
hj.
As a result
of
this convention, it more natural to label the elements
of
a matrix
representation
of
an operator A by
af
(rather than
ai')'
because then Ae, =
af
ei'
25.1 Multilinear Mappings
Sincetensors arespecialkinds
oflinear
operatorson vectorspaces,let us reconsider
t:..(V,
W),
the space
of
all linear mappings from the real vector space V to the real
vectorspace
W. We notedin Chapter3that
t:..
(V, W) is isomorphic to a space with
dimension dim V .
dimW. The following
proposition-whose
proof we leave to
the
reader-shows
this directly.
25.1.1.Proposition.
Let{etl~l
be a basis
for
V
and
(e~I:;"1
abasisforW.
Then
1. the linear transformations
T~
: V --> W in the
vector
space t:..(V,W),
defined by (note the
new
way
of
writing the Kronecker delta)
j = 1,
...
,
N1;
fJ
= 1,
...
, N2,
form
a basis in t:..(V,W). In particular, dim t:..(V,W) =
NIN2.
2.
If
r
J
are the matrixelements
of
a linear transformation T E
t:..
(V,
W),
then
T =
T:jT~.
The
dual space V*is simply the space t:..(V,
R),
Proposition25.1.1 (with N2 =
I)
then implies that dim V* = dim V, which was shown in Chapter 1.
The
dual
spaceis
important
inthediscussionoftensors, so we considersomeofits
properties
below.
The
basis
{T~I
of
Proposition 25.1.1 reduces to {T{I when W = lR and is
denoted by {eiI7=1' with
N = dimV* =
dimV.
The
ei's
have the property that
. i
e
1
(e,) = 8, . (25.1)
This relation was also established in Chapter
1.
The
basis B* =
{eilf~l
is simply
the dual
of
the basis B = {e,1;;"1'Note the "natural" position
of
the indices for B
and B*.
Now suppose that {f,I;;"1 =
B'
is another basis
of
V and R is the (invertible)
matrix carrying
B onto
B'.
Let B
'*
=
{'Pilf~l
be the dual
of
B
'.
We want to find
the matrix that carries
B* onto B'*.
If
we denote this matrix by Aand its elements
by
af
'we
have
,k
kf
(k
1)(
i)
k i ,I k 1 (
)k
OJ
=
ep
i = al e r
j
ej = al r
j
(J j = al r
j
= AR i '

730 25.
ALGEBRA
OF
TENSORS
where the first equality follows from the duality
of
B'
and
B'*. In matrix form,
this equation
can
be written as AR = 1, or A =
R-
I.
Thus,
25.1.2. Box.
The matrix that transforms bases
of
V* is the inverse
of
the
matrix that transforms the corresponding bases
ofV.
In the above equations the
upper
index io matrix elements labels rows,
and
the
lower iodex labels
columns. This
can
be remembered by noting that the column
vectors
ei
can be thought
of
as columns
of
a matrix, and the lower iodex i then
labels those columns. Similarly,
e
J
can be thought
of
as rows
of
a matrix. We now
generalize the concept
of
linearfunctionals.
multilinear
mappings
25.1.3. Definition. A map T : VI x V2 X
...
x V,
--->
W is called r-linear ifit is
defined
linear in all its variables:
ICVI,.",
aVj
+a'v~,
...
,v
r)
=
aT(vlo.'"
Vi,
...•
V
r
)
+a'T(Vl
....
,
v~,
...
,
v-)
for all i.
We can easily construct a bilioear map.
Let
71 E Vi
and
72
E
Vi.
We define
the map
71 ® 72 : VI x V2
--->
1&
by
(25.2)
The expression
71 ® 72 is called the
tensor
product
of
71 and
72.
Clearly, sioce
71 and
72
are separately lioear, so is 71 ®
72.
An
r-lioear
map
can be multiplied by a scalar, and two r-linear maps can be
added; io eachcasethe resultis an
r-lioear
map. Thus, the
set
of
r-lioear
maps from
VI x
...
x V, ioto W forms a vector space that is denoted by .G(VI,
...
,
V,;
W).
We
can
also constructmultilioearmaps on the dual space. First, we note thatwe
can
define a
naturallioear
functional on V* as follows. We let 7 E V* and v E V;
then 7(V) E
1&.
Now we twist this around
and
define a mappiog v : V*
--->
1&
givenby V(7) sa 7(V). II is easily shownthatthis mappiogis lioear. Thus, we have
naturally constructed a lioear functional on
V* by identifyiog (V*)*
with
V.
Construction
of
multilioearmapson V* is now trivial.
For
example,let VI E VI
and
V2
E V2
and
define the tensor product VI ®
V2
: Vi x
Vi
--->
1&
by
(25.3)
We can also constructmixed multilioearmaps such as v
® 7 : V* x V
--->
1&
given
by
v ®
7(0,
u) = V(O)7(U) = O(V)7(U). (25.4)

natural
pairing
of
vectors
and
their
duals
tensors;
covariant
and
contravariant
degrees
25.1
MULTILINEAR
MAPPINGS
731
There is a bilinearmap h :
V*
x V
-+
lR
that naturallypairs V and
V*;
it is
givenby h(O,
v)
'"
o(v).Thismappingis calledthe natural pairing of Vand
V*
into
lR
andis denotedby using anglebrackets:
h(O,v)
'"
(0,v) es O(v).
25.1.4.Definition.
Let Vbe a vectorspace with dual space
V*.
Then a tensor
of
type (r, s) is a multilinear mapping
2
T
r
:
V*
x
V*
x
...
X
V*
x V x V x
...
x V
-+
R
s ,
I,
, '
r times
s times
contravariant
and
covariant
vectors
and
tensors
tensors
form
an
algebra
multipiication
of
the
algebra
of
tensors
The set
of
all such mappings
for
fixed
rand
s forms a vector space denoted by
Y,(V). The number r is called the contravariant degree
of
the tensor, and s is
called the covariantdegree
of
the tensor.
As anexample,let
VI,
...
, V
r
E Vand
T
I,
...
, 1"8 E
'\7*,
anddefinethe tensor
prodnct
tj' es VI ®
...
® V
r
® TI ® ®
T'
by
VI ®
...
® V
r
® TI ®
...
®
T'
(0
1
, ,
0'
, UI,
...
, Us)
r s
=VI(0
1
)
...
VT(Or)T
I
(UI)·
..
T'
(u,)
=nn0' (Vi)T
j
(uj),
i=l
j=l
Each v in the tensorproduct requires an element of
V*;
that is why the number
of factors of
V*
in the Cartesian product equals the number of v's in the tensor
product.As explainedin Chapter0, the Cartesian product with
s factors of Vis
denotedby
V' (similarlyfor
V*).
A tensor of type (0,0) is definedto be a
scalar,
so
'J1!(V)
= R A tensorof
type (1,0), an ordinaryvector,is calleda contravariant vector, and one of
type
(0, 1), a dualvector(oralinearfunctional),is called a covariantvector. A tensor
of type
(r, 0) is called a contravarianttensor of rank r, and one of type (0, s) is
called a covariant tensor of rank s. The union of
Y,(V)for all possibler ands
canbe madeinto an(infirtite-diroensional) algebra,calledthealgebraof tensors,
by defining the following product onit:
25.1.5.Definition.
The tensorproduct
of
a tensorT
of
type (r, s) and a tensor U
of
type (k,
1)
is a tensor T ® U
of
type (r +k, s +
1),
defined, as an operator on
(V*yH x
V,+I,
by
T ® U(OI, , OTH, UI, , U,+I)
= T(OI, ,
0',
UI, , u,)U(OT+I,
...
,
r»,
U,+1,
...
,U,+I).
This product turns the (infinite-dimensional) vector space
of
all tensors into an
associative algebra calleda tensoralgebra.
2Justas the space
'\1*
of linearfunctionals of a vectorspaceVis isomorphicto V,so is the spaceof tensorsof type (T,s) to
thetensorproductspace
Vr,s (seeExample1.3.19).In fact,
~
(V) = Vi,s. as shownin
[Warner,
83] on p. 58.

732 25.
ALGEBRA
OF
TENSORS
This definition is a generalization
of
Equations (25.2), (25.3), and (25.4).
It
is
easily verified that the tensor product is associative and distributive (over tensor
addition),
but not commutative.
Making computations with tensors reqnires choosing a basis for V and one for
V'
and
representing the tensors in terms
of
numbers (components).
This
process
is not,of
course,
new.
Linear
operators
are
represented
by
arrays
of
nwnbers,
i.e.,
matrices.
The
case
of
tensors is merely a generalization
of
that
of
linearoperators
and
can
be stated asfollows:
25.1.6.
Theorem.
Let {eilf'::l be a basis in V, and
(.ijJ=l
a basis in
V',
usually
taken to be the dual of{eilf=l' Then the set
of
all tensor products eil @
...
@ei, @
.h
@
...
@.i,
forms abasis
for
T; (\1). Furthermore, the components
of
any tensor
A E
T;(V)
are
A
h
...
i
,
A(
hi,·
)
i " =
€,
...
,€
,eil"'"
ei
s
.
l ... s
Proof
The
proof
is a simpleexercisein employingthe definition
of
tensorproducts
and keeping track
of
the multilinear property
of
tensors. Details are left for the
reoo~
D
A useful result
of
the theorem is the relation
(25.5)
'11
(V),I:(V), and
qv')
areallthe
same
Note
thatfor everyfactorin the basis vectors
ofT;
(V)
thereare N possibilities,
each
one
giving a new linearly independent vector
of
the basis. Thus, the number
of
possible tensor products is N
r
+" and we have
dim
T;
(V) = (dim
VY+'
.
25.1.7.
Example.
Let us consider the special case of
'11
(V) as an illnstration. We can
writeA E
'J'}
(\7)asA=
A~ei
® e
j
. Givenanyv E V,we
obtain
2
A(v)=
(A~ei
0 ·.i)(v) =
A~ei
r.
i
(v)].
'-v-'
ER
ThisshowsthatA(v) E V andAcanbe interpretedasa linear operatoronV,i.e.,A E
qV).
Similarly,
forT E
\7*
we get
A(r)
=
(A~ei
0e
i)(r)
=
A~
[ei(r)]e
i.
'-v-'
ER
Thus,A EL(V*).Wehaveshown
that
givenA E
'JJ
(V),
there
corresponds
a
linear
operator
belongingtoI:(V) [or
qv')
1havinganatnralretationto A.Similarty,givenanyAE
I:(V)
[or
I:(V")]
with a matrixrepresentationin the basis {eilf'::l of V(or{e
i
lJ=l
of V*)given
by
A~,
then
corresponding
to it in a
natural
way is atensorin
'JfcV),
namely
A~ei
® e
i
.
Problem
25.5 shows
that
the
tensor
defined
in this way is
basis-independent.
Therefore,
