Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

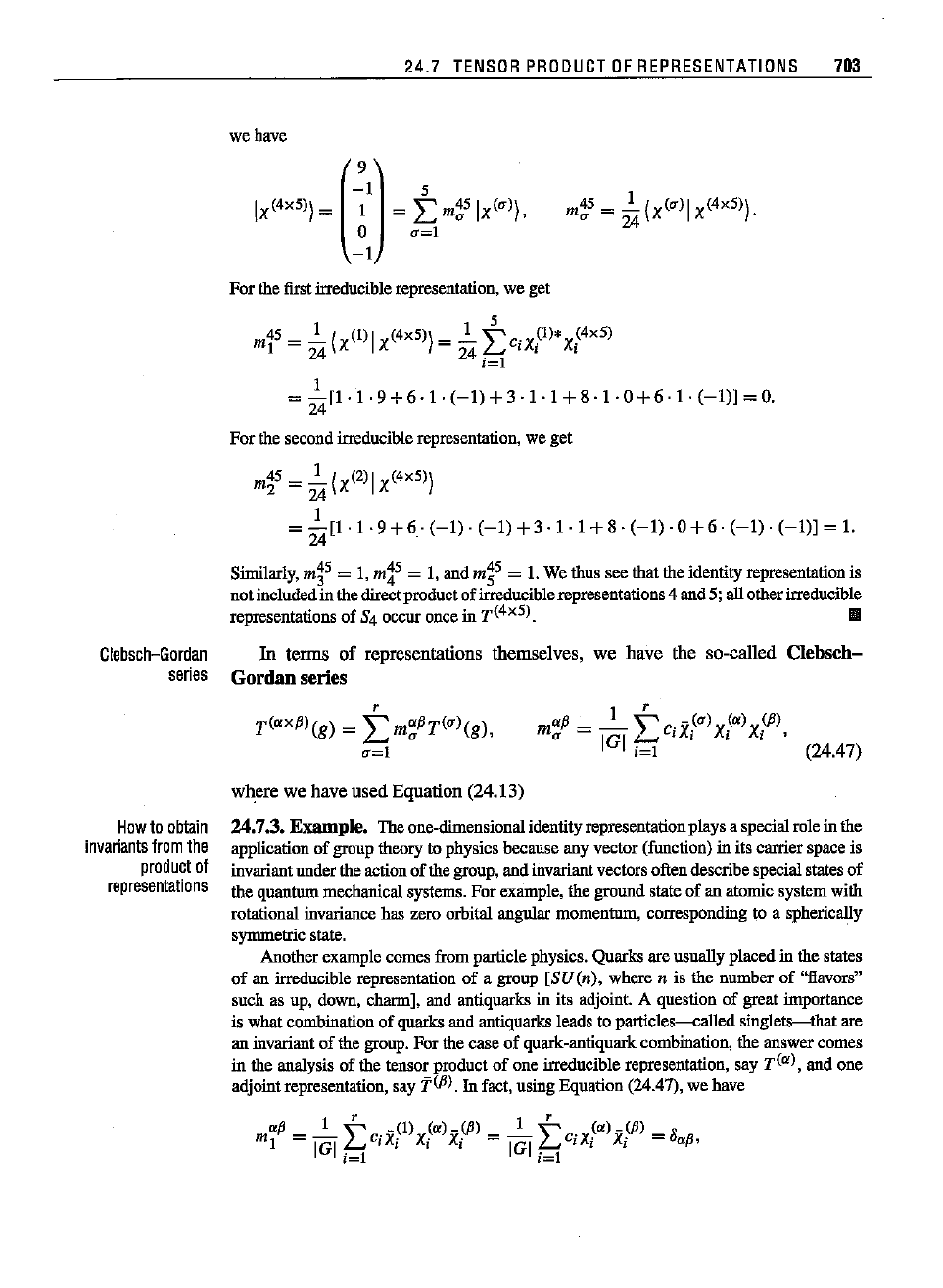
24.7
TENSOR
PRODUCT
OF
REPRESENTATIONS
703
wehave
Clebsch-Gordan
series
Forthe
first
irreducible
representation,
we get
5
45 _
-.!.-
( (1)I (4X5») _
-.!.-"
. (1)*
(4x5)
ml
- 24 X X -
24~CIXi
Xi
1=1
1
=
24
[1·1
·9
+
6·1
.
(-1)
+
3·1·1
+
8·1
·0
+
6·1
.
(-1)]
=
O.
Forthesecond
irreducible
representation,
we get
m~5
=
~
(x(2)1
X(4X5»)
1
= - [1. 1
·9
+
6·
(-1)
.
(-1)
+3 . 1 . 1+8 .
(-1)
.0 +6 .
(-1)
.
(-1)]
=1.
24
Similarly,
mj5 = 1,
m1
5
= I, and
m~5
= 1.Wethussee thatthe
identity
representation
is
not
included
inthe
direct
product
of
irreducible
representations
4 and5;all
other
irreducible
representations
of 84 occuroncein
T(4x5).
II
In terms of representations themselves, we have the so-called Clebsch-
Gordan series
r
T(ax~)(g)
= L
m~~T(U\g),
cr=l
How
to
obtain
invariants
from
the
product
of
representations
where we have used Equation (24.13)
24.7.3. Example. The
one-dimensional
identity
representation
playsa
special
roleinthe
application
of
group
theory
tophysicsbecauseanyvector
(function)
in its
carrier
spaceis
invariant
under
theactionof the
group,
and
invariant
vectors
often
describe
special
states
of
the
quantum
mechanical
systems.For
example,
the
ground
state
of anatomicsystemwith
rotational
invariance
has zero
orbital
angular
momentum,
corresponding
to a spherically
symmetric
state.
Another
example
comesfrom
particle
physics.
Quarks
areusuallyplacedin the
states
of an
irreducible
representation
of a
group
[SU(n), wheren is the
number
of
"flavors"
suchas up,
down,
charm],
and
antiquarks
in its
adjoint.
A
question
of
great
importance
is what
combination
of
quarks
and
antiquarks
leadstoparticles---called singlets-that
are
an
invariant
of the
group.
Forthecaseof quark-antiquark
combination,
the
answer
comes
in theanalysis of the
tensor
product
of one
irreducible
representation,
say
r(a),
andone
adjointrepresentation, say
t(fJ).
In fact,usingEquatiou(24.47), wehave
1 r 1 r
ma~
=
_"
c'x-(l)X(a)X-(~)
=
-"
c'X(a)X-(~)
=
~
Q
1
IGI
~
I l I I
IGI
~
l I
lap'
l=l
1=1

704 24.
GROUP
REPRESENTATION
THEORY
whereweusedEquatiou(24.13)andthefactthatallcharacters oftheidentityrepresentation
are
unity.
Thusto
construct
an
invariant
state,
we needto combinea
representation
withits
adjoint,
in
which
casewe
obtain
the
identity
representation
onlyonce. II
Paul Albert Gordan (1837-1912), the sonof David
Gordan,
a
merchant,
attended
gymnasium
and
business
school,
then
workedfor severalyearsin
banks.
His earlyinterestin
math-
ematics
was
encouraged
by the
private
tutoring
he
received
fromaprofessorattheFriedrich
Wilhelm
Gymnasium.
He at-
tended
Ernst
Kummer's
lecturesin
number
theoryatthe
Univer-
sityof Berlinin 1855,then studiedat theuniversities of Bres-
lau,Konigsberg, andBerlin.AtKonigsberg he cameunderthe
influenceof
Karl
Jacobi's
school,andatBerlinhisinterestin al-
gebraicequationswasaroused. Hisdissertation (1862), which
concernedgeodesics onspheroids, receiveda
prizeofferedbythephilosophy facultyofthe
Universityof Breslau. Thetechniques that
Gordan
employedin it werethose of
Lagrange
and
Jacobi.
Gordan's
interestin functiontheory led him to visit G.
F.
B.
Riemann
in
GOttingen
in 1862, but
Riemann
was ailing, and their association was brief. The following
year,
Gordan
was invitedto Giessenby Clebsch, thusbeginningthe
fruitful
collaboration most
physicists recognize.
Together
they
produced
work on the theory
of
Abelian functions,
basedon Riemann's
fundamental
paperon thattopic, andseveralof Clebsch's
papers
are
considered
important
steps
toward
establishing for Riemann's theoriesa finn foundation
in tenus of pure algebraic
geometry.
Of conrse, the Clebsch-Gordancollaboration also
produced
thefamouscoefficientsthatbeartheirnames, so indispensable to the theoryof
angularmomentomcoupling foundinahnusteveryareaofmodemphysics.In 1874Gurdan
becameaprofessorat
Erlangen,
whereheremained untilhis
retirement
in 1910.He
married
Sophie
Deuer,
thedanghterofa GiessenprofessorofRomanlaw,in 1869.In 1868Clebsch
introduced
Gordan
tothetheoryof
invariants,
whichoriginated inanobservation of
George
Boole's
in 1841andwasfortherdevelopedby Arthnr
Cayley
in1846.Following theworkof
thesetwoEnglishmen, aGermanbranchofthetheorywasdeveloped by S.
H.
Aronhold
and
Clebsch, the latter elaborating the former's symbolicmethodsof characterizing algebraic
forms andtheir
invariants.
Invariant
theorywas
Gordan's
main
interestfortherestof his
mathematical
career;
hebecameknownasthegreatestexpertinthefield,developingmany
techniques for
representing
and
generating
formsandtheir
invariants.
Gordan
made
important
contributions
to algebraandsolutionsof algebraic equations,
andgave simplifiedproofsof thetranscendence of
e andx .The overallstyle of
Gordan's
mathematical
workwas algorithmic. He shiedawayfrompresenting his ideasin
informal
literary
forms.
He
derived
hisresultscompntationally, workingdirectlytowardthedesired
goalwithoutofferingexplanations of theconceptsthatmotivated his work.
Gordan's
only
doctoral
student,
Emmy
Noether,
was one of the firstwomento receive
adoctorate
in
Germany.
She
carried
on his workin
invariant
theoryfora while, but
under
thestimulus of
Hilbert's
school at
GOttingen
herinterests shiftedandshebecameoneof the
primary
contributors
tomodem
algebra.

24.7
TENSOR
PROOUCT
OF
REPRESENTATIONS
705
So far, we have concentrated on the reduction
of
the operators and carrier
spaces into irreducihle components.
Let
us now direct our attention to the vec-
tors themselves. Given two irreducible representations
T{a)
and
T{P)
with carrier
spaces spannedby vectors
{14>!a»))~:l
and
(1",jP»))~:l'
we form the directproduct
representation
T{a
xP)
with the carrierspace spanned by vectors
(14)!a)
'"
jP»)).
We
know that
T{axp)
is reducible, and Equation
C24.47)
tells us how many times each
irreducible factor occurs in
T{axp).
This means that the span
of
(14)!a),,,jP)))
can
be decomposedintoinvariantirreduciblesubspaces; i.e., theremustexista basis
of
the carrier
of
the productspace the vectors
of
whichbelongto irreduciblerepresen-
Clebsch-Gordan
tations
of
G. More specifically, we shouldbe able to form the linear combinations
coefficients
I"'t),q)
= L CCap; a, q
lij;
k) I
4>!a)",jP»)
,
ij
C24.48)
which transform according to the rows
of
the c thirreducible representation. Here
the subscript
k refers to the row
of
the crth representation, and q distinguishes
among functions that have the same
o and k, corresponding to the case where
m':!
:::
2. For simply reducible groups, the label q is unnecessary.
The
coefficients
CCap; a, qlij; k) are called the
Clebsch-Gordan
coefficientsfor G. Thesecoef-
ficients are normalized such that
L C*Cap; a,qlij; k)CCap; a', q'lij; k
')
= 8
u
u,8
q
q,8kk"
ij
LC*Cap; a, qlij; k)CCap; a, qli't'.k) =
8w8jj'.
aqk
This will guarantee that
I"'ku),q)
are orthonormal
if
the product vectors form an
orthonormalset. Usingtheserelations,we can write the inverse
of
Equation
C24.48)
as
14>!a)",jP»)
= L C*Cap; a, qlij; k) I
"'ku),q}
.
oqk
24.7.2 IrreducibleTensorOperators
C24.49)
irreducible
setof
operators
An operator A acting in the carrier space
of
the representation
of
a group G is
transformedinto anotheroperator, A
f--* T
gAT;
1
,by the action
of
the group. Just as
in the case
of
vectorspaces, one can thus construct a set
of
operators thattransform
among themselves by such action and lump these operators in irreducible sets.
24.7.4.Definition. An operator
A!a)
is said to to transform according to the
ith
row
of
the
ath
irreducible representation if there exist n
a
-
lather
operators

706 24.
GROUP
REPRESENTATION
THEORY
(24.50)
where
(T~'\g))
is the matrix representation
of
g. The set
of
such operators is
called anJirreducible set
of
operators (orirreducible tensorial set).
scalar
operator
In
particular, if
T,J'\g)
= 8ij, i.e.,
if
the representation is the identity repre-
sentation, then
A=
TgAT;I,
and Ais called a scalar
operator.
The term "scalar"
refers to the fact that
Ahas ouly one "component," in contrastto the otheroperators
of Equation (24.50), which may possess several components.
Consider the set
of
vectors (functious) defined by IVrij}
==
Ai')
l<I>j~)),
where
I<I>(/l))
transform according to the ,Bthirreducible represeutation. These vectors
J di
transform accor ng to
n
u
np
T
g
IVrij)
=
TgAi')T;I
Tg
1<1>1))
=
LJk\'\g}Ak·)
L
Tz1\g}
I<I>?))
k~1
1~1
= L
Tk\')(g)Tz1)(g)Ak')
I<I>?))
=
LTk\:rf!(g)
I
Vrkl)
,
U U
~.5D
i.e., according to the representation
T('X~).
This means that the vectors IVrij)
have the same transformation properties as the tensor product vectors
l<I>i')
Vr
f)).
Therefore, usiug Equation (24.49), we can write
Ai')
l<I>j~))
= L C*(a,B;
rr,
qlij; k)
Iw~D'),q),
aqk
and more importantly,
(<1>,\1')1
Ai')
I<I>(~))
= LC*(a,B; a, qlij; k)
(<1>,\1')1
wt),q)
J "
oqk
'
use
Eq.(24.38) here
= LC*(a,B; y, qlij; m)
(<1>,\1')
1
w,\1'),q).
q
(24.52)
Wigner-Eckart
theorem
and
reduced
matrix
elements
It
follows that the matrix element of the operator
Ai')
will vanish uuless tJie
irreducible representation
T(Y)
occurs in the reduction of the teusor product
T(')
®
T(~),
and this can be decided from the character tables and the Clebsch-
Gordan series, Equation (24.47).
There is another remarkable property of Equation (24,52) that has significant
physical consequences. Notice how the dependence ou
i and j is contained en-
tirely
in the Clebsch-Gordan coefficients. From Equation (24.39) it follows that

24.8
REPRESENTATIONS
OF
THE
SYMMETRIC
GROUP
707
(q,;,;)
I
>It;';).q)
is independent of m. Therefore, this dependence must also be con-
tained entirely in Clebsch-Gordan coefficients. One therefore writes
(24.52) as
reduced matrix element
(24.53)
(24.54)
This equation is known as the
Wigner-Eckart
theorem,
and the numbers mul-
tiplying the Clebsch-Gordan coefficients are known as the
reduced
matrix
ele-
ments.
From the point of view of physics, Equation
(24.53) can be very useful in
calculating matrix elements (expectation values and transition between states),
once we know the transformation properties
of
the physical operator.
For
example,
for a
scalar
operator
S, which, by definition, transforms according to the identity
representation,
(24.53) becomes
i.e., scalar operators have no matrix elements between different irreducible repre-
sentations of a group, and within an irreducible representation, they are multiples
of
the identity matrix. This result is also a consequence
of
Schur's lemma.
24.8 Representations of the Symmetric Group
The symmetric (permutation) group is an important prototype
of
finite groups. In
fact,
Cayley's
theorem
(see [Rotm 84, p. 46] for a proof) states that any finite
group of order
n is isomorphic to a subgroup
of
Sn. Moreover, the representation
of
Sn leads directly to the representation
of
many of the Lie groups encountered
in physical applications.
It
is, therefore worthwhile to devote some time to the
analysis of the representations
of
Sn.
24.8.1 Analytic Construction
The starting point
of
the construction
of
representations of the symmetric group is
Equation
(24.35), which is valid for any finite group. Thereis one simple character
that every group has, namely, the character
of
the one-dimensional symmetric
representation in which
all elements of the group are mapped to 1 E R. Setting
~y)
= I in (24.35), and noting that
Lj
dj = ds,we obtain
1{rH
=
IGldi
, - IHlci'
where
{1{ri
H}
are the components
of
a compound character
of
G.
Frobeniushas shown that by a clever choice
of
H,
one
can
completelysolve the
problemof the construction
of
the irreduciblerepresentations of Sn. He proceeded

708 24.
GROUP
REPRESENTATION
THEORY
as follows. Consider a partition (A) = (AI
•...•
An)
ofn.
The
symmetric groups
IS).,}
are subgroups
of
Sn and have no elements in
common-therefore
they all
commute with one another.
The
direct product
of
these subgroups is a subgroup
of
Sn.which we denote by
S().):
S().)
=
S).,
x···
X
S).".
If
we denote thecompound character
of
Equation (24.54) by
11/J().))
in this case.
calculate
IHI.
Ci. and
di.
and substitute the results in (24.54}--all
of
which can be
done in closed
form-we
obtainan explicit formula for the components
of
11/J().)).
Tbis formula is messy. and we shall not derive it here. The interested reader may
refer to [Harne 89. pp. 189-192] for details.
We are really interested in the simple characters
of
Sn. and Frobenius carne
up with a powerful method
of
calculating them. Since there is a one-to-one cor-
respondence between the irreducible representations and conjugacy classes. and
another one betweenconjugacy classes
of
Sn and partitions
of
n. we shall label the
simple characters of
Snby partitions
of
n. Thus. instead
of
our common notation
xi
a
).
we use
x&~).
where (A) denotes a partition
of
n, and (I) a cycle structure
of
s;
Suppose we want to find the irreducible characters corresponding to the cycle
structure
(I) =
(I
a•2
P•
3
Y
• • • •
).
These form a column under the class (I) in a
character table. To calculate the irreducible characters. form two polynomials in
(XI. X2• . . . •x
n)
as follows.
The
first one. which is completely symmetric in all
variables,
is
.
(n
)a
(n
)P
(n
) Y
8(1)
==
?=Xi
?=Xf
?=xi
l=l
1=1 1=1
The
second one is completely antisymmetric, and can be written as
(24.55)
D(Xl
.....
x
n
)
sa det
I I I
=IT(Xi-Xj)
i<j
(24.56)
It
can be shown that the simple characters
of
Sn are coefficients
of
certain terms
of
the product
of
these polynomials. To be exact. we have
(24.57)

24.8
REPRESENTATIONS
OF
THE
SYMMETRIC
GROUP
709
The
outer
sum
goes
over
all
partitions
of
n,
the
inner
sum
over
all
permutations
of
Sn.
The
procedure
for
finding
the
simple
characters
of
Sn
should
now
be
clear
from
(24.57):
24.8.1.Box. To find the simple character
xg~iPA~\,
construct the corre-
spondingsymmetric
and
antisymmetricpolynomials. multiplythem together,
collectall terms
of
the
form
Al+n-l
A2+n-2
A
n_l+l
An
X,,(I)
X,,(2)
•••
X,,(n_l)
x,,(n)
for
all permutations
11:
E Sn; the coefficient
of
such a term will be the desired
character.
S
·
th
ffi . f A1+
n-
1
A2+
n-
2
A
n_1
+1
An < .
'di""
mce e coe cients 0 x
1r
(l) x
rr
(2)
...
xrr(n_l)x
1r(n)
ror
VarIOUS
n
suer
by a sign, in
practice,
thecoefficient of just
x~l+n-l
...
X;~11+1x;n
is
precisely
the
simple
character
we
are
looking
for.
24.8.2.Example. The best way to understaod the procedure described above is to go
through
an
example
in
detail.
We
calculate
the
characters
of 83 usingthe
above
method.
Label the rows of the character table with the partitions of 3. These are (3),
(2,1),
and
(1,1,
I). Similarly, label the colwnns with the conjugacy classes, or cycle structures: (1
3
),
(1,2),
and (3). The first cyclestructure has a =3,
{3
=0 =y. Therefore,
_ ( )3 _ 3 3 3
S(l3)
- XI
+X2+
x3
-XI
+x2
+x3
+
3(xrX2
+XrX3 +XI
X~
+
X~X3
+XI
X~
+
X2X~)
+
6xj
X2X3
(24.58)
and
so
that
(24.59)
Now we oote that for (A)
= (3), AI = 3, A2 = 0, and
A3
=
O.
Therefore, the
ffi
. f
A1+
n
-
1
A2+n-2
An 5 . (3)
Simi!'
I •
(')
(2 1 0)
coe ciento Xl X
2
"'X
n
=X1X2gIVeSX(l3)'
any.ror A = ,
..
Al = 2,
).,2
= 1, and).3 = 0, and the coefficient
of
x~1+n-lx~2+n-2
...
x;n =
xtxi
gives
X&i;).
Finally, for (A) = (1, I, I), Al = A2 =
A3
= 1, and the coefficient of
A1+
n-1
A2+
n-
2
An 3 2 .
(1,1.1)
Th ffi . b d
ff
Xl X
2
...
X
n
=Xl X
2X3
grves X(!3) . ese coe cients can e rea 0 by
scanning through Equation (24.58) while multiplyingits terms by those
of
Equation(24.59)
and
keeping
track
of the coefficients of the
products
of the
relevant
powers
of
xj
, x2. and
x3. The
reader
may
verify
that
there
isonlyone
term
ofthe
form.
x~
X2. whosecoefficient is

710
24.
GROUP
REPRESENTATION
THEORY
(1
3
)
(1,2)
(3)
T(3)
T(2.1)
T(I,I,I)
1 1 1
2 0
-1
1
-1
1
Table 24.5 Thecharacter table for 83. Each column corresponds to a coojugacy class,
eachrowtoa
partition
of 3.Thelasttwo
rows
havebeen
switched
compared
to
Table
24.4.
1, giving
xgJ)
= 1;
there
aretwo
terms
of
the
form
xixi.
whosecoefficients are
-1
and
3, giving
Xg3~)
= 2;
and
there
are
four
terms
of the
form
XiX~X3,
whosecoefficients
are
+1,
-3,-3,
and
+6.
giving
xg;~,I)
= 1.Therefore, the firstcolumn of the character table
of 83is
(i).
To
obtain
thesecondcolumn,weconsider thesecondconjugacyclass,
0,2),
with a = I =
fJ
and y =
O.
The corresponding symmetric polynomial is
8(1,2) = (XI + x2 + X3)(X[ +
x~
+
xl)
333222222
= Xl
+x2
+x
3
+X
1X2
+xIX3
+Xlx2
+x2x3
+Xlx3
+X2
x3'
(24.60)
V(XI,
x2, X3) is the sarne as before. Multiplying and keeping track of the coefficients of
5 4 2 3 2 . (3) (2,1) (1,1.1)
XI x2, XI x
2'
and Xl x
2x3,
we obtam X(l,2) = I,
X(l,2)
=0, and X(l,2) =
-1.
It
follows
that
thesecondcolumnof the
character
tableof 83is
(~1)'
The last column is obtained similarly. We note that a =0 =
fJ,
and y = 1.Therefore,
the synnnetric polynomial is
333
8(3) =XI
+x2
+x3'
and
the
antisymmetric
polynomial
isthesameas
before.
Multiplying
thesetwo
polynomials
d
.
th ffici t b & (3) I (2,1) I d (1,1,1) 1
an extracting e coe
Cleo
s as erore, we get X(3) =
,X(3)
= -
,an
X(3)
=.
It
follows that the third column of the character table of 83 is (
~
I
).
Collecting allthe
data
obtained
above,wecan
reconstruct
the
character
tableof83.This
is shown
in
Table
24.5. The
irreducible
representations
arelabeledby the
three
possible
partitions
of 3, andtheconjugacy classesby the
three
cycle
structures.
II
24.8.2 GraphicalConstruction
The
analytic
construction
of
the
previous
subsection
can
be
handled
using
graphical
techniques
that
are
considerably
simpler. To
begin
with,
let
us find
the
character
of
the
identity
element
of
Sn.
The
cycle
structure
is
(In),
i.e.,
all
cycles
consist
of
a
single
element.
Thus,
" = n,
and
{J,
y,
etc.
are
all zero.
It
follows
that
the
LHS
of
Equation
(24.57)
is
(Lxi)n
D(xj).
We
calculate
this
product
one
power
of
2>i

24.8
REPRESENTATIONS
OF
THE
SYMMETRIC
GROUP
711
at a time.
For
the
same
reasonas in
the
example
above,
xg2)
will be the coefficient
of
Ferdinand Georg FrobeDins (1849-1917), the son of a par-
son,was
born
in
Berlin
andbeganhis
mathematical
studies
at
G5tti.ngenin
1867.Hereceivedhis
doctorate
inBerlin
three
years
later.
Four
years
later,
onthebasisof his
mathematical
research,
hewas
appointed
assistant
professor
atthe
University
of
Berlin.
He
achieved
the
rank
of full
professor
at the Eidgeniissische
Polytechnikum
Ziirichbefore
returning
to
Berlin
as a
professor
of
mathematics
in
1892.
During
theearly
years
of
Frobenius's
career,
modem
group
theory
was in its
infancy.
He
combined
its three main branchesof
study-the
theory of solutions to al-
gebraic
equations
(permutation
groups
and
theworkof
Galois),
geometry
(transformation
and
Lie
groups),
and
number
theory-to
produce
the
concept
of
the
abstract
group.
He
collaborated
with
Issai
Schur
in
representation
and
character
theory
of groups.
His
paper
Uberdie Gruppencharactere is of
fundamental
importance.
It was
presented
to the BerlinAcademyon 16July 1896andit contaiusworkthatFrobeuiushad douein the
preceding
few
months.
Inaseriesof
letters
to
Dedekind,
the
first
on12April 1896. hisideas
on
group
characters
quickly
develop,
and
Frobenius
is ableto
construct
a
complete
setof
representationsby complexuumbers.
In a letter to Dedekindon 26 Apri11896 Frobeuius
finds the irreduciblecharactersfor the alternatinggroup, and the symmetricgroups.
Iu 1897Frobeuiusreformulatedtheworkof Molien-theLatvianstudeutofKlein,who,
inhis
thesis,
classifiedthesemisimple
algebras
usingthe
method
of
group
rings-in
terms
of
matrices
and
then
showed
thathis
characters
arethe
traces
of
the
irreduciblerepresentations.
Frobenius's
character
theory
found
important
applications
in
quantum
mechanics
and
was
used with great effect by
Burnside,
who wrote it up iu the 1911 edition of his Theory
of
GroupsofFiniteOrder.
Frobenius
is also
remembered
asthe
originator
of a series
method
forsolving
ordinary
differential
equations.
Despitetheclearly
greater
importance
of hisworkin
group
theory,
thismethodof
Frobenius
serves
admirably
to
perpetuate
his
name.
If
we multiply
D(x
j)
by 2:Xi one X at a time, we increase the
power
of
one
of
the
Xi'S
by one.
If
at any stage, two
of
the
exponents
become
equal, the
term
must vanish, due to the antisymmetry
of
(2:
Xi
)D(x
j)'
Therefore, as we raise
the
degree
of
the polynomial by
one
at each stage, the
power
of
Xl must be raised at
leastas fast as
X2,
and
the
power
of
X2 must be raised at leastas fast as
X3,
etc.
Our
goal is to raise the
power
of
Xl
by AI,that
of
X2 by
A2,
and, in general, the
power
of
Xi by
Ai,
making surethat at
each
stage, the
number
of
multiplications by Xl is
greater thanor equalto
the
number
of
multiplications by
X2,
etc. The total
number

712 24.
GROUP
REPRESENTATION THEORY
ofwaysby whichwe canreach this goalwill be
xg2),
whichis alsothe dimension
of the irreducible representation
(A)
by Equation (24.9).
Tosee the argumentmore clearly,supposethatwe areinterestedin the dimen-
sion of the irreduciblerepresentationof S4correspondingto (3, I). Then we must
raise the power of
Xl
by 3 and the powerof X2 by I; X3 and X4 will remain intact,
and therefore will not enter in the following discussion.
It
follows that
D(x
j)
is
to be multipliedby
xi
X2, one x-factor at a time, the number of Xl-factorsalways
exceedingthe number of x2-factors.The possible ways of doing this are
xix2'
XrX2XI, XIX2Xr.
(24.61)
Note that as we count the factors from left to right, the number of
XI'S
is always
greater than or equal to the number of
X2
'so
Thus
x2xi
is absent because X2 oc-
curs without
Xl
occurring first.
It
follows that the dimension of the irreducible
representation (3, I) is 3.
A graphical way to arrive at the same result is to draw AI = 3 boxes on top
and
A2
= I box belowit:
DOD
o
The next stepis to fillin the boxes withnumbers correspondingto the position of
Xl (fillingup the firstrow) and
X2 factors (fillingup the second row) in Equation
(24.61). Since in the first term of (24.61), the
XI'S
occupy the first, second, and
third positions, we enter I, 2, and 3 in the first row, and 4 in the second row
correspondingto thelast position occupiedby
X2. Similarly,in the secondterm of
(24.61), the
XI'S
occupythe first, second, and fourth positions;therefore,weenter
I,
2, and 4 in the firstrow,and 3 in the second row correspondingto the position
occupiedby
X2. Finally,in the last termof (24.61), the
XI'S
occupy the first,third,
and fourth positions; therefore, we enter I, 3, and 4 in the first row, and
2 in the
second row correspondingto the position occupied by
X2. The result is the graph
shownbelow:
Young
frame
defined
24.8.3. Definition. Let
(A)
= (AI,
A2,
...
, An) be a partition
of
n. The Young
frame (or the Young pattern) associated with
(A) is a collection
of
rows
of
boxes
(squares) aligned at the left such that the first row has AI boxes, the second row
A2
boxes, etc. Since Ai 2: AH
J,
the length
of
the rows decreases as one goes to the
bottom
of
theframe.
The Youngframe associated with
(A)
represents
xt'
...
x;",
which multiplies
the antisymmetric polynomial
D(xj).
To find the dimension of the irreducible
representation
T(A),
we have to count the number of ways in which the x-factors
can be permuted among themselves such that as we scan the product, thenumber
of
Xi'S
is neverlessthan number of X
/s
if
j > i. This leads to
