Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


24.5
RELATIONSHIP
OF
CHARACTERS
TOTHOSE
OF
ASUBGROUP
693
is
XCa\h) =
Lrna"~C"\h),
o
h e H. (24.32)
(24.33)
Multiply both sides by
~CK)*(h),
sum over h E
H,
aud take the complex conjugate
at the end. Then by the orthogonality relation (24.11), applied to H, we obtain
rn
aK
=
I~I
L
X(a)*(h)~CK)(h).
heR
Now multiply both sides of Equation (24.33) by X(a)(g), sum over ct, aud use
Equation
(24.30) to obtain
"rn
X(a)(g) = IGI
"8(K
G
KG).CK)(h).
L..,
cac
IHI
IKGI
L..,
h'
g 5
a g
heH
(24.34)
The sum on the right cau be trausformed into a snm over conjugacy classes of H.
Then Equation (24.34) becomes
" (a) _
JQL
" d
.•
(K)
L..,rnaKXi - IHI .
L..,
j5j
,
a C
l
j
i =
1,2,
...
, r,
(24.35)
where the sum on the LHS is over irreduciblerepresentations of G, aud on the RHS
it is over those
H-classes j that lie in the
ith
G-class. Note that the coefficients
IGldj/(IHlci)
are integers by Problem 23.17.
Equations (24.34) aud (24.35) are useful for obtaining characters of G when
those of a subgroup H are known. The general procedure is to note that the RHS
of these equations are completely determined by the structure of the group G aud
the characters
of
H.
Varying
i,
the RHS
of
(24.35) determines the r components
of a (compound) character I
J/r),
which, by the LHS, can be written as a linear
combination of characters
of
G:
r
IJ/r)
sa
Lrn
a
Ix
C
a»),
«=1
(24.36)
where we have suppressed the irrelevaut subscript
1<:.
If
we know some of the
Ix(a»)'s, we may be able to determine the rest by taking successiveinner products
to find the integers
rna, aud subtractingeach irreduciblefactor of the sum from the
LHS. We illustrate this procedure for
S«
in the following example.
24.5.1.
Example.
Let K 1 = (1
2
)
and K2 = (2) for S2 (see Section23.4 for notation).
Example
24.1.13
showed
that
wecan
construct
two
irreducible
representations
for
any
Sn,
the
symmetric
and
the
antisymmetric
representations.
The
reader
mayverify
that
these
two
representations
are
inequivalent.
Sincethe
number
of
inequivalent
irreducible
representa-
tions
is
equal
to the
number
of classesin a
group,
we haveallthe
information
needed
to

694
24.
GROUP
REPRESENTATION
THEORY
Table
24.2
Character table for 82.
1
1
1
-1
IKj
3K2
2K3
T(l)
I I 1
T(2)
1
-1
I
T(3)
? ?
?
Table
24.3
Partially filled character table for 83.
construct the character table for 82. Table 24.2 shows this character table. We want to use
the 82 character table to construct the character table for 83. With our knowledge of the
symmetric and the antisymmetric representations, we can partially
fill
in the 83 character
table.
LetKj
= (1
3
),
K2 = (2,
I),
aod K3 = (3)
aodnote
that
cj
= I, C2 = 3, aod C3 = 2.
Then we obtain Table 24.3. To complete the table, we start with K =1, and write the RHS
of Eqnation (24.35) as
>/Ii
= ~
Ld·«I)
=
~
L«I)
2ei j J J Ci j J
because d
j
==
1 for the two classes
of
82. The sum on the RHS is over Sz-cIasses that are
inside the ith S3-class. For i = 1, only the the first Sz-class contributes. Noting that
gy)
are the entries
of
Table 24.2, we get
3 (I) 3
>/It
=
-<I
= - . I = 3.
CJ
I
Similarly,
3 (1) 3 3
>/12
=
-<2
= - . I = I aod
>/13
= - . 0 =
O.
~ 3
~
The second equation follows from the fact that there are no classes
of
82 inside the third
class of 83. Eqnation (24.36) now gives
1>/1)
=
(i)
=
i:>.
Ix(·»).
o
a=l
We can find the number
of
times
Ix(l))
occurs in this compound character by taking the
innerproduct:
r
(x(l)
I
>/I)
= L
m.
(x(l)
I
x(·»)
=
mIlGI
=
6ml·
a=l
24.6 IRREDUCIBLE
BASIS
FUNCTIONS
695
1Kl
3K2
2K3
T(l)
1
1 1
T(2)
1
-1
1
T(3)
2
0
-1
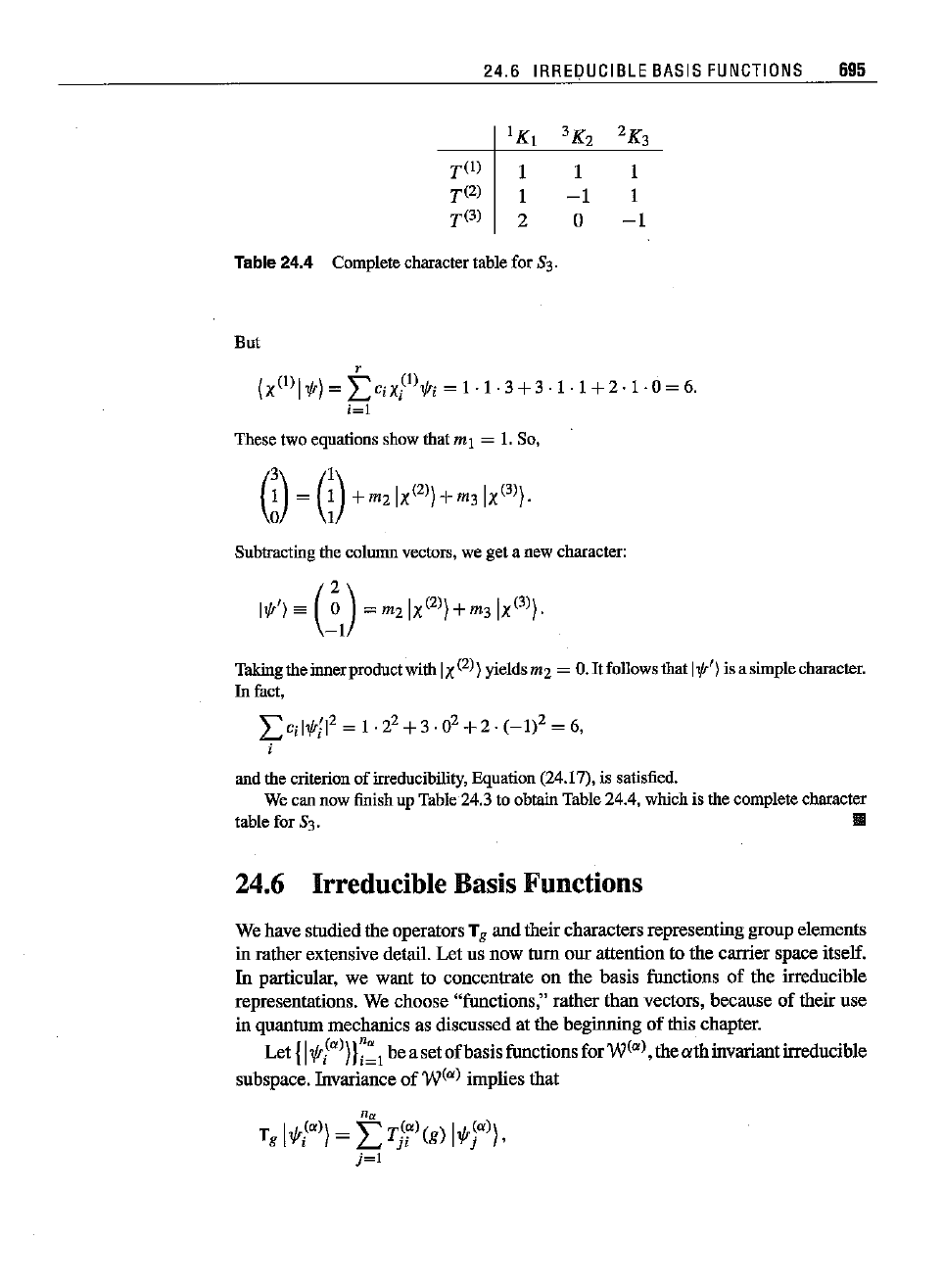
Table 24.4
Complete
character
tablefor83.
But
,
(x(l)
11/1)
= I:;c;
Xil)1/Ii
= I . I
·3
+
3·
I .
1+2·
I
·0
= 6.
i=l
Thesetwo
equations
show
that
m1= 1. So,
Subtracting
thecolumnvectors,we get a new
character:
Takingtheinnerproductwith 1X
(2»
yieldsm2 =
O.
It
followsthat
11/1')
isasimplecbaracter.
In fact,
I:;
c;l1/l[1
2
= I . 2
2
+3 . 0
2
+2 .
(_1)2
= 6,
aod the criterion of irreducibility,Equation (24.17), is satisfied.
Wecannow
finish
up
Table
24.3to
obtain
Table
24.4, whichis
the
complete
character
table for 53.
III
24.6 Irreducible Basis Functions
We have studied the operators
T.
and their characters representing group elements
in rather extensive detail. Let us now
tum
our attention to the carrier space itself.
In particular, we want to concentrate on the basis functions of the irreducible
representations.
Wechoose
"functions,"
rather
than
vectors, because of
their
use
in quantum mechanics as discussed at the beginning of this chapter.
Let {Iti
a»)
1:':1
be a set
of
basis functions forW(a), the
ath
invariantirreducible
subspace. Invariance
of
wCa) implies that
n.
T.
Iti·»)
= L
Tj~·)
(g)
Itja»),
j~1

696
24.
GROUP
REPRESENTATION
THEORY
(24.38)
functions
beionging
totheith
row
ofthe
ath
irreducibie
representation
where
Tj~a)
(g) are elements
of
the matrix
T1
a
)
representing g E G.
24.6.1. Definition.
Afunction
(or vector)
I<p/(a))
is said to belongto the Uh row
of
the
ath
irreducible representation (or to transform according to the
ith
row
of
the
ath
irreducible representation) ifthere exists a basis
{11ft/
aJ
)}
;:!
of
the
ath
irreducible representation
of
G with matrices
(Tj~a)
(g»)
and
n
a
-
lather
functions
{1<pja
J)}
such that
n.
T.
1<p/(a
J
)
=
LTj~aJ(g)
l<pja
J).
(24.37)
j~!
Fnnctions that belong to rows of irreducible representations have some re-
markable properties.
Let
11ft/
aJ
) and
l<pjP))
transform according to the
ith
and
jth
rows
of
the
ath
and fith irreducible representations, respectively. Choose an inner
productfor the carrier space such that all represeutatiousare unitary. Thenwe have
(
1ft/
a
)
I
<Pj~))
=
(T.
1ft/
aJ
IT
g<P
j~J)
IIIlI np
= L L
Tz~a)*(g)T~J
(g)
(1fti
aJ
I
<p~l).
[=1
m=l
Summing this equation over g yields IGI
(1ft/
aJ
I
<PjPJ)
for the LHS, while
CIGI/n('t)8
0/
pd/
mdij
n
a
nfJ,
. ,
RHS
= L L L
Tz~aJ*(g)T~)(g)
(1fti
aJ
I
<p~))
[=1
m=lgeG
-
~B
e.
~(.I.<aJI.J.(fJ)\
-
apUI]
L...J
'Pi
'Y[
I'
n
a
[=1
where we have made use of Equation (24.8). Therefore,
(1ft/
a
)
I
<Pj~J)
=
2.-Ba~Bij
t
(1fti
a
)
I
<p/(a)).
not
1=1
This shows that functions belonging to differentirreduciblerepresentations are
orthogonal. We should expect this, because in
our
construction of invariant irre-
duciblesubspaces,we kept dividingthe whole spaceinto orthogonalcomplements.
What
is surprising is thatfunctions transforming according to different rows
of
an
irreducible representation are orthogonal.
We
had
no control over this property!
It
is a consequence of Equation (24.37). Another surprise is the independence of
the innerproduct from
i:
If
we let i = j and a = fi on both sides
of
(24.38), we
obtain
(24.39)

L
Tl~)·(g)Tg
Iti·»)
gEG
symmetry
and
the
quantum
mechanical
perturbation
theory;
lilting
of
degeneracy
24.6
IRREDUCIBLE BASIS FUNCTIONS
697
which indicates thatthe inner prodnct on the LHS is independent
of
i,
24.6.2.
Example.
The quantum-mechanical perturhation theory starts with a known
Hamiltonian
Howitheigenvalues Ej andthecorresponding eigenstatesIEj}. Subsequently,
a (small)
perturbing
"potential"
Vis addedto the Hamiltonian, andthe eigenvaluesand
eigenstates of the new
Hamiltonian
H = Ho + V are
sought.
One can
draw
important
conclusions
about
the
eigenvalues
and
eigenstates
of thetotal
Hamiltonian
by
symmetry
arguments.
Supposethesymmetry groupof
HO
is G, andthatof His H, whichhas tohea subgroup
of G. In most cases,theeigenspaces of
HO
are
irreducible
carrier
spacesof G, i.e.,
their
basisvectorstransform according totherowsof irreducible
representations
of G.
If
H is a
propersuhgroupof G, thenthe eigenspacesof Howill splitaccordingto Equation(24.31).
We say thatsome of thedegeneracy is lifted because
of
the
perturbation
V.The
nature
of
the split,
i.e., the
number
andthe dimensionality of the vectorspaces into whicha given
eigenspacesplits,canbeobtainedhy the charactersof G andH andEquation(24.32).The
originaleigenspaces are
represented
on an energy diagram with a line
corresponding
to
eacheigenspace.Thesplitof theeigenspaceinto knew subspaces is thenindicated by the
branching
of theoldline intoknew lines.
To the lowest approximation-first-order
perturbation
theory-the
magnitude
of the
split,i.e., the difference between the eigenvaluesof
HO
andthose of H,is given by [see
Equation (21.57)] the expectationvalue
(oPi·)
IV
loP;·»),
where
loPi·»)
helongs to the ith
row of the
«th irreducihlerepresentation, and
loP]"»)
to its
jth
row (i #
j).
Only if this
expectation valueis
nonzero
will a split
occur.
This, in turn,
depends
on the
symmetry
of
V:
If
V is at least as
symmetric
as
Ho
(corresponding to G = H), then
(¢ja)
IV
ItP)a)}
=0,
andno splitting occurs
(Problem
24.17). If, on the otherhand,V is less
symmetric
than
Ho (corresponding to H c G), then V
loP;·»)
will not belong to the
jth
row of the
cth
irreducIble representation, and in general,
(oPi·)
IV
loP;·»)
#
O.
III
We
had
decomposed the carrier space V
of
a representation into invatiant
irreducible subspaces
WC.).
The
argumentabove shows that
each
WC.) has a basis
consisting
of
the "rows"
of
the irreducible representations. Correspondingto such
a basis, there is a set
of
projection operators
pl·)
with the property L.,i
pla)
= 1
(Chapter 4).
Our
aim is to find an expression for these operators, which have the
defining property
pl·)
Iti
a»)
=
Iti·»).
We start with Eqnation (24.37), multiply
both sides
of
it by
T/~).
(g),
sum over g E G,
and
use Equation (24.8) to obtain
n.
= L
Itya»)
L
T/~)·(g)Tj~a)(g)
j=l
geG
IGI
~
I
(a»)
IGI
I
(.»)
= -
LJ
t
j
8tj8
m
i
8
.p
=-
t
1
8
m
i
8
.p.
n
a
j=l
n
a
Let
fJ
= 01, m = I = i,
and
multiply both sides by n
a
/ 1GI.Then this equation
becomes
698 24.
GROUP
REPRESENTATION
THEORY
projection
operator
onto
the
i1h
row
of
the
eth
irreducible
representation
which
suggests
the
identification
p(.)
=
~
""
r.(.)*(
)T
I
IGI
LJ
/I
g s
geG
with
the properties
(24.40)
(24.41)
where
l4>i
a
») is the projectiou
of
14»
aloug
the
ith
row
of
the
ath
irreducible
representation.
We are also interested in the projection
operator
that
projects outo the irre-
ducible subspace
W(·)
.
Such
an operatoris
obtained
by
summing
pi·)
over i. We
thus obtain
projection
operator
onto
the
ath
irreducible
representation
n na n
p(.)
= ; L L r,\a)*(g) T
g
= ; L X
(·)*(g)T
g
1 1
geG
,~,
. 1 1
geG
=x(oo*(g)
and
(24.42)
(24.43)
where
14>(·»
is
the
projection
of
14>}
onto
the
ath
irreducible invariant subspace.
These
formulas are extremely useful in identifying the irreducible subspaces
of
a
given carrier space:
Start
with
a basis {Ia,)}
of
the
carrier space,
apply
p(.)
to all
basisvectors,
and
collectall the linearly
independent
vectors
of
the
form
p(.)
la,).
These
vectors form a basis
of
the
oth
irreducible representation.
The
following
example illustrates this point.
24.6.3.
Example.
Considerthe representation of 83 givenin Example
24.1.8,
wherethe
carrier space is the spanof the three functions
It,)
=
xy,
It2)
= yz, and
It3)
=
xz.
Werefer to the character table for 83 (Table24.4) and nse Equation (24.42) to obtain
p(l)
=
~(T,
+T2 +T3
+T4
+ Ts
+T6),
p(2) =
~(T,
-T2
-T3
-T4+TS
+T6),
6
p(3) =
~(2T,
- TS- T6),
6
where,asinExample24.1.8.wehaveusedthenotation
r,
forT1l'j,andtheresultnl = nz = 1
and n3 = 2 obtained from Eqnation (24.18), Theorem 24.4.4, and the fact that 83 is
nonabelian.
Toget thefirstirreduciblesubspaceof thisrepresentation,we apply
p(l)
to I
tI).
Since
this subspaceis one-dimensional, the
procedure
will give a basis for it if the vector so
24.7
TENSOR
PROOUCT
OF
REPRESENTATIONS
699
obtained
is
nonzero:
p(l) I'h) =
~(T1
+T2
+ T3 + T4
+TS
+T6)
I'h)
I I
= "6(1"'1)+ 1"'1)+ 1"'2) + 1"'3) + 1"'3) + 1"'2)) = 3(1"'1) + 1"'2) + 1"'3))'
Thisis a basisforthe
carrier
spaceof the
irreducible
identity
representation.
Forthesecond
irreducible
representation,
we get
Similarly,
p(2) 1"'2) = 0 and p(2) 1"'3) = O.This meansthat
T(2)
is not inclndedin the
representation
we are
working
with. We shouldhave expectedthis,becauseif this one-
dimensional
irreducible
representation
wereincluded, it wouldforce thelast
irreducible
representation
to be one-dimensional as well [see
Equation
(24.18)],
and,
by
Theorem
24.4.4,
thegronp
S3
tobe
abelian!
Thelast
irreducible
representation
is
obtained
similarly.
Wehave
Thesetwo
vectors
are
linearly
independent.
Therefore,
they
form
a basis forthe last ir-
reducible
representation.
The
reader
maycheck
that
p(3)It3}is a
linear
combination
of
p(3) 1"'1) andp(3) 1"'2)'
l1li
24.7 TensorProduct
of
Representations
A simple quantummechanical system possessingagroup of symmetryis described
by vectors that transform irreducibly (or according to a row
of
an irreducible
representation). For example, a rotationally invariant system can be described by
an eigenstate of angnlar momentum, the generator
of
rotation.
s
These eigenstates
transform as rows of irreducible representations of the rotation group. At a more
fundarnentallevel, the very concept of a particle or field is thought
of
as states
that transformirreducibly under the fundamental group of spacetime, the Poincare
group.
Often these irreducible states are "combined"to form new states. For example,
the state
of
two (noninteracting) particles is described by a two-particle state,
labeled by the combined eigenvalues
of
the two sets of operators that describe
each particle separately. In the case of angular momentum, the single-particle
states may be labeled as
Iii, mil for i =
1,2.
Then the combined state will be
labeled as
111,
m1;
12,
m2), and one can define an action of the rotation group on
5Chapter
27will
make
explicitthe
connection
between
groups
~d
their
generators.

700
24.
GROUP
REPRESENTATION
THEORY
the vectorspace spannedby these combinedstates to construct a representation.
Wenowdescribethewayin whichthisis done.
Snpposethat
T : G -+
GL(V)
and S : G -+
GL(W)
are tworepresentations
ofa
gronpG. Let V<8lWbethe tensorproductof Vand W (seeExample1.3.19).
Now define an actionof the group G on V
<8l
W via the representation T
<8l
S :
G -+
GL(V
<8l
W) givenby
T
<8l
S(g)(lv),
Iw»
=
(T(g)
Iv),
S(g)
Iw».
Wenotethat
T
<8l
S(gjgz)(lv),
Iw»
=
(T(gjgz)
Iv),
S(gjgz) Iw}) =
(T(gj)T(gz)
Iv),
S(gj)S(gz) Iw})
= T
<8l
S(gj)(T(gz)
[u}, S(gz) Iw» = T e
S(gj)T
e
S(gz)(lv},
Iw».
Kronecker
product
It
followsthatT
<8l
Sis indeedarepresentation, calledthetensorprodnctordirect
representation
product
or
Kronecker
productrepresentation.
It
is common, especiallyin the
physicsliterature,to write[u,
wi,
orsimplyIvw) for (lu) , Iw}),andTS for T
<8l
S.
If
we choosethe orthonormal bases
Ilvi}}
for V and
Ilw
a}}
for W, and definean
innerproducton V
<8l
W by
(v, wi v', w')
ea
(vi
v')
(wi
w'),
character
of
a
product
representation
isa
product
of
characters
we obtaina matrixrepresentationof the gronp withmatrixelementsgivenby
(T
<8l
S)ia,jb(g)
==
(Vi, wal T e S(g) IVj,
Wb}
= (vii
T(g)
IVj) (wal S(g)
IWb)
==
T;j(g)Sab(g).
Notethattherowsandcolumnsofthismatrixaredistinguishedbydoubleindices.
If
the matrix T is m x m and S is n x n, then the matrixT
<8l
S is (mn) x (mn).
The characterof the tensorproductrepresentationis
XT®s(g) =
L(T
<8l
S);a,ia(g) = L T;i
(g)Saa
(g) = L T;i(g) L Saa(g)
t.a
i,a i a
(24.44)
So the characterof thetensorproductis the prodnctof theindividualcharacters.
An importantspecialcase is thetensorproduct of arepresentationwithitself.
Forsucha representation, thematrixelementssatisfythe symmetryrelation
(T
<8l
T)ia,jb(g) =
(T
<8l
T)ai,bj(g). This symmetry can be used to decompose the
tensor product space into two snbspaces that are separately invariantunder the
actionof the group.To do this, take the span of all the symmetricvectors of the
form
(Iviwj)
+ IVjwi» E V
<8l
V and denote it by (V
<8l
Vis. Similarly, takethe
spanof all the antisymmetric vectorsof theform
(Iviw
j)
-
IVjwi})
E V
<8l
Vand
denoteitby (V
<8l
Via. Nextnote that
IViWj) =
!(Iviwj)
+IVjwi» +
!(Iviwj)
-Ivjwi».
24.7
TENSOR
PROOUCT
OF
REPRESENTATIONS
701
It follows that every vector of the product space can be written as the sum of a
symmetric and an antisymmetric vector. Furthermore, the only vector that is both
symmetric and antisymmetric is the zero vector. Therefore,
V® V = (V® V),
Eil
(Ve V)a.
Now consider the action of the group on each of these subspaces separately.
From the relation
T®
T(g)
IViWj) es T ®
T(g)(lvi)
, IWj))
=
(~Tki(g)
IVk)
,
~
Tlj(g)
IWI))
= L
niTlj(g)(g)
(IVk),
IWI))
=
L(T
e
T)kl.ij(g)
IVk
W/)
k,/
k,1
weobtaio
T ®
T(g)(lviWj)
± IVjWi)
= L
[(T
e T)kl,ij (g) ±
(T
®
T)kl,ji
(g)] IVkWI).
k,/
(24.45)
Kronecker
product
reduces
tothe
symmetric
and
the
antisymmetric
representations
Problem 24.21 shows that the RHS can be written as a sum over the symmetric
(for the plus sign) or antisymmetric (for the minus sign) vectors alone.
It
follows
that
24.7.1. Box. The Kronecker product
of
a representation with itself is al-
ways reducible into two representations, the symmetrizedproduct and the
antisymmetrizedproduct representations.
24.7.1 Clebsch-Gordan Decomposition
A common situation in quantum mechanics is to combine two simple systems
into a composite system and see which properties
of
the original simple systems
the composite system retains. For example, combining the angular momenta of
two particles gives a new total angnlar momentum operator. The question of what
single-particle angnlar momentum states are included in the states of the total an-
gular momentumoperatoris the content of selection
rules
and is
of
great physical
interest: A qnark and an antiquark (two fermions) with
spin!
always combine
to form a mesou (a boson), because the resnltiug composite state has no projec-
tion onto the subspace spanned by half-integer-spin particles. In this section, we
study the mathematical foundation of this situation. The tensor product of two
irreducible representations
T(a)
and
T(jJ)
of
G is denoted by rv-». and it is,

702 24.
GROUP
REPRESENTATION
THEORY
in general, a reducible representation.
The
characters, generally compound, are
denoted by X(ax
Pl.
Equation (24.14), combined with Equation (24.44), tells us
what irreducible representations are present in the tensor product, and therefore
onto whichirreduciblerepresentations the productrepresentationhas nonzeropro-
jection:
r
xi
axPl
=
xi
a1
.
X'<pl
= L
m':/
xi
a1
,
0'=1
where
m~fJ
are
nonnegative
integers.
We
rewrite
this
more
conveniently
in
terms
of vectorsas
r
Ix(ax
P
1}
= Lm,:/ Ix(a
l},
0'=1
map = _1_
(x(all
X(axPl) =_1_
~
c'X-(alX(alX(/ll
a IGI
IGI~
I I I I •
simply
reducible
A group for which
m':/
= 0, 1is called
simply
reducible.
group
(24.46)
Rudolph Friedrich Alfred C1ebsch (1833-1872) studied math-
ematics
in the
shadow
of
Jacobi
at the
University
of
Konigsberg,
twoof his
teachers
having
been
students
of
Jacobi.
After
gradua-
tionhehelda
number
ofpositions in
Germany,
including
positions
atthe
universities
of
Berlin,
Giessen,
and
finally
Gottingen,
where
he
remained
untilhis
death.
He and
Carl
Neumann,
sonof one of
the
aforementioned
Jacobian
teachers,
founded
the
Mathematische
Annalen.
Clebsch
began
his
career
in
mathematical
physics,
producing
a
doctoral
thesison
hydrodynamics
andabookonelasticity in which
he
treated
theelastic
vibrations
ofrods
and
plates.
These
works
were
primarily
mathematical,
however,
andhesoon
turned
his
attention
more
to
pure
mathemat-
ics. His
links
to
Jacobi
gaveriseto his
first
work
in
that
vein,
concerning
problems
in
variational
calculus
and
partial
differential
equations,
in
which
he
surpassed
the
results
of
Jacobi's
work.
Clebsch
first
achieved
significant
recognition
forhis
work
in
projective
invariants
and
algebraicgeometry.He wasintriguedby theinterplaybetween algebra and geometry,and,
since
many
results
inthe
theory
of
invariants
have
geometric
interpretations,
thetwo
fields
seemed
natural
choices.
24.7.2.
Example.
ReferringtoTable24.8of Problem24.15,andnsingEqnation(24.44),
wecanconstructthecompoundcharacter
IX(4x5)} with components9,
-1,1,0,
-1.
Then,
