Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


left
action,
right
actlon.len
invariance
and
right
invariance
any
group
is
isomorphic
toa
subgroup
of
the
group
of
transformations
of
an
appropriate
set
orbit,
stabilizer;
transitive
action
and
effective
action
23.3
GROUP
ACTION
663
which
Abel
incorporated
their
inverse
functions
toshow
that
they
are
anatural
generalization
of the
trigonometric
functions.
In later
research
in this
area,
Abel foundhimselfin stiff
competition
with
another
young
mathematician,
K.G. J.
Jacobi.
Abelpublished some
papers
on
functional
equations
and
integrals
in 1823.hi ithe givesthe
first
solutionof an
integral
equation.
In 1824he
proved
theimpossibility of solvingalgebraically the
general
equation
of the
fifth
degreeand
published
it athis ownexpensehopingto
obtain
recognition forhis
work.
Despitehis
proven
intellectual success,Abelnever
achieved
material
success,noteven
a
permanent
academic
position.In December of 1828, while
traveling
bysled to visit his
fiance
for
Christmas,
Abelbecameseriously illanddiedacoupleof
months
later.
Ironically,
his
death
from
tuberculosis
occurred
twodaysbeforeCrelle
wrote
withthe
happy
newsof
an
appointment
forAbel ata scientific
institute
in Berlin.In Abel's eulogyin his
journal,
Crelle
wrote:
"He distinguished himselfequallyby thepurityaudnobilityofhis characteraudby a
rare
modestywhichmadehisperson
cherished
tothesamedegreeaswashis
genius."
23.3
Group
Action
The transformation groups introduced at the beginning
of
this chapter can be
described in the language of abstract groups.
23.3.1.
Definition.
Let
G be a group and M a set. The
left
action
of
G on M is
a map
<I>
: G x M --> M such that
1.
<I>(e,m)
= m
for
all m
EM;
2.
<l>(g1g2,
m) =
<I>(gl,
<I>
(g2,
m».
One usually denotes
<I>(g,
m) by
g.
m or more simply by gm. The right action is
defined similarly. A subset N
c M is called
left
(right) invariant ifg .
mEN
(m'g
EN)forallg
E
G,wheneverm
E N.
23.3.2.
Example.
lfwe
define
fg : M
-->
M by fg(m) es
<1>(g,
m) =
g.
m, thenI
g
is
recognized as a
transformation
of M. Thecollectionof such
transformations
is asubgroup
ofthesetof all
transformations
of M.
Indeed,
the
identity
transformation
is simply[e, the
inverse
of
/g
is
/g-I,
andthe(associative) lawof composition is
fg
1
0
fg
2
= /gI82'
There
is a
general
theorem
in
group
theory
stating
thatany
group
is
isomorphic
to a
subgroup
of
the
group
of
transformations
of an
appropriate
set.
IIIlII
23.3.3. Definition.
Let
G
acton
M and let mo E M. The
orbitofmo,
denoted by
Gmo, is
Gmo = {m E M Im =gmo
for
some g E
Gl·
The action is called transitive ifGmo = M. The stabilizer
ofmo
is G
mo
= {g E
G Igmo = mol· The group action is called effective ifgm = m
for
all m E M
implies that g = e.
664 23.
GROUP
THEORY
Stabilizer
isa
subgroup.
The
reader may verify thatthe orbit Gmo is the smallest invariant subset
of
M
containing
mo,
and that
23.3.4. Box.
The stabilizer
ofmo
is a subgroup
of
G, which is sometimes
called the little group
of
G at mo.
A transitive actiou is characterized by the fact that given any two points
ml,
m2 E
M,
one can find e g e G such that m2 = gm«.
23.3.5.
Example.
LetM = lit
2
and G =
80(2),
theptanarrotationgroup.Theactiouis
rotation
of
a pointin the planeaboutthe origin by an angle (1. The orbits are circles centered
at the origin.
The
action is effective but not transitive.
The
stabilizer
of
every point in the
plane is
{eI,except the origin, for which the whole group is the stabilizer.
Let M = SI, the unit circle, and G =
80(2),
the rotation group in two dimensions.
The action is displacement
of
a
point
on the circle. There is only one orbit, the entire circle.
The action is effective and transitive. The stabilizer
of
every point on the circle is {e}.
Let M = G. a group, and let a (proper) subgroup S act on G by left multiplication.
The orbits are rightcosers Sg
of
the subgroup.
The
actionis effective but not transitive.
The
stabilizer of every point in the group is {e}.
Let
M =
1R
U {oo},the set
of
real numbers including
"the
point at infinity." Define an
actionof 8L (2, lit) on M by
(
a
b).x=ax+c.
c d bx
+d
The
readermay checkthatthis is indeeda groupaction with a law
of
multiplicationidentical
to the matrix multiplication,
and
that the action is transitive, but
not
effective.
realization
ofa
group
Let M be a set and H the group of transformations of
M.
Suppose that there is a
homomorphism f :G --+ H from a group G into H.
Then
there is a natural action
of
G on
M givenby g . m
'"
[f(g)](m).
The homomorphism f is sometimes called a realization
ofG.
III
23.4 The Symmetric Group s,
Because
of
its primary importance as the prototypical finite group, and because
of
its sigoificance in quantum statistics, the symmetric (or permutatiou) group
is briefly discussed in this section.
It
is also
used
extensively in the theory
of
represeutation
of
the general linear group and its subgroups,
A generic permutation
n
of
n numbers is shown as
2
".(2)
".(i)
(23.1)
Because the mapping is bijective, no two elements can have the same image, and
".(1), ".(2),
...
,
".(n)
exhaust all the elements in the set {iJi'=j'
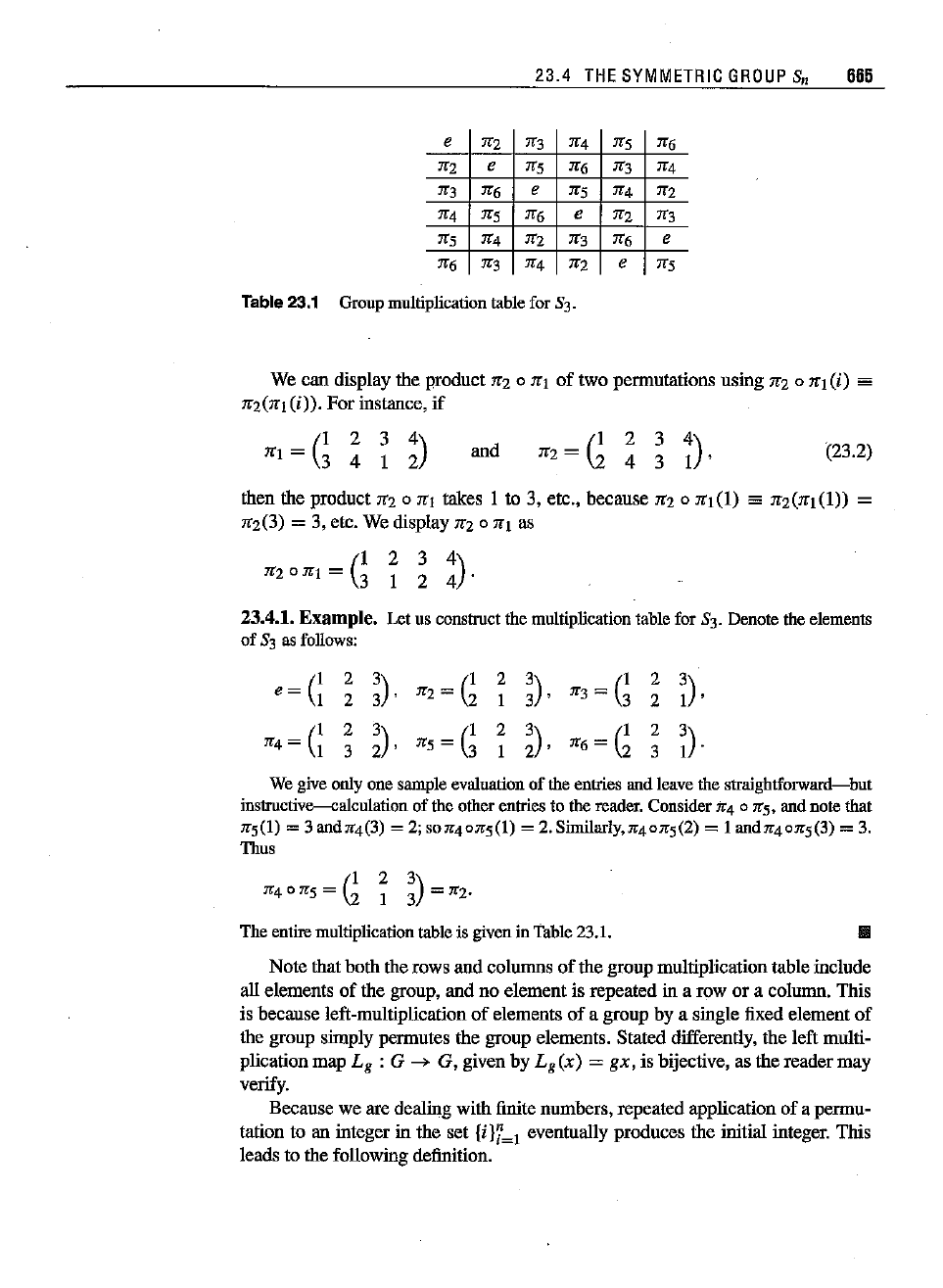
23.4
THE
SYMMETRIC
GROUP
8" 665
e
"Z
"3
"4
"s
"6
"z
e
"S
"6
"3
"4
"3
"6
e
"S
"4
"z
"4
"s
"6
e
"Z
"3
"S
"4
"Z
"3 "6
e
"6
"3
"4
"z
e
"s
Table
23.1
Groupmultiplicatiou tablefor 83-
We
can
display the product "z 0
"1
of two penuutations using "z 0
"I
(i)
==
"z
("I
(i)).
For
instance,
if
(
1 2 3
4)
"1=3412
and
(I
2 3
4)
"z=\243I'
(23.2)
then the product
"z 0
"1
takes I to 3, etc., because "z 0
"I
(1)
ea
"Z("I
(1)) =
"z(3) = 3, etc. We display
"z
0
"I
as
(
I 2 3 4)
"z 0
"1
= 3 1 2 4 .
23.4.1. Example. Let us constructthe multiplication tablefor 83- Deuotetheelements
of
83 as
follows:
e = G
~
;),
»z
=G
~
;),
"3
=G;
i),
"F
G;
;),
"s =G
~
;),
"6
= G;
i)-
Wegiveonlyone
sample
evaluation
of the
entries
andleavethestraightforward-c-but
instructive---calculation
of the
other
entries
to the
reader.
Consider
ft4 0
:n'5.
andnote
that
"s(l)
= 3and"4(3) = 2;so
''4o"s(l)
=2.Similarly, "40"S(2) = I and
"4
0"S(3) = 3.
Thus
(I
2 3)
n'4 0 :lr5 = \2 1 3 = ]f2·
The
entire
multiplication
table
is givenin
Table
23.1.
III
Note that both the rows and columns
of
the group multiplication table include
all elements
of
the group, and no element is repeated in a row or a column. This
is because left-multiplication
of
elements of a group by a single fixed element of
the group simply penuutes the group elements. Stated differently, the left multi-
plication map
L
g
:
G --> G, given by Lg (x) = gx, is bijective, as the reader may
verify.
Because we are dealing with finite numbers, repeated application of a permu-
tation to an integer in the set
{i}i~1
eventually produces the initial integer. This
leads to the following definition.

666 23.
GROUP
THEORY
cycles
of
symmetric
group
23.4.2. Definition.
Let:n:
E Sn, i E {I, 2,
...
, n},
and
let r be the smallestpositive
integer such that
n"
(i)
= i. Then the set
ofr
distinctelements
{:n:
k
(i)}~:b
is called
a cycle of:n:
of
length r or an r-cycle generated by i.
Start with 1and
app1y:n:
to it repeatedlyuntil you obtain 1again. The collection
of elements so obtained forms a cycle in which 1 is contained. Then we select a
second number that is not in this cycle and apply
:n:
to it repeatedly until the
original number is obtained again. Continuing in this way, we produce a set of
disjoint cycles that exhausts
all elements
of
{I, 2,
...
, n}.
23.4.3. Proposition.
Any
permutation can be broken up into disjoint cycles.
It
is customary to write elements of each cycle in some specific order within
parentheses starting with the first element, say
i, on the left, then
:n:(i)
immediately
to its right, followed by
:n:
2(i),
and so on.
For
example, the permutations
:n:\
and
:n:2
of
Equation (23.2) and their product have the cycle structures rrj = (13)(24),
:n:2
=(124)(3),
and:n:2
o:n:\
=(132)(4), respectively.
23.4.4.
Example.
Let:n:\,:n:2
E Sg be givenby
(
1 2 3 4 5 6 7 8)
:n:\
= 3 5 7 I 2 8 4 6 '
The
reader
mayverify
that
(
I 2 3 4 5 6 7 8)
:n:2=
2 5 6 8 I 7 4 3 .
2 3 4 5 6 7
78)
t
425
3 8
and that
,,\
= (1374)(25)(68),
"2
= (125)(36748), "20:n:\ = (16342)(5)(78).
In general,
permutations
donot
commute.
The
product
inreverse
order
is
(
I 2 3 4 5 6 7 8)
:n:\
o:n:2
= 5 2 8 6 3 4 I 7 = (15387)(2)(46),
whichdiffers
from.7l'2
osrj
,
However,
notethatithasthesamecycle
structure
as
Jr2
01l'"I.inthat
cyclesof equallengthappear
in
both. Thisis a generalpropertyof allpermutations,
11II
cyclic
permutations
23.4.5. Definition. If:n: E S« has a cycle
of
length r
and
all other cycles of:n:
defined
have only one element, then
:n:
is called a cyclic
permutation
of
length r.
It
follows !hat:n:2 E S4 as defined earlier is a cyclic permutation of length 3.
Similarly,
(
1 2 3 4 5 6)
:n:= 6 2 1 3 5 4
is a cyclic permutation
oflength
4 (verify this).

23.4 THE SYMMETRIC
GROUP
s;
667
transpositions
23.4.6. Definition. A cyclic permutation
of
length 2 is called a transposition.
defined
A transposition (I
j)
simply switches i and
j.
23.4.7.
Example.
Products of (notnecessarily disjoint)cyclesmaybeassociated witha
permutation whose actionon i is
obtained
by
starting
with thefirstcycle (at the extreme
right),
locating
the
first
occurrence
ofi, and
keeping
track
of
what
eachcycledoesto itorits
image
under
the
preceding
cycle.For
example,
let1fl E S6begivenasa
product
of cycles
by
rrj
= (143)(24)(456). Tofindthepermutation, we startwith1aodfollowtheactionof
thecycles on it,
starting
fromtheright.The firstandsecond cycles leave 1 alone,andthe
last cycle takes it to 4. Thus, 1fl(1) = 4. For 2 we note that the first cycle leaves it alone,
the secondcycletakesit to 4, aodthe lastcycletakes4 to 3. Thus,"'\ (2) = 3.
Similarly,
"'\ (3) = 1,"'1 (4) = 5,"'\ (5) = 6, aod"'\ (6) = 2. Therefore,
(
1 2 3 4 5 6)
"'\
= 4 3 1 5 6 2 .
Wenote
that
1l'1
is acyclicpermutation
of
length
6.
It
is left to the reader to show that the permutation"'2 E S5 givenby the product
"'2 = (13)(15)(12)(14) is
cyclic:
"'2 = (14253).
11II
The square of any transposition is the identity. Therefore, we can iuclude it iu
any product of permutations without changing anythiug.
23.4.8. Proposition.
An r-cycle
(iI,
[Z,
...
,
i,)
can be decomposed into theprod-
uct
of
r - 1transpositions:
(1\, tz,
...
,
i,)
=
(lli,)(i\i,-\)'"
(1\;3)(1\;2).
Proof The proof iuvolves keepiug track
of
what happens to each symbol when
acted uponby the RHS and the LHS and showiug that the two give the same result.
This is left as an exercise for the reader. 0
parity
ofa
permutation
defined
parity
ofa
permutation
is
unique
Although the decomposition
of
Proposition 23.4.8 is not mtique, it can be shown
that the
parity
of the decomposition (whether the number
of
factors is even or
odd) is unique. For iustance, it is easy to verify that
(1234)
= (14)(13)(12) = (14) (34)(34) '(23) (12)(12)(23)(13)(12).
'-v----' '-v----'
1 1
That is, (1234) is written as a product of 3 or 9 transpositions, both
of
which are
odd.
We have already seen that any permutation
can
be written as a product of
cycles.
Inaddition, Proposition 23.4.8 says that these cycles can be further broken
down iuto products of transpositions. This implies the followiug (see [Rotm 84,
p.38]):
23.4.9. Proposition. Any permutation can be decomposed as a product
of
trans-
positions. The parity
of
the decomposition is
unique.'

668
23.
GROUP
THEORY
even
and
odd
permutations
23.4.10. Definition. Apermutationis even(odd)
ifit
can be expressedasaproduct
of
an even (odd) number
of
transpositions.
The parity
of
a permutation can be determined from its cycle structure and
Proposition 23.4.8.
The reader may verify that the mapping from Sn to the multiplicative group of
{+I,
-I}
that assigns +I to even permutations and
-I
to odd permutations is a
group homomorphism.
It
follows from the first isomorphism theorem (Theorem
23.2.16) that
23.4.11. Box.
The set
of
even permutations, denotedby An
,forms
a normal
subgroup
of
Sn·
This homomorphism is usually denotedby E. We therefore define
)
_
{+I
ifn
is even,
E(n =
E"
=
-I
ifnisodd.
(23.3)
Sometimes
8(n)
or 8" as well as
sgn(n)
is also used. The symbol,
Eili,
...i, used in
the definition
of
determinants, is closely related to E(n). In fact,
E
n(l)Jl'(2)
...Jt(n)
==
En·
-I
Suppose
it,
a E Sn, and note that?
a(i)
H i
~
neil
~
a 0
n(i),
i.e.,
the composite
a 0
no
a-
t
of
the three permutations takes
a(i)
to a 0
neil.
This
compositecan be thought of as the permutation obtained by applying
a to the two
f
(
t 2
n)
rows0 n =
n(l)
n(2)
Jt(n) :
-1
(a(l)
a (2)
a
0 n 0 a = a 0
n(l)
a 0
n(2)
a(n)
)
a
on(n)
.
In particular, the cycles of a 0 n 0 a
-1
are obtained by applying a to the symbols
in the cycles of
it
. Since a is bijective, the cycles so obtained will remain disjoint.
It
follows that a 0
tt
0 a- t, a conjugate
of
n , has the same cycle structure as x
itself. In fact, we have the following:
23.4.12.
Theorem.
Two permutations are conjugate ifand only ifthey have the
same cycle structure.
To find the distinct conjugacy classes
of
Sn, one has to construct distinct cycle
structures of
Sn. This in
tum
is equivalent to partitioning the numbers from I to n
7Recall from Chapter 0 that x
J..-"
y means y =
f(x).

23.5
PROBLEMS
669
into sets
of
various lengths. Let Vk be the number
of
k-cycles in a permutation.
The
cycle structure
of
this permutationis denotedby
(I'",
2
V2
,
•••
, n
V
, ) .
Sincethe total
number
of
symbols is n, we
must
have
I:k~1
kVk
= n. Defining Aj es
I:k~j
Vb
we have
(23.4)
partition
ofn
Conjugacy
classesof
S, are
related
tothe
partition
of
n.
The splitting
ofn
into nonnegative integers (AI, A2,
...
, An) as in Equation(23.4)
is called a
partition
of
n. There is a
I-I
correspondence between partitions
of
n
and the cyclestructure
of
Sn. We saw how Vk 's gave rise to A'S.Conversely, given a
partition
of
n, we can construct a cycle structure by Vk =
Ak
-
Ak+
I.
For
example,
the partition (32000)
of
Ss corresponds to
VI
= 3 - 2 = I, V2 = 2 - 0 = 2,
i.e., one I-cycle and two 2-cycles. One usually omits the zeros and writes (32)
instead
of
(32000). When some
of
the A'Sare repeated, the number
of
occurrences
is indicated by a power
of
the corresponding A; the partition is then written as
(J.L~I,
tL?,
...
,f.J-~r),
whereitis understood thatAl
through
AnI
havethe
common
value ILl, etc.
For
example, (3
21)
corresponds to a partition
of
7 with Al = 3,
A2
= 3, and
A3
= 1.
The
corresponding cycle structure is
VI
= 0, V2 = 2, and
V3 = I, i.e., two 2-cycles and
one
3-cycle.
The
partitions
of
length 0 are usually
ignored. Since
I:
Ai
= n, no confusion will arise as to which symmetric group
the partition belongs to. Thus (32000) and (332000) are written as (32) and (3
22),
and it is clearthat (32) belongs to Ss and (3
22)
to Ss.
23.4.13.
Example.
Let us findthe differeutcycle structuresof 84' This correspoods to
different
partitions
of 4. Wecan
take
Al = 4 andtherestof the
},,'s
zero.
Thisgives the
partition(4).Next,weletaI =3;then
1.2
mustbe I, givingthepartition(31).WithAl = 2,
A2
canbe
either
2 or1.In the
latter
case,
),,3
mustbe1aswell, andwe obtaintwo
partitions,
(2
2)
and (21
2).
Finally,
if
Al = 1,all othernonzeroA'smustbe 1 as well(remember that
Ak 2: Ak+I).Therefore, thelastpartitionisoftheform(1
4
).
Weseethatthereare5different
partitionsof 4. It follows thatthereare5 different conjugacyclasses in84. II
23.5 Problems
23.1. Let S be a subset
of
a group G. Show that S is a subgroup
if
and only if
ab-
I
E S whenever a, b E S.
23.2. Show that the intersection
of
two subgroups is a subgroup.
23.3. Let X be a
subset
of
a group G. A
word
on X is either
eo
or an element w
of
G
of
the form
where
Xi E X and e; = ±1. Show that the set
of
all words on X is a subgroup
of
G.

670
23.
GROUP
THEORY
23.4.
Let
la, b] denote the commutator
of
a
and
b. Show that
(a) la,
bj-I
= [b,a],
(b) [a,a] =
eforalla
E G, and
(c)
ab = la, b]ba. It is interestiog to compare these relations with the familiar
commutators
of
operators.
23.5. Show that if S is a subgroup, then S2 sa SS =S,
and
tS =S
if
and only
if
t E S. More generally, TS = S if
and
only if T c S.
23.6. Show that if S is a subgroup, then
Sa = Sb
if
and only
if
ba:"
E S and
ab-
I
E S
(as
= bS if and only if
a-Ib
E
Sand
b-1a
E S).
23.7.
Let
S be a subgroup
of
G. Show that a I> b defined by
ab-
1
E S is an
equivalence relation.
23.8. Show that CG
(x)
is a subgroup
of
G.
Let
H be a subgroup
of
G
and
suppose
x E H. Show that CH(X) is a subgroup
of
CG(x).
23.9. (a) Show that the only element a in a group with the property a
2
= a is
the identity.
(b) Now use ec *eo = eo to show that any homomorphism maps
identity to identity, (c) Show that
if
f : G --> H is a homomorphism, then
f(g-I)
=
[f(gJrl.
23.10. Establisha bijectionbetweenthe set
of
rightcosets and the set
ofleft
cosets
of
a subgroup. Hiot: Define a map that takes St to
t-
I
S.
Lagrange
theorem
23.11.
Let
G be a finite group
and
S one
of
its subgroups. Convince yourselfthat
the union
of
all right cosets
of
Sis
G. Now use the fact that distioctrightcosets are
disjoiot and thatthey have the same cardinality to provethat the order
of
S divides
the order
of
G. In fact, IGI =
ISIIGISI,
where IGISI is the number
of
cosets
of
S (also called the iodex
of
S io G). This is
Lagrange's
theorem.
23.12.
Let
f : G --> H be a homomorphism. Show that ¢ : GI ker f --> f
(G)
given by
¢(glker
f])
sa
¢([ker
f]g)
=
f(g)
is an isomorphism.
23.13.
Let
G'
denote the commutator subgroup
of
a group G. Show that
G'
is a
normal subgroup
of
G and that
GIG'
is abelian.
23.14.
Let
M = IRU {oo}, and define an action
of
SL(2,
IR)on M by
(
a
b).x=ax+c.
c d bx
+d
Show that this is iodeed a group action with a
law
of
multiplication identical to
the matrix multiplication,
and
that the action is transitive,
but
not
effective.
23.15. Show that two conjugacy classes are either disjoiot or identical.
23.16. Show that if all conjugacy classes
of
a group have only one element, the
group
must
be abelian.

23.5
PROBLEMS
671
23.17. Consider a map from the conjugacy class of G containing x E G to the
set of (left) cosets
G/CG(x)
given by
q,(axa-
t
)
= aCG(x). Show that q, is a
bijection.
In
particular, show that ICG(x)1 =
IGI/IK~I
where
K~
is the class
in
Gcontaining x and
IK~I
its order (see Problem 23.11). Use this result and
Problems 23.8 and 23.11 to show that
IHI/IK:I
divides
IGI/IK~I.
23.18. Show that R
R.
(0)
R-
1
corresponds to a rotationof angle O.Hint: Consider
the effect of rotation on the vectors in the plane perpendicular to
e,
and note that
the rotated plane is perpendicularto
f/ es
Re.
23.19. Let G act on M and let mo E M. Show that
Gmo
is the smallest invariant
subset of
M containing mo.
23.20. Suppose G is the direct product
of
HI and H2 and g = hlh2. Show that
the factors
hI and h2 are unique and that HI and H2 are normal.
23.21. Show that
(g, h), (g',
h')
E
GxH
are conjugate
ifandoulyifg
isconjugate
to
g' and h is conjugate to h', Therefore, conjugacy classes of the directproduct
are obtained by pairing one conjugacy class from each factor.
23,22. Findthe products
ITI 0 IT2 and IT2 0 ITI
of
the two permutations
(
1 2 3 4 5 6)
ITI = 3 4 6 5 1 2
and
23.23. Find the inverses
of
the permutations
(
1 2 3 4 5 6 7 8)
ITI = 3 5 7 I 2 8 4 6 '
_
(1234)
-
3241'
and show directly that (ITI 0
IT2)-1
= IT
Z
1
0
ITil.
23.24. Find the inverse of each
of
the following permutations: ITI
IT2
=
(]
gg),
IT
3
=
(~B
~
W'andrra=
<§
gW·
23.25. Express each
of
the following products in terms of disjoint cycles. Assume
that all permutations are in 87.
(a) (123)(347)(456)(145). (b) (34)(562)(273). (c) (1345)(134)(13).
23.26. Express the following permutations as products
of
disjoint cycles, and
determine which are cyclic.
()
(
1 2 3 4 5 6 )
a
134562.
(
b)
(123456)
214563
.
()(
1 2 3 4 5 )
c
13542.
2327
E th
. -
(12345678)
"d
f .
. . xpress e permutation Jr - 2 4 1 3 6 8 7 5 as a pro uet 0 transposi-
tions.Is the
permutation
evenorodd?

672 23.
GROUP
THEORY
23.28. Express the following permutations as products of transpositions, and de-
termine whetherthey are even or odd.
(a) G
(c)
(~
2 3
4 2
2 . 3
4 5
(b)(4
1 2 3 4 5 6 7 8)
1 7 8 3 6 5 2 .
(d)(6
1 2 3 4 5 6 7)
724153'
23.29. Show that the product
oftwo
even or two odd permutations is always even,
and the product of an even
and
an odd permutation is always odd.
23.30. Show that
ic and
n-
1
have the same parity (hoth even or both odd).
23.31. Find the number of distinct conjugacy classes
of
Ss and S6.
Additional Reading
1. Hamermesh, M. Group Theory and its Application to Physical Problems,
Dover, 1989. The classic textbook on group theory written specifically for
physicists.
2. Rottnan, J.
An Introduction to the Theory
of
Groups, 3rd ed., Allyn and Ba-
con, 1984. An excellent (formal, but readable) introduction to group theory
with many examples and lots of explanations.
3. Wigner, E. Group Theory
and
its Application to the Quantum Mechanics
of
Atomic Spectra, Academic Press, 1959. Another classic written
by
the
master of group theory himself.
