Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


24.2
ORTHOGONALITY
PROPERTIES
683
The
presence
of
the completelyarbitraryoperatorXindicatesthatEquation(24.6)is
a powerful statement about-s-and a severe restriction
on-the
operators
TCa)
(g).
This becomes
more
transparent if we select a basis, represent all operators by
matrices,
and
for X, the matrix representation
of
X, choose a matrix whose only
nonzero element is
I
and
occurs at the lth row
and
mth
column.
Then
Equation
(24.6) becomes
L
T,~a)(g)T:!Y(g-l)
= Alm
8ap8ij,
geG
where Aim is a constant that can be evaluated as follows.
Set
j = i, Cl =
fJ,
and
sum over i,
TheRHS
will give Aim
Li
8ii = Almna, where n
a
is the dimension
of
the carrier space
of
r».
For the LHS we
get
LHS
= L
LT~a)(g)T~I(g-l)
= L (TCa)(g-I)T(a)(g))ml
geG i gEG
= L
T~7)(g-1
g) = L
T~7)(e)
= IG[8
ml,
geG
geG
'-.---'
=(1)ml
where [GI is the order
of
the group. PUlling everything together, we obtain
"Ca)
CP)
-I
[G[
LJ
Til
(g)T
mj
(g )
=
-8
mI8ap
8ij,
gEG
n
u
or
(24.7)
(24.8)
character
01
a
representation;
simple
character,
compound
character
if the representation is unitary.
Equations (24.7) and (24.8) depend on the basis chosen in which to express
matrices. To eliminate this dependence, we first introduce the innportant concept
of
character.
24.2.6. Definition.
Let
T : G
--+
GL(V)
be a representation
of
the group G. The
character
of
this representation is the map X : G
--+
C given by
X(g)
==
trT
g
=
LTu(g),
i
where T(g) is the matrixrepresentation
ofT
g
in any basis
ofV.
1fT
is irreducible,
the character is called simple; otherwise, it is called compound.
The
character
of
the identity element in any representation can be calculated
innmediately. Since a homomorphism maps identity onto identity,
T
e
= 1. There-
fore,
x(e)
=
tr(l)
= dim V. (24.9)

684 24.
GROUP
REPRESENTATION
THEORY
Recall that two elements x, y E G belong to the same conjugacy class if there
exist
g E G such that x =
gyg-I.
This same relation holds for the operators
representing the elements: T
x = TgTyT
rl.
Taking the trace of both sides, and
noting that
Trl
= T
g
1,
one can show that
24.2.7. Box,
All
elements
of
a group belonging to the same conjugacy class
havethesame
character.
Setting i =I and j =m in (24.7) and summing over i and i, we obtain
"(.J
(OJ
-I
IGI" IGI"
L..,X
(g)X"
(g ) =
-8.p
L..,8ji
8
ij
=
-8.p
L..,8jj
=
IGI8.p,
geG
n«
t.i
n.
j (24.10)
-------
=na
or
I:
X(·J(g)X(PJ*(g) =
IGI8.p
geG
(24.11)
if
the representation is unitary. This equation suggests a useful interpretation:
Characters can be thought
of
as vectors in a IGI-dimensional inner productspace.
Accordingto Equation
(24.11), the characters of inequivalentirreduciblerepresen-
tations are orthogonal.
In particular, since there cannotbe moreorthogonalvectors
than the dimension of a vector space, we conclude that the number
of
irreducible
inequivalent representations
of
a group cannot be more that the cardinality
of
that
group. Actually, we can do better. Restricting ourselves to unitary representations
and collecting all elements belonging to the same conjugacy class together, we
write
,
" (.J
(fJJ*
IGI8
L-CiXi
Xi =
af3
;=1
(24.12)
where i labels conjugacy classes, c; is the number of elements in the
ith
class,
r is the number
of
classes in G, and
Ix(·J)
E
<C'
is an r-dimensional vector
with components
ki
/2
xi·J}i~l'
Equation (24.12) shows that vectors belonging to
different irreducible representations are orthogonal. Since there cannot be more
orthogonal vectors than the dimension of a vector space, we conclude that
the
number
of
inequivalentirreducible representations
of
a group
cannot
be more that
the number
of
conjugacy classes
of
the group, i.e., p::::r,
The characters of the adjoint representation are obtained from
X(g)
=
X(g-I)
=}
Xi
=Xi',
where
Kj'
is the class consisting
of
all elements inverse to those of the class
Ki.
The equations involving characters of inverses
of
group elements can be written

24.3 ANALYSIS
OF
REPRESENTATIONS
685
in terms of the characters of the adjoint representation. For example, Equation
(24.10) becomes
L X(·)(g)X(Pl(g) =
IGI8.
p
=}
gEG
,
LCiXi·1x?)
=
IGI8.p.
i=l
(24.13)
Otherrelations can be obtained similarly.
24.3 Analysisof Representations
We can use the results obtained in the last section to gain insight into a given
representation. Take the trace
of
both sides
of
Equation (24.4) and write the result
as
p
X(g)=mlx(ll(g)+
...
+mpx(pl(g)
==
Lm.x(·)(g);
Cl!=1
(24.14)
i.e., a compound character is a linear combination of simple characters
with non-
negative integer coefficients.
Furthermore, the orthogonality
of
simple characters
gives
(24.15)
yielding the number
of
times the irreducible representation
T(·)
occurs in the
representation
T.
Anotherusefulrelationwill be obtained
if
we multiply Equation (24.14) by its
complex conjugate and sum over
g; the result is
L
Ix(g)1
2
= L X(g)X*(g) = L
Lm.X(·l(g)
LmpX(Pl*(g)
geG geG
geG
a
f3
=
Lm.mp
L X(·)(g)X(PJ*(g) =
IGI
Lm~.
"p
gEG a (24.16)
=IGI'.~
In
particular, if T is irreducible, all
m.
are zero exceptfor one, which is unity. We
therefore obtain the
criterion for irreducibility:
criterion
for
irreducibility
,
L
Ix(g)1
2
=L
c
ilxil
2
=
IGI
geG
i=l
if
T is irreducible. (24.17)
For groups of low order and representations
of
small dimensions, Equation
(24.16) becomesa powerfultool for testing the irreducibility of the representation.

686 24.
GROUP
REPRESENTATION
THEORY
24.3.1.
Example.
Let a = 83 and considerthe representation of Exampte24.1.8.The
characters
of the
first
three
elements
of this
representation
are
easily
calculated:
Xl =tr Tj = 3,
X2 =trT2 = I,
X3
ee
tr Tj =I.
Similarly, onecanohtainX4 = I, X5 = 0, andX6 =
O.
Substituting thisinEqnation
(24.16)
yietds
6
L Ix(g)1
2
= L
Ixjl2
= 3
2
+1
2
+1
2
+1
2
+0
2
+0
2
= 12.
geG
j=l
Comparing this with theRHS of (24.16)with 101 = 6 yields
I:am~
= 2. This restricts
the
nonzero
a's to two, saya = 1and
ex
=2.
Moreover,
ml andmz canbe only1.Thus,
the
representation
of
Example
24.1.8 is
reducible,
and
there
arepreciselytwo
inequivalent
irreducible
representations
in it, each
occurring
once.
Wecan
actually
findthe
invariant
subspaces
corresponding
tothetwo
irreducible
repre-
sentations
revealed
above.
The
first
iseasytoguess.Just
taking
thesumofthe
three
functions
tlot2,and t3 givesa one-dimensional invatiantsnbspace; so, let
¢I
sa
tl
+t2 +t3,
andnote
that
thespaceWI
spanned
by
cPt
is
invariant.
Thesecondis
harder
to
discover.
However,
if weassume
that
1/11,
1/12.
and
1/13
areorthonormal,
then
usingtheGram-Schmidt
process, we can findthe othertwo functions orthogonalto
cPt
(butnot orthogonalto each
other!).These are
regular
representation
Thereaderisnrged to convince himselflherselfthatthe subspace
W(2)
spannedby ¢2 and
¢3 is the complement
of
W(l)
[i.e., V =
W(l)
Ell
W(2)j
and that it is invatiantunderall
Tg's. II
A very useful representation can be conslrncted as follows.
Let
G =
{gj
It=l'
and recall that left multiplication
of
elements
of
G by a fixed element gi is a
permutation
of
(gl'
g2,
...
,gm). Denote this permutation by :J(i. Now define a
representation
R ; G
--->
GL(IR
m
),
called the
regular
representation,
by
That this is indeed a representation is left as a problem for the reader. One can
obtain a matrix representation
of
R by choosing the standard basis {ej
lj'=l
ofIR
m
and noting that
Rg;ej
=
en,-l(j),
From such a matrix representation itfollows that
all characters
XR
of
the regular representations are zero except for the identity,
whose character is
xR(e)
= m [see Equation (24.9)]. Now use Equation (24.14)
for
g = e and for the regularrepresentation to obtainm =
I:~=1
mana where n
a
is the dimension
of
the a-th irreducible representation. We can find m
a
by using
Equation (24.15) and noting that only
g = e contributes to the sum:

24.4
GROUP
ALGEBRA
687
In
words,
24.3.2. Box.
The number
of
times an irreducible representation occurs in
the regular representation
is equal to the dimension
of
that irreducible rep-
resentation.
We therefore obtain the important relations
p
xl=
IGI
8
i1 =
Lnaxi
a)
a=l
and
p
IGI=
Ln~,
a=l
(24.18)
group
algebra
defined
where we have assumed that the first conjugacy class is that
of
the identity. For
finite groups
of
small order, the second equation can be very useful in obtaining
the dimensions
of
irreducible representations.
24.3.3.
Example.
A groupof order 2 or 3 has only one-dimensional inequivalent irre-
ducible representations, because theonly way that Equation (24.18)
can
be satisfied for
IG I= 2 or 3 is for all
nO!'s
to be 1. A group
of
order 4
can
have either 4 one-dimensional
or one 2-dimensional inequivalent irreducible representations.
The
symmetric group 83.
being
of
order6, can have 6 one-dimensional, or 2 one-dimensional and
one
2-dimensional
inequivalentirreduciblerepresentations. We shall see laterthat
ifall inequivalentirreducible
representations
of
a group are one-dimensional,
then
the group
must
be abelian. Thus, the
firstpossibility for 83 must be excluded.
III
24.4 Group Algebra
Think
of
group elements as (linearly independent) vectors.
In
fact, given any set,
one
can
generate a vector space by taking linear combinations
of
the elements
of
the set assumedto form a basis. In the case
of
groups
one
gets a bonus:
The
product
already defined on the basis (group elements)
can
be extended by linearity to all
elements
of
the vector space to turn it into an algebra called the
group
algebra.
For G =
{gj
lj'=to
a typical element
of
the group algebra is a =
L:~=t
aigi.
One
can
add two vectors as usual.
But
the product
of
two vectors is also defined:
where
Ok
is a sum involving
a,
and bi-
The
best
way to
leam
this is to see an
example.
688 24.
GROUP
REPRESENTATION
THEORY
24.4.1. Example.
LetG
= S3and consider a =
211"t-311"3+11"5
andb
=
11"2-211"4+311"6'
Then,using
Table
23.1,we
obtain
ab =
(211"1
-
311"3
+
11"5)(11"2
-
211"4
+
311"6)
=
21l'I1l'z
-
41l'1n'4
+61l'pr6 -
3n'31l'2
+
6Jr31f"4
-
91l"31l'6
+
1r51C2
-
2.7Z'57l'4
+
31l'51r6
=~-~+~-~+~-~+~-~+~
=
311"1
-
711"2
-
211"3
-
311"4
+
611"5
+
311"6.
III
Let A be any algebra. As a finite-dimensional vectorspace, we can always find
two proper subspaces ,c1
and,c2
such that A is a direct sum Of,c1 and ,c2. We
write this as
2
A = ,c1 +,c2.
If
we demand that this sum be invariant under left
(right) algebra multiplication, then it is clear that ,c1
and,c2
must be left (right)
ideals,in whichcasewe
write
Now assume
thatA
has an identity1,whichas avectorin A can be decomposed
uniquely as
(24.19)
Y a
EA.
Multiplying these two equations, we obtain
The uniqueness of the decomposition of a now implies that
So, if a
E ,c1, then a2 = 0 and
with a similar result for a E ,c2. Since 11 E ,c1 and 12 E ,c2, we have
(24.20)
idempotent
element
of
algebras
An element a E A that satisfies a
2
= a is called an
idempotent.f
Thus, 11
and 12 are idempotents. Furthermore, they generate
L,
and ,c2, respectively; i.e.,
,c1
=
All
and,c2
= A12 (see the last section
of
Chapter 1).
2Thereasonforchangingto thisnew
notation
is toreserve
EEl
forthefollowing.
3Inthealgebra of
operators,
we calledsuchanelementa
projection
operator.

resolution
ofthe
identity
24.4
GROUP
ALGEBRA
689
24.4.1 Group Algebra and Representations
Group algebrais very usefulfor the construction and analysis of representations of
groups. In fact, we have already used a similar approach in the construction of the
regular representation. Instead of
IR"'
used before,
use
the m-dimensional vector
space
A, the group algebra. Then left-multiplication by a group element g can be
identified with
T~R)
, the operators
of
the regular representation, and the invariant
subspaces of
A become the leftideals of A, and we can write
Moreover, since the identity element
of
the group is the identity element
of
the
algebra as well, the argument at the end
of
the lastsubsection gives the resolution"
e = el +...+e
r
(24.21)
essentially
idempotent
elements
It
is clear that if a
2
=
ua,
then
a/a
will be idempotent. So, we
can
essentially
ignore the constant
a, which is why a is called essentially
idempotent.
Now
consider the element
of
the gronp algebra
p=I>
XEG
and note that gP =
LXEG
gx =
P.
It
follows that
p
2=
LgLx=
LLg
x=
L
P=
IGIP.
geG xeG geG xeG
geG
(24.22)
primitive
idempotents
So, P is essentially idempotent. Furthermore, the reader may verify that the ideal
generated by P is one-dimensional.
Anidempotentthat cannotbe resolved into otheridempotents satisfying Equa-
tion (24.21) is called a
primitive
idempotent. The reader may check that the
following holds.
24.4.2. Proposition.
A left ideal is minimal if and only if it is generatedby a
primitive idempotent.
Let
us now apply the notion of the group algebra to derive further relations
aroong characters. Denote the elements
of
the
ith
class KI of G by
{x?)lf::l
and
constructthe elementof the group algebrae,
'"
Lf::1
x?J.
If
in the product
oftwo
such quantities
ci Cj
'"
'"
(I)
(j)
Ki/(j
=
L...J
L..J
Xl X
m
,
1=1
m=l
(24.23)
4Inthe
algebra
of
operators,
wecalled
elements
satisfying these
relations
orthogonal
projection
operators.

690 24.
GROUP
REPRESENTATION
THEORY
x?)
x;;p
sa Y E G, is in a certain conjugacy class, then the rest
of
that class can be
obtained by taking all conjugates of y, i.e., elements of G that can be written as
It
follows that
if
one member
of
a class appears in the double sum of Equation
(24.23), all members will appear there. The reader may check that
if
y occurs k
times in the double sum, then all members
of
the class of y occur k times as well.
Collecting all such members together, we can write
r
KiKj
=
LCijIKl,
1~1
(24.24)
where
Cijl
arepositive
integers.
Now consider the
ath
irreducible representation, and add all operators corre-
sponding to a given class:
r
r
(a)
=
"r(a)
~
r(a)r(a) -
"c
.. r(a)
i - L.J g
--r
t j - L."
ijl
I '
geKj
[=1
(24.25)
where the second equation follows from the same sort
of
argument used above
to establish Equation (24.24). One can show that ria) commutes with all r1
a).
Therefore, by Schur's lemma, ria) = Ai
a
)1, and the second equation in (24.25)
becomes
,
Aia)Aja) =
Lc;jIA!a)
1~1
(24.26)
Taking the characters
of
both sides of ria) = Ai
a
)1 and using the first equation in
(24.25), noting that all elements
of
a class have the same character, we get
Substituting this in Equation (24.26), we obtain
,
CiCjxtt)xJct)
= n«
LCij1CiXl(Cl).
1=1
(24.27)
This is another equation that is useful for computing characters. Note that this
equation connects the purely group properties (c; 's and
Cijl
's) with the properties

24.4
GROUP
ALGEBRA
691
of
the representation (xia),s and na). Summing Equation (24.27) over a and using
the first equation in (24.18), we get
p r p
'"
(a) (a)
'"
'"
(a)
CiC)
L-Xi
Xj =
L-CijlCJ
L-naXI
ct=l
[=1
a=l
'---,,-'
=IGlolI
by (24.18)
= CfjllGI
because CI = 1 (there is only one element in the class of the identity). Problem
24.12 shows that
Cijl
= Ci[,i' j where
Kjl
is the class consisting of inverses of
elements of
Ki,
It
then follows that
(24.28)
For a unitary representation,
x5
a)
=
xi
a)*,
so Equation (24.28) becomes
(24.29)
where
IXi) E
iC
P
is a p-dimensional vector with components
{Xia)}~=I'
This
equation can also be written in terms of group elements ratherthan classes. Since
xi
a)
= X(a)(x) for any x E Ki, we have
i:
X(a)(x)X(a)*(y) =
1~~18(K:,
K~),
a=l
x
(24.30)
character
table
ofa
finite
group
where
K:
is the conjugacy class of G containing x, IK:I is the number
of
its
elements,
and
Equation (24.29) shows that the r p-dimensional vectors
xi
a
)
are mutually
orthogonal; therefore, r
::::
p. The statementafter Equation(24.12) was
thatr
2: p.
We thus have the following:
24.4.3.
Theorem.
The number
of
inequivalent irreducible representations
of
a
finite group is equal to the number
of
conjugacy classes in the group.
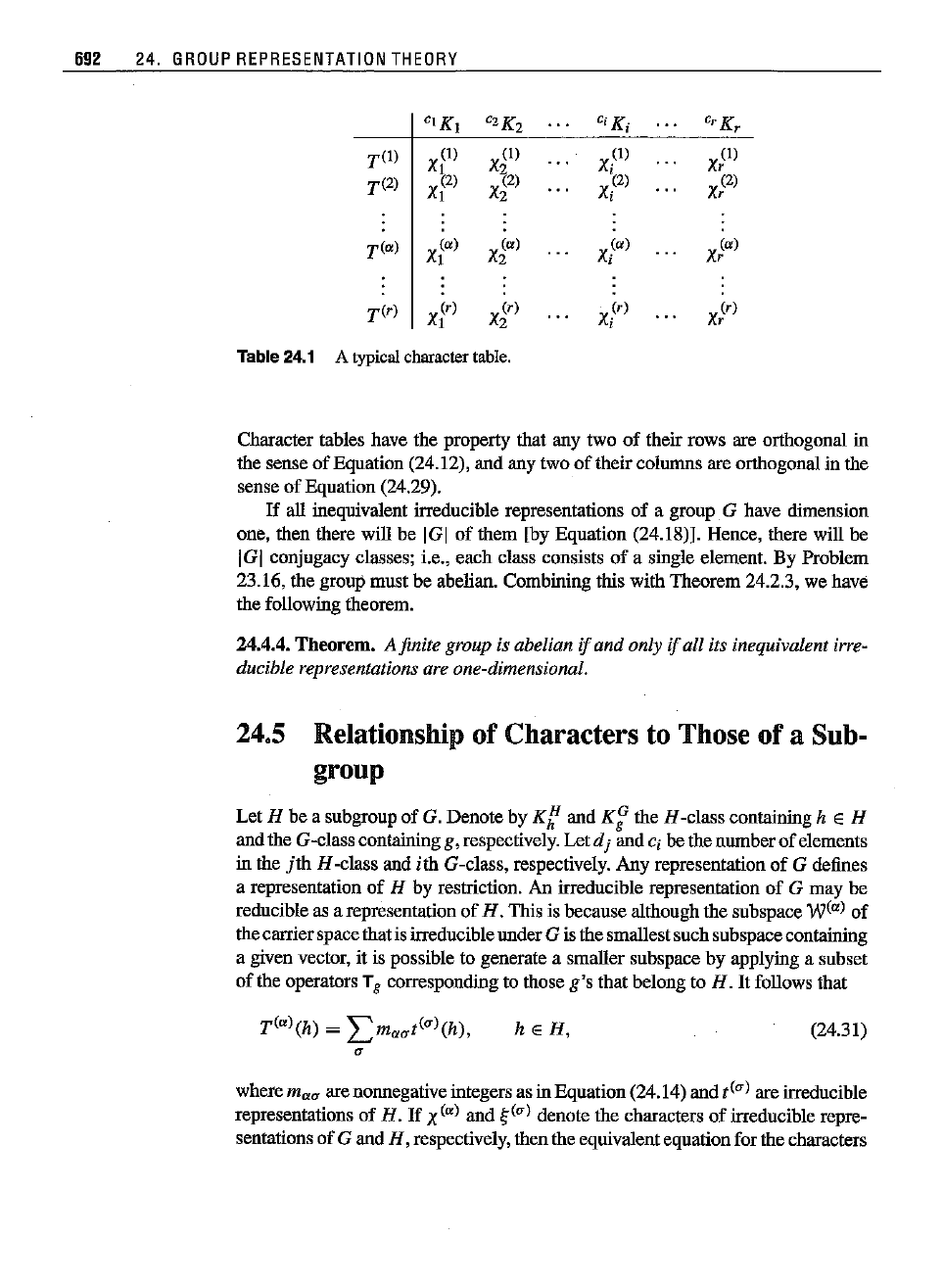
It is convenient to summarize our result in a square table with rows labeled by
the irreducible representation and columns labeled by the conjngacy classes of G.
Then on the
ath
row and ith colunmwe list
xi
a)
,
and we get Table 24.1, calledthe
character
tahle
of G. Note that Ci, the order of
Ki,
is written as a left superscript.
692 24.
GROUP
REPRESENTATION
THEORY
«x,
c2K2
«x,
C
r
K,
T(I)
(I)
(I)
(I) (I)
Xl
X~2)
Xi
X,
T(2)
(2)
(2)
(2)
Xl X2 Xi
X,
T(a)
(a)
(c)
xi
a
)
(a)
XI
X2
X,
T(r)
(,)
(,)
(,.)
(r)
XI X2 Xi
X,
Table24.1 Alypicalcharacter table.
Character tables have the properly that any two
of
their rows are orthogonal in
the sense
of
Eqnation (24.12), and any two
of
theircolumns are orthogonal in the
sense of Eqnation (24.29).
If
all inequivalent irreducible representations of a group G have dimension
one, then there will be IGI of them [by Equatiou (24.18)]. Heuce, there will be
IGI conjugacy classes; i.e., each class consists
of
a single element. By Problem
23.16, the group must be abelian. Combining this with Theorem 24.2.3, we have
the following theorem.
24.4.4.
Theorem.
A finite group is abelian if
and
only ifall its inequivalent irre-
ducible representations are one-dimensional.
24.5 Relationship of Characters to Those of a Sub-
group
Let H be a subgroup of G. Denote by
Kf!
and
Kf/
the H-class containing h E H
and the G-class containing g,respectively. Let dj and ci be the number
of
elements
in the
jth
H -class and
ith
G-class, respectively. Any represeutation
of
G defines
a representation
of
H by restriction. An irreducible representation of G may be
reducible as a represeutation
of
H.
This is because although the subspace
w(a)
of
the carrierspace that isirreducibleunder G isthe smallestsuch subspacecontaining
a given vector, it is possible to generate a smaller subspace by applying a subset
of the operators T
g
corresponding to those
g's
that belong to
H.
It
follows that
T(a)(h)
=
Lma,,-t("-)(h),
o
h e H,
(24.31)
where
m
a,,-
are nonnegative integers as in Equation (24.14) and
t("-)
are irreducible
representations of H.
If
X(a) and
~(,,-)
denote the characters ofirredncible repre-
sentations of G and
H,
respectively, then the equivalentequationfor the characters
