Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


Sylvester's
theorem
Orthonormal
bases
give
thesame
oriented
volume
element.
25.4
INNER
PROOUCT
REVISITED
753
25.4.9.
Theorem.
Forevery symmetricbilinearformg on
V,
there is an orthonor-
mol
basis. Furthermore;
n+o
n_,
and
no are the same in all orthonormal bases.
For
a
proof
of
the last statement, also
known
as
Sylvester's
theorem,
see
[Bish 80, p. 104]. Orthonormal bases allow ns to speak
of
the oriented volume
element. Suppose
leilf=1 is an oriented basis
of
V*.
If
IcI'}f~1
is another or-
thonormal basis in the same orientation
and
relatedto lei} by a matrix R, then
<pI
/\
<pz
/\
...
/\ <pN =
(detR)el
/\ eZ
/\
...
/\
eN.
Since
l<pk}
and lei} are orthonormal, the determinant
ofg
is
(_I)n-
in
both
of
them.
Problem
25.17 then implies that (det R)z = I or det R = ±1. However,
l<pk}
and
lei} belong to the same orientation. Thus,
detR
= +1, and
IcI'}
and
lei} give the same volume element.
25.4.10.
Example.
Let V =
lll.3
and vi =
(XI>
YI,
ZI), vz =
(xz,
YZ,
zz),
and v =
(x, Y, z). Definethe synunetricbilinearform
9(VI, vz) = !(X1YZ
+xZYI
+YIZZ
+YZZI
+xIZZ
+XZZI)
so
that
g(v, v) = xy-l-yz-l-xz, Wewishto
find
asetofvectors
in]g3
that
areorthonormalwith
respectloIbisbilinearfonn. Clearly, et = (I, 1,0) issuchthatg(el,
el)
= 1.Soej isoneof
ourvectors.Considerv = (1,0, I) andnotethatthevector
rj
=
v-
[g(v,
el)/g(el,
el)lel>
suggestedby the Gram-Schmidt process, is orthogonal to
ej
.
Furthermore,
it is easily
verifiedthatg(r2. r2)
= -i-Therefore, oursecondvectoris
rZ
(I
3 2)
02 = "Ilg(rz, rz)1 = -
-"IS'
-
-"IS'
-"IS
with g(eZ,02) =
-1.
Finally,we take w =(0, I, I). Then
r3
=w - g(w,
el)
ei
_ g(w, 02)02 = 4
(-3,
I, I)
g(el>
el)
g(02,ez)
III
will
be orthogonalto both
ej
and 02with g(r3, r3) =
-~.
Thus, the !birdvector can be
chosentobe
r3
(3
I I)
e3 = v'lg(r3,
r3)1
= -
-"IS'
-"IS'
-"IS
,
and we obtaing(el,
el)
= I, g(eZ,02) =
-I,
g(e3' e3) =
-I,
g(e" ei) = °for i
i'
i-
Wealsohaven+ = 1,n_ = 2, and
no
= 0, i.e., theindexof 9 is 2, audits
signature
is
-1.
Although
we have
worked
in a
particular
basis,
Theorem
25.4.9
guarantees
that
n+. n_.
and
no
are
(orthonormal)
basis-independent. II
We should emphasize that the invariance
of
n+, n_,
and
no is true for g-
orthonormalbases. As a counterexample, considerg
of
Example 25.4.10 applied
to the standardbasis
of
R
3
, which we designate
with
a prime.
It
is readily verified
that
g(e;,
e;) = 0 for i =
1,2,3.

754 25.
ALGEBRA
OF
TENSORS
So it
might
appear
that no = 3
for
this basis. However, the
standard
basis
is not
g-orthonormal. In fact,
(
'
')
1
('
')
('
')
9
el,e
Z
=
'2
=g
el,e3
=g
e
Z,e3'
That
is
why
the
nonstandard
vectors
ei,
v,
and
w
were
chosen
in
Example
25.4.10.
In an
orthonormal
basis
the
matrix
of
9 is diagonal,
with
entries +
I,
-I,
and
O.
In particular,
if
9 is to be nondegenerate,
that
is, to be an
inner
product, no
must
be zero.
Thus,
a general
inner
product
on
an
N-dimensional
vector
space
satisfies
the
conditions n+ +
n_
= N
and
n+ -
n_
= s
which
give s = N -
2n_.
25.4,11.
Definition.
An inner productspace with n.: = I or n: = N - I is called
a Minkowskispace. For N
= 4 this is the space
of
the special theory
of
relativity.
An inner product space with n.:
= 0 (or
n_
=
N)
is called a Euclidean space.
25.4.12.
Example.
Let
{eil~1
be a basis of Vand
{ei}~l
its dual. We can define the
permutation
tensor
permutation tensor:
~ili2
.•.iN it i2
iN(
)
"'J'
.
=e
Ae
A···Ae
e
J'l,e
J'2,···,ejN·
it
2···JN
(25.20)
III
It is clear from this definition that
D)i,Il)'?
••i
J
,! is completely skew-symmetric in all
upper
1 2
...
N
indices.
That
it is also skew-symmetric in the
lower
indices can be
seen
as follows.
Assume
that two of the lowerindices are equal. Thismeans baving two ej 's equal in (25.20).These
two ej 's will contract with two e
i
'5, say e
k
and e
l
. Thus, in the expansion there will be a
term
C.!'(ej).f(ej),
where C is the product of all the other factors. Since the product is
completely skew-symmetric in the
upper
indices, there
must
also exist another term, with a
minus sigo and in which the upper indices k and I are interchanged:
-Cet(ej).f«ej)'
This
makes the sum zero, and by Theorem 25.2.7, (25.20) is antisymmetric in the lower indices
as well.
This suggests that
~i.li~
...i!f ex Eiliz...iNE"
'.
Tofind the proportionality constant
}I1Z
...
}N
JI1Z
..
·}N
'
we note that (see Problem 25.16)
,12 N "
,I
,2
,N
"12 N =
L.,
E,,(I),,(2)
...
,,(N)",,(I)",,(2)
...
",,(N)'
zr
The only contributionto the sum comes from the permutation with the property n(O =i,
Thisis the identity permutationfor which
Err
= 1. Thus, we have
~g:::~
= 1. On the other
hand,
e
I2
...
N
EI2...N = E
I2
...
N
=
(-1)"-.
Therefore, the proportionalityconstant is
(_l)n-
. Thus
Eitiz
...
iNE"
. _
(_l)n_~iliz
iN
}lJ2
..
·}N
-
hh
ts
We
can
lind an explicit expression for
the
permutation
tensor
of
Example
25.4.12.
Expanding
the
RHS
of
Equation
(25.20)
using
Equation
(25.10), we obtain
(25.21)
III
25.4
INNER
PRODUCT
REVISITED
755
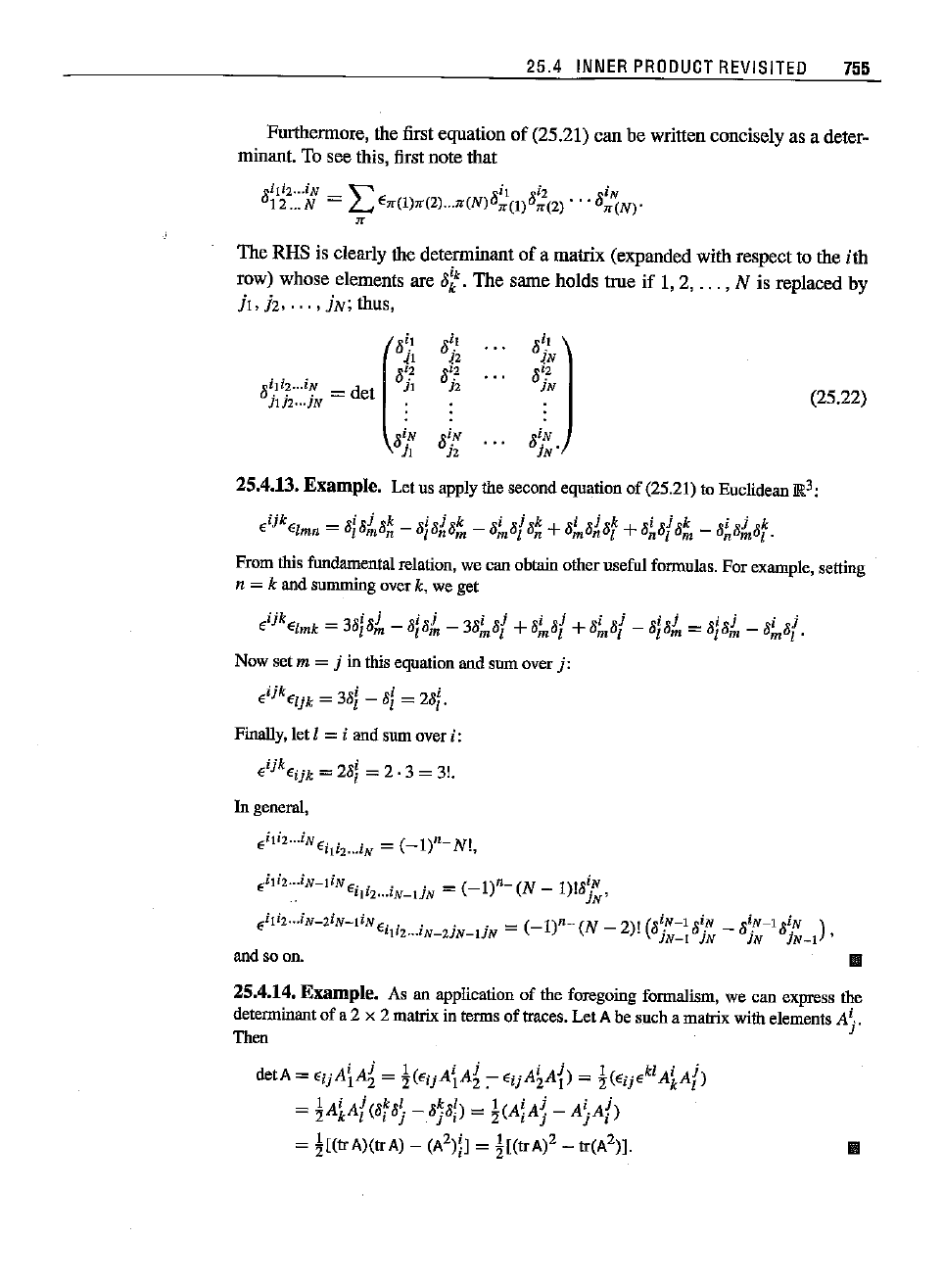
Furthermore, the first equation
of
(25.21)
can
be written concisely as a deter-
minant. To see this, first note that
~ili2
iN
~
i'ir
I'!iz
'il:iN
"12
N = L...,E"CI)"C2)..."C
N)""CI)""C2)
...
""CN)·
n
The RHS is clearly the determinant
of
a matrix (expanded withrespect to the
ith
row) whose elements are
81'.
The same holds true if
1,2,
...
, N is replaced by
h,
n. ...
.i»,
thus,
["
s:
,"
J
;1 ;2
;N
..
. f/?
{/~
8l~
8lIl2
... IN _ d t
it
J2
IN
(25.22)
...
- e
JIl2···}N :
f/
N
f/N
(/N,
n
J2
IN
25.4.13.
Example.
Letus applythesecondequationof (25.21) to Euclidean
lll.3:
ijk
,i
,j
,k ,i
,j
sk
,i
,j
,k +
,i
,j
sk + ,i
,j
sk
,i
,j
,k
€ €lmn = UtUmUn - VI
"nvm
- UrnOt "n UmUnUt unul Urn -
UnUmUt"
From this fundamental relation, we can obtain other useful formulas, For example, setting
n = k and summingover k, we get
ijk
_
3,i,j
,i,j
3,i
,j
+,i
,j
+,i
,j
,i,j
_
,i,j
,i
,j
€ €lmk -
ulum
-
ulum
- UrnUt UrnOt UrnUt -
CJlf)m
-
ulum
- umoZ .
Nowset m = j in this equation and sum over
j:
cik
...
ell
fljk
= 38i - 8i = 28i.
Finally, let1=i andsumoveri:
··k
.
ell
€ijk
= 28; =2 .3 =
31.
In general,
eitiz
...
iNe··
. -
(_I)n-NI
IPZ···IN
- .,
eiliZ
..
.iN-liNe·
. . -
(-l)n-(N
_l)ISiN
.' 1I
12···
IN_llN
- •
iN'
(/liz
•..
iN_2
iN_liN
E"
, . . =
(-l)n-(N
_ 2)1
(f/N-18j~
_ 8
i
!i-
1
si,
N )
llI2···
lN_21N_IlN
IN-1
IN
IN
IN-l'
~OO~
•
25A.14.
Example.
As an application of the foregoing
formalism,
we can
express
the
determinant
ofa
2 x 2 matrixin tenus of traces.LetA be sucha matrix withelements
A~.
Then
detA
=
EijA\
A~
=
!(EijA\
A~
:-
EijAA{)
=!(EijEkZ
A~A{)
_lijkl
kl_l
ij
jj
-
2AkAZ(8i8j
-8
j8
i)
-
2(A
iA
j
-AjA
i)
=
![(trA)(lrA)
- (A
2
)iJ=
![(lrA)2
_tr(A
2)].

756 25.
ALGEBRA
OF
TENSORS
We can generalize the result
of
the exampleabove and express the determinant
of an
N x N matrix as
(25.23)
(25.24)
25.5 The Hodge Star Operator
It
was established in Chapter 2 that all vector spaces of the same dimension are
isomorphic (identical). Therefore, the two vector spaces
AP(V)
and
AN-p(V)
having the same dimension,
(~)
=
(N~)'
mustbe isomorphic. In fact, there is a
natural isomorphism between the two spaces:
Hodge
star
operator
25.5.1. Definition. Let 9 be an inner product and
[e;}~1
an ordered g-
orthonormal basis
of
V.
The Hodge star operator is a linear mapping, *
AP(V)
-->
AN-P(V),
given by (remember Einstein's summation convention!)
( )
I
ip+l
...iN
* Cit
/\
...
A ei
p
es (N _ p)!f:
i1
..
.i
p
ei
p
+l
/\
...
A
eiN'
where all the indices
of
the < tensor are raised bygij.
Although this definition is based on a choice
of
basis, it can be shown that the
• . s:
b"
de d m th
ip+l
..
.iN
± be
operator
IS
In
ract asis-m pen ent. we note at E
il
...
fp
;;;;;;
Eit..
.ipip+t
...iN -
cause
each
index raisingintroduceseithera +
lora
-1.
In particular, for Euclidean
spaces, in which
n_ = 0, the two epsilon symbols are the same.
25.5.2.
Example.
Let us appty Definition 25.5.t 10 AP(R3) for p = 0,
1,2,3.
Lei
let. e2. e3}bean
oriented
orthonormal
basis
ofR
3.
(a)ForA
O(R3)
=
JR
abasisis I, aod (25.24)gives
I
"k
*1 =
-E
lj
ej
1\ e
J
' 1\ ek =
ei
1\
ez
A eg,
3!
(b) For A
I(R3)
=
JR3
a basis is {e" e2,e3), aod (25.24)gives*ei =
-ir.(k
ej
/\ ek-
or
*el
= e2 1\ eg, 'sez = e3 A CI, *e3 =
ei
A e2·
(e)For A
2(JR3)
abasisis
[e1/\
e2,
ej
A e3,e2 /\ eg}, aod(25.24)gives
.ei
/\ ej =
<tek'
or
.(e1/\
02)= eg,
.(el/\
e3) =
-e2,
*(e2/\ e3) =
el·
(d)ForA
3(R3)
abasisis Iei /\ e2/\ eg], aod (25.24)yietds
III
The preceding example may suggest that applying the Hodge star operator
twice (composition
of
* with itself, or * 0 *) is equivalent to applying the identity
operator. This is partially troe. The following theoremis aprecise statementof this
conjectme. (For a proof, see [Bish
80, p.
Ill].)

antlsyrnmetnc
tensors
with
numerical
coefficients
Cross
product
is
defined
onlyinthree
dimensions!
25.5
THE
HOOGE
STAR
OPERATOR
757
25.5.3.
Theorem.
Let V be an oriented space with an inner product g. For A E
AP(V),
we have
* 0 *A '" * *A =
(-Ij"-(-I)p(N-P)A,
(25.25)
where n.: is the index
oig
and N = dimV.
In
particular,
for
Euclidean spaces with an
odd
number
of
dimensions (such as
1R
3
),
**A = A.
One
can
extend the
star
operation to
any
A E AP (V) by writing A as a linear
combination
of
basis vectors
of
AP(V)
constructed
out
of
{eil~I'
and
using the
linearity
of
*.
Io
the
discussion
of
exterior algebra
one
encounters sums
of
the form
Ait··.i
pv
·
A···
/\
v'
II
I
p
'
It
is important to keep in
mind
that
Ail".i
p
is
assumed
skew-symmetric.
For
ex-
ample,
if
A = ei /\ ez,
then
in thesumA = A
ij
ei A.ei- the
nonzero
components
consist
of
A12 = !
and
A
21
= -!.Similarly,
when
B =
ei
1\ e2 1\ e3 is written in
the form B
=
Bijk
ei
1\ ej 1\ ek, it is understood
that
the nonzero components
of
B
are
not
restricted to B
123.
Other
components,
such
as B 132,B
231,
and
so
on,
are
also
nonzero.
In fact,we have
B
123
=
_B132
=
_B213
= B231 = B
312
=
_B
321
=
~.
This
should be
kept
in
mind
when
sums
over
exterior
products
with
numerical
coefficients are
encountered.
25.5.4.
Example.
Let a,
bE
]R3
and (eloe2, e3}an oriented orthonormal basis of]R3.
Then
a =
aiel
andb =
biej.
Letus
calculate
a Ab and*(a Ab). We
assume
a Euclidean
9 on
1R
3.
Thena
A b = (aief) A
(hiej)
= albici 1\
ej.
and
*(a
1\ b) =
*(aiei)
1\
(,)
ej)
=a
i,)
* (ei 1\
ej)
=a
i,)
(Etek) = (Efja
i
,))ek.
Weseethat«(aAb) is avectorwithcomponents [*(3Ab)]k =
Etaibi,
whichareprecisely
the
components
of
ax
b.
The
correspondence
between
a A b anda x b holdsonlyin three dimensions,
because
dbnA
1(\7)=dbn A
2(\7)
onlyifdim \7=3.Thatiswhytbecrossproduct canbe
defined-
as a
"machine"
that
takes
two
vectors
in V and
manufactures
a vector in
V-only
if
V is
three-dimensional.
II
25.5.5.
Example.
Wecan use tbe resnlts of Examples
25.4.13
and
25.5.4
to establish a
sample
of
familiar
vector
identities
componentwise.
(a)Forthe
triple
cross
product,
wehave
[a x (b x c)]k =
Etai
(b x
c)j
=
Etai
(4mbl
em) =
aibl
c"l
E
kij
EjIm
b
i m kij
b1c"'(,k,i
,koi)
=ai e E EImj =ai 01
om
- 0mOI
=
aibkci
-
aibick
=
(a'
c)b
k
-
(a-
b)c
k,

758
25,
ALGEBRA
OF
TENSORS
whichis the kth componentof b(a . c) - c(a ' b). In derivingthe above"bac cab"rule, we
usedthefactthatonecanswapan
upper
indexwiththesamelower
index:
a
i
hi = ai b
i
(b) Next we showthe
familiar
statement
that
thedivergence of curlis zero.Let 8i denote
differentiation
with
respect
to Xi. Then
V.
(V
x a) =
ai(V
x
ali
=
aif~kajak
=
fijk8i8jak
'~
.~
'~
F
_El'
Bj8jak
=
-El!
8j8jak
=
-8j(E
j l
BiOk)
=-a
/V
x
a)j
= - V . (V x a)
=}
V,
(V x a) = 0,
Theabovestepsshowin
general
that
25.5.6.Box. When the product
of
two tensors is summed over a pair of indices in
whichoneofthetensorsis symmetricandtheotherantisymmetric, theresultiszero.
(c)
Finally,
we show
that
curlof
gradient
is
zero:
[V x (V
f)]k
=
f~k8j8k
f =
fijk8jakf
= 0,
•
becauseEijk
is
antisymmetric
in
jk,
while
8j8kf
is
symmetric
in
jk.
25.6 Problems
III
25.1. Show that the mapping v : V*
--+
~
given by v(T) = T(V) is linear.
25.2. Show that the components
of
a tensor product are the products
of
the com-
ponents
of
the factors:
25.3. Show that eh
181
181
ej,
181
€il
181
...
181
€i, are linearly independent. Hint:
Consider A
J,,')
..
,j,
e
J
')
181 181
e
J
',
181
€il
181
..
'
181
€i, = 0
and
evaluate the LHS on
1···l
s
appropriate tensors to show that all coefficients are zero.
25.4.
What
is the tensor product
of
A = 2e
x
- e
y
+3e
z
with
itself?
25.5.
If
A E .G(V) is represented by
A~
in the basis {eil
and
by A;k in
{ep.
then
show that
A'ke'
iO\
e'l - Ai e. 101 ""j
1 k'O' - j
I'O'~'
where
{€j}
and
{e'l}
are dual to Ie;} and
{ep,
respectively.
25.6. Prove that the linear functional F : V
--+
~
is a linear invariant. i.e., basis-
independent, function.

25.6
PROBLEMS
759
25.7. Show that tr :
'Ji
-->
]R
is ao invariaot linear function.
25.8.
If
Ais skew-symmetric in some pair
of
variables, show that S(A) =
O.
25.9. Using the exterior prodnct show whether the following three vectors are
linearly dependent or independent:
VI
=
2el'-
e2
+3e3
- e4,
V2
=
-ret
+
3~
- 2e4,
V3
= 3el + 2e2 - 4e3 + e4.
25.10. Let A E 'J(j(V) be skew-symmetric. Show that
if
r
l
,
...
,r'
E V* are
linearly dependent, then
A(r
l
,
...
, T'") =
O.
25.11. Show that (ek A e.} with k < i are linearly independent.
25.12. Let v E Vbenonzero, aod let A E
AP(V). Show that v AA = 0
ifaodonly
if
there exists B E A
p-I
(V) such that A = v A B. Hint: Let v be the first vector of
a basis; separate out v in the expaosion
of
Ain terms of the
p-fold
wedge products
of
basis vectors, aod multiply the resnlt by v.
25.13. Let A E A
2
(V) with components
A
ij
. Show that A AA = 0
if
.and only if
Aii
A
k1
_ A
ik
Ai
i
+
Ail
Ai
k
= 0 for all i,
j,
k, I in aoy basis.
25.14. A linear operator acting on a vector in one dimension simply multiplies
that vectorby some constaot. Show that this constaot is independent of the vector
chosen. That is, the constaot is ao intrinsic property
of
the operator.
25.15. Let {el, e2, e3} be aoy basis in
]R3.
Define ao operator E :
]R3
-->
]R3
that permutes aoy set of three vectors {VI,
V2,
V3}
to {Vi,
vi,
Vk}. Find the matrix
representation of this operator aod show that det E =
'ijk.
25.16. (a) Starting with the definition of the permutation tensor
8Jil'J·2
..
.iJN
, aod
I 2·" N
writing the wedge product in terms of the aotisynnnetrized tensor product, show
that
(b) Show that the inverse of the (diagonal) matrix
of
gin
ao orthonormal basis is
the same as the matrix of g.
(c) Now show that .12...N =
(-I)'-'12
...N =
(-1)'-.
25.17. Let{edf:" be a g-orthonormal basis
ofV.
Let
~ii
=
±8}
be the matrix of
9 in this orthonormal basis.
Let
{Vi Jf=1 be aoother (not necessarily orthonormal)
basis of V with a traosformation matrix R. Using G to denote the matrix
of 9 in
{Vi},
show that
det G
= det 7](detR)2 =
(-1)'-
(det R)2.

760 25.
ALGEBRA
OF
TENSORS
In particular, the sign of this determinant is invariant. Why is det G not equal
to det
'I)?
Is there any conflict with the statement that the determinant is basis-
independent?
25.18. Show that the kemel of 9 : V --> V* consists of all vectors u E V such that
g(u, v) = 0 for all v E V. Show also that in the g-orthonormal basis leiJ, the set
lei Ig(ei, ei) =
OJ
is a basis
of
kerg,
and therefore
no
is the nullity of g.
25.19. Use Equation (25.23) to show that for a 3 x 3 matrix A,
25.20. Show that a 2-form w is nondegenerate if and only
if
the determinant of
(wii)
is nonzero
if
and only if w
b
is an isomorphism.
25.21.
Let
V be a finite-dimensional vector space and w E A
2
(V*). Suppose there
exist a pair
of
vectors
ej
, ei E V such that
w(e\,
ei)
i'
O.
Let
p\
be the plane
spanned by e\ and
ei,
and V\ the w-orthogonal complement of Pi- Show that
V\ n p\ = 0, and that v - w(v,
eiJe\
+w(v,
e\)ei
is in VI.
25.22. Show that
Lj~\
.1
1\
.i+
n
,
in which
l.iJ7=\
is dual to
led!",\,
the canon-
ical basis of V,has the same matrix as w.
25.23. Suppose that v, Vi E Vare expressed in a canonical basis of V'with coeffi-
cients {Xi, Yi, Zi} and{x;,
y;,
z~}.
Show that
n
w(v, Vi) =
~)XiY[
- X[Yi).
i=l
25.24.
Let
V be a vector space and V* its dual. Define w E A
2
(V
Ell
V*) by
w(v
+
<p,
v+
<p')
sa
<p'
(v) -
<p(v
')
where v,
Vi E V and
<p,
<p'
E V*. Show that (V
Ell
V*,
w)
is a symplectic vector
space.
25.25. By taking successive powers of w show that
n
w
k
= L e
it
1\ e
h
+
n
1\
...
/\
e
ik
/\
e
A
+
n.
it
...
jk=l
Conclude that
where
[n/2]
is the largest integer less than or equal to
n/2.

25.6
PROBLEMS
761
25.26. Show that the conditionfor a matrix Ato be symplectic is AtJA = J where
J
=
(~I
A)
is the representation of w in the canonical basis.
25.27. Show that
Sp(V, w) is a snbgroup of
GL(V).
25.28. Find the index and the signature for the bilinear form 9 on
~3
given by
g(VI, V2) = XI)'2
+X2YI
- YIZ2 - Y2ZI·
25.29. In relativistic electromagnetic theory the currentJ and the electromagnetic
field tensorF are, respectively, a four-vector" and an antisymmetric tensor of rank
2.
Thatis,J
=
Jk
ek
and F =
Fijei
/\ej.
Find the components
ohJ
and *F. Recall
that the space
of
relativity is a 4D Minkowski space.
25.30. Show that where there is a sum over an upper index and a lower index,
swapping the upper index to a lower index, and vice versa, does not change the
sum. In other words,
Ai
B,
=
Ai
B
i
.
25.31. Show the following vectoridentities, using the definition of cross products
interms of
Eijk.
(a) A x A
=0.
(b)
V·
(A
x B) =
(V
x
A)·
B -
(V
x
B)·
A.
(e) V x
(A
x B) = (B .
V)A
+
A(V
.B) -
(A·
V)B
-
B(V
.
A).
(d) V x
(V
x A) =
V(V·
A) - V
2A.
25,32. A vector operator Y is defined as {yl, y2, y3}, a set of three opera-
tors, satisfying the following commutation relations with angular momentum:
[yi,
Jj]
=
i.ijkYk.
Show that
ykYk
commutes with all components of angular
momentum.
25.33. The Pauli spin matrices
I
(0
I)
0"=10'
3 =
(I
0)
0"
0-1
describe a particle with spin tin nonrelativistic quantum mechanics. Verify that
these matrices satisfy
[ui,u
i
]
=uiu
J
--'-uJa
i
=2iE~ak,
{ui,uJ}=uia
J
+aJa
i
=2B~12'
where 12is the unit 2 x 2 matrix. Show also that
O"i
O"j =
i.~
O"k
+
8}
12,and for
any two vectors a and b,
(0""
a)(O"'·b) =
a-
b12 +
iO"'·
(a x b).
25.34. Show that any contravarianttensor
of
rank two can be written as the sum of
a symmetrictensorand an antisymmetrictensor. Can this be generalizedto tensors
of arbitrary
rank?
6It
turns
outto bemore
natural
to considerJ asa 3-form.However,sucha finedistinctionis not of anyconsequenceforthe
presentdiscussion.

762 25.
ALGEBRA
OF
TENSORS
Additional Reading
1. Abraham, R., Marsden, J. and Ratiu, T. Manifolds, Tensor Analysis, and
Applications,
2nd ed., Springer-Verlag, 1988. A comprehensivetextbookon
tensors with many examples drawn from physics.
2. Bishop, R. and Goldberg, S.
Tensor Analysis on Manifolds, Dover, 1980.
This little and lucid book is one of the earliest ones on index-free tensor
analysis.
3. Flanders, H.
Differential Forms with Applications to Physical Sciences,
Dover, 1989. One ofthe firstbooks on exterioralgebrawritten for physicists.
It
has many examples drawn from various areas of physics.
