Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations

Подождите немного. Документ загружается.

26.2

CURVES

AND

TANGENT

VECTORS

773

The operator!is an abstraction

the derivative operator. Note

thatt(f),

(P),

f(P),

and t(g) are all real numbers.

The reader may easily check that ifadditionand scalar multiplicationoftangent

tangent

space

vectors are defined in an obvious way, the set of all tangent vectors at P E M

defined

becomes a vector space, called the

tangent

space

at P and denoted by 'Jp

(M).

U is an open subset

M (therefore, an open submanifold

M),

then it is clear

that

'Jp(U)

'Jp(M)

foraliPEU.

(26.4)

Definition 26.2.3 was motivated by Equations (26.2) and (26.3).

Let

us go back-

wards and see

(26.2) is indeed a tangent, that is, if it satisfies the two conditions

of Definition 26.2.3.

vectors

tangentto a 26.2.4. Proposition.

Let

y be a e

curve in M such that

y(c)

= P. Define ;Y(c)

curve

d I

(J(c))(f)

sa du f 0 Y

u~c

for every f E

FOO(P).

Then;Y(c) is a tangentvectorat P calledthe vector tangent

to y

ate.

Proof

We have to show that the two conditions of Definition 26.2.3 are satisfied

for f, g E

FOO(P)

and 01,

E R The first condition is trivial. For the second

condition, we use the product rule for ordinary differentiation as follows:

did

(J(c))(fg)

= du

(fg)

0 Y

u~c

;;: du

[(f

y)(g

0 y)]

u~c

[:u

y)luJ

(g 0

Y)u~c

[:u

(g 0

y)luJ

[(;y(c»(f)]g(y(c))

+f(y(c))[(;Y(c))(g)]

[(;Y(c»(f)]g(P)

+f(P)[(;Y(c))(g)].

Note that in going from the first equality to the second, we used the fact that by

definition, the product

two functions evaluated at a point is the product of the

values of the two functions at that point. D

Let us now consider a special curve and corresponding tangent vector that is

of extreme importance in applications.

Let

••.

x'")

be a coordinate

system at

where xi : M

--+

Ris the

ith

coordinatefunction. Then

is abijective

mapping from the manifold

Minto

Its inverse, <p-l : Rm

--+

is also a

mapping. Now,the

ith

coordinate

Pis

therealnnmberu

xi(P).

Suppose

that all coordinates of

P are held fixed exceptthe

ith

one, which is allowed to vary

with u describing this variation.

774

26.

ANALYSIS

TENSORS

coordinate

curve,

coordinate

vector

field,

and

coordinate

frames

26.2.5.Definition. Let (Up, rp)beachartatP

EM.

Thenthe curve y' :

-+ M,

defined by

yi (u) = rp-I(xl (P),

...

xi-I(p),

xi+l(p),

...

xm(P))

is called the ith coordinate curve through P. The tangent vector to this curve

at P is denoted by aiIp and is called the ith coordinate vectorfield at P. The

collection

all vectorfields at P iscalled a coordinateframe at P. The variable

u is arbitrary inthe sense that it can be replaced by any (good)junction

Let c = xi (P). Then for f E F""(P), we have

• d il

(ailp)f

(Yi(C»(f)

0 y u=c

.!:.-

f(rp-I(xl(p),

...

,xi-l(p),

u,xi+l(p),

...

,xm(pml

du u=c

::i

ailp =

a~i

, (26.5)

wherethe last equalityis a (natural)

definition of the partial derivative of f with

respect to the ith coordinateevaluatedat the point

P. This partial derivative is

againa

e""

functionat P. Wethereforehavethe following:

26.2.6.Proposition.

The coordinateframe (aiIp

Ir=1

at P is a set

operators

ai(P) : F""(P) -+

given by

(ailp)f=

afl

(26.6)

ax'

f(rp-I(xl(p),

...

xi-l(p),

xi+l(p),

...

xm(p»)1

u=c

Anotherconunonnotationfor

af/ax

is f.i

26.2.7.Example.

Pick

point

p =

(sinB

cosrp,

sinB

sinrp,

cosB)onthe

sphere

S2ina

chart

(Up, 1")

given

I"(sin

Bcosip, sinBsinip, cosB) = (B,

rp).1f

Bis

kept

constant

and

is allowedtovaryovervaluesgivenby u, thenthe

coordinate

curveassociated with

given

y~(u)

= I"-I(B,u) =

(sinB

cosu,

sinB

sinu, cosB).

As u

varies,

ytp

(u)

describes

a curveon S2. Thiscurveis simplya circle of

radius

sine.

The

tangent

to this

curve

atanypointis

fJ/ocp,

orsimply

arp,

the

derivative

with

respect

the

coordinate

ip,

Similarly,

the

corve

(u)

describes

great

circle

onS2

with

tangent

aB.

III

The vector space Tp

(M)

of all tangents at P was mentioned earlier. In the

case of 8

this tangent space is simply a plane tangent to the sphere at a point.

Also,thetwovectors,

aeand

enconnteredinExample26.2.7areclearlylinearly

independent.Thus,theyform a basisforthe tangentplane.This argumentcan be

generalizedto anymanifold.The following theoremis such a generalization(for

a proof,see [Hish80,pp. 51-53]):

(26.7)

Remember

Einstein's

summation

convention!

26.2

CURVES

AND

TANGENT

VECTORS

775

26.2.8.

Theorem.

Let M be an m-dimensional manifold and P E M. Then the

set {aiIp}7::dorms a basis oj'J'

p(M).

In particular, 'J'p(M) is m-dimensional. An

arbitrary vector,

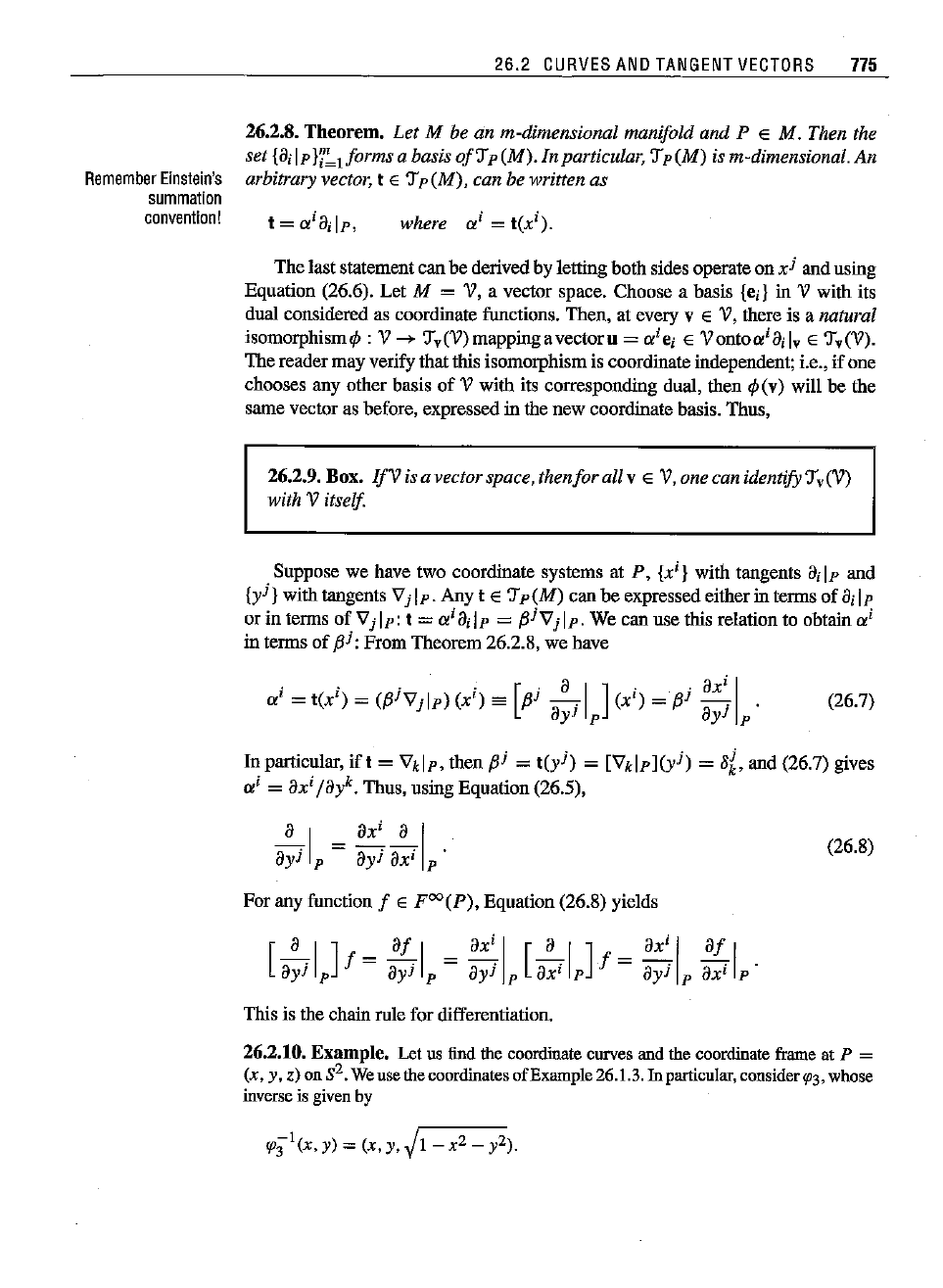
I E 'J'p(M), can be written as

Thelast statement can be derived by letting both sides operate on

and usiog

Equation (26.6). Let

M = V, a vector space. Choose a basis {e.} io V with its

dual

considered as

coordinate

functions.

Then,ateveryv E V,

there

is a natural

isomorphisme :V --> 'J'v(V)mappiogavectoru = aiei E

Vontoaiailv

E 'J'v(V).

The readermay verify that this isomorphism is coordioate iodependent; i.e.,

one

chooses any other basis

V with its corresponding dual, then .p(v) will be the

samevectoras before,expressedinthenew coordinate basis.Thus,

26.2.9. Box.

IjV

isa vector space, thenjorall v E V,one can identify

'J'v

(V)

with Vitself.

Suppose we have two coordinate systems at P, {xi} with tangents ailp and

{yi} with tangents

Ip. Any

'J'

p(M)

can be expressed either io terms of ailp

or in terms of

Ip: I = a

ailp =

Ip. We can use this relation to obtaio a

in terms of

pi:

From Theorem 26.2.8, we have

=I(x

(piVilp}(xi)

[pi

](xi)

=pi

aXi.J

ay} p ay} p

In particular,

t =

Vklp,

then

I(yi)

= [Vklp](yi) =

81,

and (26.7) gives

= ax

/a/.

Thus, usiog Equation (26.5),

(26.8)

For any function j E FOO(P),Equation (26.8) yields

This is the chaio rule for differentiation.

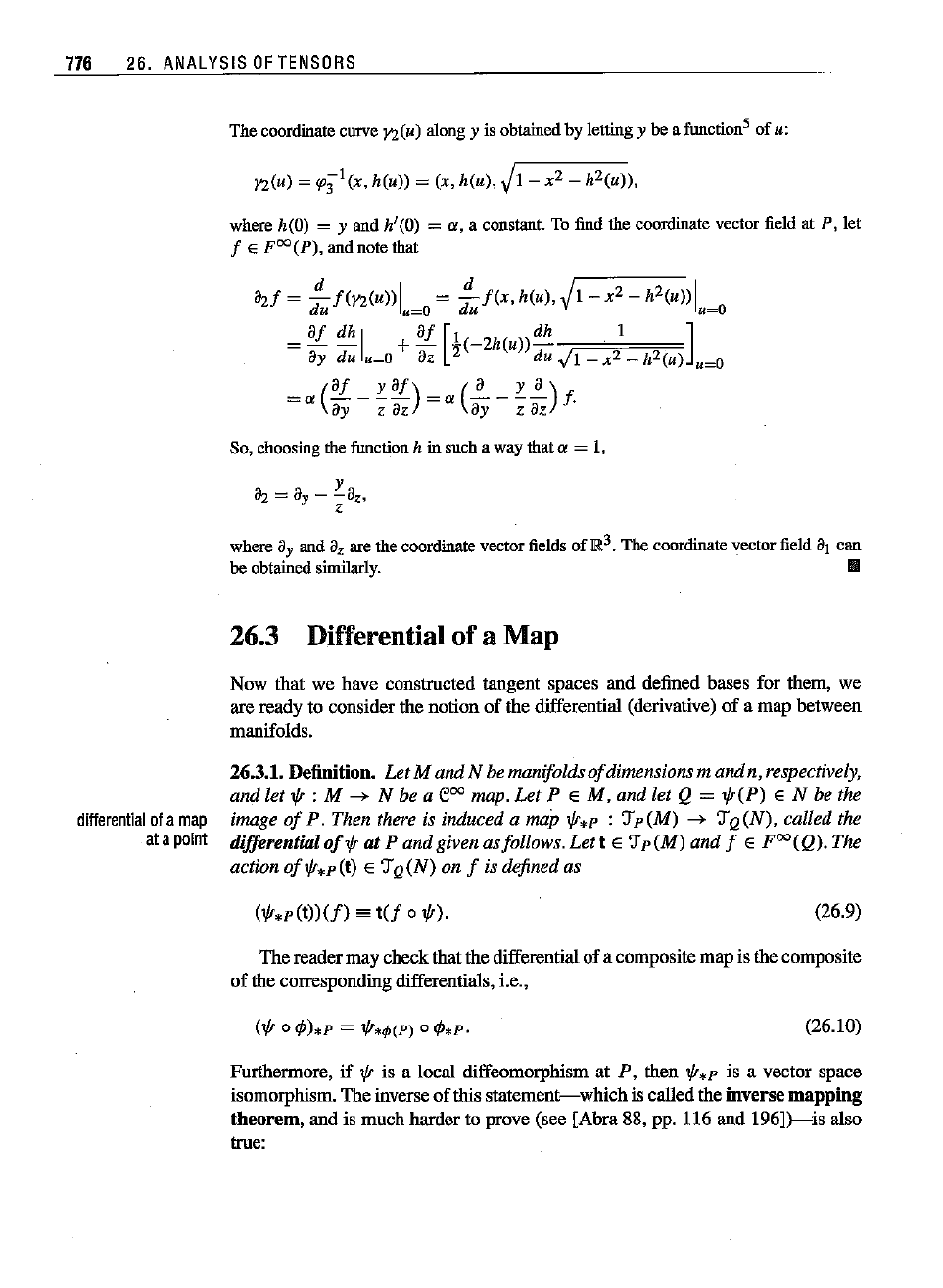
26.2.10.

Example.

Let us find the coordinate curves and the coordinate frame at P =

(x, y, z) on S2. Weusethe

coordinates

ofExample26.1.3.In

particular,

consider

({J3.

whose

inverse

is givenby

776 26. ANALYSIS

TENSORS

Thecoordinate corvey.!(u) alongy is obtainedby letting y be afunction

of u:

where h(O) = y and h'(O) = a, a constant.To find the coordinatevector field at P, let

f E

FOO(P),

andnotethat

azf

.'!...-

f(Y.!(u)) I =

.'!...-

f(x,

h(u),

JI-

- h

2(u))

u=o

u=O

dh I

dh 1 ]

= - - +-

-(-2h(u))

ay du u=o az 2 du

,}I-

- h

2(u)

u=o

=a(a

_?'.-af)=a(~_?'.-~)f.

ay z az ay z az

So, choosing the function h in such a way that a = 1,

=ay- -ar,

where a

and

are the coordinate vector fields of IR

The coordinate vector field

can

obtainadsimilarly. ill

26.3 Differential of a Map

Now that we have constructed tangent spaces and defined bases for them, we

are ready to consider the notion

the differential (derivative)

a map between

manifolds.

differential

ofa

map

ata

point

26.3.1. Definition.

Let

and

N be manifolds

dimensionsm

and

n, respectively,

and

let

1ft

: M --> N be a e

TYUlp.

Let

P E

and

let Q = 1ft(P) E N be the

image

P. Then there is induced a

map

1ft,P :

'Jp(M)

-->

'JQ(N),

called the

differentialof1ft

and

given

asfollows.

Let

p(M)

and

f E FOO(Q). The

action

of1ft,p(l)

E 'J

Q(N)

on f is defined as

(1ft,p(I»(f)

l(f

0 1ft). (26.9)

The

readermay checkthat the differential

a compositemap is the composite

the corresponding differentials, i.e.,

(1ft

</J),p

= 1ft,¢(P)0

</J,p.

(26.10)

Furthermore, if

1ft

is a local diffeomorphism at

theu

1ft

is a vector space

isomorphism. The inverse

this

statement-which

is called the

inverse

mapping

theorem,

and is much harder to prove (see [Abra 88, pp. 116 and 196]}--is also

true:

inverse

mapping

theorem

26.3

DIFFERENTIAL

MAP

777

26.3.2.

Theorem.

(inverse mapping theorem)

1ft

: M

--+

N is a map

and

1ftoP

'Jp(M)

--+

'Jy,(p)(N) is a vector space isomorphism, then

1ft

is a local

dijfeomOlphism at P.

Letus seehowEquation(26.9)looksintemnsofcoordinatefunctions.Suppose

that {xili'=l are coordinatesat P and

{yal:=l

are coordinatesat Q = 1ft(P). We

notethat

ya o

1ft

is areal-valuede

functionon M. Thus,wemay write(withthe

functionexpressedin

temns

of coordinates)

Wealso have

ailp. Similarly,1ftop(l) = tJa(a/aya)IQ because {a/aya)IQl

fomn

a basis.Theorem26.2.8and Definition26.3.I now give

tJa = 1ftop(I)(ya) = I(ya 0

1ft)

l(fa)

at"

=[aiailp](t")=ai-.I

="'ai_·I·

ax'

L.,

ax'

1=1

Thiscan be writtenin matrix

fomn

(

)

(afl/aXI

tJ2

af2/ax

· .

tJn

af"/ax

afl/aX

af2/ax

· .

afn/ax

(26.

II)

Jacobian

matrix

differentiable

map

Differential

constant

map

the

zero

map.

The n x m matrix is denoted

J and is called the

Jacobian

matrixof

1ft

with

respect to the coordinates

and ya. On numerous occasions the two manifolds

are simplyCartesianspaces,so that

1ft

--+

R",

sucha case,

is naturally

writtenas 1ft",and the Jacobianmatrix will haveelementsof the

fomn

a1ft"

/ax

An important special case of the differential of a map is that of a constant

map.Let

1ft

: M

--+

{Ql E N be such a map;it maps allpoints of M onto a single

point

Q of

For any f E Foo(Q), the function f 0

1ft

FOO(P)

is constantfor

all P

EM.

Let I E 'Jp

(M)

be an arbitraryvector.Then

(1ftop(I))(f) es

l(f

1ft)

= 0

(26.12)

because

I(c) = 0 for any constantc. So,

26.3.3.Box.

1ft

: M

--+

lEN

is a constant map, so that it maps the

entire manifold M onto a

point

then

1ftoP

'Jp(M)

--+

Q(N)

the zero map,

SSeethelast

statement

of Definition 26.2.5.

778 26.

ANALYSIS

TENSORS

1\\'0 other special cases merit closer attention: M =

for arbitrary N, and

N =

for arbitrary M. In either case 'J,(JR)is one-dimensional with the basis

vector

(dldu)

I,.When M =

JR,

the mapping becomes a curve, y :

-->

N. The

only vector whose image we are interested in is

(dldu)I" with

y(c)

= P.

From (26.9) using Proposition 26.2.4 in the last step, we have

[

yo,

I] f =

f 0 y I =

(f(c»(f).

U c U u=c

This tells us that the differential

a curve at c is simply its tangent vector at y (c).

is commonto leave out the constant vector

(dldu)

I" and write y*, for the LHS.

components

tangenfs

curves

26.3.4. Example. Itis usefultohavean

expression

forthe

components

ofthe

tangent

acurvey atan

arbitrary

pointonit.Since y mapsthereal line to

witha

coordinate

patch

established

we can

write

y as y =

(yl,

...

, ym)

where

r' = xi 0 yare

ordinary

functions

ofone

variable.

Proposition

26.2.4

then

yields

did

I d

y*t!

= - f 0 y = -

f(y(u))

= -

f(y

(u),

...

, y (u»

du u=1 du u=t du u=t

= af

dyi

I es af

dyi

·yiad,

ax' du

u=1

ax' dt

(26.13)

Forthis

reason,

Y*t

sometimes

denoted

by y.

When N =

JR,

we are dealing with a real-valued function f :M

-->

R The

differential of

fat

P is fop :

'Jp(M)

-->

'J,(IR), where c =

f(P).

Since 'J,(JR)

is one-dimensional, for a tangent

'Jp(M),

we have f*p(l) =

a(dldu)I,.

Let

g :

-->

be an arbitrary function on R Then [f*p(l)](g) =

a(dgldu)"

or, by

definition of the LHS,

l(f

0 g) =

a(dgldu),.

To find a we choose the function

g(u) =u,i.e.,theidentityfunctionjthen zigyds =I

andl(f

og)

l(f)

=a. We

thus obtain

f.p(t)

l(f)(dldwlk.

Since 'J,(JR)is a flat one-dimensional vector

differentialofa

space, all vectors are the same.and there is no need to write

(dldu)I,.

Thus, we

real-valued

function

define the differential of

denoted by

f.,

as a map

'Jp(M)

-->

given by

df(l)

l(f).

(26.14)

In particnlar, if f is the coordinate function

and I is the tangent to the

jth

coordinate curve aj I

we obtain

(26.15)

Remember

Einstein's

summation

convention!

26.3

DIFFERENTIAL

MAP

779

This shows that

26.3.5. Box.

{dx'

}?::I

is dual to the basis

{ajlp

}j=1

of'J

p(M).

26.3.6.

Example.

Letf:

M -+

Rbe

areal-valuedfunctionnnM. Letxi becnnrdinates

atP. We

want

express

terms

coordinate

functions.

Fort E 'Jp (M) wecanwrite

t =a

ailp

and

df(t)

t(f)

= ar'[8;1p

](f)

,,!a,(f),

wberein the last step,we suppressed the P. Theorem26.2.8and Equation(26.14)yield

ee'

t(x')

= (dx')(t). Wethusbave

df(t)

8,(f)[(dx')(t)]

[8i(f)(dx

i)](t).

Sincethisis

true

forallt, we get

~8f

8,(f)(dx')

L..

(dx' ) =

L..

-,dx'.

i=l

(26.16)

Thisis theclassical

formula

forthe

differential

of a

function

we chooses', the

jth

member

of anew

coordinate

system,

forf. we

obtain

whichisthe transformation dualto Eqnation(26.8).

(26.17)

III

The

following is a powerful theorem that constructs a submanifold out

differeutiable map (for a proof, see [Warn 83, p. 31]):

26.3.7.

Theorem.

Assume

than?

: M

N is a

e""

map, that Q is a

point

in the

range

1/J,

and

that

1/J.

'Jp(M)

--'> 'J

Q(N)

is surjective

for

all P E 1/J-I(Q).

Then 1/J-I(Q) is a submanifold

and

dim 1/J-I(Q) =

dimM

dimN.

Compare this theorem with Propositiou26.1.10. There, V was an open subset

and since

r:'

(V)

is open, it is automatically an open submanifold.

The

difficulty in proving Theorem26.3.7 lies in the fact that

1/J

-I

(Q)

is closed because

{Q}, a single point

N, is closed.

We can justify the last statement

the theorem as follows. From Equation

(26.12), we readily conclude that 'Jp (1/J-I

(Q))

= ker 1/J.p.The dimension theo-

rem, applied to

1/J.P

'Jp(M)

--'>'J

Q(N),

now gives

dim

'Jp(M)

=dimker1/J.p +rank1/J.p

dimM

= dim 1/J-I(Q)

-+-

dimN,

where the last equality follows from the surjectivity

1/J.p.

780

26. ANALYSIS

TENSORS

26.3.8.Example. Consider a e

map / : R" -+

lEI.

Let c E

lEI

snch that the partial

derivatives

of/ are

defined

andnotallzeroforall pointsof

/-1

(c).Then,

according

toEqna-

tion(26.11), a vector

cia, E

Tp(lEI

)

is mappedhy

to thevector

(Jl'

(aJ/ax')

f=cd/dt.

Sinceai/ax' arenot allzero,hyproperly choosing

(Jl',

wecanmake

(Jl'

(a//ax')

f=cd/dt

sweepoverall

real

numbers.

Therefore,

f* is

surjective,

andby

Theorem

26.3.7,

j-ICc)

is an (n - I)-dimensional

submanifold

of R". A

noteworthy

specialcase is the

function

defined

/(x!, x

...

, x

)

= (x

+(x

+...+(x

andc = r

Then,/-I

(c),an(n-I)-sphereofradinsr, isasubmanifold ofR".

III

26.4 TensorFields on Manifolds

So far we have studied vector spaces, learned how to construct tensors ont

vectors, tonched on manifolds (the abstraction of spaces), seen how to construct

vectors at a single point in a manifold by the use

the tangent-at-a-curve idea,

and even found the dual vectors

dx'

Ip to the coordinate vectors 8,Ip at a point P

of a martifold. We have everything we need to study the analysis of tensors.

26.4.1

Vector

Fields

We are familiar with the concept of a vector field in 3D: Electric field, magnetic

field, gravitational field, velocity field, and so forth are all familiar notions. We

now want to generalize the concept so that it is applicable to a general martifold.

To begin with, let us consider the following definition.

26.4.1.Definition. The union

all tangent spaces atdifferentpoints0/a manifold

tangent

bundle

M is denoted by

T(M)

and called the tangent bundle

defined

T(M)

= U

'Jp(M)

PeM

can be shown ([Bish 80, pp. 158-164])that T

(M)

is amanifold

dimension

2dimM.

vector

field

defined

vector

fields

bya

map

26.4.2.Definition. A vectorfield X on a subset U

a manifold M is a mapping

X : U

T(M)

such that

X(P)

Xlp

X»

E 'Jp(M). The set

vector

fields on M is denoted by X(M).

Let

M and N be manifolds and F : M

N a

differentiable map. We say that the two vector fields

X E

X(M)

and Y E

X(N)

are F-related

F.(X

= YF(p)/orall P

EM.

This is sometimes written simply

F.X

= Y.

is worthwhile to point

out

that

F.X

is not, in general, a vector field on N. To

be a vector field,

F.X

mnst be defined at all points

N. The natural way to define

F.X

at Q E N is

[F.X(Q)](f)

X(f

0 F) where P is the preimage

Q,i.e.,

26.4

TENSOR

FIELDS

MANIFOLDS

781

F(P)

= Q.But there may not existany such P

may not be onto), or there may

be more than one

P (F may not be one-to-one) with such property. Therefore, this

natural construction does not lead to a vector field on N.

F.X

happens to be a

vector field on N, then it is clearly F-related to X. In terms of the coordinates

xi,

at each point P E

Xlp

X~Bilp,

where the real numbers

are components

X» in the basis {Bilp

[.As

P moves

around in

the real numbers

keep chimging. Thus, we can think of

as a

function of P and define the real-valued function Xi : M

--+

R by Xi

(P)

X~.

Therefore, the components

a vector field are real-valued functions on M.

26.4.3.

Example. Let M = ]R3. At eachpoint P = (x, y, z) E ]R3, let (ex, ey, e

) be a

basis for

}R3.

Let

Vp bethe vector space at

Then

TOR

)

the

collection

all vector

spacesVp for all P.

We can determine the value of an electric field at a point in

JR3

by first specifying

thepoint,as Po = (xo,

YO,

zo), for

example.

Thisuniquely determines the tangentspace

'JPo(lR.

). Once we have the vector space, we can

ask

what

the components

the electric

field are

in that space. These components are given by three numbers: Ex(xQ.

YO,

ZO),

Ey(xO,

YO,

zo), andEz(xo,

YO,

ZO)·

Theargoment is thesameforanyothervector

field.

To specify a "point" in

TOR

) ,

we need three numbers to determine the location in

}R3

and

another three numbers to determine the components of a vector field at that point.

Thus, a "point"

in TOR

)

is givenby six "coordinates" (x, y, z,

Ex.

z),

and

T(}R3) is

a six-dimensionalmanifold.

III

We know how a tangent vector t at a point P E M acts on a function f E

FOO(P) to give

areal

numbert(f).

Wecan extendthis, point by point, for a vector

field X and define a function

X(f)

[X(f)](P)

Xp(f),

P E U,

(26.18)

where U is a subset

M on which both X and f are defined. The RHS is well-

defined because we know how

Xp,

the vector at P, acts on functions at P to give

the real number [Xp

](f).

On the LHS, we have

X(f),

which maps the point P

onto a real number. Thus,

X(f)

is indeed a real-valued function on M. We can

therefore define vector fields directly as operators on

functions satisfying

X(otf

+fJg) =

otX(f)

+fJX(g),

X(fg)

[X(f)]g

[X(g)]f.

vector

fields

A prototypical vector field is the coordinate vector field B

•

general,

X(f)

is not a e

function even if f is. A vector field that produces a e

function

X(f)

for every e

function f is called a e

vector field. Such a vector field has

components that are e

functions on M.

The set of tangent vectors 'Jp

(M)

at a point P E M form an m-dimensional

vector space. The set of vector fields

X(M)-which

yield a vector at every point

782

26.

ANALYSIS

TENSORS

of the

manifold-also

constitutes a vector space. However, this vectur space is

(uncountably) infinite-dimensional. A property

X(M)

that is absent in

p (M)

is composition." This suggests the possibility

defining a "product" ou

X(M)

tum

it into an algebra. Let X and Y be vector fields. For X 0 Y to be a vector field,

it has to satisfy the derivatiou property. But

x 0

Y(fg)

X(Y(fg))

X(Y(f)g

fY(g))

(X(Y(f)))g

Y(f)X(g)

X(f)Y(g)

f(X(Y(g)))

(X 0

Y(f))g

f(X

Y(g)).

However, the reader may verify that Xo Y- Y

does iudeedsatisfy the derivatiou

The

setof

vector

property. Therefore, by defining the binary operation X(M) x X(M) --> X(M) as

fields

form

Lie

algebra.

[X, Y]

X 0 Y - Y 0 X,

X(M)

becomes an algebra, called the

Lie

algebra

of vector fields of M. The

binary operation is called the

Lie

bracket.

Although it was not mentioned at the

time, we have encountered another example

a Lie algebra in Chapter 2, namely

£,(V) under the binary operation

the commutation relation. Lie brackets have

the following two properties:

[X,

-[V,

X],

[[X, V], Z] +[[Z, X], Y] +[[Y, Z], X] =

These two relations are the defining properties

all Lie algebras. The last re-

Jacobi

identity

lation is called the

Jacobi

identity.

X(M)

with Lie brackets is an example of

an infinite-dimensional Lie algebra;

£,(V) with commutators is an example of a

finite-dimensional Lie algebra.

We shall have occasion to use the following theorem in our treatment of Lie

groups and algebras in the next chapter:

26.4.4.

Theorem.

Let M and N be manifolds and F : M

-->

N a differentiable

map. Assume that Xi E

X(M)

is F-related to Yi E

X(N)

for i = 1,2. Then

[XI, X2] is F-relatedto [YI, Y2], i.e.,

F.[XI,

X2] =

[F.XI,

F.X2].

Proof. Let f be an arbitrary fuoction on N. Then

(F.

[XI, X2]) f es

[Xl,

X2](f

0 F) = XI

(X2(f

F))

- X2

(XI(f

F))

= XI

([F.X2(f)]

0 F) - X2

([F.XI(f)]

0 F)

F.XI

(F.X2(f))

F.X2

(F.XI

(f))

[F.X

I, F.X2]f,

6Recall that a typical element

ofT

p(M)

is a map t : FOO(P)

--+-

R for which composition is meaningless.