Haddad W.M. Nonlinear Dynamical Systems and Control: A Lyapunov-Based Approach

Подождите немного. Документ загружается.

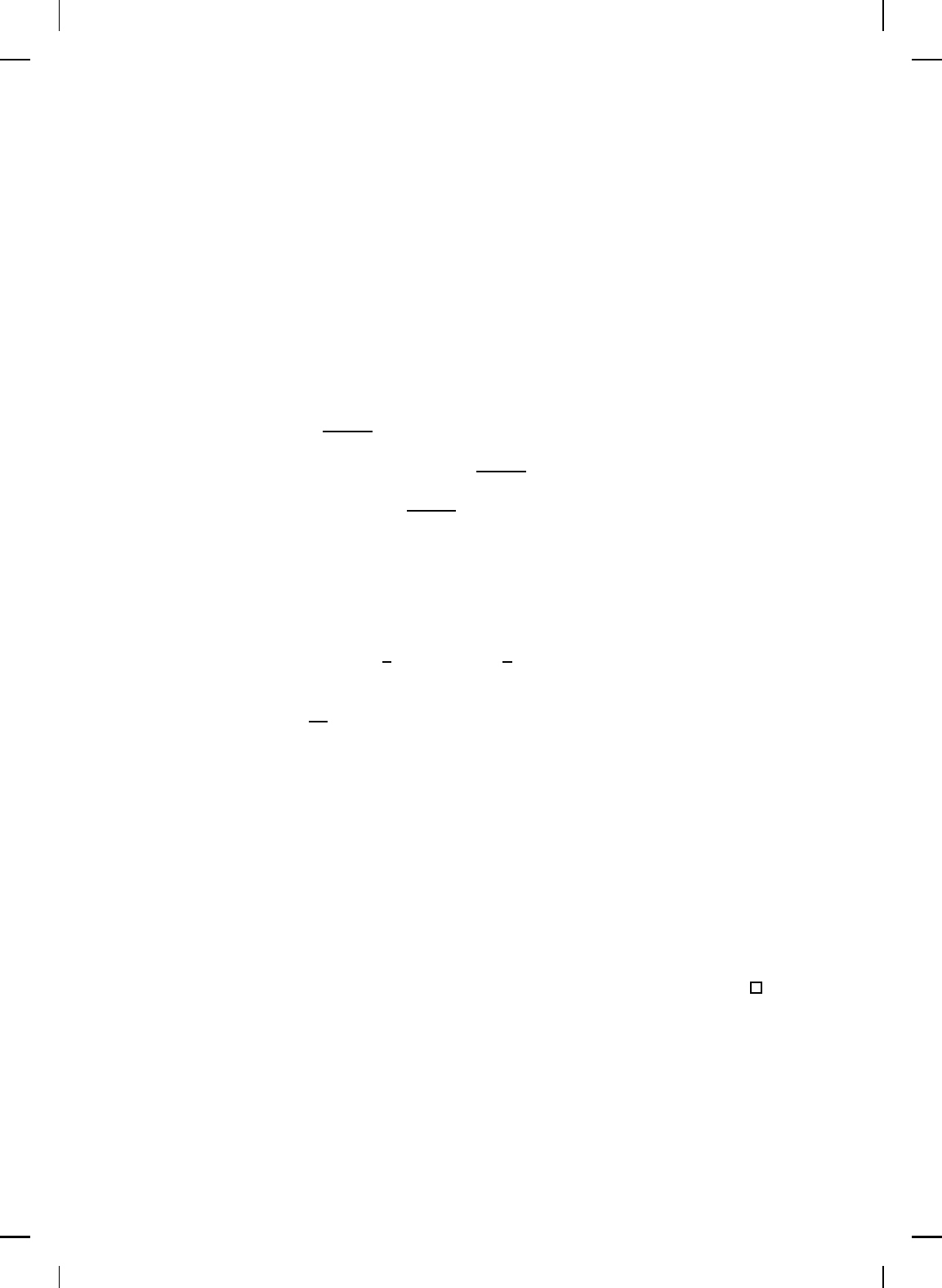
NonlinearBook10pt November 20, 2007

OPTIMAL NONLINEAR FEEDBACK CONTROL 535

or, equivalently, (σ

) −αv

)(σ

) −βv

) < 0, for all v

6= 0, i = 1, . . . , m.

In this case, the closed-loop system (8.100) and (8.101) with u = σ(−y) is

given by

˙x(t) = f (x(t)) + G(x(t))σ(φ(x(t))), x(0) = x

, t ≥ 0. (8.123)

Next, consider the Lyapunov function candidate V (x), x ∈ R

, satisfying

(8.107) and let

V (x) denote the Lyapunov derivative along the trajectories

of the closed-loop system (8.123). Now, it follows f rom (8.107) and (8.122)

that

V (x) = V

′

(x)f(x) + V

′

(x)G(x)σ(φ(x))

≤ V

′

(x)f(x) + V

′

(x)G(x)σ(φ(x)) + L

(x)

−

4(1−θ

)

(x)R

−1

(x)L

(x)

+(1 − θ

)

σ(φ(x)) +

2(1−θ

)

−1

(x)L

(x)

σ(φ(x)) +

2(1−θ

)

−1

(x)L

(x)

= V

′

(x)f(x) + L

(x) + V

′

(x)G(x)σ(φ(x))

+(1 − θ

)σ

(φ(x))R

(x)σ(φ(x)) + L

(x)σ(φ(x))

= φ

(x)R

(x)φ(x) −2φ

(x)R

(x)σ(φ(x))

+(1 − θ

)σ

(φ(x))R

(x)σ(φ(x))

i=1

(x)(

(−y

) + y

)(

(−y

) + y

)

αβ

i=1

(x) (σ

(−y

) + αy

) (σ

(−y

) + βy

)

≤ 0,

which implies that the closed-loop system (8.123) is Lyapunov s table.

Next, let R

△

= {x ∈ R

V (x) = 0} and note that

V (x) = 0 if

and only if y = 0. Now, since G is zero-state observable it follows that

△

= {x ∈ R

: x = 0} is the largest invariant set contained in R. Hence,

it follows from Theorem 3.5 that x(t) → M = {0} as t → ∞. Thus, the

closed-loop system (8.123) is globally asymptotically stable for all σ(·) such

that αv

< σ

< βv

, v

6= 0, i = 1, . . . , m, which implies that the

nonlinear system G given by (8.100) and (8.101) has sector (and, hence,

gain) margins (α, β).

Note that in the case where R

(x), x ∈ R

, is diagonal, Theorem 8.7

guarantees larger gain and sector margins to the gain and sector margin

guarantees provided by Theorem 8.6. However, T heorem 8.7 d oes not

provide disk margin guarantees.

NonlinearBook10pt November 20, 2007

536 CHAPTER 8

8.6 Inverse Optimality of Nonlinear Regulators

In this section, we give suﬃcient conditions that guarantee that a given

nonlinear feedback controller has prespeciﬁed disk, sector, and gain m argins.

First, we present the following generalization of the results given in [321].

Proposition 8.2. Let θ ∈ (0, 1) and let R

∈ R

m×m

be a positive-

deﬁnite matrix. Consider the nonlinear dynamical system G given by (8.100)

and (8.101), where φ(x) is a stabilizing feedback control law. Then there

exist functions V : R

→ R, L

: R

→ R, and L

: R

→ R

1×m

such that

φ(x) = −

−1

′

(x)G(x) + L

(x)]

, V (·) is continuously diﬀerentiable,

V (0) = 0, V (x) > 0, x ∈ R

, x 6= 0, and for all x ∈ R

0 = V

′

(x)f(x) + L

(x) − [V

′

(x)G(x) + L

(x)]R

−1

′

(x)G(x) + L

(x)]

(8.124)

0 ≤ (1 − θ

(x) −

(x)R

−1

(x), (8.125)

if and only if, for all u(·) ∈ U and t

, t

≥ 0, t

< t

, there exists V : R

→ R

such that V (0) = 0, V (x) > 0, x ∈ R

, x 6= 0, and the solution x(t), t ≥ 0,

to (8.100) satisﬁes

V (x(t

)) ≤



[u(t) + y(t)]

[u(t) + y(t)] −θ

(t)R

u(t)



+V (x(t

)). (8.126)

Proof. If there exist functions V : R

→ R, L

: R

→ R, and

: R

→ R

1×m

such that φ(x) = −R

−1

′

(x)G(x) + L

(x)]

and (8.124)

and (8.125) are satisﬁed, then it follows from Lemma 8.2 that (8.126) is

satisﬁed. Conversely, if for all t

, t

≥ 0, t

< t

, and u(·) ∈ U the solution

x(t), t ≥ 0, to (8.100) satisﬁes (8.126), then with Q = R

, S = R

, and

R = (1 −θ

, it follows from (5.81) of Theorem 5.6 that

0 ≥ V

′

(x)f(x) −φ

(x)R

φ(x) +

4(1−θ

)

[2φ

(x)R

+ V

′

(x)G(x)]

·R

−1

[2φ

(x)R

+ V

′

(x)G(x)]

, x ∈ R

The result now follows with L

(x) = −V

′

(x)f(x) + φ

(x)R

φ(x) and L

(x)

= −[2φ

(x)R

+ V

′

(x)G(x)].

Note that if (8.124) and (8.125) are satisﬁed then it follows fr om

Theorem 8.4 that the feedback control law φ(x) = −R

−1

′

(x)G(x) +

(x)]

minimizes the cost functional (8.58). Hence, Proposition 8.2

provides necessary and suﬃcient conditions for optimality of a given

stabilizing feedback control law with prespeciﬁed disk margin guarantees.

The following result presents speciﬁc disk margin guarantees for inverse

optimal controllers.

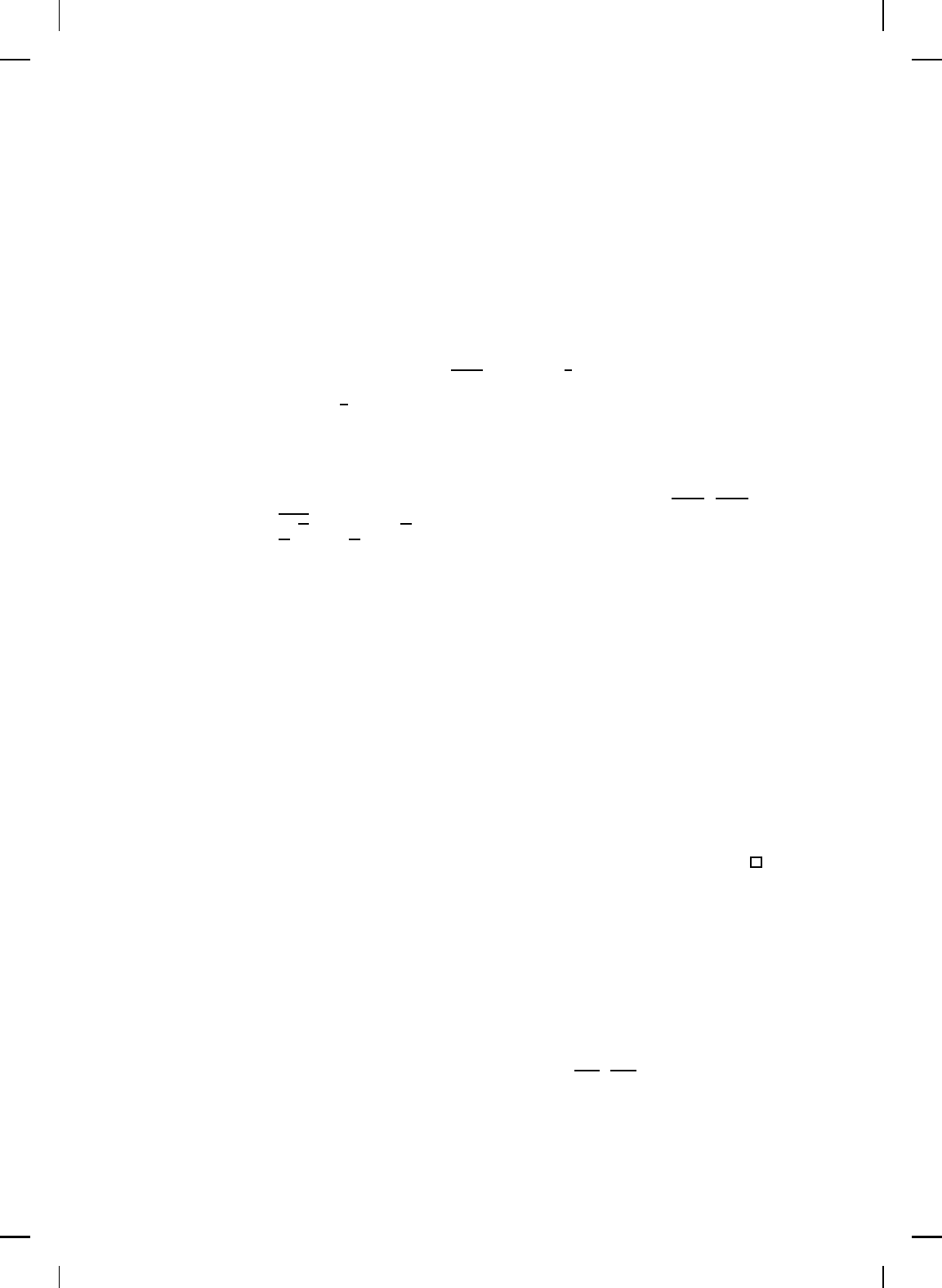
NonlinearBook10pt November 20, 2007

OPTIMAL NONLINEAR FEEDBACK CONTROL 537

Theorem 8.8. Let θ ∈ (0, 1) be given. Consider the nonlinear

dynamical system G given by (8.100) and (8.101) where φ(x) is a stabilizing

feedback control law. Assume that there exist functions V : R

→ R and

: R

→ R

m×m

such that V (·) is continuously diﬀerentiable, R

(x) > 0,

x ∈ R

, and

V (0) = 0, (8.127)

V (x) > 0, x ∈ R

, x 6= 0, (8.128)

′

(x)[f(x) + G(x)φ(x)] < 0, x ∈ R

, x 6= 0, (8.129)

′

(x)f(x) −φ

(x)R

−1

(x)φ(x) +

1−θ



(x) +

′

(x)G(x)R

−1

(x)



·R

(x)



(x) +

′

(x)G(x)R

−1

(x)



≤ 0, x ∈ R

, (8.130)

and

V (x) → ∞ as kxk → ∞. (8.131)

Then the nonlinear dynamical system G h as a disk m argin (

1+ηθ

1−ηθ

where η =

γ/γ and γ and γ are given by (8.121). Furtherm ore, with the

feedback control law φ(x) the performance functional

J(x

, u(·)) =

∞

[−V

′

(x(t))(f(x(t)) + G(x(t))u(t))

+(φ(x(t)) − u(t))

(x(t))(φ(x(t)) − u(t))]dt (8.132)

is minimized in the sense that

J(x

, φ(x(·))) = min

u(·)∈S(x

)

J(x

, u(·)), x

∈ R

. (8.133)

Proof. The result is a direct consequence of Theorems 8.4 and 8.6

with L

(x) = −V

′

(x)f(x) + φ

(x)R

(x)φ(x) and L

(x) = −(2φ

(x)R

(x)+

′

(x)G(x)). Speciﬁcally, in this case, all the conditions of T heorem 8.4 are

trivially satisﬁed. Furthermore, note that (8.130) is equivalent to (8.119).

The result is now immediate.

The next result provides suﬃcient conditions that guarantee that a

given nonlinear feedback controller h as prespeciﬁed gain and sector margins.

Theorem 8.9. Let θ ∈ (0, 1) be given. Consider the nonlinear dynami-

cal system G given by (8.100) and (8.101) where φ(x) is a stabilizing feedback

control law. Assume there exist functions R

(x) = diag[r

(x), . . . , r

(x)],

where r

: R

→ R, r

(x) > 0, i = 1, . . . , m, an d V : R

→ R such that

V (·) is continuously diﬀerentiable and satisﬁes (8.127)–(8.131). Then the

nonlinear dynamical system G has a disk margin (

1+θ

1−θ

). Furtherm ore,

with the feedback control law φ(x) the performance functional (8.132) is

NonlinearBook10pt November 20, 2007

538 CHAPTER 8

minimized in the sense that

J(x

, φ(x(·))) = min

u(·)∈S(x

)

J(x

, u(·)), x

∈ R

. (8.134)

Proof. The result is a direct consequence of Theorems 8.4 and 8.7

with the proof being id entical to the proof of Theorem 8.8.

8.7 Linear-Quadratic Optimal Regulators

In this section, we specialize Theorems 8.5 and 8.6 to the case of linear

systems. Speciﬁcally, consider th e stabilizable linear system given by

˙x(t) = Ax(t) + Bu(t), x(0) = x

, t ≥ 0, (8.135)

y(t) = −Kx(t), (8.136)

where A ∈ R

n×n

, B ∈ R

n×m

, and K ∈ R

m×n

, and assum e that (A, K)

is detectable and the linear system (8.135) and (8.136) is asymptotically

stable with the feedback u = −y or, equivalently, A + BK is Hurwitz.

Furth ermore, assume that K is an optimal regulator which minimizes the

quadratic performance functional given by

J(x

, u(·)) =

∞

(t)R

x(t) + 2x

(t)R

u(t) + u

(t)R

u(t)]dt, (8.137)

where R

∈ R

n×n

, R

∈ R

n×m

, and R

∈ R

m×m

are such that R

> 0,

−R

−1

≥ 0, and (A, R

) is observable. In this case, it follows f rom

Theorem 8.4 with f(x) = Ax, G(x) = B, L

(x) = x

x, L

(x) = 2x

(x) = R

, φ(x) = Kx, and V (x) = x

P x that the optimal control law

K is given by K = −R

−1

P + R

), where P > 0 is th e solution to the

algebraic regulator Riccati equation given by

0 = (A−BR

−1

)

P +P (A−BR

−1

)+R

−R

−1

−P BR

−1

(8.138)

The following results provide guarantees of disk, sector, and gain margins

for the linear system (8.135) and (8.136).

Corollary 8.5. Consider the linear dynamical system (8.135) and

(8.136) with performan ce functional (8.137) and let σ

max

) < σ

min

)

·σ

min

). Then , with K = −R

−1

P +R

), wh ere P > 0 satisﬁes

(8.138), the linear system (8.135) and (8.136) has disk margin (and, hence,

sector and gain margins) (

1+ηθ

1−ηθ

), where

η =

min

)

max

)

, θ =



1 −

max

)

min

)σ

min

)



1/2

. (8.139)

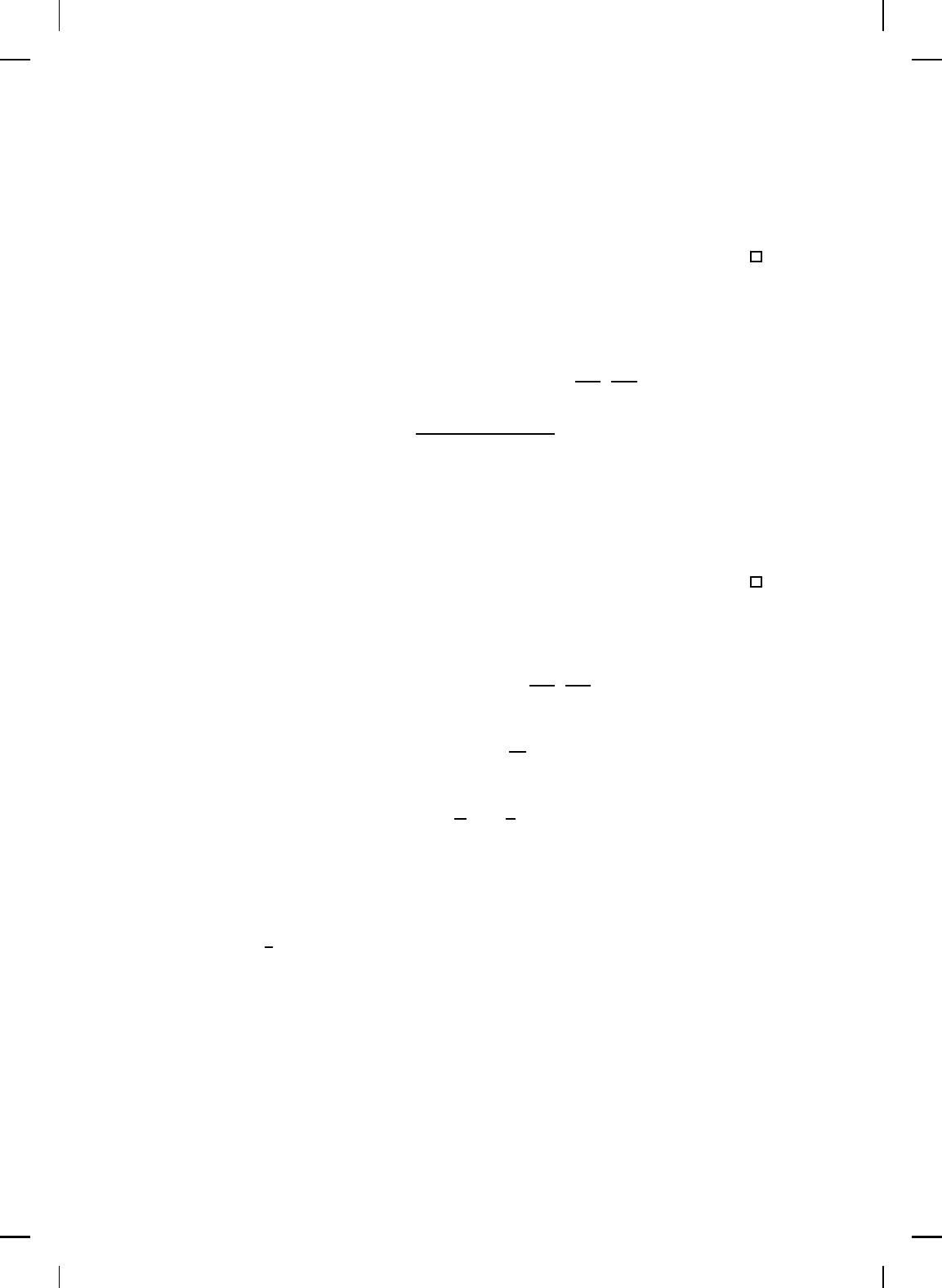
NonlinearBook10pt November 20, 2007

OPTIMAL NONLINEAR FEEDBACK CONTROL 539

Proof. The result is a direct consequence of Theorem 8.6 with f (x) =

Ax, G(x) = B, φ(x) = Kx, V (x) = x

P x, L

(x) = x

x, and L

(x) =

. Speciﬁcally, note that (8.138) is equivalent to (8.107). Now, with

θ given by (8.139), it follows that (1 − θ

− R

−1

≥ 0, and hence,

(8.122) is satisﬁed so that all the conditions of Theorem 8.6 are satisﬁed.

Corollary 8.6. Consider the linear dynamical system (8.135) and

(8.136) with performance f unctional (8.137) and let σ

max

) < σ

min

)

·σ

min

), where R

is diagonal. Then, with K = −R

−1

P +R

), where

P > 0 satisﬁes (8.138), the linear system (8.135) and (8.136) has structured

disk margin (and, hence, gain and sector) margin (

1+θ

1−θ

), where

θ =



1 −

max

)

min

)σ

min

)



1/2

. (8.140)

Proof. The result is a direct consequence of Theorem 8.5 with f (x) =

Ax, G(x) = B, φ(x) = Kx, V (x) = x

P x, L

(x) = x

x, and L

(x) =

. Speciﬁcally, note that (8.138) is equivalent to (8.107). Now, with

θ given by (8.140), it follows that (1 − θ

− R

−1

≥ 0, and hence,

(8.122) is satisﬁed so that all the conditions of Theorem 8.5 are satisﬁed.

The gain margins obtained in Corollary 8.6 are precisely the gain

margins given in [95] for linear-quadratic optimal regulators with cross-

weighting terms in the performance criterion. Fu rthermore, since Corollary

8.6 guarantees structured disk margins of (

1+θ

1−θ

), it follows that th e

linear system has a phase margin φ given by (see Problem 6.20)

cos(φ) = 1 −

, (8.141)

or, equivalently,

sin





. (8.142)

In the case where R

= 0 it follows f rom (8.140) th at θ = 1, and hen ce,

Corollary 8.6 guarantees a phase margin of ±60

◦

in each input-output

channel. In addition, requiring that R

≥ 0, it follows from Corollary 8.6

that the linear system given by (8.135) and (8.136) has a gain and sector

margin of (

, ∞).

Finally, we specialize Proposition 8.2 to the case of linear systems

to provide necessary and suﬃcient conditions for optimality of a given

stabilizing linear feedback control law.

Proposition 8.3. Let θ ∈ (0, 1) and let R

∈ R

m×m

be a positive-

deﬁnite matrix. Consider the linear system given by (8.135) and (8.136)

NonlinearBook10pt November 20, 2007

540 CHAPTER 8

where K ∈ R

m×n

is such that A + BK is Hurwitz. Then the following

statements are equ ivalent:

i) There exist matrices P, R

∈ R

n×n

, and R

∈ R

n×m

such that K =

−R

−1

P + R

), P is positive deﬁnite, R

is nonnegative deﬁnite,

and

0 = A

P + P A + R

− (P B + R

−1

(P B + R

)

, (8.143)

0 ≤ (1 − θ

− R

−1

. (8.144)

ii) The transfer function θR

1/2

[I − G(s)]

−1

−1/2

is bounded real, where

G(s)

△

= K(sI − A)

−1

iii) The transfer function R

[(1 + θ)I −G(s)][(1 −θ)I −G(s)]

−1

is positive

real.

Proof. The result follows from Proposition 8.2 with f(x) = Ax,

G(x) = B, φ(x) = Kx, L

(x) = R

, L

(x) = 2x

, and V (x) = x

P x.

Speciﬁcally, it follows f rom Proposition 8.2 that K, P , R

, R

, and

satisfy (8.143) and (8.144) if and only if the linear system (8.135)

and (8.136) is dissipative with respect to the supply rate r(u, y) = [u +

[u + y] − θ

u. Now, it follows from Problem 5.14 that the linear

system (8.135) and (8.136) is dissipative with respect to the supply r ate

r(u, y) = [u+y]

[u+y]−θ

u if and only if G

∗

(s)R

G(s)−G

∗

(s)R

−

G(s) + (1 − θ

≥ 0, Re[s] > 0. T he result now follows as a direct

consequence of Problem 5.15.

8.8 Stability Margins, Meaningful In verse Optimality, and

Control Lyapunov Functions

In this section, we specialize the results of Section 8.5 to the case where

L(x, u) is nonnegative for all (x, u) ∈ R

× R

. In the terminology of

[128,395] this corresponds to a meaningful cost functional. Here, we assume

(x) ≡ 0 and L

(x) ≥ 0, x ∈ R

. In this case, we show that for every

nonlinear dynamical system for which a control L yapunov function can be

constructed there exists an inverse optimal feedback control law with sector

and gain margins of (

, ∞). The ﬁrst result specializes T heorem 8.4 to the

case in which L

(x) ≡ 0.

Theorem 8.10. Consider the nonlinear dynamical system (8.100) with

performance functional (8.102) with L

(x) ≡ 0 and L

(x) ≥ 0, x ∈ R

Assume th ere exists a continuously diﬀerentiable function V : R

→ R such

NonlinearBook10pt November 20, 2007

OPTIMAL NONLINEAR FEEDBACK CONTROL 541

that

V (0) = 0, (8.145)

V (x) > 0, x ∈ R

, x 6= 0, (8.146)

0 = L

(x) + V

′

(x)f(x) −

′

(x)G(x)R

−1

(x)G

(x)V

′T

(x), x ∈ R

(8.147)

and

V (x) → ∞ as kxk → ∞. (8.148)

Furth ermore, assume that the system (8.100) and (8.101) is zero-state

observable with y = L

(x). Then the zero solution x(t) ≡ 0 of the closed-

loop system

˙x(t) = f (x(t)) + G(x(t))φ(x(t)), x(0) = x

, t ≥ 0, (8.149)

is globally asymptotically stable with the feedback control law

φ(x) = −

−1

(x)G

(x)V

′T

(x), (8.150)

and the performan ce functional (8.102) is minimized in the sense that

J(x

, φ(x(·))) = min

u(·)∈S(x

)

J(x

, u(·)), x

∈ R

. (8.151)

Finally,

J(x

, φ(x(·))) = V (x

), x

∈ R

. (8.152)

Proof. The proof is similar to the proof of T heorem 8.3.

Next, we show that for a given nonlinear dynamical system G given

by (8.100) and (8.101), there exists an equivalence between optimality and

passivity. For the following result we assume that for a given nonlinear

system (8.100), if there exists a feedback control law φ(x) such that it

minimizes the performance functional (8.102) with R

(x) ≡ I, L

(x) ≡ 0,

and L

(x) ≥ 0, x ∈ R

, then there exists a continuously diﬀerentiable

positive-deﬁnite function V (x), x ∈ R

, such that (8.147) is satisﬁed.

Theorem 8.11. Consider th e nonlinear dynamical system G given by

(8.100) and (8.101). The feedback control law u = φ(x) is optimal with

respect to a perf ormance functional (8.102) with R

(x) ≡ I, L

(x) ≡ 0,

and L

(x) ≥ 0, x ∈ R

, if and only if the n on linear system G is dissipative

with respect to the supply rate r(u, y) = y

y + 2u

y and has a continuously

diﬀerentiable positive-deﬁnite, radially unbounded storage fun ction V (x),

x ∈ R

Proof. If the control law φ(x) is optimal with respect to a performance

functional (8.102) with R

(x) ≡ I, L

(x) ≡ 0, and L

(x) ≥ 0, x ∈ R

NonlinearBook10pt November 20, 2007

542 CHAPTER 8

then, by assumption, there exists a continuously diﬀerentiable positive-

deﬁnite function V (x) su ch that (8.147) is satisﬁed. Hence, it f ollows from

Proposition 8.2 that the solution x(t), t ≥ 0, to (8.100) satisﬁes

V (x(t

)) ≤



[u(t) + y(t)]

[u(t) + y(t)] − u

(t)u(t)



dt + V (x(t

)),

0 ≤ t

≤ t

which implies that G is dissipative with respect to the supply rate r(u, y) =

y + 2u

Conversely, if G is dissipative with respect to the supply rate r(u, y) =

y + 2u

y and has a continuously diﬀerentiable positive-deﬁnite storage

function, then, with h(x) = −φ(x), J(x) ≡ 0, Q = I, R = 0, and S = 2I,

it follows from Theorem 5.6 that there exists a function ℓ : R

→ R

such

that φ(x) = −

(x)V

′T

(x) and, for all x ∈ R

0 = V

′

(x)f(x) −

′

(x)G(x)G

(x)V

′T

(x) + ℓ

(x)ℓ(x).

Now, the result follows from Theorem 8.10 with L

(x) = ℓ

(x)ℓ(x).

Example 8.4. Consider the nonlinear scalar dynamical system dis-

cussed in Example 6.6 given by

˙x(t) = x

(t) + u(t), x(0) = x

, t ≥ 0. (8.153)

Recall th at the optimal controller φ(x) = −x

− x

√

+ 1 minimizes the

cost functional J(x

, u(·)) =

∞

(t) + u

(t)]dt. Furthermore, recall that

V (x) =

+ (x

+ 1)

3/2

− 1) satisﬁes the Hamilton-Jacobi-Bellman

equation. Now, it follows from Theorem 8.11 that (8.153) is dissipative

with r espect to the supply rate r(u, y) = y

+ 2uy, where y = −φ(x) =

√

+ 1. To show this consider the storage function V

(x) = V (x) and

note that

(x) = V

′

(x)(x

+ u) = 2(x

+ x

√

+ 1)(x

+ u) = 2(yx

+ yu).

Now, noting that 2x

+ 2x

√

+ 1 ≤ (x

+ x

√

+ 1)

it follows that

2yx

≤ y

, and hence,

(x) ≤ y

+ 2uy. △

The next result gives disk and structured disk margins for the

nonlinear dynamical system G given by (8.100) and (8.101).

Corollary 8.7. Consid er the nonlinear dynamical system G given by

(8.100) and (8.101) where φ(x) is a stabilizing feedback control law given

by (8.110) with L

(x) ≡ 0 and where V (x), x ∈ R

, satisﬁes (8.107).

Furth ermore, assume R

(x) = diag[r

, . . . , r

], where r

> 0, i = 1, . . . , m,

and L

(x) ≥ 0, x ∈ R

. Then the non linear dynamical system G has

a structured disk margin (

, ∞). If, in addition, R

(x) ≡ I

, then the

nonlinear system G has a disk margin (

, ∞)

NonlinearBook10pt November 20, 2007

OPTIMAL NONLINEAR FEEDBACK CONTROL 543

Proof. The r esult is a direct consequence of Theorem 8.5. Speciﬁcally,

if L

(x) ≥ 0, x ∈ R

, and L

(x) ≡ 0, then (8.119) is trivially satisﬁed f or all

θ ∈ (0, 1). Now, the result follows immediately by letting θ → 1.

Next, we provide sector and gain margins for the nonlinear dynamical

system G given by (8.100) and (8.101).

Corollary 8.8. Consid er the nonlinear dynamical system G given by

(8.100) and (8.101) where φ(x) is a stabilizing feedback control law given

by (8.65) with L

(x) ≡ 0 and where V (x), x ∈ R

, satisﬁes (8.107).

Furth ermore, assume R

(x) = diag[r

(x), . . . , r

(x)], where r

: R

→ R,

(x) > 0, i = 1, . . . , m, and L

(x) ≥ 0, x ∈ R

. Then the n onlinear

dynamical system G has a sector (and , hence, gain) margin (

, ∞).

Proof. The r esult is a direct consequence of Theorem 8.7. Speciﬁcally,

if L

(x) ≥ 0, x ∈ R

, and L

(x) ≡ 0 then (8.119) is trivially satisﬁed for all

θ ∈ (0, 1). Now, the result follows immediately by letting θ → 1.

Finally, we show that given a control Lyapunov function for a

controlled nonlinear system, the feedback control law given by (6.81)

guarantees sector and gain margins of (

, ∞).

Theorem 8.12. Consider the nonlinear dynamical system G given

by (8.100) and let the continuously diﬀerentiable positive-deﬁnite, radially

unbounded function V : R

→ R be a control Lyapunov f unction of (8.100),

that is,

′

(x)f(x) < 0, x ∈ R, (8.154)

where R

△

= {x ∈ R

: x 6= 0, V

′

(x)G(x) = 0}. Then with the feedback

stabilizing control law given by

φ(x) =







−



α(x)+

√

(x)+(β

(x)β(x))

(x)β(x)



β(x), β(x) 6= 0,

0, β(x) = 0,

(8.155)

where α(x)

△

= V

′

(x)f(x), β(x)

△

= G

(x)V

′T

(x), and c

> 0, the nonlinear

dynamical system G given by (8.100) and (8.101) h as a sector (and, hence,

gain) margin (

, ∞). Furthermore, with the feedback control law u = φ(x)

the performance functional

J(x

, u(·)) =

∞

[α(x(t)) −

γ(x(t))

(x(t))β(x(t)) +

2γ(x(t))

(t)u(t)]dt,

(8.156)

NonlinearBook10pt November 20, 2007

544 CHAPTER 8

where

γ(x)

△









α(x)+

√

(x)+(β

(x)β(x))

(x)β(x)



, β(x) 6= 0,

, β(x) = 0,

(8.157)

is minimized in the sense that

J(x

, φ(x(·))) = min

u∈S(x

)

J(x

, u(·)), x

∈ R

. (8.158)

Proof. The result is a direct consequence of Corollary 8.7 and Theorem

8.10 with R

(x) =

2γ(x)

and L

(x) = − α(x)+

γ(x)

(x)β(x). Speciﬁcally,

it follows fr om (8.157) that R

(x) > 0, x ∈ R

, and

(x) = −α(x) +

γ(x)

(x)β(x)

(



(x)β(x) − α(x) +

(x) + (β

(x)β(x))



, β(x) 6= 0,

−α(x), β(x) = 0.

(8.159)

Now, it follows from (8.159) that L

(x) ≥ 0, β(x) 6= 0, and, since V (·) is

a control Lyapunov function of (8.100), it follows from Theorem 6.7 that

(x) = −α(x) ≥ 0, for all x ∈ R = {x ∈ R

: x 6= 0, β(x) = 0}. Hence,

(8.159) yields L

(x) ≥ 0, x ∈ R

, so that all conditions of Corollary 8.7 are

satisﬁed.

Theorem 8.12 shows that given a nonlinear system for which a control

Lyapunov function can be constructed, the feedback control law given by

(8.155) is inverse optimal with respect to a meaningful cost functional and

has a sector (and , hence, gain) margin (

, ∞).

Example 8.5. In this example, we demonstrate the extra ﬂexibility

provided by inverse optimal controllers with performance criteria involving

cross-weighting terms over inverse optimal controllers with meaningful cost

functionals. Speciﬁcally, we consider the controlled Lorentz dynamical

system discussed in Example 8.2. Our objective is to design and compare

inverse optimal and meaningful inverse optimal controllers that stabilize the

origin of the Lorentz equations (8.80)–(8.82). In particular, we compare the

guaranteed gain and sector margins to input saturation-type nonlinearities

and the tr ansient performance in terms of maximum overshoot of both

designs. Recall that the control law φ(x) = −(

σ + r)x

stabilizes the

nonlinear system (8.80)–(8.82) while minimizing the performance functional

(8.102) with L

(x) given by (8.85) and L

(x) given by (8.84). In this case,

(8.119) becomes

(1 − θ

)[ˆx

Qˆx + 2p

] − ˆx

ˆx ≥ 0, (8.160)