Haddad W.M. Nonlinear Dynamical Systems and Control: A Lyapunov-Based Approach

Подождите немного. Документ загружается.

NonlinearBook10pt November 20, 2007

DISSIPATIVITY THEORY FOR NONLINEAR SYSTEMS 405

iv) |σ(u) − cu|

≤ r

|u|

, u ∈ R, where c

△

(α + β) and r

△

(α −β).

Problem 5.54. Consider the damped Mathieu equation

¨x(t)+2µ ˙x(t)+(µ

−q cos ωt)x(t) = 0, x(0) = x

, ˙x(0) = ˙x

, t ≥ 0,

(5.315)

where q, µ, a > 0. Use the circle criterion to obtain suﬃ cient conditions

in terms of q, µ, and a such that the zero solution x(t) ≡ 0 to (5.315) is

globally exponentially stable. For which values of ω does your result hold?

Is stability guaranteed if (cos ωt)x(t) is replaced by σ(x, t), where σ(·, ·)

is a memoryless time-varying nonlinearity belonging to the sector [−1, 1]?

Explain your answer.

Problem 5.55. Consider the absolute s tability problem with forward

dynamics given by G(s) =

(s+1)(s+2)(s+3)

. Use the circle criterion to

determine the largest range [M

, M

] such that the feedback system is

globally exponentially stable for all memoryless, time-varying nonlinearities

σ(·, ·) belonging to the sector [M

, M

]. Repeat your analysis for G(s) =

(s−1)(s+1)

Problem 5.56. Consider the feedback system shown in Figure 5.3 with

G(s) =

(s+1)

. Use the positivity and Popov theorems to determine the

maximum allowable slope M on the saturation n onlinearity such that the

feedback system is globally (uniformly) asymptotically stable. Repeat your

analysis for G(s) =

s+3

+7s+10

Problem 5.57. Consider the damped Matheiu equation

¨x(t) + a ˙x(t) + (A + B cos t)x(t) = 0, x(0) = x

, ˙x(0) = ˙x

, t ≥ 0,

(5.316)

where a > 0. Give suﬃcient conditions such th at the zero solution to (5.316)

is asymptotically stable. Show th at this condition determines a region in

the (A, B) plane wh ich is bounded by straight lines and a parabola. (Hint:

Use Corollary 5.8 to obtain suﬃcient conditions for exponential stability.)

Problem 5.58 (Shift ed Popov Criterion). Consider the controllable

and observable dynamical system

˙x(t) = Ax(t) + Bu(t), x(0) = x

, t ≥ 0, (5.317)

y(t) = Cx(t), (5.318)

where x(t) ∈ R

, u(t) ∈ R

, and y(t) ∈ R

, with feedback nonlinearity

u(t) = −σ(y(t)), (5.319)

NonlinearBook10pt November 20, 2007

406 CHAPTER 5

where

σ(·) ∈ Φ

△

= {σ : R

→ R

: σ(0) = 0, [σ(y) −M

[σ(y) − M

y] ≤ 0,

y ∈ R

, and σ(y) = [σ

), σ

), . . . , σ

)]

= diag[M

, M

, . . . , M

], M

= diag[M

, M

, . . . , M

], and M

−M

0. Show that if there exists N = d iag[N

, N

, . . . , N

], N

≥ 0, i = 1, . . . , m,

such that I

+(M

−M

)(I

+N s)(I

+G(s)M

)

−1

G(s) is strictly positive

real and det(I

+ λN) 6= 0, where G(s) = C(sI

−A)

−1

B and λ ∈ spec(A),

then the feedback interconnection (5.317)–(5.319) is asymptotically stable

for all σ(·) ∈ Φ. (Hint: Use the shifted Lur´e-Postnikov Lyapunov function

candidate

V (x) = x

P x + 2

i=1

[σ

(s) − M

s]N

ds, (5.320)

where y

∈ R denotes th e ith component of y ∈ R

, and state un der wh at

conditions this is a valid Lyapunov function.)

Problem 5.59 (Oﬀ-Axis Circle Criterion). Show that in the case whe-

re m = 1 the frequency domain condition for the shifted Popov criterion

given in P roblem 5.58 involves a f requency domain test in the Nyquist plane

in terms of a family of frequ en cy-dependent oﬀ-axis circles. In addition,

show that the circle centers vary as a function of the phase of the Popov

multiplier, but each circle has the s ame real axis intercepts. Give the real

axis intercepts and the centers of the circles.

Problem 5. 60 (Parabola Criterion). Show that in the case where m =

1 and M

> 0, the frequency domain condition for the shifted Popov

criterion given in Problem 5.58 can be shown to provide a frequency domain

test in the Popov plane in terms of a ﬁ xed parabola. Give the real axis

intercepts of the parabola and show that the parabola will be tangent to

straight lines drawn through the crossings of the real axis intercepts with

slopes ±1/N.

Problem 5.61. Consider the nonlinear dynamical system G given by

˙x(t) = f (x(t)) + G(x(t))u(t), x(0) = x

, t ≥ 0, (5.321)

y(t) = h(x(t)) + J(x(t))u(t), (5.322)

u(t) = −σ(y(t), t), (5.323)

where x ∈ R

, u, y ∈ R

, f : R

→ R

, G : R

→ R

n×m

, h : R

→ R

J : R

→ R

m×m

, and σ(·, ·) ∈ Φ

prg

, where

prg

△

= {σ : R

× R

→ R

: σ(0, ·) = 0, σ

(y, t)y ≥ 0, y ∈ R

, a.e.

t ≥ 0, and σ(y, ·) is Lebesgue measurable for all y ∈ R

Furth ermore, suppose that G is zero-state observable and exponentially

NonlinearBook10pt November 20, 2007

DISSIPATIVITY THEORY FOR NONLINEAR SYSTEMS 407

passive with a continuously diﬀerentiable, radially unbounded storage

function V

(·). Show that the negative feedb ack interconnection of (5.321)–

(5.323) is globally uniformly asymptotically stable for all σ(·, ·) ∈ Φ

prg

Problem 5.62. Consider the nonlinear dynamical system G given by

˙x(t) = f(x(t)) + G(x(t))u(t), x(0) = x

, t ≥ 0, (5.324)

y(t) = h(x(t)) + J(x(t))u(t), (5.325)

u(t) = σ(y(t), t), (5.326)

where x ∈ R

, u ∈ R

, y ∈ R

, f : R

→ R

, G : R

→ R

n×m

h : R

→ R

, J : R

→ R

l×m

, and σ(·, ·) ∈ Φ

. Furthermore, s uppose

that G is zero-state observable and exponentially nonexpansive with a

continuously diﬀerentiable, radially unbounded storage function V

(·). Show

that the feedback interconnection of (5.324)–(5.326) is globally uniformly

asymptotically stable for all σ(·, ·) ∈ Φ

Problem 5.63. Consider the nonlinear dynamical system G given by

˙x(t) = f(x(t)) + G(x(t))u(t), x(0) = x

, t ≥ 0, (5.327)

y(t) = h(x(t)) + J(x(t))u(t), (5.328)

u(t) = −σ(y(t), t), (5.329)

where x ∈ R

, u, y ∈ R

, f : R

→ R

, G : R

→ R

n×m

, h : R

→

, J : R

→ R

m×m

, and σ(·, ·) ∈ Φ

. Furthermore, suppose that G

is zero-state observable and exponentially dissipative with respect to the

supply rate r(u, y) = u

u + u

My and with a continuously diﬀerentiable,

radially unbounded storage function V

(·), where M = M

∈ R

m×m

. Show

that the feedback interconnection of (5.327)–(5.329) is globally uniformly

asymptotically stable for all σ(·, ·) ∈ Φ

. How would you modify r(u, y) to

address σ(·, ·) ∈ Φ?

Problem 5.64. Consider the nonlinear dynamical system G given by

˙x(t) = f(x(t)) + G(x(t))u(t), x(0) = x

, t ≥ 0, (5.330)

y(t) = h(x(t)), (5.331)

u(t) = −σ(y(t)), (5.332)

where x ∈ R

, u, y ∈ R

, f : R

→ R

, G : R

→ R

n×m

, h : R

→ R

and σ(·) ∈ Φ

. Furthermore, suppose that G is zero-state observable and

exponentially dissipative with respect to the supply rate r(u, y) = 2u

y +

My + ˙y

Nu and with a continuously diﬀerentiable, radially unbounded

storage function V

(·), where M is positive deﬁnite and N is a nonnegative-

deﬁnite diagonal matrix. In addition, s uppose that y(t) + N ˙y(t) 6= 0, t ≥ 0,

for u(t) ≡ 0. Show that the negative feedback interconnection of (5.327)–

(5.329) is globally asymptotically stable for all σ(·) ∈ Φ

. (Hint: To address

NonlinearBook10pt November 20, 2007

408 CHAPTER 5

˙y in the supply rate r(u, y) form an augmented system with output ˆy =

˙y

]

and construct a supply rate of the form r(u, ˆy).)

Problem 5.65. Consider the nonnegative dynamical system G (see

Problem 5.9) given by (5.160) and (5.161) and assume that (A, C) is

observable and G is exponentially dissipative with respect to the s upply rate

s(u, y) = e

u − e

My, where M >> 0. Show that the positive feedback

interconnection of G and σ(·, ·) is globally uniformly asymptotically stable

for all σ(·, ·) ∈ Φ, where

△

= {σ : R

× R

→ R

: σ(·, 0) = 0, 0 ≤≤ σ(t, y) ≤≤ M y, y ∈ R

a.e. t ≥ 0, and σ(·, y) is Lebesgue measurable for all y ∈ R

(5.333)

M >> 0, and M ∈ R

m×l

. (Hint: Use Problem 5.11 to s how that if G is

exponentially dissipative with respect to the supply rate s(u, y) = e

u −

My, then there exists p ∈ R

, l ∈ R

, and w ∈ R

, and a scalar ε > 0

such that

0 = A

p + εp + C

e + l, (5.334)

0 = B

p + D

e − e + w. (5.335)

Now, use the Lyapunov function candidate V (x) = p

x.)

5.11 Notes and References

The original work on dissipative dynamical systems is due to J. C.

Willems [456, 457]. Lagrangian and Hamiltonian dynamical systems arose

from Euler’s variational calculus and are due to the fundamental work of

Joseph-Louis Lagrange [251] on analytical mechanics and William Rowan

Hamilton’s work on least action [183], developed in the eighteenth and

nineteenth centuries, respectively. Port-controlled Hamiltonian systems

were introduced by Maschke, van der Schaft, and Breedveld [305] and

Maschke and van der Schaft [303, 304]. See also van der Schaft [441].

Theorem 5.6 pr esenting necessary an d su ﬃcient conditions for dissipativity

with respect to qu ad ratic supply rates is due to Hill and Moylan [188]. The

concepts of exponential dissipativity, exponential passivity, and exponential

nonexpansivity are due to Chellaboina and Haddad [88]. The concepts

of input strict passivity, output strict passivity, and input-output strict

passivity are also due to Hill and Moylan [189]. The classical concepts

of passivity and nonexpansivity can be found in Popov [362, 364], Zames

[476, 477], Sandberg [389], and Deso er and Vidyasagar [104]. Positive real

and bounded real trans fer functions are discussed in Anderson [7, 8] and

Anderson and Vongpanitlerd [11]. The Kalman-Yakubovich-Popov lemma,

also known as the positive real lemma, was discovered ind ependently by

NonlinearBook10pt November 20, 2007

DISSIPATIVITY THEORY FOR NONLINEAR SYSTEMS 409

Kalman [226] and Yakubovich [468]. For an excellent treatment of the

positive real lemma and the boun ded real lemma see Anderson [7, 8] and

Anderson and Vongpanitlerd [11]. The linearization results for dissipative

and exponential dissipative dynamical systems are due to Haddad and

Chellaboina [158] and Chellaboina and Haddad [88].

The ab s olute stability problem was ﬁrst formulated in 1944 by A. I.

Lur´e and V. N. Postnikov [292]. In particular, suﬃcient conditions for

absolute stability in terms of a set of quadratic equations were derived using

a Lyapunov function containing a quadratic plus an integral term involving

the feedback nonlinearity [290,291]. This approach was further developed in

a series of papers by Yakubovich [467], Malkin [297], and Rozenvasser [372].

The frequency domain approach to absolute stability was ﬁrst developed by

the Rumanian mathematician Vasile-Mikhai Popov [361]. Yakubovich was

the ﬁrst to show that the Lur´e-Postnikov Lyapunov function is necessary

for proving the Popov criterion [468]. Ever since Popov derived a frequency

domain condition for absolute stability, considerable eﬀort by numerous

researchers was devoted in deriving similar criteria for absolute stability

[5, 64, 65, 67, 70, 71, 94, 326, 328–331, 361, 362, 364, 425, 427, 458, 476, 479].

The circle criterion evolved from this activity and was ﬁrst derived by

Bongiorno [64], with a Lyapunov function proof ﬁrs t given by Narendra

and Goldwyn [327]. An extensive development of absolute stability theory

is given in the classical monographs of Aizerman and Gantmacher [5],

Lefschetz [265], Popov [364], and Narendra and Taylor [331].

NonlinearBook10pt November 20, 2007

Chapter Six

Stability and Optimality of Feedback

Dynamical Systems

6.1 Introduction

In this chapter, we use the stability and dissipativity results developed

in Chapters 3–5 to d evelop stability and optimality results for feedback

dynamical s ystems. Speciﬁcally, general stability criteria are given for

Lyapunov, asymptotic, and exponential stability of feedback dynamical

systems. Furthermore, energy-based controllers for port-controlled Hamil-

tonian systems are established using passivity theory. In particular,

constructive suﬃcient conditions for feedback stabilization are derived that

provide a sh aped energy function for the closed-loop system, while preserving

the Hamiltonian structure at the closed-loop system level. In addition,

relative stability margins are also derived for nonlinear regulators, and sector

and disk margins are introduced as a generalization of classical gain and

phase margins. The notion of a control Lyapunov function is also introduced,

and it is shown that the existence of a smooth control Lyapunov function

implies smooth (almost everywhere) stabilizability of a controlled nonlinear

dynamical system. Next, a nonlinear control problem involving a notion

of optimality with respect to a non linear-nonquadratic cost fun ctional is

introduced. Finally, we close the chapter by introducing the notions of

feedback linearization, zero dynamics, and minimum-phase systems.

6.2 Feedback Interconnections of Dissipative Dynamical Systems

In this section, we consider feedb ack interconnections of dissipative dynam-

ical systems. Speciﬁcally, using the notion of dissipative and exponentially

dissipative dynamical systems, with appropriate storage functions and

supply rates, we construct Lyapunov functions for interconnected dynamical

systems by appropriately combining storage functions for each subsystem.

The feedb ack system can be nonlinear and either dyn amic or static. In

the dynamic case, for generality, we allow the nonlinear feedback system

NonlinearBook10pt November 20, 2007

412 CHAPTER 6

(compensator) to be of ﬁxed dimens ion n

that may be less than the plant

order n.

We begin by considering the nonlinear dy namical system G given by

˙x(t) = f (x(t)) + G(x(t))u(t), x(0) = x

, t ≥ 0, (6.1)

y(t) = h(x(t)) + J(x(t))u(t), (6.2)

where x ∈ R

, u ∈ R

, y ∈ R

, f : R

→ R

satisﬁes f (0) = 0, G :

→ R

n×m

, h : R

→ R

satisﬁes h(0) = 0, and J : R

→ R

l×m

, w ith the

nonlinear feedback system G

given by

˙x

(t) = f

(t)) + G

(t), x

(t))u

(t), x

(0) = x

, t ≥ 0, (6.3)

(t) = h

(t), x

(t)) + J

(t), x

(t))u

(t), (6.4)

where x

∈ R

, u

∈ R

, y

∈ R

, f

: R

→ R

satisﬁes f

(0) = 0,

: R

× R

→ R

×l

, h

: R

× R

→ R

satisﬁes h

(0, 0) = 0, and

: R

× R

→ R

m×l

. We assume that f (·), G(·), h(·), J(·), f

(·), G

(·),

(·, ·), and J

(·, ·) are continuous mappings and the required properties for

the existence and uniqueness of solutions of the feedback interconn ection of

G and G

are satisﬁed. Note that with the negative feedback interconnection

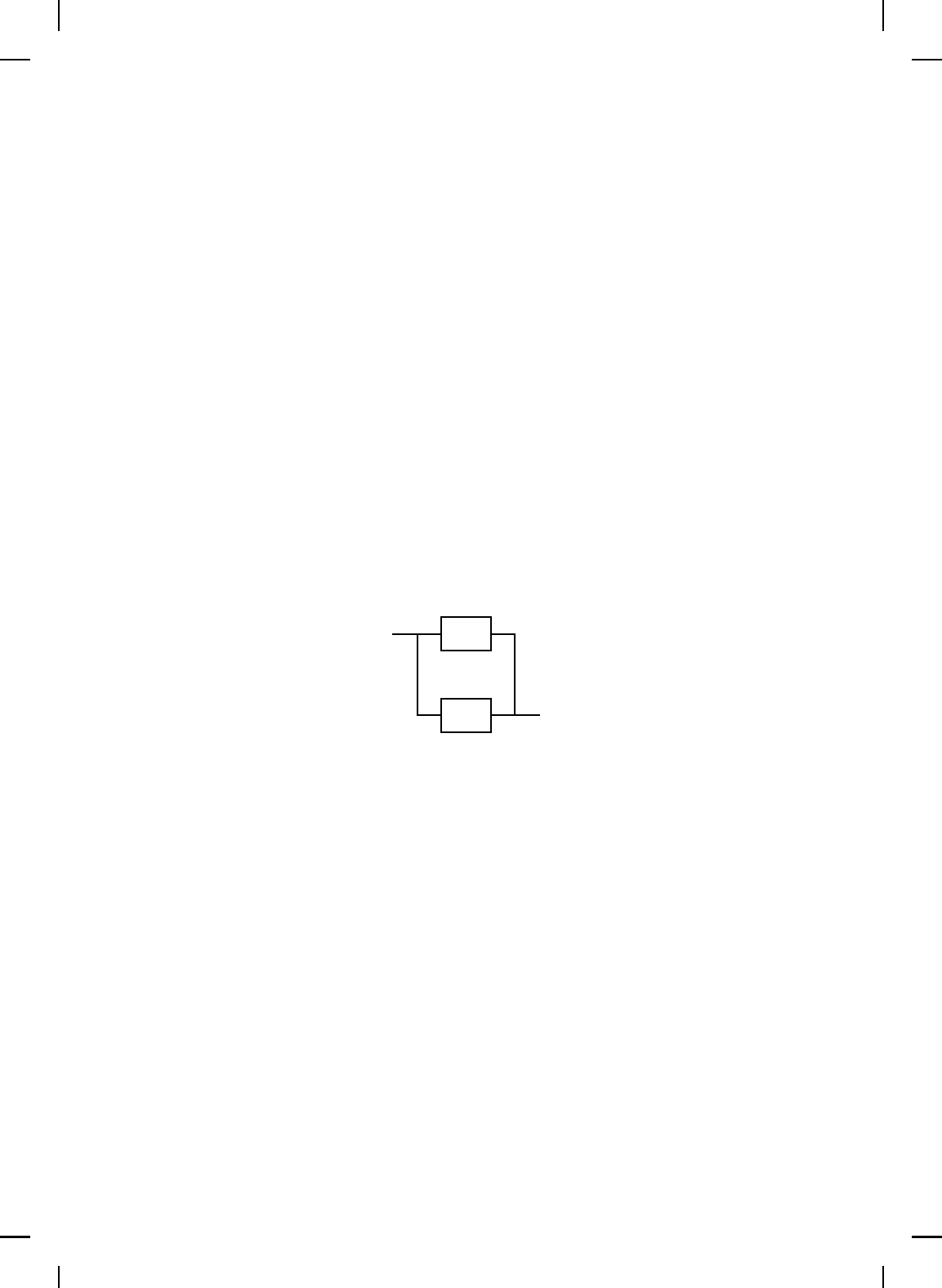
given by Figure 6.1, u

= y and y

= −u. Here and henceforth in the book



–

Figure 6.1 Feedback interconnection of G and G

we assume that the negative feedback interconnection of G and G

is well

posed, that is, det[I

+ J

(y, x

)J(x)] 6= 0 for all y, x, and x

The following results give s uﬃcient conditions for Lyapunov, asymp-

totic, and exponential stability of the feedback interconnection given by

Figure 6.1.

Theorem 6.1. Consider the closed-loop system consisting of the

nonlinear dynamical systems G and G

with inpu t-outpu t pairs (u, y) and

, y

), respectively, and with u

= y and y

= −u. Assume G and G

are

zero-state observable and dissipative with respect to the supply rates r(u, y)

and r

, y

) and with continuously diﬀerentiable, radially unbounded

storage functions V

(·) and V

(·), respectively, such that V

(0) = 0 and

(0) = 0. Furthermore, assume there exists a scalar σ > 0 such that

NonlinearBook10pt November 20, 2007

STABILITY OF FEEDBACK SYSTEMS 413

r(u, y) + σr

, y

) ≤ 0. Then the following statements hold:

i) The negative feedback interconnection of G an d G

is Lyapunov s table.

ii) If G

is exponentially dissipative with respect to supply rate r

, y

)

and rank[G

, 0)] = m, u

∈ R

, then the negative feedback

interconnection of G and G

is globally asymptotically stable.

iii) If G and G

are exponentially dissipative with respect to su pply r ates

r(u, y) and r

, y

), respectively, and V

(·) and V

(·) are such that

there exist constants α, α

, β, and β

> 0 such that

αkxk

≤ V

(x) ≤ βkxk

, x ∈ R

, (6.5)

≤ V

) ≤ β

, x

∈ R

, (6.6)

then the negative feedback interconnection of G and G

is globally

exponentially stable.

Proof. i) Consider the Lyapunov fun ction candidate V (x, x

) = V

(x)

+σV

). Now, the corresponding Lyapunov derivative is given by

V (x, x

) =

(x)+ σ

) ≤ r(u, y) + σr

, y

) ≤ 0, (x, x

) ∈ R

×R

which implies that the negative feedback interconnection of G and G

Lyapunov s table.

ii) If G

is exponentially dissipative it follows that for some scalar

> 0,

V (x, x

) =

(x) + σ

)

≤ −σε

) + r(u, y) + σr

, y

)

≤ −σε

), (x, x

) ∈ R

×R

Next, let R

△

= {(x, x

) ∈ R

× R

V (x, x

) = 0} and, since V

)

is positive deﬁnite, note that

V (x, x

) = 0 only if x

= 0. Now, since

rank[G

, 0)] = m, u

∈ R

, it follows that on every invariant set M

contained in R, u

(t) = y(t) ≡ 0, and hence, by (6.4), u(t) ≡ 0 so that ˙x(t) =

f(x(t)). Now, since G is zero-state observable it follows that M = {(0, 0)}

is th e largest invariant set contained in R. Hence, it follows from Theorem

3.5 that (x(t), x

(t)) → M = {(0, 0)} as t → ∞. Now, global asymptotic

stability of the negative feedback interconnection of G and G

follows from

the f act that V

(·) and V

(·) are, by assumption, radially unbounded.

iii) Finally, if G and G

are exponentially dissipative it f ollows that

V (x, x

) =

(x) + σ

)

≤ −εV

(x) − σε

) + r(u, y) + σr

, y

)

NonlinearBook10pt November 20, 2007

414 CHAPTER 6

≤ −min{ε, ε

}V (x, x

), (x, x

) ∈ R

× R

which implies that the negative feedback interconnection of G and G

globally exponentially stable.

The next result presents Lyapunov, asymptotic, and exponential

stability of dissipative feedback systems with quadr atic supply rates.

Theorem 6.2. Let Q ∈ S

, S ∈ R

l×m

, R ∈ S

, Q

∈ S

, S

∈ R

m×l

and R

∈ S

. Consider the closed-loop system consisting of the nonlinear

dynamical systems G given by (6.1) and (6.2) and G

given by (6.3) and

(6.4), and assume G and G

are zero-state observable. Furthermore, assu me

G is dissipative with respect to the quadratic supply rate r(u, y) = y

Qy +

Su + u

Ru and has a continuously diﬀerentiable, radially unbounded

storage function V

(·), and G

is diss ipative with respect to the quadratic

supply rate r

, y

) = y

+ 2y

+ u

and has a continuously

diﬀerentiable, radially unbounded storage fun ction V

(·). Finally, assume

there exists σ > 0 such that

△



Q + σR

−S + σS

−S

+ σS

R + σQ



≤ 0. (6.7)

Then the following statements hold:

i) The negative feedback interconnection of G an d G

is Lyapunov s table.

ii) If G

is exponentially dissipative with respect to supply rate r

, y

)

and rank[G

, 0)] = m, u

∈ R

, then the negative feedback

interconnection of G and G

is globally asymptotically stable.

iii) If G and G

are exponentially dissipative with respect to su pply r ates

r(u, y) and r

, y

) and there exist constants α, β, α

, and β

> 0 such

that (6.5) and (6.6) hold, then the negative feedback interconnection

of G and G

is globally exponentially stable.

iv) If

Q < 0, then the negative feedback interconnection of G and G

globally asymptotically stable.

Proof. Statements i)–iii) are a d irect consequence of Theorem 6.1 by

noting that

r(u, y) + σr

, y

) =









and h en ce, r(u, y) + σr

, y

) ≤ 0.

To show iv) consider the Lyapunov function candidate V (x, x

) =

(x) + σV

). Noting that u

= y and y

= −u it follows that the