Haddad W.M. Nonlinear Dynamical Systems and Control: A Lyapunov-Based Approach

Подождите немного. Документ загружается.

NonlinearBook10pt November 20, 2007

DYNAMICAL SYSTEMS AND DIFFERENTIAL EQUATIONS 105

Proposition 2.36. Consider the nonlinear d ynamical system G given

by (2.224). Then x ∈ D is a periodic point of G if and only if there exists

T 6= 0 such that x = s(T, x).

Proof. Necessity is immediate. To show suﬃciency let t ∈ R and note

that s(t, x) = s(t, s(T, x)) = s(t + T, x), w hich proves the result.

The next theorem establishes an important relationship between

periodic points, ω-limit sets, and positive orbits.

Theorem 2.43. Consider th e nonlinear dynamical system G given by

(2.224). Then x ∈ D is a periodic point of G if and only if O

= ω(x).

Proof. Let O

= ω(x). In this case, x ∈ ω(x) and since ω(x) is

invariant if follows that O

= ω(x) = O

. Hence, s(τ, x) ∈ O

for each

τ < 0, an d hence, there exists µ ≥ 0 such that s(τ, x) = s(µ, x). Now, it

follows from the group axiom that s(t, x) = s(t+µ−τ, x) for all t ∈ R, which

establishes that x is periodic with period T = µ − τ > 0. The converse is

immediate.

For ﬁnite-dimensional dynamical systems wherein D ⊆ R

, it can be

shown that x ∈ D is a periodic point of G if and only if ω(x) = α(x) = O

(See Problem 2.127.)

It is important to distinguish between trivial periodic orbits and

nontrivial periodic orbits or limit cycles. To see the distinction consider

the dynamical equations for the simple h armonic oscillator given by

˙x

(t) = x

(t), x

(0) = x

, t ≥ 0, (2.225)

˙x

(t) = −x

(t), x

(0) = x

, (2.226)

where the solution is characterized by

(t) = A cos(t −θ

), x

(t) = −A sin(t −θ

), (2.227)

where A =

+ x

and θ

= tan

−1

(

). Note th at the solution to

(2.225) and (2.226) is periodic for all initial conditions x

, x

∈ R. That

is, given any initial condition in the plane x

-x

, one can ﬁnd a periodic

solution passing through this point. This is called a trivial periodic orbit.

This is in contrast to a nontrivial periodic orbit or limit cycle wherein a

dynamical system possesses an isolated periodic orbit, that is, there exists a

neighborhood of the periodic orbit that does not contain any other periodic

solution. To see this, cons ider once again (2.220) and (2.221) given in

Example 2.38. In particular, using separation of variables the solution to

NonlinearBook10pt November 20, 2007

106 CHAPTER 2

(2.220) and (2.221) is given by

r(t) =



1 +



− 1



−2t



−1/2

, (2.228)

θ(t) = t + θ

. (2.229)

Clearly, (2.228) shows that (2.220) and (2.221) has only one nontrivial

periodic solution corresponding to r = 1, that is, x

+ x

= 1. In light

of the above observations we have the following deﬁnition for a nontrivial

periodic orbit or limit cycle.

Deﬁnition 2. 54. Consider the nonlinear dynamical system (2.224). A

limit cycle of (2.224) is a closed curve

Γ ⊂ R

such that Γ is the positive

limit set of the positive orbit O

of (2.224) or th e negative limit set of the

negative orbit O

−

of (2.224) for x 6∈ Γ.

Note that it follows from Deﬁnition 2.54 that a limit cycle is compact

and invariant. Furthermore, if a nontrivial periodic solution Γ is isolated

such that it is the positive limit set of the positive orbit O

of (2.224) or

the negative limit set of the negative orbit O

−

of (2.224) for x 6∈ Γ, then G

is a limit cycle.

Next, we present a key result due to Ivar Bendixson [39] that

guarantees the absence of limit cycles for planar systems. For this result,

we consider second-order nonlinear dynamical systems of the form

˙x

(t) = f

(t), x

(t)), x

(0) = x

, t ∈ R, (2.230)

˙x

(t) = f

(t), x

(t)), x

(0) = x

, (2.231)

where f

, x

) and f

, x

) are continuously diﬀerentiable. Furthermore,

deﬁne the divergence operator

∇f(x)

△

∂f

∂x

, x

) +

∂f

∂x

, x

). (2.232)

The following deﬁnition is required for the statement of Bendixson’s

theorem.

Deﬁnition 2.55. A s ubset D ⊆ R

is simply connected if there exist

y ∈ D and a continuous function g : [0, 1] × D → D su ch that g(0, x) = x

and g(1, x) = y for all x ∈ D.

Theorem 2.44 (Bendixson’s Theorem). Consider the second-order

nonlinear dynamical system (2.230) and (2.231) with vector ﬁeld f : D →

, where D is a simply connected region in R

such that there are no

Γ is a closed curve contained in R

if there exists a continuous mapping γ : [0, 1] → R

such

that γ(0) = γ(1), Γ = {γ(σ) : σ ∈ [0, 1]}, and Γ 6= {γ(0)}.

NonlinearBook10pt November 20, 2007

DYNAMICAL SYSTEMS AND DIFFERENTIAL EQUATIONS 107

equilibrium points of (2.230) and (2.231) in D. If there exists x ∈ D such

that ∇f(x) 6= 0 and ∇f(x) does not change sign in D, then (2.230) and

(2.231) has no periodic orbits lying entirely in D.

Proof. Suppose, ad absurdum, that Γ = {x ∈ D : x = x(t), 0 ≤ t ≤ T }

is a closed periodic orbit lying entirely in D. Then, for each x = (x

, x

) ∈ Γ,

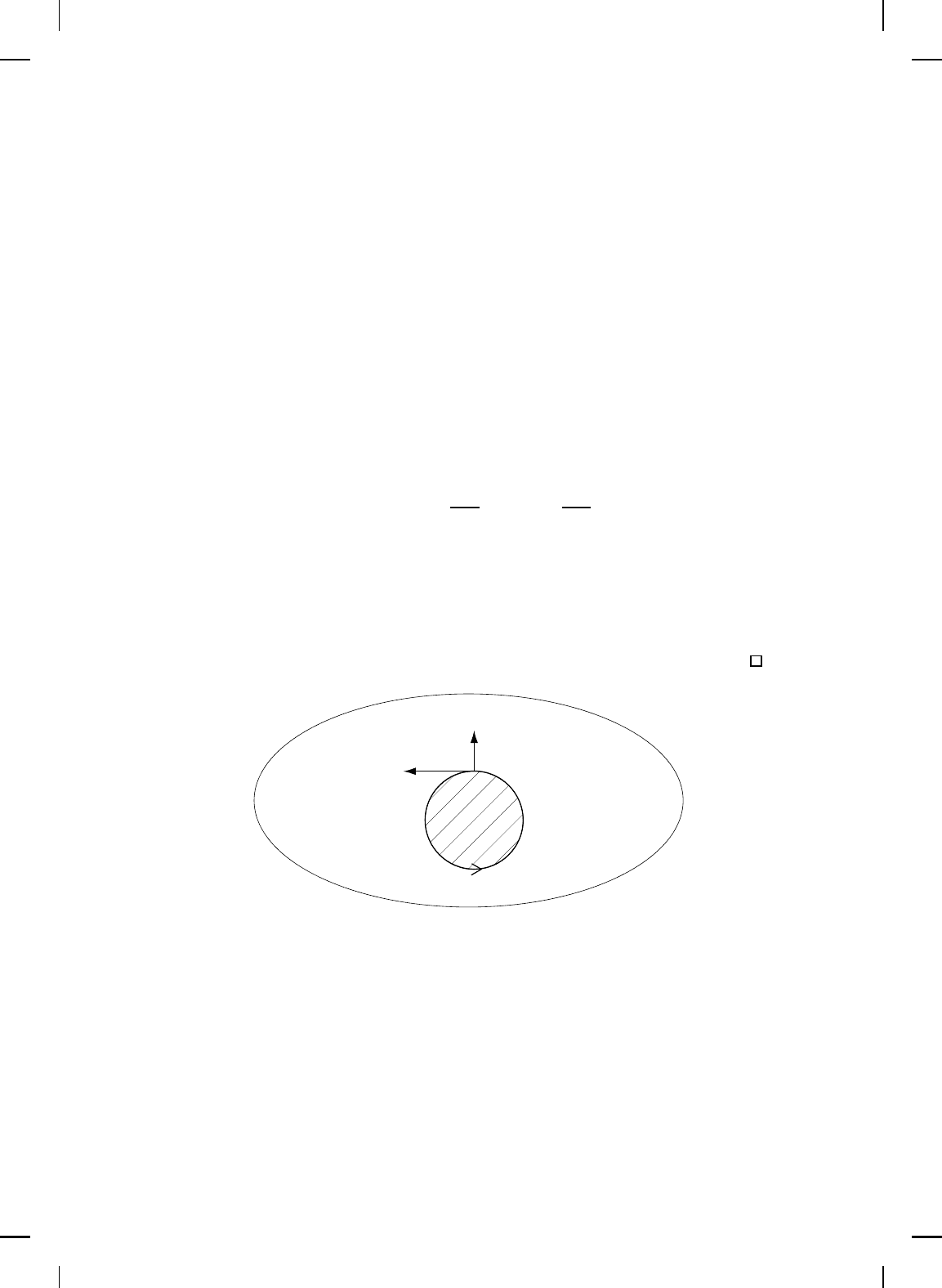
f(x) is tangent to Γ, that is, f(x)·n(x) = 0 for all x ∈ Γ, where n(x) denotes

the outward normal vector to Γ at x (see Figure 2.7). Hence, it follows from

Green’s theorem that

f(x) ·n(x)dΓ =

Z Z

∇f(x)dS = 0, (2.233)

or, equivalently,

, x

)dx

− f

, x

)dx

)

Z Z



∂f

∂x

, x

) +

∂f

∂x

, x

)



= 0, (2.234)

where S is the area enclosed by Γ. Now, if there exists x ∈ D such that

∇f(x) 6= 0 and ∇f (x) does not change sign in S, then it follows from

the continuity of the divergence operator ∇ f (x) in D that (2.233) is either

positive or negative, which leads to a contradiction. Hence, D contains no

periodic solutions of (2.230) and (2.231).

n(x)

f(x)

Figure 2.7 Visualization of sets and vector ﬁeld used in the proof of Theorem 2.30.

Example 2.39. Consider the second-order nonlinear dynamical system

¨x(t) + α ˙x(t) + g(x(t)) = 0, x(0) = x

, ˙x(0) = ˙x

, t ≥ 0, (2.235)

where α 6= 0 and g : R → R is continuous and satisﬁes g(0) = 0. Note that

with x

= x and x

= ˙x, (2.235) can be equivalently written as

˙x

(t) = x

(t), x

(0) = x

, t ≥ 0, (2.236)

NonlinearBook10pt November 20, 2007

108 CHAPTER 2

˙x

(t) = −g(x

(t)) −αx

(t), x

(0) = ˙x

. (2.237)

Now, computing ∇f(x

, x

) yields

∇f(x

, x

) =

∂f

∂x

, x

) +

∂f

∂x

, x

) = −α 6= 0, (x

, x

) ∈ R ×R.

(2.238)

Hence, it follows from Bendixson’s theorem that (2.235) has no limit cycles

in R

. It is interesting to note that for the special case where g(x) = −x+x

and α > 0, (2.235) is known as Duﬃng’s equation. △

Example 2.40. Consider the van der Pol oscillator given by

¨x(t)−ε[1−x

(t)] ˙x(t)+ x(t) = 0, x(0) = x

, ˙x(0) = ˙x

, t ≥ 0, (2.239)

where ε > 0. Now, with x

= x and x

= ˙x, (2.239) can be equivalently

written as

˙x

(t) = x

(t), x

(0) = x

, t ≥ 0, (2.240)

˙x

(t) = −x

(t) + ε[1 −x

(t)]x

(t), x

(0) = ˙x

. (2.241)

Computing ∇f(x

, x

) yields

∇f(x

, x

) =

∂f

∂x

, x

) +

∂f

∂x

, x

) = ε(1 −x

). (2.242)

Hence, it follows from Bendixson’s theorem th at if the van der Pol oscillator

were to possess a periodic solution it would have to intersect {(x

, x

) : x

1} or {(x

, x

) : x

= −1} or both sets. △

Example 2.41. Consider the linear dynamical system

˙x(t) = Ax(t), x(0) = x

, t ≥ 0, (2.243)

where x(t) ∈ R

, t ≥ 0, and A ∈ R

2×2

. From linear sys tem theory it

follows that a necessary and suﬃcient condition f or the existence of periodic

solutions to (2.243) is Re λ = 0 and Im λ 6= 0, where λ ∈ spec(A). Recall

that the eigenvalues of A for a second-order linear system are given by

det(λI

−A) = λ

− tr Aλ + det A = 0. (2.244)

Hence, (2.243) possesses periodic solutions if and only if tr A = 0 and

det A > 0. A contrapositive statement to the above yields that the absence

of period ic solutions will be guaranteed if and only if tr A 6= 0 or det A ≤ 0.

Using Bendixson’s theorem it follows th at ∇f (x) = tr A, x ∈ R

. Requiring

tr A 6= 0 thus guarantees the absence of periodic solutions to second-order

linear systems. △

A more general criterion for ruling out closed orbits in a simply

connected region of R

is due to Dulac.

NonlinearBook10pt November 20, 2007

DYNAMICAL SYSTEMS AND DIFFERENTIAL EQUATIONS 109

Theorem 2.45 (Dulac’s Theorem). Consider the second-order non-

linear dynamical system (2.230) and (2.231) with vector ﬁeld f : D →

, where D is a simply connected region in R

such that there are no

equilibrium points of (2.230) and (2.231) in D. If there exists x ∈ D and a

continuously diﬀerentiable function g : D → R such that ∇(g(x)f(x)) 6= 0

and ∇(g(x)f(x)) does not change sign in D, th en (2.230) and (2.231) has

no closed orbits lying entirely in D.

Proof. The proof of this result is also based on Green’s theorem and

is virtually identical to the proof of Theorem 2.44.

Example 2.42. Consider the second-order nonlinear dynamical system

˙x

(t) = x

(t)[2 − x

(t) −x

(t)], x

(0) = x

, t ≥ 0, (2.245)

˙x

(t) = x

(t)[4x

(t) −x

(t) −3], x

(0) = x

. (2.246)

To show that (2.245) and (2.246) has no closed orb its in the positive

orthant R

, consider the Dulac function g(x

, x

) = 1/x

. Computing

∇(g(x

, x

)f(x

, x

)) yields

∇(g(x

, x

)f(x

, x

)) =

∂

∂x

(g(x

, x

)) +

∂

∂x

(g(x

, x

))

∂

∂x



2 − x

− x



∂

∂x



− x

− 3



= −1/x

< 0, x

> 0, x

> 0. (2.247)

Since {(x

, x

) ∈ R × R : x

> 0 and x

> 0} is simply connected in R

and g(·) is continuously diﬀerentiable it follows from Dulac’s theorem that

(2.245) and (2.246) has no closed orbits in R

. △

The next theorem is due to Henri Poincar´e [358–360] and Ivar

Bendixson [39] and gives conditions for the existence of periodic orbits for

planar systems.

Theorem 2.46 (Poincar´e-Bendixson Theorem). Consider the second-

order nonlinear dynamical system (2.230) and (2.231). Let O

be a positive

orbit of (2.230) and (2.231) with positive limit set ω(x

). If O

is contained

in a compact subset M of D ⊂ R

and ω(x

) contains no equilibria of (2.230)

and (2.231), then ω(x

) is a periodic orbit of (2.230) and (2.231).

An analogous result is valid for negative orbits O

−

with negative limit

set α(x

). The proof of the Poincar´e-Bendixson theorem is based on a series

of technical lemmas from algebraic topology involving positive limit sets for

two-dimensional systems and will not be given here. The interested r eader

is referred to [349, 454] for the proof. Nevertheless, the result itself is quite

NonlinearBook10pt November 20, 2007

110 CHAPTER 2

intuitive. In particular, the theorem states that if a compact s et M ⊂ R

can

be constructed that does not contain any equilibrium points of (2.230) and

(2.231) and all limit points of (2.230) and (2.231) are contained in M, th en

M must contain at least one period ic orbit. Of course, it is necessary to ﬁnd

such a region M. One way to do this is to construct M such that M does

not contain any equilibria of (2.230) and (2.231) and is positively invariant.

In this case, O

⊆ M for x ∈ M and since M is compact it contains all

its limit points, and hence, ω(x

) ⊆ M for x

∈ M. Hence, every compact,

nonempty positively invariant set M contains either an equilibrium point

or a periodic orbit. This result is stated as a theorem below.

Theorem 2.47. Consider the second-order n on linear dynamical sys-

tem (2.230) and (2.231). Let M be a compact, positively invariant set with

respect to (2.230) and (2.231). Then M contains an equilibrium point or a

periodic orbit.

Proof. If x ∈ M, then ω(x) is a nonempty subset of M, that is,

ω(x) ⊂ M. The result now f ollows as a direct consequence of th e Poincar´e-

Bendixson theorem.

An identical r esult is true for compact, negatively invariant sets.

Example 2.43. To illustrate the Poincar´e-Bendixson theorem we

consider once again the simple harmonic oscillator given by (2.225) and

(2.226). Now, letting V (x

, x

) = x

+ x

it follows that

V (x

, x

) =

˙x

+ 2x

˙x

= 0. Hence, the system trajectories of (2.225) and (2.226)

cannot cross the α-level set of V , that is, V

−1

(α) = {(x

, x

) ∈ R

V (x

, x

) = α}, for every α > 0. Now, deﬁne the [α, β]-sublevel set

△

= V

−1

([α, β]) = {(x

, x

) ∈ R

: α ≤ V (x) ≤ β}, where β > α > 0.

Note that M is compact and, since

V (x

, x

) = 0, positively invariant.

Hence, since M contains no equilibrium points it follows from the Poincar´e-

Bendixson theorem that M contains a periodic orbit. △

Example 2.44. Once again, consider the nonlinear dynamical system

(2.220) and (2.221) given in Example 2.38. Letting V (x

, x

) = x

+ x

follows that

V (x

, x

) = 2x

˙x

+ 2x

˙x

= −2x

+ 2x

(1 − x

− x

) + 2x

+ 2x

(1 − x

− x

)

= 2V (x

, x

)[1 − V (x

, x

)]. (2.248)

Note that

V (x

, x

) > 0 for V (x

, x

) < 1 and

V (x

, x

) < 0 for V (x

, x

) >

1. Hence, on the α-level set V

−1

(α), where 0 < α < 1, all the system

trajectories of (2.220) and (2.221) are moving out toward the circle x

= 1. Alternatively, on the β-level set V

−1

(β) for β > 1, all system

NonlinearBook10pt November 20, 2007

DYNAMICAL SYSTEMS AND DIFFERENTIAL EQUATIONS 111

trajectories of (2.220) and (2.221) are moving in toward the circle x

= 1.

Hence, M = {(x

, x

) ∈ R

: α ≤ V (x) ≤ β} is positively invariant.

Since M is compact and does not contain any equilibrium points, it follows

from the Poincar´e-Bendixson theorem that M contains a periodic orbit.

Furth ermore, since, for every 0 < α < 1 and β > 1, M contains a periodic

orbit it follows that C = {(x

, x

) ∈ R

: x

+ x

= 1} contains a periodic

orbit. △

2.14 Problems

Problem 2. 1. Let A ∈ R

n×n

be such that det A 6= 0. Show that there

exists ε > 0 such that kAxk ≥ εkxk, x ∈ R

, where k·k is a vector norm on

Problem 2.2. Let p ∈ [1, ∞], ¯p = p/(p − 1), and let A ∈ R

m×n

. Show

that:

i) kAk

2,2

= σ

max

(A).

ii) kAk

p,1

= max

i=1,...,n

kcol

(A)k

iii) kAk

∞,p

= max

i=1,...,m

krow

(A)k

¯p

Problem 2.3. Using the results of Problem 2.2 show that for A ∈

m×n

the f ollowing hold:

i) kAk

1,1

= max

i=1,...,n

kcol

(A)k

ii) kAk

∞,∞

= max

i=1,...,m

krow

(A)k

iii) kAk

∞,1

= max

1,...,m

kAk

∞

iv) kAk

2,1

= d

1/2

max

A).

v) kAk

∞,2

= d

1/2

max

(AA

In iv) and v) d

max

(X)

△

= max

i=1,...,n

(i,i)

for X ∈ R

n×n

Problem 2.4 (Pythagorean Theorem). Let x, y ∈ R

. Show that if

y = 0, then kx + yk

= kxk

+ kyk

Problem 2.5. Show that Axiom iv) of Deﬁnition 2.6 holds as an

equality if and only if x, y ∈ R

are linearly dependent.

NonlinearBook10pt November 20, 2007

112 CHAPTER 2

Problem 2.6. Show that the Euclidean norm satisﬁes Axioms i)–iv)

of Deﬁnition 2.6.

Problem 2.7. Show that equality holds in (2.18) if and only if |x

y| =

|x|

|y| and

|x| ◦|y| = kyk

∞

|x|, p = 1,

kyk

1/p

|x|

{1/q}

= kxk

1/q

|y|

{1/p}

, 1 < p < ∞,

|x| ◦|y| = kxk

∞

|y|, p = ∞,

where |z| ∈ R

is a vector whose components are the absolute values of

z ∈ R

, ◦ denotes the Hadamard product (i.e., component-by-component

product), and z

{α}

△

= [z

, . . . , z

]

Problem 2.8. Let S

△

= {x ∈ R

: −1 ≤ x

< 1, −1 ≤ x

< 1}. Obtain

the closure, interior, and boundary of S. Is S open? Is S closed?

Problem 2. 9. Show that the union of a ﬁnite number of bounded sets

is bounded.

Problem 2.10. Let S ⊆ R

. Show that x ∈ S if and only if B

(x)∩S 6=

Ø for every ε > 0.

Problem 2.11. Show that th e sequ en ce {x

}

∞

n=1

, where x

= (1 +

1/n)

, is a convergent sequence.

Problem 2.12. Let {x

}

∞

n=1

⊂ R. For i) x

= (−1)

(1 + 1/n), ii)

= (−1)

, iii) x

= (−1)

n, and iv) x

= n

sin

(

nπ

), compute the limit

inferior and limit superior of x

Problem 2.13. Consider the sequence of functions {f

}

∞

n=0

, where f

R → R is given by f

(x) = 1/(1 + x

). Sh ow that the sequence {f

}

∞

n=0

exhibits point wise convergence on R.

Problem 2.14. Consider the sequence of functions {f

}

∞

n=1

, wh ere

(x) = ln(1 + x) = x −

− ··· +

(−1)

n−1

+ ···. Show that

this sequence converges f or |x| < 1 and diverges for |x| > 1.

Problem 2.15. Consider the sequence of functions {f

}

∞

n=0

, where f

[0, 1] → R is given by f

(x) =

∞

n=0

)

. Show that the sequence

}

∞

n=1

converges uniformly to f (x) = e

Problem 2.16. Consider the sequence of functions {f

}

∞

n=1

, where f

[0, 1] → R is given by f

(x) = n

x(1 − x)

. Show that even though the

NonlinearBook10pt November 20, 2007

DYNAMICAL SYSTEMS AND DIFFERENTIAL EQUATIONS 113

sequence {f

}

∞

n=1

converges,

lim

n→∞

(x)dx 6=

lim

n→∞

(x)dx.

Problem 2.17. Construct an example of a convergent sequence of

diﬀerentiable functions {f

(x)}

∞

n=1

such that, for all x ∈ R, lim

n→∞

′

(x)

does n ot exist.

Problem 2.18. Let {x

}

∞

n=0

⊂ R be a scalar sequence. Show that:

i) inf x

≤ lim inf

n→∞

≤ lim su p

n→∞

≤ sup x

ii) {x

}

∞

n=0

converges if and only if lim inf

n→∞

= lim sup

n→∞

lim

n→∞

iii) If lim sup

n→∞

(respectively, lim inf

n→∞

) is ﬁnite, then show that

lim sup

n→∞

(respectively, lim inf

n→∞

) is the largest (respec-

tively, smallest) limit point of {x

}

∞

n=0

Problem 2.19. Explain why i) of T heorem 2.6 may not be true for

inﬁnite intersections.

Problem 2.20. Let f : R → R and g : R → R be given by f(x) = x−1

and g(x) = sin x. Find the composite maps f ◦g and g◦f. Is the composition

of fun ctions commutative?

Problem 2. 21. Let X ⊆ R and Y ⊆ R, and let f : X → Y be given by

f(x) = sin x. What is the inverse image of Y under f if i) X = Y = R and

ii) X = [−π/2, π/2] and Y = R.

Problem 2.22. Let A ∈ R

n×n

and deﬁne the map f : R

n×n

→ R

by f(A) = det A. Show that f is s urjective. Is f bijective? What is

−1

(R \ {0})?

Problem 2.23. Let f : R → R be given by f (x) = x

− 1. Find the

image of X under f, where X = [−1, 2] and X = R. Is f surjective, injective,

or bijective?

Problem 2.24. Let f : X → Y and let U ⊆ X be an open set. Prove

or refute that f(U) is open in Y.

Problem 2.25. Let X ⊆ R

and Y ⊆ R

, and let f : X → Y. Show

that f is injective if and only if f (Q) ∩ f(X \ Q) = Ø for all Q ⊆ X.

Problem 2.26. Let X and Y be sets, let A, A

, and A

be s ubsets of

NonlinearBook10pt November 20, 2007

114 CHAPTER 2

X, and let B be a subset of Y. Show that the following statements hold:

i) f (A

∩ A

) ⊆ f(A

) ∩f(A

ii) f

−1

(f(A)) ⊇ A.

iii) f(f

−1

(B)) ⊆ B.

iv) f(A

∩ A

) = f(A

) ∩f(A

) if and only if f is injective.

v) f

−1

(f(A)) = A if and only if f is inj ective.

vi) f(f

−1

(B)) = B if and only if f is surjective.

Problem 2.27. Prove or refute that the union of convex sets is convex.

Problem 2.28. Let C ⊂ R

and Q ⊂ R

be convex sets. Show that:

i) αC = {x ∈ R

: x = αy, y ∈ C} is convex, where α ∈ R.

ii) C + Q is convex.

Problem 2. 29. Let k·k : R

→ R be a vector norm on R

. Show that

k· k is convex.

Problem 2.30. Let I be an index set, C ⊂ R

be a convex s et, and

: C → R be a convex function for each i ∈ I. Show that g : C → R ∪{∞}

given by g(x) = sup

i∈I

(x) is convex.

Problem 2.31. Let C ⊂ R

be a convex set and let f : C → R be

continuously diﬀerentiable. Show that:

i) f is convex if and only if

f(y) ≥ f (x) + (y − x)

′

(x), x, y ∈ C. (2.249)

ii) If the inequality in (2.249) is s trict whenever x 6= y, then f is s trictly

convex.

Problem 2.32. Let C ⊂ R

be a convex set, let f : C → R be two-times

continuously diﬀerentiable, and let P be an n ×n symmetric matrix. Show

that:

i) I f f

′′

(x) ≥ 0, x ∈ C, then f is convex.

ii) If f

′′

(x) > 0, x ∈ C, x 6= 0, then f is strictly convex.