Gupta D. (Ed.). Diffusion Processes in Advanced Technological Materials

Подождите немного. Документ загружается.

damage in the Al. The variation of line resistance in the Cu alloy lines can

be roughly divided into three damage stages: an incubation stage, during

which resistance does not increase, followed by slow-increasing and

steady-state stages. During the incubation stage, an initial reduction in

resistance is observed, due to the depletion of the Sn solute in the grains,

which decreases the contribution of solute scattering to resistivity. Once

the void forms, the resistance of the lines starts to increase. Similar slopes

for pure Cu and Cu(0.5%Sn), as shown in Fig. 9.15, in the final steady-

state stage suggest that the Cu depletion rate in the Cu(Sn) sample is the

same as in pure Cu. This was also observed in our previous results for

sample temperatures above 250°C.

[28, 91]

The effect of Sn solute in bulk Cu is different from that in thin films.

In bulk Cu(Sn) samples, Sn solute enhances Cu and Sn diffusion in

Cu(Sn),

[106]

while solute Sn decreases Cu diffusion in Cu(Sn) grain bound-

aries of thin films.

[83]

These observed behaviors are similar to Pd in

Cu(Pd)

[93]

and Au in Au(Ta)

[107]

studies. The effect of Sn in Cu is similar

to Ta in Au and Pd in Cu. A 0.5 wt.% Sn addition can cause the time

required for ∆R  1Ω (equivalent to a 1.6-mm edge displacement) to

increase by a factor of 10 at 203°C. The nature of solute and solvent inter-

action at grain boundaries and surfaces is not clear. However, the obser-

vations of reducing Cu grain boundary diffusion in Cu(Sn) alloys can be

qualitatively interpreted in terms of the solute Sn reducing the grain

boundary energy

[108]

and/or acting as a trapping site

[109, 110]

for Cu. Both

models predict D(Cu)/D(Cu(Sn)) ∼ 1  ZC

exp((∆E  T∆S)kT),

where D(Cu) and D(Cu(Sn)) are the Cu diffusivities in pure Cu and

Cu(Sn) alloy, C

is the solute concentration, Z is the coordination number

for the solute atom, and ∆E and ∆S are the corresponding binding energy

and entropy for grain boundary and solute interaction, respectively. The

free Cu atoms or vacancies are drastically reduced in the fast paths

because of the Sn-Cu atom or Sn-vacancy interactions, which depend on

the diffusion mechanisms

[111]

in the grain boundaries. If we assume that

the ratio of time for ∆R  0.5Ω(∆L  0.8 mm) for pure Cu to Cu(Sn)

alloys is due to changes in effective diffusivity (that is, changes in Z

r are

small), then a binding energy ∆E of the order of 0.5 ± 0.3 eV between

Sn-Cu atoms and/or Sn-vacancy at grain boundaries is obtained from

Fig. 9.15. The binding energy ∆E ∼ 0.5 eV is the same order as the

increase in the activation energy for grain boundary diffusion observed in

Cu upon addition of 2 wt.% Sn.

[83]

In summary, electromigration mass transport rates in Blech-type test

structures have been observed. The drift velocity of Cu can be greatly

enhanced or reduced by solute addition. The electromigration surface acti-

vation energy in pure PVD-ion-milled Cu lines measured by void edge

displacement in nitrogen ambient was found to be 0.77 ± 0.04 eV. The

432 DIFFUSION PROCESSES IN ADVANCED TECHNOLOGICAL MATERIALS

Chapter-09 11/29/04 6:50 PM Page 432

ELECTROMIGRATION IN CU THIN FILMS, HUETAL. 433

corresponding edge displacement rate of Cu was increased by alloying

with Mg and slowed down with Pd, Zr, or Sn additions.

9.8 Lifetime Distribution

9.8.1 Single-Damascene Line on W Via

It is customary to plot the lifetime distribution in a log-normal

function, which translates the lifetime from a linear to a log scale. The log-

normal distribution, f, and log-normal cumulative probability, F, func-

tions

[22]

can be described as follows:

f  e





(lnt 

)



(13)

and

F  





(lnt 

)



dlnt, (14)

where t

and s are the median lifetime and the deviation for the log-

normal distribution function, respectively. A least-squares fitting method

was used to obtain the best adjustable fitting parameter values of t

ands

by minimizing c

, which is defined by:

[112]



i1

, (15)

where the values of F

and y

are the estimated function and data point at

ln(t

), respectively; e

is the uncertainty of y

; and n is the sample size. The

fitting procedure, which is dependent on the values of e

, is assumed to be

1 for the case of cumulative probability data. On the other hand, the fre-

quency count in failure time distribution is graphed as a histogram show-

ing the number of observations f

recorded for each ln(t

), and an error bar

for the frequency f

can be estimated as f

(1



n)



[113]

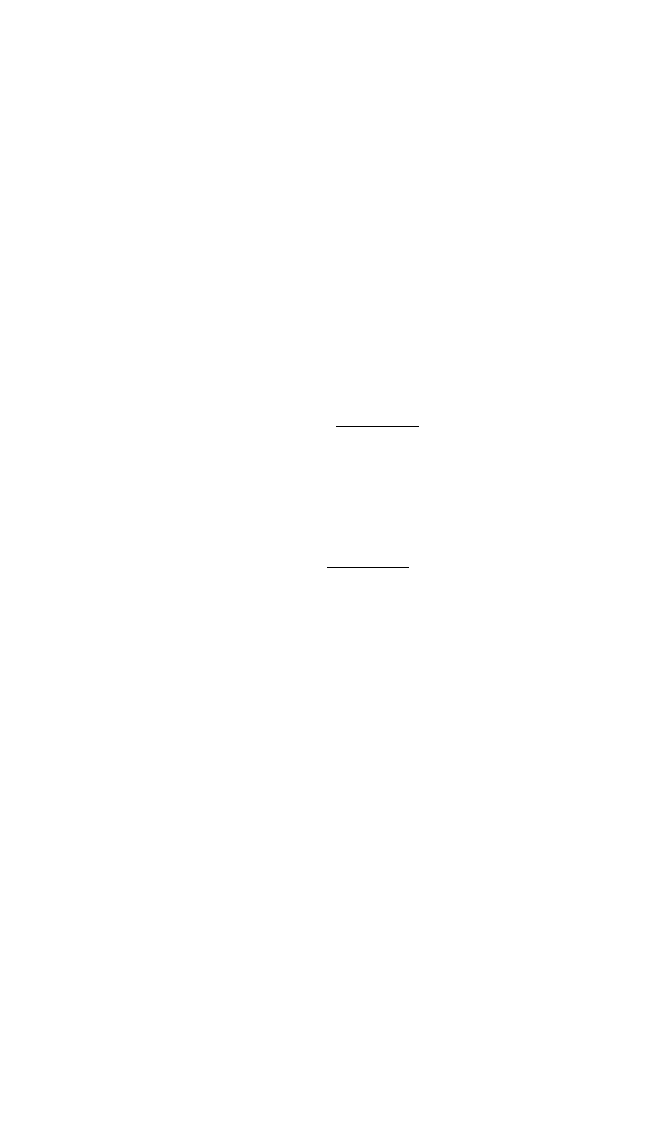
Figure 9.16(a) shows the normalized line resistance vs. time at a sam-

ple temperature T  296°C with current density, j, of 32 mAmm

in M1

and electron flow from W M0 to W CA to Cu M1 embedded in SiO

. The

line resistance initially slowly decreased, then increased, followed by a

 y

)



2ps



2ps



(lnt  lnt

)

(lnt  lnt

)

Chapter-09 11/30/04 3:43 PM Page 433

period of rapid rise. The initial line resistance decrease was most likely due

to reduction of contact resistance and/or purification of the Cu line. Asharp

resistance increase occurred when a void grew completely across the line or

line/via interface as the current had to pass through the high-resistance thin

liner. With this rapid increase in line resistance, the lifetime difference

between ∆RR  1% and ∆RR  20% in most of the samples tested was

small. However, if the thickness of the liner were increased, there would

have been a gradual line resistance increase during this time period, as

shown in the case of Fig. 9.7. Here the lifetime defined by time at ∆RR



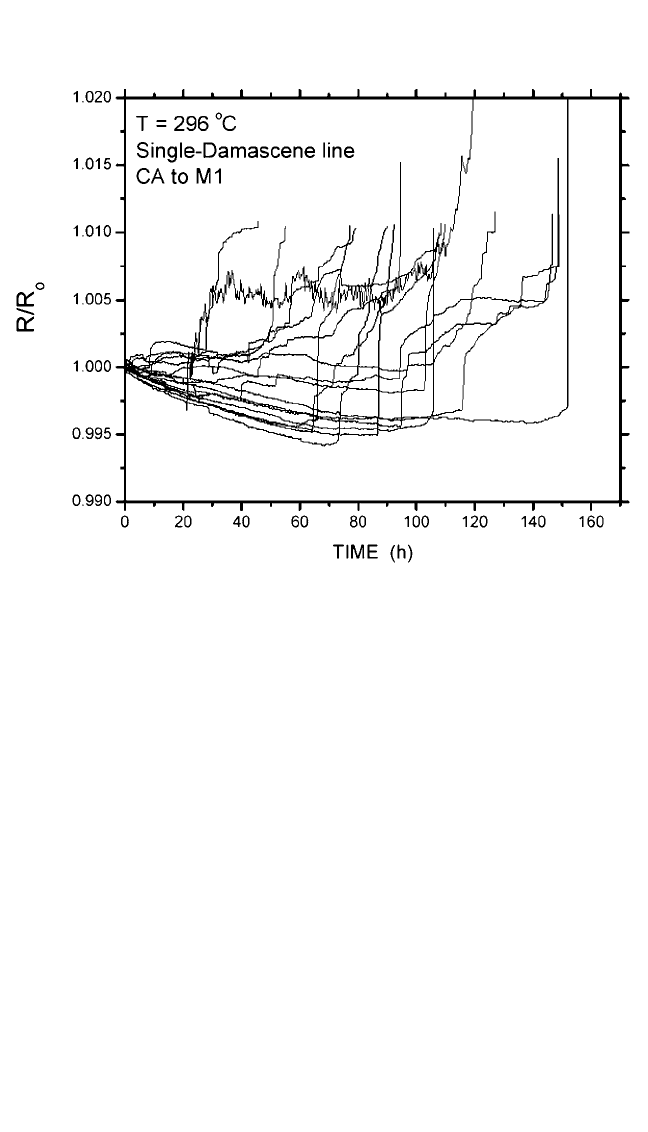
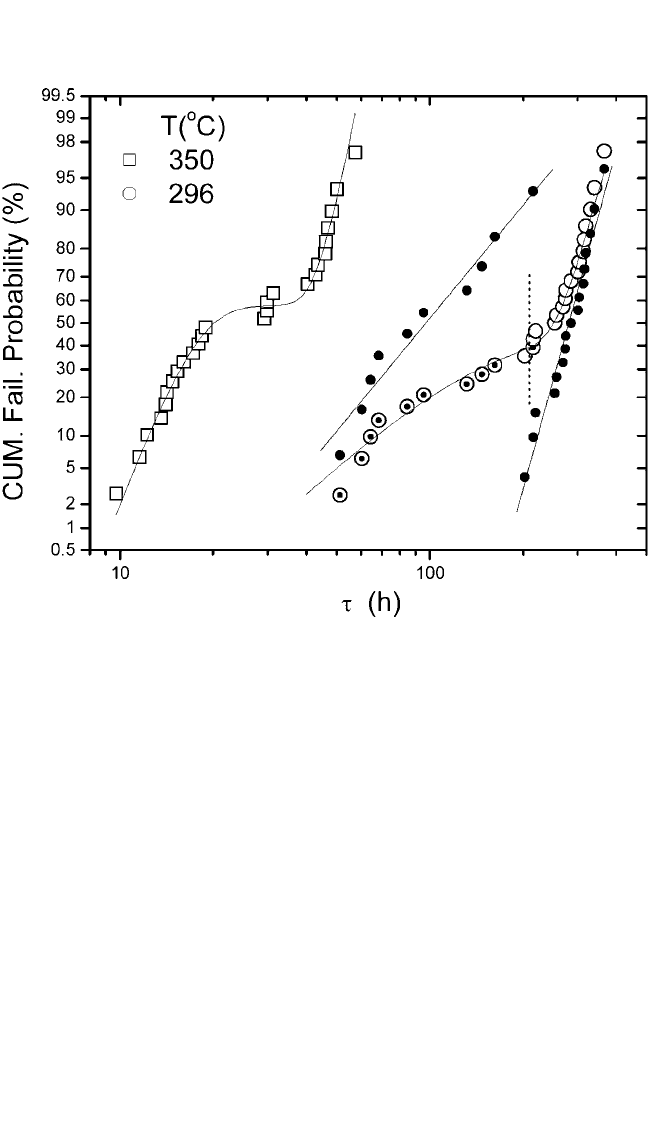
1%, 20%, or 60% will differ significantly. Figure 9.17 is a graph of cumu-

lative failure probability (%) vs. lifetime(t) on a log-normal scale from the

data shown in Fig. 9.16. For comparison, the data for the electron flow from

Cu V1 via to Cu M1 line at 296°C and from CAto M1 at a sample temper-

ature at 255°C are also plotted. In this test structure, the V1 diameter is

twice the linewidth. A linear behavior in a log-normal scale indicates that

the failure distribution can be well represented by a single log-normal func-

tion. Arather tight distribution with deviation s of around 0.3 was obtained

in all cases. The similar median lifetime between the samples tested with

electron flow from W CA to M1 and Cu V1 to M1 suggests that the liners

434 DIFFUSION PROCESSES IN ADVANCED TECHNOLOGICAL MATERIALS

Figure 9.16 Resistance change in single-damascene test lines vs. time.

Chapter-09 11/29/04 6:50 PM Page 434

ELECTROMIGRATION IN CU THIN FILMS, HUETAL. 435

at the bottom of V1 in these samples are good diffusion blocking boundaries.

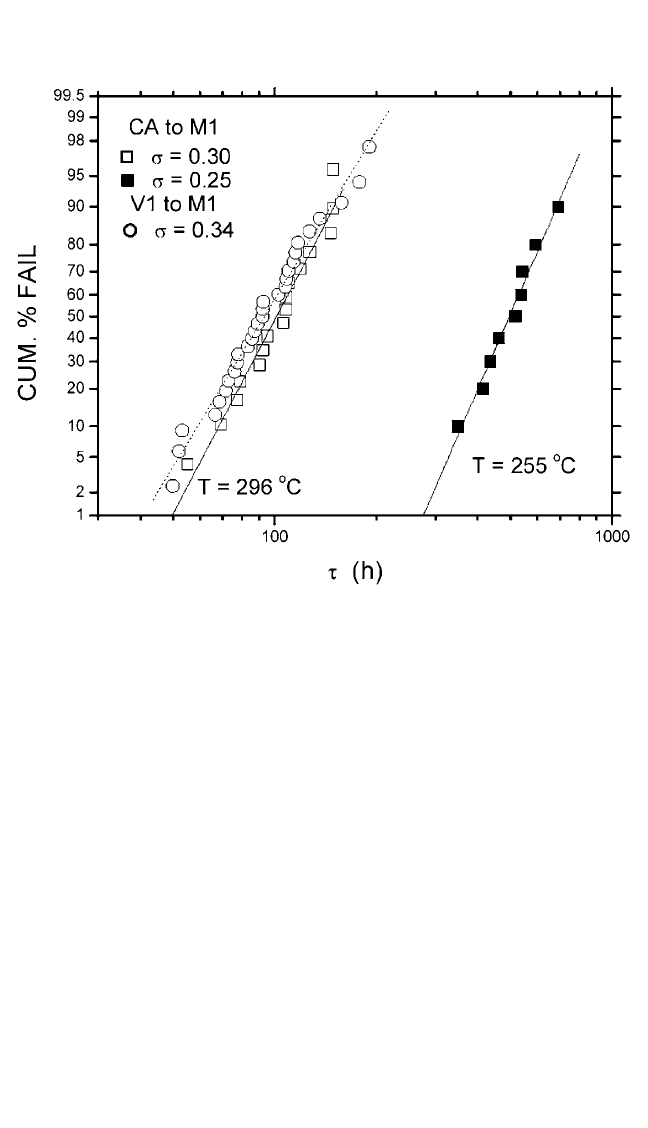
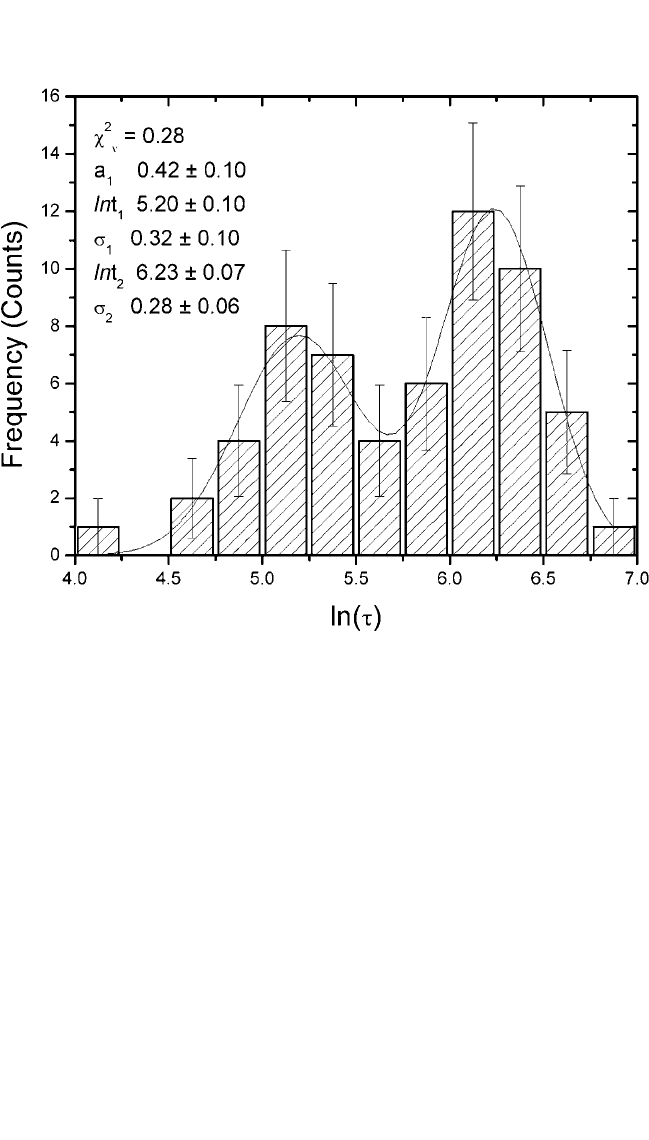
Figure 9.18 is a graph of the frequency count as a histogram in failure time

distribution showing the number of observations f

recorded for each ln(t

)

and their error bars. The plot shows a Gaussian peak, and the fluctuating

character reflects the finite collection of data points. The solid curve repre-

sents a Gaussian distribution of the data to Eq. (13) by minimizing c

. The

extracted value of c

 (c

n) of 0.9 from the least-squares fit indicates rea-

sonably good fit, where n is the degree of freedom. The extracted values of

s and median lifetime from either frequency count or cumulative failure

probability methods are in good agreement.

9.8.2 Dual-Damascene Line on W Line

9.8.2.1 Multiple-Cu Vias

Let us discuss a case of a 0.9-mm-wide Cu line embedded in SiO

where the cathode end of the line has four Cu vias connected to a W

Figure 9.17 Cumulative percentage failure vs. t for single-damascene lines on a

log-normal scale. Solid and dashed lines are the least-squares fits to the data.

Chapter-09 11/29/04 6:50 PM Page 435

underlying line.

[45]

This structure can be divided into a line section and a

reservoir section (line/via overlapped region). The current distribution in

multiple via test structures has been reported.

[114]

It was shown that the

highest current density was at the line section. The material that drifted

away along the Cu/SiN

interface in the line section was replenished by

the material from the reservoir section and by the section without electri-

cal current flow. Voids grown in the overlying Cu lines/Cu studs produce

resistance changes over time. The observation of a stepwise resistance

increase during the current stress was due to mass depletion within the

four-via region of the reservoir section. Each resistance step probably cor-

responded to the formation of a void in one of the four studs at the

Cu/underlying W interface. Once a void is formed across the entire inter-

section of the reservoir and line sections, the supply of material from the

reservoir is cut off, marking the end of the resistance incubation period. A

sharp increase in line resistance is observed after the small resistance

change period, since the remaining overlying line is connected to W by a

resistive Ta/TaN liner. Figure 9.19(a) through (c) shows optical micrographs

436 DIFFUSION PROCESSES IN ADVANCED TECHNOLOGICAL MATERIALS

Figure 9.18 Histogram of frequency, f, vs. measured log(t).The solid curve is the

estimated Gaussian function.

Chapter-09 11/29/04 6:50 PM Page 436

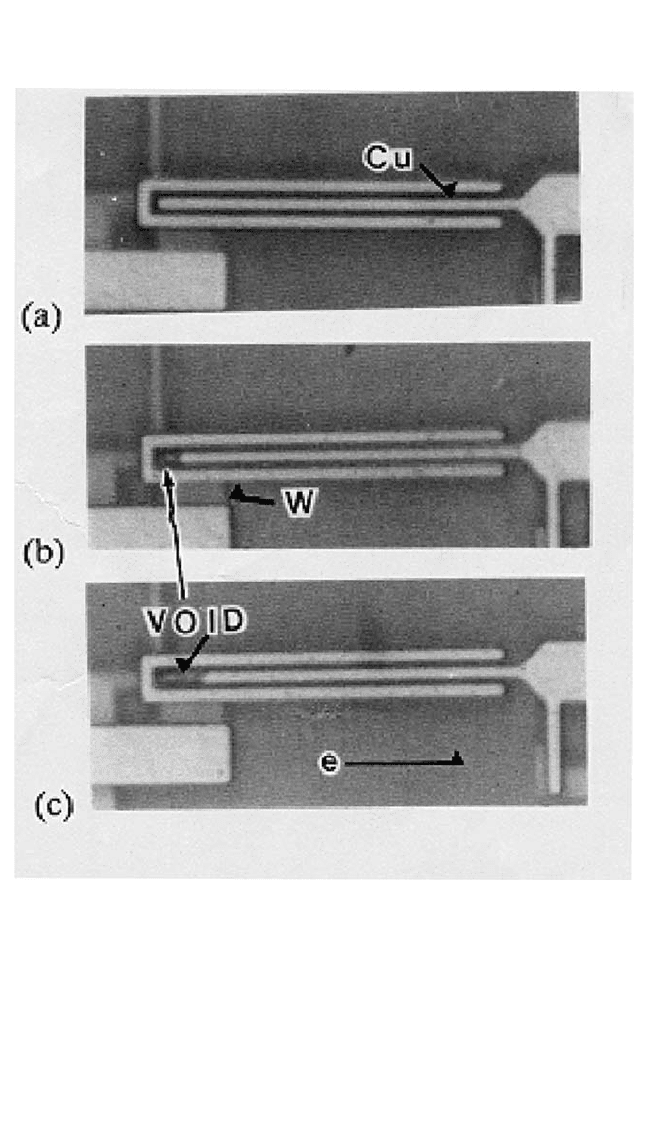
ELECTROMIGRATION IN CU THIN FILMS, HUETAL. 437

of Cu samples that were electromigration-stressed at 350°C and failed at

181, 382, and 595 hours, respectively. No formation of voids and hillocks

was found anywhere in the line section, but voids did grow in the vicinity

of the cathode studs (reservoir section). Figure 9.19(a), the earliest failed

sample, shows no visible void anywhere in the Cu overlying line. The FIB

Figure 9.19 Optical micrographs of 0.9-mm-wide Cu lines after electromigration

stress with a current density of 2  10

A/cm

at 350°C for (a) 181, (b) 382, and

Chapter-09 11/29/04 6:50 PM Page 437

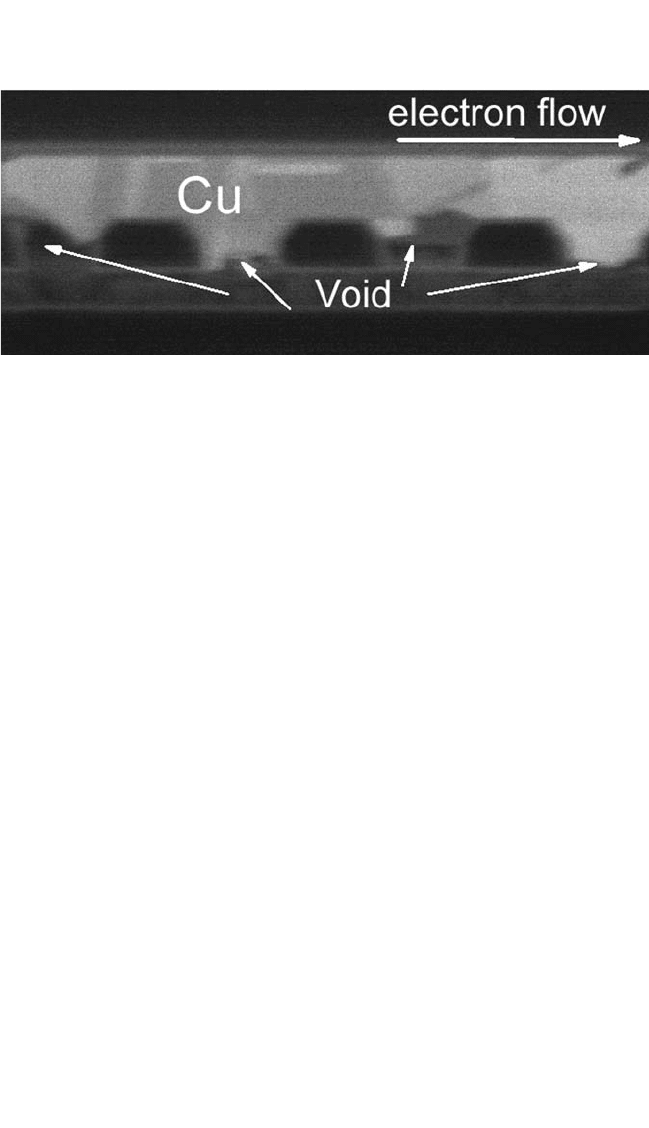
cross-section image in Fig. 9.20 shows that voids formed at the four inter-

faces of the Cu studs/W underlying line, which resulted in failure but were

not observed from top-down optical micrographs. A void size of several

microns is evident in the reservoir section of the sample that failed at an

intermediate time [Fig. 9.19(b)], apparently before the four Cu stud/W

interfaces opened. Figure 9.19(c) shows a case of complete depletion of

the entire 4.2-mm-long reservoir section, which appeared in the sample

with the longest lifetime. Log-normal fitting of the lifetime cumulative

data results in the large values for s of 0.4 to 1, in contrast to the small

(0.1 to 0.3) values of s observed for two-level single-damascene struc-

tures. The large values of s are due to void formation in different locations

from sample to sample. Amore reasonable approach for failure analysis is

to separate failure times into three different groups corresponding to three

different individual failure modes, as shown in Fig. 9.19(a) through (c).

[45]

As an example, the s for the samples that showed 4.2-mm depletion mode

is reduced to about 0.2.

9.8.2.2 Single-Cu Via

Now let us consider a simpler case of a dual-damascene line with

one Cu via on Winstead of four vias as in the case discussed in Section

9.8.2.1. We attempt to correlate the lifetime failure distribution with

the void growth and location and to present methods of data analyses

in detail for the failure lifetime distributions. Even in this simple struc-

ture, the lifetime distribution has been reported and was also found to

have multiple failure modes.

[37]

Awide spread in the lifetime distribution

438 DIFFUSION PROCESSES IN ADVANCED TECHNOLOGICAL MATERIALS

Figure 9.20 FIB image of the electromigration-tested line from Fig. 9.19(a). The

image was taken at an ion beam angle of 45 degrees.

Chapter-09 11/29/04 6:50 PM Page 438

ELECTROMIGRATION IN CU THIN FILMS, HUETAL. 439

can be seen in Figs. 9.21 and 9.22, which are the graphs of ln(t) vs.

frequency count and cumulative failure probability (%) vs. lifeime(t)

on a log-normal scale, respectively. The data in Fig. 9.21 clearly show

a two-Gaussian function. The solid curves respresent a double-Gaussian

function fit. The extracted value of c

 (c

v) of 0.3 from the least-

squares analysis implies a reasonably good fit. The nonlinear behavior

in Fig. 9.22 shows that the failure distribution is not a single log-

normal function; however, it can be plotted as two individual log-

normal functions. For samples tested at 296°C, the data points can also

be separated into two individual groups as shown in Fig. 9.22. The ver-

tical dotted line is the border between these two distributions; this

point was determined by the transition of void growth from the bottom

via to the line/via or to the line itself, which will be discussed later in

this section. The cumulative failure distribution is analyzed either by

separating the data points into two individual groups

[115]

or by using a

bimodal distribution function.

[34, 37–39]

The bimodal distribution

Figure 9.21 Histogram of frequency, f, vs. measured log(t).The solid curve is the

estimated double-Gaussian function.

Chapter-09 11/29/04 6:50 PM Page 439

function is given by:

F 

e



(ln

(

t

))



d ln t  e



(ln

(

t

))



d ln t, (16)

where t

, s

and t

are the median lifetimes and the deviations for the

first and second log-normal distribution functions, respectively, and a

the fraction of the total population in the first log-normal function. Anon-

least-squares fitting method was used to obtain the best adjustable fitting

parameter values of t

, s

, t

and a

. The voids in most of the

electromigration-damaged lines were cross-sectioned and imaged using a

FIB. For example, Fig. 9.23(a) to (d) shows cross-sectional-view FIB

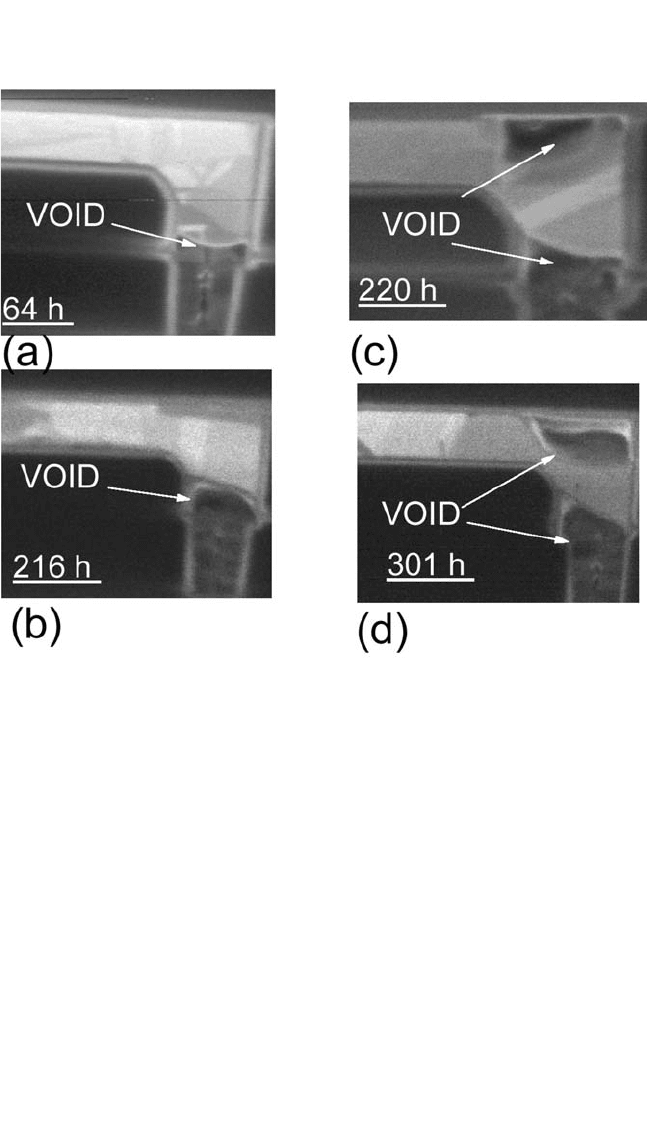
images of voids in the Cu dual-damascene lines tested at 296°C for life-

times of 64, 216, 220, and 301 hours, respectively, taken at an ion beam

(1  a

)



2ps





2ps



440 DIFFUSION PROCESSES IN ADVANCED TECHNOLOGICAL MATERIALS

Figure 9.22 Cumulative percentage failure vs. t on a log-normal scale. Solid lines

are the least-squares fits to the data. The dotted line is the border between two

Gaussians.The solid circles are a replot of the data points (open circles) into two

individual groups.Open circles with center dots correspond to the first-failure group.

Chapter-09 11/29/04 6:50 PM Page 440

ELECTROMIGRATION IN CU THIN FILMS, HUETAL. 441

angle of 45°. The samples with a short lifetime of 64 hours, Fig. 9.23(a),

and a medium lifetime of 216 hours, Fig. 9.23(b), showed that no voids

grew in the line, although voids did grow at the bottom of the Cu via.

However, the samples in the long-lifetime t

group had mixed mode

line/via voids [see Fig. 9.23(c) and (d)] or line-only voids. From the fail-

ure analysis sampling, voids were only seen at the bottom of the via for

samples in the short-lifetime t

group. From our analyses, the tested sam-

ples with lifetimes around 200 hours have a mixed mode, and with lifetimes

greater than 216 hours no longer will have void growth at the via bottom

only. Once the separation of the two groups was determined by FIB analy-

sis, the data points could be simply plotted into two individual log-normal

distribution functions (Two-Probit), as shown in Fig. 9.22. In this way, the

strong correlation between five fitting-adjusted parameters in a bimodal

function can be reduced. This method allows a more precise fit of the data

to a log-normal function. The extracted values from the three methods

Figure 9.23 FIB images of samples tested at 296°C, taken at an ion beam angle

of 45 degrees, for lifetimes of (a) 64, (b) 216, (c) 220, and (d) 301 hours, respectively.

Chapter-09 11/29/04 6:50 PM Page 441