Greiner W., Schrammm S., Stein E. Quantum Chromodynamics
Подождите немного. Документ загружается.


378 5. Perturbative QCD I: Deep Inelastic Scattering
Example 5.17
Expanding the series
α
s
(µ
2
)
1 +β
0
α
s
(µ
2
) ln
−k
2
/µ
2
e
−C
=
∞
n=0
(
α
s
)
n+1
(
−β
0
)
n
ln
n
−
k
2
µ
2
e
−C
(28)
and performing the Borel transformation α
n+1
s
→u
n
/n! we find
∞
n=0
(
α
s
)
n+1
(
−β
0
)
n
ln
n
−
k
2
µ
2
e
−C
⇒
∞
n=0
u
n
n!
(−β
0
)
n
ln
n
−
k
2
µ
2
e
−C
= exp
−β
0
u ln
−
k
2
µ
2
e
−C
=
µ
2
e
−C
−k
2
β
0
u
(29)
and get for the transformed propagator
BT
α
s
(k
2
)D
AB
µν
(k)
(u) =
iδ
AB
−k
2
g
µν
+
k
µ
k
ν
−k
2
µ
2
e
−C
−k
2
β
0
u
. (30)
The result means the following: To obtain an all-order expression for a diagram
that only contains one bubble chain one only has to calculate the normal α
s
cor-
rection but with the simple gluon propagator replaced by the Borel transformed
propagator in (30). From the technical point of view one has to change the nor-
mal power 1/(−k
2
) to 1/(−k
2
)
1+β
0
u
. This simple fact was first observed in.
35
To obtain fixed-order contributions at the end one can apply (9). As a result for
such a calculation we will give the perturbative expansion of the Bjorken sum
rule to all orders in the Borel representation. Let us write
+
1
0
dxg
p−n
1
(x, Q
2
)
(1/6)g
A
/g
v
=1 +
n
a
n
α
n+1
s
+
n
δ
n
α
n+1
s
. (31)
Here the coefficients a
n
stem from the insertion of n bubbles and the δ
n
,which
shall be neglectded in the following, can only be obtained from an exact all-order
calculation. The generating function for the a
n
is obtained by the calculation of
all the diagrams in Fig. 5.21 and using the propagator in (30). The calculation is
straightforward by following the general procedure explained in the section on
Wilson coefficients. An important simplification arises, however, since we are
only interested in the first momentum of the structure function g
1
. This is the
contribution ∼ ω
0
=
2p ·q/Q
2
0
and therefore does not depend on the target
momentum p. Therefore, in the following we can set p = 0 without any loss of
generality. This will simplify the calculations tremendously. Some intermediate
steps of the calculation will be given in the following: For the self-energy one
35
M. Beneke: Nucl. Phys. B 405, 424 (1993).

5.6 The Spin-Dependent Structure Functions 379
gets
S(q, d, s) = (−ig)
2
(µ
2
)
2−d/2
×
d
d
k
(2π)
d
γ
µ
i
q/ −k/
γ
ν
−i
k
2
g
µν
+
k
µ
k
ν
(−k
2
)
µ
2
e
−C
(−k
2
)
s
, (32)
where the propagator (30) was used with the substitution s = β
0
u. In principle
such calculations have to be performed in d dimensions to regulate divergen-
cies. On the other hand, using the special propagator (30) the additional power
of s serves as a regulating device. Divergencies that commonly appear at d = 4
will not appear at s = 0. The final result, i.e. the sum of all diagrams, which
is finite, will not depend on the used procedure of regulation. At intermediate
steps, however, results for single diagrams will differ for different regularization
procedures.
Performing the integrals one is led to the following expression:
S(q, d, s = 0) =0 ,
S(q, d = 4, s) =
3i
(4π)
2
g
2
µ
2
e
−C
−q
2
s
q/
Γ(1 +s)Γ(1 −s)
Γ(3 −s)Γ(2 +s)
. (33)
The first equation reflects the fact that the self-energy vanishes in the Lan-
dau gauge. The second equation shows that the limits s → 0andd → 4donot
commute. For the vertex correction one gets
Γ
µ
(q, d, s) =−ig
2
(µ
2
)
2−d/2
(µ
2
e
−C
)
s
×
d
d
k
(2π)
d
γ
α
(q/ −k/)γ
µ
k/γ
β
(−(q −k)
2
)(−k
2
)
3+s
g
αβ
(−k
2
) +k
α
k
β
, (34)
which can be evaluated to give
Γ
µ
(q, d → 4, s = 0) =−
g
2
8π
2
q
µ
q/
(−q
2
)
+γ
µ
Γ
µ
(q, d = 4, s) =−
g
2
(4π)
2
µ
2
e
−C
Q
2
s
×
Γ(1 +s)Γ(1 −s)
Γ(3 −s)Γ(2 +s)
γ
µ
+O(q
µ
q/) . (35)
Terms of the order q
µ
q/ do not contribute to the antisymmetric part of the Comp-
ton amplitude and therefore can be neglected. For the diagram with self-energy
insertion one finds
t
(1)
µν
S(q,4,s)
p=0
g
2
=−
3
(4π)
2
g
2
µ
2
e
−C
Q
2
s
Γ(1 +s)Γ(1 −s)
Γ(3 −s)Γ(2 +s)
×
γ
µ
i
q/
γ
ν
+(µ → ν, q →−q)
=−
3
4π
α
s
µ
2
e
−C
Q
2
s
Γ(1 +s)Γ(1 −s)
Γ(3 −s)Γ(2 +s)
t
(0)
µν
p=0
.
(36)
Example 5.17

380 5. Perturbative QCD I: Deep Inelastic Scattering
Example 5.17
Here we have introduced the notion
t
(0)
µν
p=0
=γ
µ
i
q/
γ
ν
+(µ → ν, q →−q) (37)
for the tree-level, i.e. the O(g
2
= 0) contribution. The result for diagrams with
vertex corrections gives, according to (35),
t
(1)
µν
Γ(q,4,s)
p=0
g
2
=−
2
4π
α
s
µ
2
e
−C
Q
2
s
Γ(1 +s)Γ(1 −s)
Γ(3 −s)Γ(2 +s)
t
(0)
µν
p=0
. (38)
Calculation of the remaining box diagram
t
(1)
µν
B(q,d,s)
p=0
g
2
= (−ig)
2
(µ
2
)
2−d/2
µ
2
e
−C
s
×
d
d
k
(2π)
d
γ
i
k/
γ
ν
i
k/ +q/
γ
µ
i
k/
γ
σ
i(g
σ
(−k
2
) +k
k
σ
)
(−k
2
)
2+s
, (39)
yields for d = 4, s = 0 the following result:
t
(1)
µν
B(q,4,s)
p=0
g
2
=−
1
4π
α
s
µ
2
e
−C
Q
2
Γ(1 +s)Γ(1 −s)
Γ(3 +s)Γ(2 −s)
t
(0)
µν
p=0
.
(40)
The sum of all contributions (36), (38), and (40) therefore gives
t
(1)
µν
p=0
g
2
=−α
s
C
F
π
µ
2
e
−C
Q
2
s
Γ(1 +s)Γ(1 −s)
Γ(3 −s)Γ(3 +s)
(3 +s)
t
(0)
µν
p=0
=−α
s
C
F
π
µ
2
e
−C
Q
2
s
3 +s
(1 −s)(1 +s)(2 −s)(2+s)
t
(0)
µν
p=0
s=0
=−
α
s
π
t
(0)
µν
p=0
, (41)
where we have inserted the color factor C
F
=4/3. Also we used the prop-
erty of the Γ -function xΓ(x) = Γ(x +1).Fors = 0 we reproduce the one-loop
correction to the Bjorken sum rule.
C
A
(1,α
s
(Q
2
/µ
2
)) = 1 −
α
s
(Q
2
)
π
+··· (42)
For s = 0 one gets from (41) the generating functional for the a
n
:
B[R](s) =−
C
F
π
µ
2
e
−C
Q
2
s
3 +s
(1 −s
2
)(4 −s
2
)
=−
C
F
3π
µ
2
e
−C
Q
2
s
2
1 −s
+
1
1 +s
+
5
4(2−s)
−
1
4(2+s)
.
(43)

5.6 The Spin-Dependent Structure Functions 381
With s = β
0
u the relevant equations now read
R −R
tree
=
1
β
0
∞
0
ds e
−s/β
0
α
s
B
[
R
]
(s) (44)
B
[
R
]
(s) =
∞
m
s
β
0
m
a
m
m!
(45)
a
m
=β
m
0
d
m
ds
m
B
[
R
]
(s)
|
s=0
(46)
(compare with (7), (8), and (9)).
Applying (46) and setting µ
2
= Q
2
one generates a series in β
0
α
s
:
+
1
0
dxg
p−n
1
(x, Q
2
)|
NNA
(1/6)g
A
/g
V
=1 −
α
s
π
1 +2β
0
α
s
+
115
18
β
2
0
α
2
s
+
605
27
β
3
0
α
3
s
+...
, (47)
which reproduces the expansion in (26) with the corresponding insertion of β
0
.
In Fig. 5.37 we have plotted the NNA approximation to the perturbative ex-
pansion of the Bjorken sum rule. One recognizes the typical behavior of an
asymptotic series: up to a maximal order of expansion the terms converge to
a minimal value characterized by n
0
, but from there on n > n
0
the series starts to
diverge. Analyzing (43) we see that the Borel representation exhibits four renor-
malons. With u = s/β
0
we find infrared renormalons at 1/β
0
and 2/β
0
, while the
ultraviolet renormalons are situated at −1/β
0
,and−2/β
0
.
The first IR renormalon leads to a fixed sign factorial divergence
d
n
ds
n
1
1 −s
=n! . (48)
012345678910
n
Q =2 GeV
Q = 10 GeV
22
22
0.0
-0.05
-0.1
-0.15
-0.2
-0.25
-0.3
-0.35
-0.4
-0.45
-.05
a(Q)
nS
a
n+1 2
Fig. 5.37. NNA corrections
to the Bjorken sum rule.
We have chosen Λ
MS
=
200 MeV and Q
2
= 2GeV
(full data) and Q
2
=10 GeV
(triangles). On the n axis
we have drawn the order of
expansion
Example 5.17

382 5. Perturbative QCD I: Deep Inelastic Scattering
Example 5.17
The pole next to the origin dominates the asymptotic expansion. Applying
the Leibnitz rule of differentation we are able to give closed formulas for the
asymptotic behavior resulting from (43).
With a being some number (which can be read off from the first, second, ...
fourth term in (43)) we find
d
n
ds
n
e
−C
µ
2
Q
2
s
1
1 +as
=
n
k=0
n
k
ln
k
e
−C
µ
2
Q
2
e
−C
µ
2
Q
2
s
(n −k)!
(−a)
n−k
(1 −s)
n−k+1
s=0
= n!(−a)
n
n
k=0
1
k!
1
−a
k
ln
k
e
−C
µ
2
Q
2
= n!(−a)
n
n
k=0
1
k!
⎡
⎣
ln
e
−C
µ
2
Q
2
1
−a
⎤
⎦
k
= n!(−a)
n
e
−C
µ
2
Q
2
−1/a
, (49)
where n →∞was used in the last line. Let us now combine (49) with (46) and
determine the number a in (49) from the four terms in (43). Then, asymptotically
the series derived from (43) receives four distinct contributions which behave as
a
IR1
n
∼−
2C
F
3π
e
−C
µ
2
Q
2
n!β
n
0
,
a
IR2
n
∼−
5C
F
24π
e
−C
µ
2
Q
2
1
2
n
n!β
n
0
,
a
UV1
n
∼−
C
F
3π
e
−C
µ
2
Q
2
−1
n!(−)
n
β
n
0
,
a
UV2
n
∼
C
F
24π
e
−C
µ
2
Q
2
−2
n!
−
1
2
n
β
n
0
, (50)
where the superscripts refer to the first (IR1) and second (IR2) infrared renor-
malons and the ultraviolet (UV1, UV2) renormalons.
The obvious question now is: Which term gives the dominant contribution for
n →∞? Immediately one finds that the second renormalons (UV2 and IR2) are
suppressed by a factor (1/2)
n
. On the other hand, the contribution from the UV
renormalon (UV1) is smaller than the first IR renormalon by a factor 2. Thus, the
IR renormalon yields the most important contribution at n →∞. Asymptotically
the coefficients therefore behave as
a
n+1
a
n
∼β
0
(n +1). (51)

5.6 The Spin-Dependent Structure Functions 383
The maximal order n
0
which corresponds to the minimal term in the series (see
Fig. 5.38) follows from the condition
a
n+1
α
s
a
n
≥1, (52)
so that
n
0
=
1
β
0
α
s
−1 =ln
Q
2
/Λ
2
C
−1 , (53)
where we have used the one-loop definition of Λ
QCD
,
α
s
=
1
β
0
ln
Q
2
/Λ
2
QC D
, (54)
and introduced the index C (Λ
2
QCD
=Λ
2
C
) to remind the reader that Λ
QCD
depends on the chosen renormalization scheme.
Setting Q
2
= µ
2
and inserting (57) into (2) one finds the inherent uncertainty
∆R of the asymptotic expansion:
∆R = α
n+1
s
a
n
0
=−
2
3
C
F
π
β
ln(Q
2
/Λ
2
C
)−1
0
Γ
ln(Q
2
/Λ
2
C
)
e
−C
α
ln(Q
2
/Λ
2
C
)
s
=−
2
3
C
F
π
1
β
0
Γ
ln(Q
2
/Λ
2
C
)
e
−C
ln(Q
2
/Λ
2
C
)
−ln(Q
2
/Λ
2
C
)
. (55)
Using Sterling’s formula for the Γ function,
Γ(z) = e
−z
z
−1/2
(2π)
1/2
1 +
1
12z
+O
1/z
2
, (56)
Fig. 5.38. Contributions of
UV and IR renormalons to
the Bjorken sum rule, cf.
(50). The n axis shows the
order of expansion. The per-
turbative expansion is com-
pletely dominated by the
first IR renormalon (marked
by the full bullets)
Example 5.17

384 5. Perturbative QCD I: Deep Inelastic Scattering
Example 5.17
and therefore
Γ(z)z
−z
= e
−z
z
−1/2
(2π)
1/2
+O
1/z
2
, (57)
we obtain for ∆R
∆R =−
2
3
C
F
π
1
β
0
(2π)
1/2
Λ
2
C
e
−C
Q
2
1
ln Q
2
/Λ
2
C
+O
1
Q
2
ln Q
2
/Λ
2
C
.
(58)
As promised at the beginning, the uncertainty in the asymptotic expansion
has no logarithmic dependences on the external momentum Q
2
but a power
dependence. For large Q
2
it vanishes like 1/Q
2
.
Another more elegant way to find the uncertainty in the expansion is to take
the imaginary part of the Borel transform (divided by π). From (44) we get
Im
π
∞
0
dse
−s/β
0
α
s
B
[
R
]
(s)
=±
1
β
0
∞
0
dse
−s/β
0
α
s
(−)
2C
F
3π
µ
2
e
−C
Q
2
s
δ(1 −s)
=±
2C
F
3π
1
β
0
Λ
2
C
e
−C
Q
2
, (59)
which reproduces (58) up to logarithmic corrections.
The ambiguity in the sign of the IR renormalon contribution is due to the
two possible contour deformations above or below the pole at s = 1. The IR
renormalon pole at s = 2leadsto1/Q
4
corrections:
5C
F
12π
1
β
0
Λ
2
C
e
−C
Q
2
2
. (60)
Let us summarize our findings. For a physical quantity like the Bjorken sum rule
the perturbative QCD series is not summable, even in the Borel sense. This is
due to the appearance of fixed sign factorial growth of its coefficients. It results
in a power-suppressed ambiguity of the magnitude Λ
2
QCD
/Q
2
. The ambiguity
therefore has exactly the same power behavior as that known from higher-twist
corrections.
Since the Bjorken sum rule represents a physical quantity it must have
a unique value. The ambiguity apparent in our calculation shows the need
to include additional nonperturbative corrections which cancel the ambigu-
ity and give a meaning to the summed perturbative series. These additional
nonperturbative corrections are higher-twist corrections.
It can be shown that the higher-twist corrections themselves are ill
defined.
36,37
The ambiguity in the definition of the higher-twist corrections can-
36
E. Stein, M. Meyer-Hermann, et al.: Phys. Lett. B 376, 177 (1996); E. Stein, M. Maul
et al.: Nucl. Phys. B 536, 318 (1999).

5.6 The Spin-Dependent Structure Functions 385
cels exactly the IR renormalon ambiguity in the perturbative series of the twist-2
term. In turn, the investigation of the ambiguities in the definition of the perturba-
tive series of the leading twist shows which higher-twist corrections are needed
for the unambigious definition of a physical quantity.
Finally we would like to note that the calculation we have presented here for
the Bjorken sum rule can be easily extended to all other structure functions. Such
calculations can be easily done for all moments and hence give the IR renor-
malon ambiguity as a function of x. Since a rigorous operator product expansion
for all moments of a structure function up to twist 4, or the calculation of the
corresponding nucleon matrix elements, is beyond present-day capabilities, the
easily calculable IR renormalon ambiguity has proven to be a phenomenological
successful model for power corrections. We will not dwell on this issue here but
refer the reader to the literature.
36,37
37
For a general overview on renormalons see: M. Beneke: Phys. Rept. 317, 1 (1999).
Example 5.17
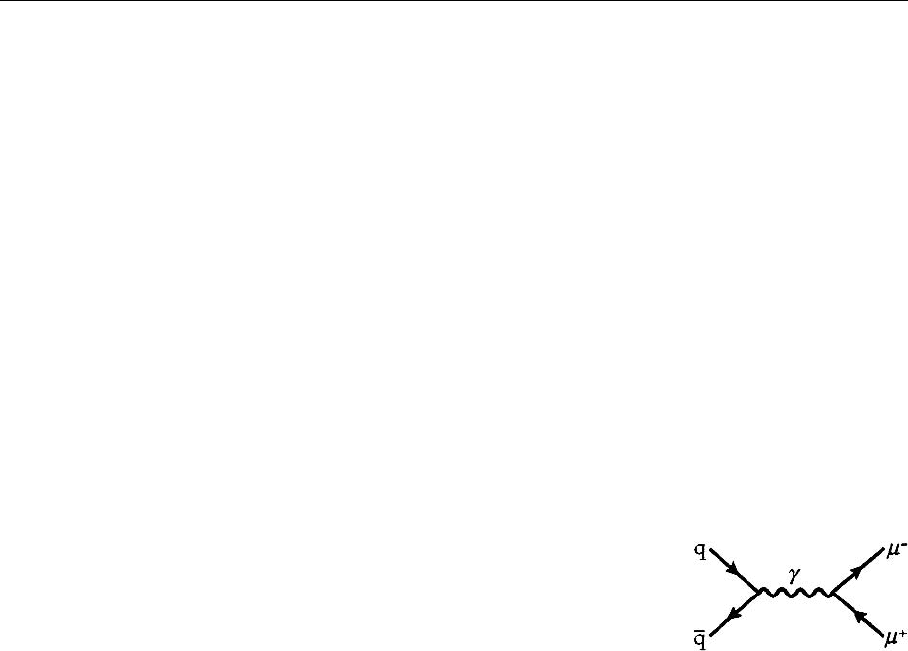
6. Perturbative QCD II: The Drell–Yan Process
and Small-x Physics
6.1 The Drell–Yan Process
Deep inelastic scattering is investigated by shooting electrons or muons at
different fixed targets or by colliding them with a proton beam. From these ex-
periments only information about nucleon structure functions can be extracted,
while little is learned about the inner structure of pions, for example. There are,
however, different options for high-energy collisions. Electrons can be made to
collide with positrons (as is done at Stanford, DESY, and CERN), and protons
can be made to collide with protons, pions, kaons, or antiprotons. The latter is
done at sites such as Fermilab, where secondary beams of pions, antiprotons, etc.
are created and collide with a fixed target.
To determine the internal structure of hadrons in these reactions, it is again
preferable to consider reactions as hard as possible. For such reactions there is
hope that perturbation theory will yield good results. To obtain information about
the inner structure of hadrons, these must be in the initial channel. We therefore
consider the collision of two hadrons. The process most similar to deep inelas-
tic scattering in these reactions is the annihilation of a quark and an antiquark,
each deriving from a different hadron, into a lepton pair (see Fig. 6.1). This is
called the Drell–Yan process. Bear in mind that the creation of hadrons in e
+
e
−
scattering is described by the very same, time-reversed graph. While quark an-
nihilation into leptons tells us about the initial momentum distribution of the
quarks inside the hadrons involved, e
+
e
−
annihilation is used to investigate how
full-blown hadrons are created from just two dissociating quarks, that is, how
the momentum originally carried by the quark–antiquark pair that has been cre-
ated is distributed between real and virtual constituents of all created hadrons.
This is mainly a dynamical problem in which nonperturbative effects are cru-
cial. Its description in the framework of QCD is of the utmost difficulty and has
consequently not yet proceeded beyond phenomenological models. Keywords
characterizing this area of research are “hadronic strings” and “jet physics”.
We shall first address the Drell–Yan process of Fig. 6.1. By carrying over our
experience of deep inelastic scattering to the Drell–Yan process, we expect the
cross section to behave like
dσ(DY) =
q
a
(x
a
)
¯
q
b
(x
b
) +q
b
(x
b
)
¯
q
a
(x
a
)
×
ˆ
σ(q +
¯
q → γ
∗
→µ
+
µ
−
) dx
a
dx
b
. (6.1)
Fig. 6.1.
The Drell–Yan process

388 6. Perturbative QCD II: The Drell–Yan Process and Small-x Physics
Here a designates the first and b the second hadron. Consequently q
a
(x
a
) gives
the probability of finding a quark of flavor q carrying the momentum frac-
tion x
a
, in the first hadron. This is based on the expectation that at sufficiently
high energies all color interactions will be suppressed by the large denomina-
tor of a propagator. However, this argument has to be treated with great caution,
since there are also soft processes that cannot be neglected (see below). Another
problematic point is the kinematic difference between Drell–Yan and deep in-
elastic scattering. While in deep inelastic scattering the photon momentum is
q
2
=−Q
2
< 0 with a large Q
2
,nowq
2
= M
2
> 0 holds with M the invariant
mass of the lepton pair. An extended formal analysis has shown that such formal
continuation is possible and (6.1) can be justified. Basically Q
2
is substituted by
−M
2
in all formulas. This, however, quite substantially changes all logarithmic
terms: ln(Q
2
/m) → ln(−M
2
/m
2
) = iπ +ln(M
2
/m
2
). The running coupling
constant still has its usual form with α(Q
2
) → α(M
2
), but in practical calcu-
lations the perturbative expansion does not converge well owing to the term
proportional to iπ. It must be conceded, therefore, that the analysis of Drell–Yan
reactions is afflicted with far more uncertainties than deep inelastic scattering.
All this will be discussed in more detail below.
First we shall evaluate (6.1) further while skipping the more involved
questions. In doing this, it is customary to define some new quantities. Let
s = (P
a
+ P
b
)
2
be the invariant mass of the colliding hadrons. The momenta of
the partons participating in the Drell–Yan process are p
a
= x
a
P
a
, p
b
= x
b
P
b
.
Neglecting hadron and quark masses, the invariant mass of the lepton pair is then
M
2
= ( p
a
+ p
b
)
2
= 2 p
a
· p
b
= 2x
a
x
b
P
a
· P
b
= x
a
x
b
(P
a
+ P
b
)
2
= x
a
x
b
s . (6.2)
It is furthermore standard to change the variables x
a
and x
a
to
τ = x
a
x
b
=
M
2
s
, y =
1
2
ln
x
a
x
b
. (6.3)
Keep in mind that for the energy and longitudinal momentum of the lepton pair
E = x
a
E
a
+x
b
E
b
, P
L
= x
a
E
a
−x
b
E
b
are valid. Since the incoming partons are parallel to the indicent protons and
therefore do not have transverse momenta, we obtain in the center-of-momentum
system of the two hadrons, E
a
= E
b
=
√
s/2,
y =
1
2
ln
E +P
L
E −P
L
.
Thus y is the rapidity of the lepton pair in the center-of-momentum system. To
rewrite (6.1) in the new variables, we need the functional determinant
∂(τ, y)
∂(x
a
, x
b
)
= 1 . (6.4)
