Galaktionov V.A., Svirshchevskii S.R. Exact Solutions and Invariant Subspaces of Nonlinear Partial Differential Equations in Mechanics and Physics
Подождите немного. Документ загружается.

8 Sign-invariants and Exact Solutions 393
Substituting in (8.37) yields
v(x, t)= C(t)ρ(x)∈ W ⇒ C
= aC, (8.39)
which gives the solutions (8.33). For ϕ(u)= u
σ+1
in (8.29), there occurs the PME
or the fast diffusion equation with reaction-absorption,
u
t
=u
σ+1
+
a
σ+1
u+
b
σ+1
u
−σ
+ acu
σ+1
+ bc (σ= 0,−1).
Forϕ(u)= tan u,wefind the parabolic equation
u
t
=(tan u)
xx
+(a tan u+ b)(c+ cos
2
u). (8.40)
Example 8.4 The quasilinear heat equation (8.40) with a= c= 1andb= 0 admits
the SI
H[u]= u
t
−
1
2
sin 2u.
It is also a zero-invariant, from which we obtain the explicit solution
u(x, t)= tan
−1
(e
t
cos x).
The existence of such a solution becomes trivial if we set v= tan
−1
u, which yields
solutionv(x, t)= e
t
cos x of the PDE (cf. (8.37))
v
t
= (1 + v
2
)(v
xx
+ v) + v.
The analogy with the invariant subspace (8.38) reveals many extensions of such
exact solutions to hyperbolicand higher-order PDEs, though, of course, any connec-
tion with SIs is then lost.
Example 8.5 (Hyperbolic equations) The hyperbolic PDE
v
tt
= F[v] ≡ (v)(v + acv) + av
admits the same solutions (8.39) with the ODE C
= aC.
Example 8.6 (Higher-order PDEs) The fourth-order parabolic equation
v
t
= F[v] ≡ (v)(−
2
v − acv) + av,
with an arbitrary function (v), has solutions (8.39).One can extend the list of PDEs
with operators preserving such linear subspaces.
Example 8.7 In a similar fashion, the fourth-order quasilinear hyperbolic PDE
v
tttt
= (1 + v
2
)(v
xxxx
− v) + v
admits explicit solutions
v(x , t) = e
t+x
+ e
−(t+x )
+ cos(x − t).
For convenience, we combine some generalizations in the following statement.
© 2007 by Taylor & Francis Group, LLC
394 Exact Solutions and Invariant Subspaces
Proposition 8.8
Let
Q(D
x
)(D
x
=∇
x
in
IR
N
)
be a linear differential operator with
constant coefficients, and
ϕ(u)
be a smooth increasing function. The equation
u
t
= Q(D
x
)[ϕ(u)] +
aϕ(u)+b
ϕ
(u)
+ d (8.41)
with constants
a = 0
,
b
,and
d
such that
ad = Q(D
x
)[b], (8.42)
admits explicit solution
(8.33)
,where
ρ(x)
solves
Q(D
x
)[ρ] = 0
in
IR
N
.
Proof. If a = 0, setting aϕ(u) + b = v yields
v
t
= ϕ
(u)
Q(D
x
)[v] +ad − Q(D
x
)[b]
+ av.
In view of (8.42), the solution is v(x, t) = ρ(x)e
at
.
If a = 0 in (8.41), setting v = ϕ(u) gives
v
t
= ϕ
(u){Q(D
x
)[v] + d}+b.
Hence, under the hypothesis Q(D
x
)1 = 0 (see (8.42) with a = 0), it admits the
solution v(x, t) = ρ(x) + bt,whereρ solves ρ +d = 0inIR
N
.
Example 8.9 Taking in (8.41) the operator
Q(D
x
)[w] = w + α(∇w ·n) + βw
with ad = βb, Proposition 8.8 yields exact solutions of the following quasilinear
heat equation with a nonlinear convective term:
u
t
= ϕ(u) + α(∇ϕ(u) · n) +
aϕ(u)+b
ϕ
(u)
+ βϕ(u) + d.
Extensions to 2mth-order quasilinear parabolic PDEs are straightforward, but then
operator (8.30) is not an SI for any m ≥ 2, and remains a zero-invariant.
Example 8.10 As a next extension of the same idea, we introduce the following
equation with two linear operators Q
i
(D
x
), and arbitrary functions
i
(u):
u
t
=
1
(u)Q
1
(D
x
)[ϕ(u)] +
2
(u)Q
2
(D
x
)[ϕ(u)] +
aϕ(u)+b
ϕ
(u)
,
where Q
1
(D
x
)[1] = Q
2
(D
x
)[1] = 0. There exist exact solutions (8.33), with a = 0,
and (8.27), with a = 0, if ρ(x) solves a system in IR
N
,
Q
1
(D
x
)[ρ] = 0,
Q
2
(D
x
)[ρ] = 0.
8.3 Stationary sign-invariants of the form H (r, u, u
r
)
Consider the SIs which do not contain the time-derivative u
t
. Important examples of
such SIs come from blow-uptheory;see Remarks.Consider the general 1D parabolic
© 2007 by Taylor & Francis Group, LLC

8 Sign-Invariants and Exact Solutions 395
equation in radial geometry
P[u] ≡ u
t
− L(r, u, u
r
, u
rr
) = 0, (8.43)
where r > 0 denotes |x|,andL(r, u, p, q) satisfies the parabolicity condition (8.11).
We take the SI in the general form
H[u] = H (r, u, u
r
), (8.44)
where smooth H (r, u, p) satisfies H
p
> 0. By H
∗
(r, u, s) we denote the inverse
function such that
H (r, u, H
∗
(r, u, s)) ≡ s for (r, u, s) ∈ IR
+
× IR × IR .
We will set h(r, u) = H
∗
(r, u, 0) and use other notations from the previous sec-
tion. In particular, in the functions L, H , and their derivatives L
r
, L
u
,..., H
r
, H
u
,...,
variables p and q are replaced by h(r, u) and h
r
+ h
u
h respectively.
Theorem 8.11
Operator
(8.44)
is a sign-invariant of the equation
(8.43)
if
H
satis-
fies the following identity for all
(r, u) ∈ IR
+
× IR
:
F(r, u) =−h
u
L + L
r
+ L
u
h + L
p
(h
r
+ h
u
h)
+ L
q
h
rr
+ 2h
ru
h + h
uu
h
2
+ h
u
h
r
+ (h
u
)
2
h
≡ 0.
(8.45)
Proof. Let J = H (r, u, u
r
),sou
r
= H
∗
(r, u, J ) and J satisfies
J
t
= H
u
u
t
+ H
p
u
tr
. (8.46)
By (8.43), u
t
= L and hence,
u
tr
= L
r
+ L
u
H
∗
+ L
p
u
rr
+ L
q
u
rrr
. (8.47)
Let us derive for J a linear parabolic PDE of the form (8.24). Since the coefficient
F in this equation is eventually derived by the standard linearization of the equation
about J = 0, this allows us to calculate the derivatives in (8.46) and (8.47) from the
equality u
r
= h(r, u). In this case,
u
rr
= h
r
+ h
u
h and u
rrr
= h
rr
+ 2h
ru
h + h
uu
h
2
+ h
u
h
r
+ (h
u
)
2
h.
Substituting into (8.46) yields (8.24) with F = 0 given by (8.45). By the MP, this
completes the proof.
8.3.1 Quasilinear heat equations
Considerthe equation(8.1) with k = ϕ
, which, for radial solutionsu = u(r, t) using
the radial Laplacian in IR
N
, is written as
u
t
= (k(u)u
r
)
r
+
N−1
r
k(u)u
r
+ f (u). (8.48)
We take the SI (8.44) in the semilinear form
H[u] = u
r
− g(r)(u), (8.49)
© 2007 by Taylor & Francis Group, LLC
396 Exact Solutions and Invariant Subspaces
where g(r) and (u) are unknown functions. Identity (8.45) then becomes simpler,
F(r, u) = g
3
(r)[
2
(k)
]
+g(r)g
(r)[3k
2
+ 2k
] + g(r)( f
− f
)
+g
(r)k +
N−1
r
g
2
(r)k
2
+
g
(r) −
g(r)
r
k
≡ 0.
(8.50)
In general, the right-hand side belongs to a 6D subspace. For N = 1, the last term
vanishes. We studied a similar identity and obtained special invariant sets and exact
solutionsin Section 7.5 by using the ODE (7.158) for g (in order to match the results,
g should be replaced by −g here). In the present more difficult case of dimensions
N > 1, we restrict our attention to the three most interesting cases:
(I) g(r) = r, (II) g(r) =
1
r
,and(III) g(r) = r
1−N
in dimension N ≥ 3.
(I) g(r) = r.
Proposition 8.12
Operator
H[u] = u
r
−r(u)
is a sign-invariant of
(8.48)
if
¯
(u) = k(u)(u) ≡ 0
satisfies the system
I
1
(u) ≡
¯
= 0,
I
2
(u) ≡
k
k
N +
f
¯
+
2ln|
¯
|+
f
¯
= 0.
(8.51)
Proof. Identity (8.50) with g(r) = r reads F (r, u) = r
3
¯
2
k
2
I
1
+ r
1
k
I
2
, and system
(8.51) follows.
The first ODE in (8.51) implies that
¯
(u) = au + b is linear, and solving the
second equation for f (u) yields PDEs possessing blow-up exact solutions.
Example 8.13 (Focusing blow-up interfaces) Consider the PDE
u
t
= ϕ(u) − (au + b)
N +
2a
ϕ
(u)
G(u)
, (8.52)
where ϕ(u) is arbitrary, ϕ
(u) ≥ 0, and a = 0. Set
G(u) =
ϕ
(u) du
au+b
,
assuming that the inverse function G
−1
exists. In the class of radially symmetric
solutions u = u(r, t), with r =|x |, this equation admits the following SI:
H[u] ≡ u
r
−r
au+b
ϕ
(u)
. (8.53)
The semilinear operator (8.53) is also a zero-invariant of (8.52), so H[u(r, t)] = 0
for t > 0, provided that H[u
0
] = 0. This implies that (G(u))
r
− r = 0 holds,
and integrating yields G(u) = C
1
(t) +
1
2
r
2
. Plugging into (8.52) gives the explicit
solution
G(u(x , t)) =
1
2
|x|
2
− e
−2at
. (8.54)
Setting a = 1andb = 0, and assuming that ϕ satisfies Osgood’s criterion
∞
ϕ
(u)
u
du < ∞,
© 2007 by Taylor & Francis Group, LLC
8 Sign-Invariants and Exact Solutions 397
u(r, t)
s(t
1
)
s(t
3
)
s(t
2
)
t
1
t
2
t
3
r =|x |
t
1
< t
2
< t
3
0
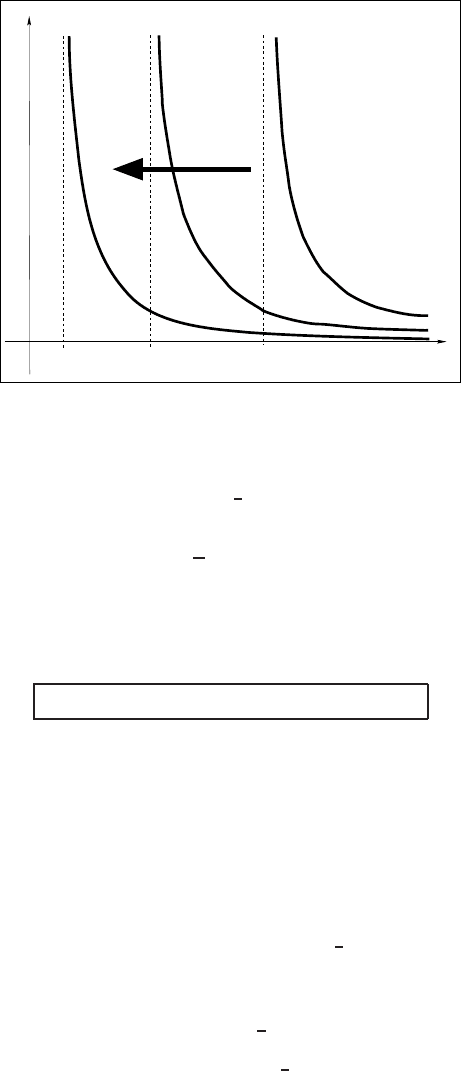
Figure 8.2 Blow-up solution (8.55) with focusing singular interface.
we obtain the blow-up solution
u(r, t) = G
−1
1
2
|x|
2
− e
−2t
. (8.55)
This has the blow-up interface (surface) focusing at the origin in infinite time,
|x|=s(t) ≡
√
2e
−t
→ 0ast →∞,
as shown in Figure 8.2.
Partially invariant 2D subspace. Let us present an invariant interpretation of such
solutions. Setting G(u) = v in (8.52) yields
v
t
= F[v] ≡ (v)(v − N ) + a(|∇v|
2
− 2v), (8.56)
where (v) = ϕ
(G
−1
(v)). Consider (8.56) on the 2D subspace W
2
= L{1, |x |
2
},
v(x , t) = C
1
(t) + C
2
(t) |x |
2
.
Obviously, W
2
is not invariant under the operator F,since
F[v] = (v)N(2C
2
− 1) + a
2C
2
|x|
2
(2C
2
− 1) − 2C
1
, (8.57)
so F[W
2
] ⊆ W
2
. But it follows from (8.57) that F admits the invariant set (i.e., W
2
is partially invariant)
M =
C
1
+ C
2
|x|
2
: C
2
=
1
2
.
Indeed, F[M] ⊆ W
2
, and more precisely, F[M] ⊆ W
1
= L{1}. Therefore, as in
several examplesin Chapter 7, the PDE (8.56) on M is reducedto an overdetermined
DS, which has a solution. Then, setting C
2
=
1
2
in (8.57),i.e.,lookingfor the solution
v(x , t) = C
1
(t) +
1
2
|x|
2
,
© 2007 by Taylor & Francis Group, LLC
398 Exact Solutions and Invariant Subspaces
yields F[v] =−2aC
1
. Hence, (8.56) on M becomes an elementary linear ODE,
C
1
=−2aC
1
,
which yields the solution (8.54). Setting in (8.52) ϕ(u) = u
2
, a = b = 1, and
G(u) = 2[u − ln(1 +u)] yields the PDE
u
t
= u
2
+
2(1+u)
u
ln(1 + u) − (N +2)(1 + u).
If ϕ(u) = ln(1 +u
2
), a = 1, and b = 0, the PDE is
u
t
= ln(1 + u
2
) − 2(1 +u
2
) tan
−1
u − Nu.
Example 8.14 (Fourth-order parabolic equation) We easily extend this simple
partial invariance analysis to higher-order PDEs, e.g., to the quasilinear equation
v
t
= (v)[−
2
v + 4N(N + 2)] + α
(v)
2
− 8(N +2)
2
v
possessing the solution on a set from L{1, |x|
4
},
v(x , t) =
1
2
|x|
4
+ e
−8(N+2)
2
αt
.
Indeed, since |x|
4
= 4(N + 2)|x|
2
and
2
|x|
4
= 8N(N + 2), the first term in
the equation vanishes, while, in the second term, we observe cancellation of both the
|x|
4
-projections.
Example 8.15 (Quasilinear KdV-type PDE) The following third-order nonlinear
dispersion equation:
v
t
= (v)(v
xxx
− 6) + α(v
x
v
xx
− 18v)
admits the solution on M ⊂ L{1, x
3
},
v(x , t) = x
3
+ e
−18αt
.
It is easy to reconstructother higher-ordermodels, including two and more nonlinear
terms annihilating on suitable sets M ⊂ W .
(II) g(r) =
1
r
.
Proposition 8.16
Operator
H[u] = u
r
−
1
r
(u) (8.58)
is a sign-invariant of the equation
(8.48)
iff
(u) ≡ 0
satisfies the system
I
1
(u) ≡ f
− f
= 0, (8.59)
I
2
(u) ≡ (k)
+ (N −4)k
− 2k
− 2(N −2)k = 0. (8.60)
Proof. Setting g(r) =
1
r
in (8.50) yields F(r, u) =
1
r
I
1
(u) +
(u)
r
3
I
2
(u).
The first ODE in the system (8.59), (8.60) implies that
f (u) = λ(u) (8.61)
© 2007 by Taylor & Francis Group, LLC
8 Sign-Invariants and Exact Solutions 399
for some constant λ. The next second-order ODE (8.60) for (u) cannot be solved
explicitly in general. We will show how to construct explicit solutions of (8.48) by
using the fact that, under hypotheses (8.61) and (8.60), the operator (8.58) is a zero-
invariant of the above quasilinear heat equation.
Example 8.17 Consider (8.48) with f(u) given by (8.61),
u
t
= k(u)
u
rr
+
N−1
r
u
r
+ k
(u)(u
r
)
2
+λ(u). (8.62)
Using the zero-invariance of the operator (8.58) means that, for t > 0,
u
r
−
1
r
(u)= 0 ⇒v≡
u
dz
(z)
= lnr+ C
1
(t). (8.63)
It follows from (8.62) thatv(r, t) solves
v
t
= k(u)
v
rr
+
N−1
r
v
r
+(k)
u
(v
r
)
2
+λ.
Substitutingv(r, t) from (8.63) yields
C
1
=
1
r
2
G(v)+λ, where G(v)=(k)
u
(u)+(N− 2)k(u). (8.64)
Equation (8.60) implies that there exists a constant d∈ IR such that
G(v)= d e
2v
. (8.65)
Indeed, it follows from (8.63) that u
v
=(u), and (8.64) yields
G
v
=(k)
uu
+(N− 2)k
.
Therefore, by (8.60), G
v
= 2G, so (8.65) holds. Finally, by (8.64), C
1
= d e
2C
1
+λ,
so, on integration,
C
1
(t)=
1
2
ln
λe
2λt
1−de
2λt
. (8.66)
By (8.63), this determines the explicit solution of (8.62).
Consider some particular cases:
(i) In the case of the semilinear heat equation with k(u) ≡ 1 in (8.62),
u
t
= u + λ(u),
the SI has the form H[u]= u
r
−
1
r
(u), where (u) solves the following nonlinear
ODE (cf. (8.60)):
− 2
− 2(N −2) = 0.
The corresponding explicit solution is again given by (8.63) and (8.66).
(ii) For N = 2, (8.60) can be rewritten as (k)
− 2(k)
= 0. Setting k = ,
and hence, =
2
, from (8.62) with λ = 1, we obtain the quasilinear equation
u
t
=
1
r
r
2
(u) u
r
r
+ 2
(u)
admitting the sign and zero-invariant H[u] = u
r
−
2
r
(u)
(u)
. The corresponding ex-
plicit solution is
(u(r, t)) = C
1
(t)r
2
,whereC
1
(t) satisfies
C
1
= 2C
1
(C
1
+ 1).
© 2007 by Taylor & Francis Group, LLC
400 Exact Solutions and Invariant Subspaces
(iii) For the function
(u)=(2− N)u (N= 2), (8.67)
which simplifies the ODE (8.60), k(u) has to satisfy (2− N)(ku)
=(4− N)k
.
Integrating yields the heat conduction coefficients k(u)= a+ bu
2
2−N
, i.e., the PDE
u
t
=∇·
a+ bu
2
N−2
∇u
+(2− N)u.
(iv) Trying in (8.60) the constant function (u)≡γ= 0 yields a linear ODE for
k(u),γ
2
k
+(N− 4)γ k
− 2(N− 2)k= 0. Hence, k(u)= ae
2−N
γ
u
+ be
2
γ
u
,which
yields the PDE withγ= 1,
u
t
=∇·
ae
(2−N)u
+ be
2u
∇u
+ 1.
(v) Finally, trying in (8.60) the linear function (cf. (8.67))(u)=γ u,γ= 0, yields
the second-order Euler ODE
u
2
k
+
2+
N−4
γ
uk
−
2(γ
+N−2)
γ
2
k= 0. (8.68)
Here, setting k(u)= u
ρ
determines th
e characteristic equation
ρ
2
+
1+
N−4
γ
ρ−
2(γ
+N−2)
γ
2
= 0.
Therefore, ρ
1
=
2
γ
and ρ
2
=−1−
N−2
γ
.Ifγ=−N, ρ
1
=ρ
2
, and the general
solution of (8.68) has the form k(u)= au
ρ
1
+ bu
ρ
2
, i.e., the PDE withγ= 1,
u
t
=∇·[(au
2
+ bu
1−N
)∇u]+ u.
If γ=−N, the general solution is k(u)= u
−
2
N
(a ln u+ b), giving the following
parabolic equation:
u
t
=∇·
u
−
2
N
ln u∇u
− Nu.
It follows from (8.63) with (u)=γ u that, in both cases, the explicit solution is
u(r, t)= r
γ
e
γ C
1
(t)
,whereC
1
(t) solves
C
1
=
a(N+γ)e
2C
1
+λ for γ=−N,
−aNe
2C
1
+λ for γ=−N.
(III) g(r)= r
1−N
. In this case, (8.50) implies that
F(r, u)= r
3(1−N)
2
(k)
− 2(N− 1)r
1−2N
(k)
+ r
1−N
( f
− f
).
By Theorem 8.11, H[u] = u
r
−r
1−N
(u) (N ≥ 3) is an SI of (8.48) if
(k)
= 0,
f
− f
= 0.
This yields quasilinear heat equations, which have been considered in Section 8.2 in
a more general setting; cf. (8.29).
© 2007 by Taylor & Francis Group, LLC

8 Sign-Invariants and Exact Solutions 401
In the 1D case N= 1, there exists another trivial choice g(r)≡ 1, where the SI
(8.49) has the form
H[u]= u
r
−(u).
Then (8.50) implies that f solves the ODE
2
(k)
+ f
− f
= 0.
Integrating once yields f(u)= [a−(k)
]. Using the operator H[u]givesthe
standard traveling wave solutions u=θ(x−λt).
8.4 Sign-invariants of the form u
t
− m(u)(u
x
)
2
− M(u)
In this section, we find more general SIs and explicit solutions of quasilinear heat
equations with various nonlinearities, including the following two models:
u
t
= u
xx
+ du
2
√
ln u+
1
√
ln u
+ u(2lnu+ 1),
u
t
=(
√
u)
xx
+
1
tan
−1
√
u
+ 1
(1+ 4
√
u tan
−1
√
u)(1+ u). (8.69)
As in Section 8.2, consider first the general nonlinear parabolic PDE
P[u]≡ u
t
− L(u, u
x
, u
xx
)= 0inIR
N
× IR
+
, (8.70)
where the function L(u, p, q) satisfies (8.11). Set (cf. (8.19))
H[u]= u
t
−ψ(u, u
x
), (8.71)
where ψ(u, p) is a smooth function. Assume that +
0
(u, p, s) is well defined by
(8.18). Let L
−1
(u, p) denote the function (8.20). In the notation of Section 8.2, the
following result is derived.
Theorem 8.18
Operator
(8.71)
is a sign-invariant of the equation
(8.70)
if
ψ(u, p)
satisfies the identity
(
cf.
(8.21))
F(u, p) = (L
u
− ψ
u
)ψ + (L
p
ψ
u
− L
u
ψ
p
) p
+ L
q
ψ
uu
p
2
+ 2ψ
up
L
−1
p + ψ
pp
(L
−1
)
2
+ ψ
u
L
−1
≡ 0.
(8.72)
Proof. This is the same as in Section 8.2. By calculating J
t
from a slightly different
formula than (8.22),
J
t
= u
tt
− ψ
u
u
t
− ψ
p
u
xt
,
u
tt
from (8.70), and also u
tx
and u
txx
by differentiating J = u
t
− ψ,wederivea
PDE such as (8.24). We also use the identities, following from (8.18),
L
u
+ L
q
(+
0
)
u
≡ 0, L
p
+ L
q
(+
0
)
p
≡ 0, and L
q
(+
0
)
s
≡ 1.
This completes the proof.
Let us solve the nonlinear PDE (8.72) for ψ(u, p) in some particular cases.
© 2007 by Taylor & Francis Group, LLC
402 Exact Solutions and Invariant Subspaces
8.4.1 Quasilinear heat equations
Consider the 1D quasilinear equation (8.1). Without loss of generality, we may take
it in the form of
P[u] ≡ u
t
− [ϕ(u)u
xx
+ f (u)] = 0, (8.73)
with a smooth function ϕ(u) ≥ 0. The equation (8.1) is reduced to (8.73) by the
transformation ˆu =
u
k(s) ds. Set
H[u] ≡ u
t
−
m(u)(u
x
)
2
+ M(u)
, (8.74)
where m(u) and M(u) are smooth unknown functions.
Corollary 8.19
Operator
(8.74)
is a sign-invariantof equation
(8.73)
if the functions
m(u)
and
M(u)
satisfy the following system of ODEs:
I
1
(u) ≡ m
ϕ + 4mm
− m
2
ϕ
ϕ
+
2m
3
ϕ
= 0, (8.75)
I
2
(u) ≡ ϕM
+ 4m
M − mf
−5m
f +
4m
2
ϕ
(M − f ) + m
ϕ
ϕ
f = 0,
(8.76)
I
3
(u) ≡ f
M − fM
+
2m
ϕ
(M − f )
2
+ M
ϕ
ϕ
(M − f ) = 0. (8.77)
Proof. ODEs (8.75)–(8.77) follow from the identity
F(u, p) ≡ I
1
p
4
+ I
2
p
2
+ I
3
,
which is easily derived from (8.72).
We now reduce system (8.75)–(8.77) to a single equation. Let us introduce the
new function v(x, t) by setting
u = E(v), (8.78)
where E is a monotone solution of the ODE
E
=
m(E)
ϕ(E)
(E
)
2
. (8.79)
In terms of v, (8.73) has the form
˜
P[v] ≡ v
t
−
ϕ(E)v
xx
+ ϕ(E)
E
E
(v
x
)
2
+
f (E)
E
, (8.80)
and the SI (8.74) becomes
˜
H[v] ≡ v
t
−
E
m(E)(v
x
)
2
+
M(E)
E
. (8.81)
It follows from (8.79) that the coefficients of (v
x
)
2
in (8.80) and (8.81) coincide, and
(8.75) implies that these are linear functions of v. By differentiating,
(E
m(E))
vv
≡
(E
)
3
ϕ(E)
I
1
(E) = 0.
Thus setting
ϕ(E)
E
E
= E
m(E) = av + b, (8.82)
where a and b are arbitrary constants, and
ϕ(E(v)) =˜ϕ(v),
f (E(v))
E
(v)
=
˜
f (v),
M(E(v))
E
(v)
=
˜
M(v), (8.83)
© 2007 by Taylor & Francis Group, LLC
