Galaktionov V.A., Svirshchevskii S.R. Exact Solutions and Invariant Subspaces of Nonlinear Partial Differential Equations in Mechanics and Physics

Подождите немного. Документ загружается.

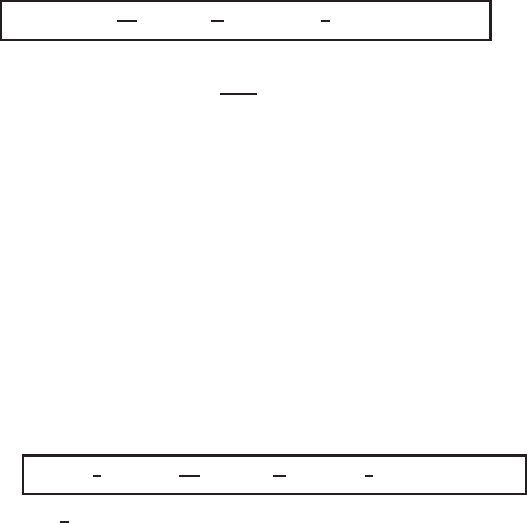
8 Sign-Invariants and Exact Solutions 423



−

)

+ 2V





)

+ 2V

+ 2



, (8.212)

with the explicit solution

∗

(x , t) = tan4t +

cos4t

sin2x ∈

= L{1, sin 2x}, (8.213)

where

is invariant under the second operator F

in (8.212). The set M ⊂

which the ﬁrst operator vanishes, F

[V ] = 0onM, takes the form

M =



V = C

+ C

sin2x : C

− C

+ 1 = 0



Plugging V = C

(t) + C

(t) sin 2x ∈

into (8.212) gives the overdetermined DS









= 2(C

+ C

) + 2,



= 4C

− C

+ 1 = 0,

(8.214)

which generates the nontrivial solution (8.213).

Example 8.45 (Fourth-order parabolic equation) Clearly, (8.213) solves the gen-

eral equation (8.210) with operators F

1,2

from (8.212). Exactly the same solution

(8.213) with the same DS (8.214) occurs for the fourth-order PDE

=−

xxxx

−

)

+ 2V +

)

+ 2V

+ 2,

since V

=−

xxxx

Remarks and comments on the literature

The ideas and methodology of differential constraints are classical in PDE theory and initially

appeared for ﬁrst-order equations. Lagrange applied differential constraints to determine total

integrals of nonlinear equations with two independent variables,

F(x, y, u, u

, u

) = 0.

Darboux extended this technique to the second-order PDEs [139], which previously had been

studied by Monge and Amp`ere by means of ﬁrst integrals. A full history of the early years of

differential substitution and constraint approaches can be found in Goursat [260] and Forsyth

[196]. See also a survey in Kaptsov [326] devoted to some generalizations of the classical

Darboux method. Differential constraints are known to lead to difﬁcult systems of PDEs.

A general theory of such overdetermined systems was developed by Riquier, Cartan, Ritt,

Spencer, and others; see Pommaret [468] for a detailed survey.

Some modern versions ofdifferential constraint approaches are related tothe classical semi-

inverse method that is well known in Continuum Mechanics and more applied areas; see a

survey paper by Nem´eny [433]. The link between the semi-inverse method and symmetries of

PDEs was ﬁrst noted by Birkhoff [61] in application to hydrodynamic problems. In particular,

Birkhoff introduced the concept of equations, written in separate form, i.e., in the form of a

linear combination



i=1

(χ)

(µ),

where the coefﬁcients ,

(χ) depend on the set of variables χ ={χ

, ..., χ

} and functions of

424 Exact Solutions and Invariant Subspaces

these variables, whereas 

(µ) depend on different variables µ ={µ

, ..., µ

} and functions

of these variables. Reducing PDEs to such separate forms makes the method successful. See

further comments in the survey in [474]. Later on, in the 1960s, a systematic approach to

differential constraints in gas dynamics was proposed by Yanenko [582], which is reﬂected in

the book [525]; see also other applications and references in [13].

In this chapter, we use the basic notions of SIs introduced in [219], where a number of

presented results can be found.

§8.1.Let us mention again that, in many fundamental problems for quasilinear parabolic

PDEs, deriving suitable one-sided estimates (or SIs) plays a key role for the existence, unique-

ness, regularity, and different asymptotic results. This is explained in many books; see [33,

205, 245, 509, 530, 533, 550].

§8.2.We follow [219] and use SIs proposed in [215]; see more references in [509, Ch. 5].

Corollary 8.2 can be found in [215] and [509, p. 303]. Kirchhoff’s transformations in heat

conduction theory have been used since 1894, [348].

We mention again that some ideas of the SIs had the origin in blow-up singularity analysis

of combustion reaction-diffusion PDEs. The ﬁrst concept of SI analysis is associated with the

notion of the ψ-criticality of solutions of parabolic equations playing an important role in

blow-up theory; see details and references in [509, Ch. 5]. In general settings, we deal with

PDIs of the type

H[u] ≡ u

−ψ(x, t, u, ∇u) ≥ 0inIR

for t > 0, (8.215)

with aprioriunknown functions ψ(x, t, u, ∇u) to be determined from the invariance condi-

tion. According to(8.215), the zero-criticality of the solution u(x, t), i.e.,the ψ -criticality with

ψ(·) ≡ 0, implies that u

≥ 0 holds for t > 0ifu

(x, 0) ≥ 0 initially. In combustion prob-

lems, the last inequality is known to characterize initial temperature of the critical ignition.

Therefore, (8.215) is a natural extension of the critical property, such as the ψ-criticality with

respect to a given function ψ. General ψ-critical conditions (8.215) of solutions of parabolic

PDEs were introduced in [215]; see extended results and references in [509, Ch. 5]. Estimates

of the type (8.215) with different functions ψ have been used in several problems for quasi-

linear heat equations (8.1), such as heat localization and blow-up behavior, [509, Ch. 5, 7]. A

similar idea to derive estimates of the type (8.215) with ψ = ψ(u) for some class of quasi-

linear heat equations was used in [533], where the applications to blow-up problems are also

given. For the semilinear heat equation (8.4), the ψ-criticality (8.215) with ψ = δ f (u), δ =

constant, has been employed in [207].

§8.3.The main results are taken from [219]. Function g(r) = r in the SI (8.49) was introduced

in [207], two other cases, g(r) =

and g(r) = r

1−N

, were established in [219].

This concept of SI analysis uses the idea by Friedman and McLeod [207]. In the study

of single point blow-up for semilinear heat equations (8.4) with nonlinearities f (u) = e

(the Frank–Kamenetskii equation or solid fuel model, [594]) and f (u) = u

, they proposed

deriving the so-called gradient estimate by using a sign-type analysis of the following ﬁrst-

order operator for radial solutions u = u(r, t), r =|x|:

∗

[u] ≡ u

+r (u) for t > 0. (8.216)

A suitable choice of the function  provides us with an optimal estimate for blow-up solu-

tions and depends on f . For quasilinear PDEs (8.1), the SI operator takes the form H

∗

[u] =

k(u)u

+ r (u). A crucial problem is then to choose an optimal function (u) satisfying a

nonlinear ordinary differential inequality, depending on the coefﬁcients k(u) and f (u) of the

parabolic equation (8.1). Various generalizations of this approach to quasilinear heat equations

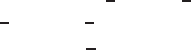
8 Sign-Invariants and Exact Solutions 425

(8.1) was performed in [233, 243]; see [245, Sect. 10.4], where the most general computations

are presented.

This approach has been extended [233] to PDEs with the p-Laplace operator

=∇·(|∇u|

∇u) + f (u), (8.217)

where the corresponding SIs are H

∗

[u] ≡|u

+r (u).

There exists a direct relation between the results on SIs and a geometric Sturmian theory of

nonlinear 1D parabolic equations, [226, Ch. 7]. Geometric theory of such PDEs uses the fact

that proper, complete (i.e., sufﬁciently dense in a natural geometric sense) sets B of particular

exact solutions can determine a property of the B-convexity/concavity that is deﬁned with

respect to the given functional set B. This property is then preserved with time and reduces to

the time-invariance of the sign of a certain nonlinear operator (actually, it is a SI) on evolution

orbits generated by the parabolic equation.

For second-order parabolic equations, some results on generalized conditional symmetries

can be translated to sign invariants; see e.g., [476] and [481], where exact solutions are con-

structed for equations u

=∇·(B(u)∇u) + A(x, u).

§8.4.The main results are obtained in [219]. Examples 8.22, 8.23 and 8.24 represent rather

elementary equations possessing solutions on invariant subspaces or on sets of lower dimen-

sions 1, 2, or 3. Various extensions of similar differential constraints to parabolic PDEs with

non-constant coefﬁcients, extra convection, and gradient diffusivity terms in Sections 8.1–8.4

can be found in [474], together with a quality survey on the relation between semi-inverse

methods and symmetries of PDEs.

The 1D invariant subspaces correspond to the functional separation of variables,wherethe

additively separable solutions

u(x, t) = φ(A(x) + B(t ))

are studied. Necessary and sufﬁcient conditions for such a general additive separation of a

PDE were obtained in [310], being an extension of the classical St¨ackel form (1893) [534].

For this separation technique, the results by Steuerwald (1936) [535] have been found ef-

fective for ﬁnding standing waves. See applications to the sine-Gordon (Enneper) equation,

= sin u, in [521], where solutions of the form u(x, t) = F( f

(t) f

(x)) were described.

Such a structure of solutions was earlier derived by Seeger (1953) by integrating Darboux’s

equation (1894) [142] for Enneper surfaces (pseudo-spherical surfaces with at least one set of

planar lines of curvature), and was shown to belong to the manifold of solutions obtained by

Steuerwald [535]; see [521].

This functional separation of variables was applied in [316] (see also [13, Ch. 5]) to the 2D

equation of vortex structures of an inviscid ﬂuid,

u ≡ u

+ u

= F(u), (8.218)

where u is stream function and F(u) is given (equation (8.218) was derived by H. Lamb

[368]; see [29]). According to this method of generalized separation of variables (GSV), it

was shown [316] that (8.218) admits nontrivial solutions

u(x, y) = h( f (x) + g(y)), (8.219)

where h, f ,andg are some unknown functions to be determined (cf. another application in

[423]) iff the right-hand side F takes one of the following ﬁve forms:

+ Be

−2u

, Au lnu + Bu, A sin u + B(sinu ln|tan

|+2sin

A sinhu + B(sinh u ln|tanh

|+2sinh

A sinhu + B(sinh u tan

−1

u/4

+ 2cosh

426 Exact Solutions and Invariant Subspaces

where A and B are constants. All these Kaptsov’s solutions of the equation (8.218) are given

by explicit formulae. Two equations from this list, the Tzitz´eica equation,

∗

u= e

− e

−2u

, (8.220)

and the Bonnet equation [71], u = sin u, are well known and exhibit special symmetry

properties and solutions; see [10, Sect. 12.3], [321] for N-soliton solutions of (8.220) and

also a survey in [138]. Two Lax pairs of (8.220) are known, and the ﬁrst one was found by

Tzitz´eica himself in 1908, and the second pair by Mikhailov seventy years later, [138, p. 2094].

Similar separation results for (8.218) were also obtained in [423], where more general aspects

of this functional separation are presented and applied to hyperbolic and elliptic PDEs (in

Riemannian and pseudo-Riemannian spaces) with the right-hand side F= F(x, y, u).

Setting in (8.219) f = ln

f and g= lnˆg yields

u(x, y)= h(ln(

f(x)ˆg(y)))≡

f(x)ˆg(y)),

exhibiting a typical structure of 1D subspaces with unknown basic functions, so the solutions

of this type are naturally associated with invariant subspaces or sets. On the other hand, dif-

ferentiating in x yields the typical SI structure u

= f



(x)H(u) studied in Section 8.3. As a

next step, for solutions on 2D subspaces u(x, y)= h( f

(x)+ f

(x)g(y)), the corresponding

differential constraint takes the form u

= g(x)h(y)H(u) (cf. [474]), or, which is the same,

[

g(x)H(u)

]

= 0. One can modify such a constraint to include solutions on three or more-

dimensional subspaces, though computations then become much more technical and possibly

unbearable.

Similar ideas apply to the Grad–Shafranov equation that describes axisymmetric steady

ﬂows of an inviscid ﬂuid [29]

+ u

= r

G(u)+ F(u),

where F and G are arbitrary functions; see [317, 322, 421]. This approach gives a similar

classiﬁcation for the semilinear Klein–Gordon equation,

− u

= F(u),

(see also [319] where the differential constraints applied) and can be extended to the semi-

linear and quasilinear heat equations u

−ϕ(u)u

= F(u); see a general classiﬁcation in

[482]. In [173], this method was applied to generalized p-Laplacian equations with source,

= (ϕ(u)(u

)

+F(u), occurring in the theory of turbulent diffusion and non-Newtonian,

dilatable, or pseudo-plastic ﬂuids. In [172], the GSV was used for general quasilinear wave

equations

= (ϕ(u)u

)

+ F(u).

The algorithm of the GSV-method admits a generalization, where the derivative u

is included

in the separation formula (cf. (8.219)) f(u, u

) = a(t)+b(x); see [597] (and earlier results in

[118]) applied to second-order parabolic PDEs u

= A(u, u

+ B(u, u

), and [596] for

the KdV-type equations u

= u

xxx

+ A(u, u

+ B(u, u

). See also [479, 483, 480] for

a generalization of separation techniques applied to PDEs u

= (D(u)u

)

+ B(x)Q(u) and

∗

This equation was found by Romanian geometer Georges Tzitz´eica in 1907, [564]. It is used in the ﬁeld

of geometry and is concerned with surfaces, on which the total curvature at each point is proportional

to the fourth power of the distance from a ﬁxed point to the tangent plane. He established its invariance

under a B¨acklund transformation, and also constructed, in 1910, a linear representation of solutions

incorporating a spectral parameter, which was rediscovered in the soliton theory seventy years later.

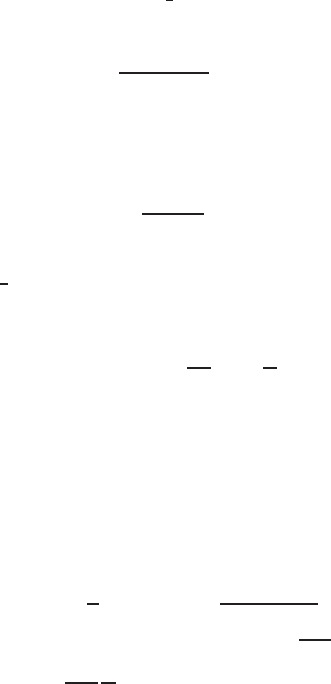
8 Sign-Invariants and Exact Solutions 427

other equations. Paper[598] contains acomplete classiﬁcation of the nonlinear wave equations

in IR

× IR ,

= A(u)u

+ B(u)u

+ C(u, u

, u

admitting solutions f (u) = X (x) + Y (y) + T (t ).

In particular, the GSV approach easily gives the solution in Example 8.24. On the other

hand, the GSV cannot detect elementary solutions in Examples 8.22 and 8.23 dealing with

subspaces of dimensions three and two respectively. A generalization of the GSV method of

[316] to two and higher-dimensional subspaces seems to be an interesting and challenging

problem. The main difﬁculty is to check whether in 2D, the method would lead to such a

simple, closed algorithm of separation as it does in 1D.

Some of the solutions on invariant subspaces and sets can be obtained by constructing

suitable differential constraints of higher order. See [325], where, in addition, a new family of

exact solutions was constructed for the fast diffusion equation

= (u

−

)

by using a third-order nonlinear differential constraint. The solutions are

u(x, t ) =−



(x)T



(t)

[X (x)+T (t )]

, where



)

= (c

+ c

X + c

+ c

)



)

= A(c

− c

T +c

− c

)

(X are T are expressed in terms of the Weierstrass function ℘)andc

and A are constants.

Another special parabolic equation,

+(v

)

admits the exact solution v = v(x, t) given implicitly by

−

[ln|sinh(3v + 2x)|−ln|cos(v + 2x)|] = t,

which was constructed in [66] by using nonclassical potential symmetries. Notice another

implicitly given solution of the equation of superslow diffusion,

= (e

−1/u

)

, where u =−

lnv

,v=

− w

)

and |x|=[2 + ln(2t)]w + (c −w) ln(c −w) − (c + w) ln(c + w)

−1/u

is associated with the famous Arrhenius law in thermodynamics; here c > 0isa

constant). This solution is key in asymptotic theory of superslow diffusion, [245, Ch. 3].

Let us brieﬂy focus on another, rather unusual applications of SIs. Consider the Aronson–

B´enilan semiconvexity estimate [17] for weak nonnegative solutions of the PME

= u

in IR

× IR

This plays a key role in general PME theory (see details and references in [245, Ch. 2]), and

has the form

u

m−1

≥−

, where C =

(m−1)N

m[N(m−1)+2]

It can be treated as the ψ-criticality (8.215). Since u

m−1

≡

m−1

− mu

m−2

|∇u|

the above estimate is exactly (8.215) with the function ψ, depending on the time-variable t,

ψ(t, u, |∇u|) = mu

m−2

|∇u|

−

m−1

§8.5.We mainly follow [236]. In [480], similar Hamilton–Jacobi SIs and solutions were

obtained for the quasilinear heat equation with lower-order reaction-convection x-dependent

terms, u

= (D(u)u

)

+ Q(x, u)u

+ P(x, u).

428 Exact Solutions and Invariant Subspaces

Equations (8.126) have a wide ﬁeld of applications in combustion theory, plasma physics,

biophysics, and mechanics of porous media. Such quasilinear evolution PDEs are popular

in group-theoretical, classical, and nonclassical methods of ﬁnding exact solutions, which

are responsible for different nonlinear phenomena. There are many directions of mathemat-

ical theory of the quasilinear heat equations concerning existence, uniqueness, regularity,

asymptotics, and other aspects of the qualitative properties of the solutions. See surveys in

[245, 509]. Construction of appropriate one-sided SIs (barrier techniques) plays a fundamen-

tal role in qualitative theory of nonlinear parabolic PDEs. For instance, classical interior regu-

larity bounds of Bernstein or similar type in the theory of parabolic equations are derived via

upper bounds on the term ξ(x)[(ρ(v))

]

(ξ ∈ C

∞

is a cut-off function, ρ depends on the

coefﬁcients of the PDE), satisfying a parabolic differential inequality. See Oleinik–Kruzhkov

[442].

A general description of explicit solutions from Section 8.5.2 was performed in [130] and

here we present a couple of examples, showing some links with the SIs. In the particular case,

K = 0andc =−

, where (8.179) is the semilinear heat equation

= v

− 2G(v), (8.221)

the evolutionary invariant set governed by the corresponding stationary ODE was introduced

in [317]. These explicit solutions have a strong exponential-type nature and have been con-

structed in [100, 329] by the Penlev´e expansion (computationally related to the Baker–Hirota

bilinear method). A complete analysis of the types of such explicit solutions of (8.221) with

the cubic function (8.177) can be found in [130]. A general classiﬁcation of exact solutions

from [130] applies to the quasilinear equation (8.179). Here, we have considered a few il-

lustrative examples only. In addition, let us mention [595], where one- (a traveling wave) and

two-soliton solutions of the third-order non-integrable PDEs with various nonlinear terms (see

(4.244) in Chapter 4) were obtained by a variant of the classical dressing method.

SIs and the corresponding solutions of parabolic PDEs with the p-Laplacian operator

=∇·(|∇u|

∇u) + f (u) in IR

× IR

(8.222)

are described in [237]. Such types of diffusion-like operators appear, for instance, in the theory

of non-Newtonian liquids and in some turbulence problems; seeBarenblatt [25] and references

therein. Such models are common in combustion problems related to solid fuels. SIs were also

detected in [237] for parabolic PDEs with a general gradient-dependent nonlinearity,

= h(|∇u|)u + f (u). (8.223)

Such nonlinearities are used in mean curvature ﬂow equations related to differential geome-

try, and to problems of motion of closed hypersurfaces in IR

by its mean curvature, [79,

107]. These PDEs are important in phase transition phenomena, [271]. The 1D equation

(8.222) corresponds to h( p) = (σ + 1) p

in (8.223). A typical example is

1+(u

)

−

in IR × IR

which describes, after a surface parameterization, the evolution of cylindrically symmetric

hypersurfaces moving by mean curvature in IR

, [178, 532]. Notice also generalized Burgers’

equation (see [362] and extensions in [115])

+ uu

=±ν

1−(u

)

[1+(u

)

]

which contains a gradient nonlinearity and describes strongly nonlinear processes governing

high-amplitude and gradient phenomena. For small gradients |u

|&1, this leads to Burgers’

equation u

+ uu

= νu

introduced by I.M. Burgers in 1948.

CHAPTER 9

Invariant Subspaces for Discrete Operators,

Moving Mesh Methods, and Lattices

In this chapter, we apply basic techniques of linear invariant subspaces and sets to nonlinear

discrete operators and equations. Symmetry analysis of ﬁnite-difference equations is a clas-

sical subject of group theory; see Handbook of Group Analysis [10, Ch. 17] for key results

and references. Concerning general theory of difference equations and literature, we refer to

Lakshmikantham–Trigiante [367].

Our main goal concerns ﬁnite-dimensional reductions of nonlinear difference equations by

using the methodology and the experience that were gained when dealing with invariant sub-

spaces for differential operators. As in the continuous case, we formulate the Main Theorem,

which establishes a general representation of the

th-order nonlinear difference operators ad-

mitting a given ﬁnite-dimensional invariant subspace. This solves the backward problem of

invariant subspaces. Some aspects of the forward problem are also considered. We describe

subspaces for quadratic ﬁrst and some higher-order operators.

We next apply these results to moving mesh methods (MMMs) for nonlinear parabolic and

hyperbolic PDEs. It is shown that there exist special approximations of differential opera-

tors, which preserve ﬁnite-dimensional evolution on 2D subspaces. This guarantees that the

MMMs under consideration may preserve not only some of the group-invariant solutions (this

is the subject of classical Lie symmetry analysis), but also exact solutions on invariant sub-

spaces. For second-order parabolic PDEs, such exact solutions can be used for comparison,

for establishing various asymptotic results, and in stabilization analysis.

In the ﬁnal section, we present examples of exact solutions of lattice dynamical systems as

discrete counterparts of parabolic, compacton, and thin ﬁlm PDEs. In particular, we discuss

periodic breathers on anharmonic lattices and detect oscillatory, changing sign behavior for

higher-order parabolic models.

9.1 Backward problem of invariant subspaces for discrete operators

Let Z ={0, ±1, ±2, ...} be the set of integers, and let V be a linear space of real-

valued functions deﬁned on Z. Given a function u : Z → IR , the notation u

= u(i),

i ∈ Z, is used. Fix a natural p and consider a nonlinear difference operator F : V →

V given by

F[u]

≡ φ(u

, u

i+1

, ..., u

i+p

, i), i ∈ Z, (9.1)

where φ : IR

p+1

× Z → IR is a real-valued function. Therefore, (9.1) is a pth-order

difference operator. Given a function g ∈ V , we study the difference equation

F[u] = g on Z, (9.2)

430 Exact Solutions and Invariant Subspaces

whichisaninﬁnite system of nonlinear algebraic equations with solutions u ∈ V .

Using techniques of linear invariant subspaces, we will describe equations (9.2),

which can be reduced to ﬁnite systems of algebraic equations.

Usual ﬁnite-difference approximations of nonlinear ODEs and PDEs lead to sys-

tems such as (9.2). The order p of the difference operator then depends on the order

of the approximation of the differential operators. For instance, p = 2 corresponds

to a symmetric three-point approximation of the second derivative,

∼



− 2u

i+1

+ u

i+2



where h > 0 is a constant step of the discretization (u

denotes the value of u at the

ith point of the grid). We have p = 2 also for a three-pointsymmetricapproximation

of the ﬁrst derivative u

of the form



i+2

− u



The preliminaries of our invariant approach to discrete operators is the same as

in Section 2.1. In what follows, we are looking for solutions of (9.2) belonging to a

linear n-dimensional subspace W

⊂ V given by the span

= L{f

, f

, ..., f

} (9.3)

of linearly independent functions f

∈ V, j = 1, ..., n. Suppose that this set of

functions represents a fundamental set of solutions [367, Ch. 2] of a linear difference

equation of the nth-order with the linear operator L : V → V ,

L[u]

≡



k=0

(i)u

i+k

= 0, i ∈ Z, (9.4)

where a

∈ V for k = 0, 1, ..., n with a

(i) = 0anda

(i) = 0onZ.

We say that a function I (u

, u

i+1

, ..., u

i+n−1

, i) : IR

×Z → IR is a ﬁrst integral

of the linear equation (9.4) if, for any solution u of (9.4),

I (u

, u

i+1

, ..., u

i+n−1

, i) ≡ constant on Z.

The notation I [u]

and I [u] are also used. Linear ﬁrst integrals I [u]ofthenth-order

are deﬁned by linear functions,

I [u]

≡ I (u

, u

i+1

, ..., u

i+n−1

, i) =



n−1

k=0

(i)u

i+k

, i ∈ Z, (9.5)

where l

∈ V for k = 0, 1, ..., n with l

n−1

(i) = 0onZ. Suppose that the nth-order

equation (9.4) admits n linearly independent ﬁrst integrals I

[u], ..., I

[u].

As usual, subspace (9.3) is said to be invariant under the operator F if

F[W

] ⊆ W

We next state the Main Theorem on the backward problem (Problem II in our classi-

ﬁcation in Section 2.1) of invariant subspaces for nonlinear operators: given a linear

subspace W

, describe the set of all the nonlinear difference operators admitting W

Theorem 9.1 (“Main Theorem”)

Operator

(9.1)

with

p ≤ n − 1

admits the

subspace

(9.3)

iff

has the form

F[u] =



j =1

[u], ..., I

[u]) f

, (9.6)

where

: IR

→ IR

for

j = 1, ..., n

are arbitrary functions.

9 Invariant Subspaces for Discrete Operators 431

We assume that p ≤ n − 1, since, by (9.4) with a

(i) = 0, u

i+n

is expressed

in terms of u

i+n−1

, ..., u

. Clearly, for any operator (9.1) restricted to W

, all the

higher-order variables u

i+k

with k ≥ n are excluded by means of the subspace equa-

tion (9.4). The set of operators admitting a given subspace is a linear space. In a way

similar to that applied in the continuous differential case in Section 2.1, these oper-

ators can be considered as the generators of the higher-order symmetries of linear

discrete equations. Theorem 9.1 then gives a complete description of such symme-

tries.

The ﬁrst application of the theorem concerns problems (9.2) with F preserving a

subspace W

and g ∈ W

. In this case, every such equation on the subspace W

,i.e.,

on the set of functions

u =



i=1

, (9.7)

reduces to a ﬁnite-dimensional system of algebraic equations for coefﬁcients {C

Plugging (9.7) into (9.2) and (9.6) and using the linear properties of the functionals

[u]} in (9.5), we obtain the following

Corollary 9.2

Let

g =



k=1

∈ W

where

for

k = 1, ..., n

are constants. Given an operator

of the form

(9.6)

equation

(9.2)

, restricted to the subspace

of functions

(9.7)

, is equivalent to the

system of

algebraic equations for coefﬁcients

, ..., C

}

(

, ..., 

) = α

, j = 1, ..., n,

where

(9.8)



, ..., C

) =



m=1

[ f

], i = 1, ..., n. (9.9)

As a second application, consider an inﬁnite-dimensional DS

= F[u], (9.10)

with solutions u(x, t) : Z × IR → IR .IfF admits the subspace (9.3) and is repre-

sentedin the form (9.6), equation (9.10)possessessolutions(9.7) with C

, depending

on t and satisfying the DS

= A

(

, ..., 

), j = 1, ..., n.

A similar reduction exists in the completely discrete case where the continuous

derivative

is replaced by the corresponding discrete approximation. A similar

ﬁnite-dimensional reduction exists for higher-order equations

)u = F[u], (9.11)

where P is an arbitrary linear polynomial



e.g., P(

) =



. Equation (9.11) can

be considered as a discrete model for the nonlinear PDE

∂

∂t

)u = F

[u],

where F is a discrete approximation of a nonlinear differential operator F

432 Exact Solutions and Invariant Subspaces

9.1.1 Proof of Theorem 9.1

The proof is straightforward and has a natural extension to other types of nonlinear

integro-differential-differenceoperators.

Proof. The sufﬁciency is obvious. Let us prove the necessity. The invariance of the

subspace (9.3) means that, for any u ∈ W

F[u] =



j =1

(u) f

where the coefﬁcients

for j = 1, ..., n are constant for any given u. Note that

every u ∈ W

, being a solution of (9.4), can be uniquely represented by the values

of the linearly independentﬁrst integrals (9.5) {I

[u], k = 1, ..., n}. Hence, one can

write

(u) ≡ A

, ..., I

), which completes the proof.

9.1.2 First integrals and operators for n = 2

We begin with n = 2, i.e., consider second-order linear difference equations (9.4),

L[u]

≡ a

i+2

+ b

i+1

+ c

= 0. (9.12)

Introducing the standard formal scalar product in V ,

(u,v) =



(k∈Z)

the adjoint operator M[v] satisfying

(L[u],v)= (u, M[v]) for any u,v∈ V

gives the adjoint (conjugate) equation

M[v]

≡ c

+ b

i−1

+ a

i−2

= 0. (9.13)

Proposition 9.3

Any function of the form

I [u]

= a

i−1

i+1

− c

, (9.14)

where

is a solution of the adjoint equation

(9.13)

,istheﬁrst integral of

(9.12)

Proof. For functions (9.14), the condition

I [u]

= I [u]

i+1

in {u ∈ V : L[u] = 0}

reduces to u

i+1

M[v]

i+1

= 0, which completes the proof.

Example 9.4 Consider the linear equation

L[u]

≡ u

i+2

−

= 0 (9.15)

= 1, b

= 0, and c

=−

). The characteristic equation is λ

−

= 0, from

which we get the following subspace W

of solutions of (9.15):

= L



−i

,(−2)

−i



. (9.16)

The adjoint equation takes the form M[v]

≡−

+ v

i−2

= 0 and possesses two