Galaktionov V.A., Svirshchevskii S.R. Exact Solutions and Invariant Subspaces of Nonlinear Partial Differential Equations in Mechanics and Physics
Подождите немного. Документ загружается.


4 Korteweg-de Vries and Harry Dym Models 213
peakons are unlikely. Solutions of (4.191) may blow-up in finite time. Questions of
existence and uniqueness are
OPEN, as well as the regularizationby adding −εu
xxxx
.
Example 4.42 Returning to the original models, recall that, for non-tautological
PDEs with the annihilating operator (4.185), e.g.,
u
t
+ εu
xxt
= F
α
[u], with ε = 1,
looking for solutions (4.187) on W
2
yields the DS C
1
= C
2
= 0, so a stationary
peakon solution
u(x , t) = A sin|x |
can still be constructed. The fact that it is a proper weak solution should be checked
byusingthecorrespondingnon-localintegralequationor by parabolicε-regularization.
These are is
OPEN PROBLEMS for such PDEs.
4.6.2 Exponential subspaces: the FFCH equation
Example 4.43 (The FFCH equation) We begin with another famous completely
integrable shallow water model of a similar form,
u
t
− u
xxt
=−3uu
x
+ 2u
x
u
xx
+ uu
xxx
≡ F
3
[u]inIR × IR . (4.192)
This is the Fuchssteiner–Fokas–Camassa–Holm (FFCH) equation, which arises as
an asymptotic model that describes the wave dynamics at the free surface of fluids
under gravity. It is derived from Euler equations for inviscid fluids under the long
wave asymptotics of shallow water behavior (where the function u is the height of
the water above a flat bottom); see Remarks.
We comment on some evolution aspects of such PDEs restricted to invariant sub-
spaces of the operator F
3
, and consider a three-parameter family of such equations
u
t
− u
xxt
= αuu
x
+ βu
x
u
xx
+ γ uu
xxx
≡ F[u]inIR × IR . (4.193)
Proposition 4.44
Equation
(4.193)
is tautological on
W
2
= L{e
x
, e
−x
}
if
α + β + γ = 0. (4.194)
The annihilating operator of W
2
is now L = I −
d
2
dx
2
,and
F
3
[u] = (αu
x
+ γ u
xxx
)L[u] +(α + β)u
xx
(L[u])
x
+ (α + β + γ)u
xx
u
xxx
.
Hence, any function
u(x , t) = C
1
(t)e
x
+ C
2
(t)e
−x
(4.195)
is a solution of (4.193) for arbitrary smooth coefficients C
1,2
(t), i.e., for initial data
u
0
∈ W
2
, the Cauchy problem admits an infinite-dimensionalset of solutions (4.195)
satisfying u(x, 0) = u
0
(x ). These solutions are unbounded in x with exponential
growth as x →±∞, so this non-uniqueness happens in the class of exponentially
growing functions.
Another feature of equation (4.193), (4.194) is that the subspace W
2
makes it pos-
sible to construct, by gluing two opposite exponents, Lipschitz continuous solutions
© 2007 by Taylor & Francis Group, LLC
214 Exact Solutions and Invariant Subspaces
admitting the discontinuousderivativesu
x
, and havingexponentialdecay as x →∞.
Such an elementary solitary wave, called peakon for the FFCH equation, is
u(x , t) = λe
−|x−λt |
, (4.196)
where λ ∈ IR is the traveling wave speed. The N-solitons, which are multipeakons
discovered for (4.192) for any N ≥ 1 in [94], adopt the form
u(x , t) =
(1≤i≤N)
p
i
(t)e
−|x−q
i
(t)|
(4.197)
with 2N functions {q
i
(t), p
i
(t)}, which are the canonical coordinates {q
i
} and mo-
ments {p
i
}, satisfying a DS to be presented. Function (4.197) has a cusp (a peak)
at each x = q
i
(t),andu(·, t) ∈ W
2
on any x-interval of C
1
-regularity. As usual,
in nonlinear PDE theory, dealing with odd-order equations admitting shock wave
or weaker singularities, special Rankine-Hugoniot-type and entropy conditions (see
mentioning Oleinik’s E-condition for scalar conservation laws in Remarks) should
be specified to detect proper unique (weak) solutions. This is achieved by writing the
equation in the conservative integral form
u
t
=−uu
x
−
ω ∗
u
2
+
1
2
(u
x
)
2
x
, (4.198)
where ω(s) =
1
2
e
−|s|
> 0inIR is the kernel of the linear operator
I −
d
2
dx
2
−1
in
L
2
(IR ). The integral representation (4.198) of the original third-order PDE correctly
describes the propagation of weak shocks (peaks) and makes it possible to establish
the global existence of a unique weak solution; see Remarks.
In particular, using the integro-differential equation (4.198) for the N -soliton so-
lutions (4.197) yields that these are governed by the Hamiltonian ODEs
˙q
i
=
∂ H
A
∂p
i
,
˙p
i
=−
∂ H
A
∂q
i
,
(4.199)
with the Hamiltonian
H
A
( p
i
, q
i
) =
1
2
N
i, j =1
p
i
p
j
e
−|q
i
−q
j
|
. (4.200)
This makes it possible to describe general evolution properties of such Lipschitz
continuous multipeakons [94] which turn out to be generic in view of their orbital
stability (see key references in the Remarks).
Similar results on the invariant subspace W
2
apply to the generalized FFCH equa-
tion [211]
u
t
− u
xxt
= αu
xxx
− β(3uu
x
− 2u
x
u
xx
− uu
xxx
)
+γ
(u − u
xx
)(u
2
− (u
x
)
2
)
x
.
The set of tautological PDEs on W
2
is wide. Fixing an arbitrary linear differential
operator M with constant coefficients and a nonlinear operator A(u, u
x
, ...), consider
u
t
− u
xxt
= M[u] − β(3uu
x
− 2u
x
u
xx
− uu
xxx
) + γ {L[u]A(u, u
x
, ...)}
x
.
For higher-order PDEs, a suitable definition of proper weak solutions with weak
shocks (constructed either via suitable integral equations inheriting correct Rankine–
© 2007 by Taylor & Francis Group, LLC

4 Korteweg-de Vries and Harry Dym Models 215
Hugoniot and entropy conditions, or by regular parabolic approximations) becomes
much more delicate and generates many mathematical
OPEN PROBLEMS.
4.6.3 Other related models
Example 4.45 (The PBBM equation) Consider the famous Peregrine–Benjamin–
Bona–Mahoney (PBBM) equation [461, 38]
u
t
− u
xxt
= uu
x
in IR × IR , (4.201)
also known as the regularized long-wave equation. This is derived from the KdV
equation written in the form
u
t
+ u
xxx
= uu
x
by formally justifying that u
t
+ u
x
≈ 0, and hence, replacing u
xxx
by −u
xxt
. Equa-
tion (4.201) also admits the integral representation
u
t
=
1
2
ω ∗ u
2
x
ω(s) =
1
2
e
−|s|
, (4.202)
where the derivation imposes the continuity condition of u(x, t).Sinceω
(s) is dis-
continuous at s = 0, (4.202) may admit solutions that are only Lipschitz continuous
in x , similar to peakons. The regularized PBBM equation
u
t
− u
xxt
= uu
x
+ ε(I − D
2
x
)u
xx
(ε > 0), (4.203)
reduces to a uniformly parabolic equation with non-local perturbation,
u
t
= εu
xx
+
1
2
ω ∗ u
2
x
, (4.204)
and admits smooth classical solutions.It would be interestingto describe the passage
to the limit ε → 0 in both PDEs (4.203) and (4.204); these are
OPEN PROBLEMS.
Example 4.46 The non-tautologicalPDE on W
2
,
u
t
− εu
xxt
= F
3
[u], with ε = 1,
with the general operator (4.193), (4.194) still admits a stationary 1-peakon
u(x , t) = A e
−|x|
,
with unknown evolution properties; an
OPEN PROBLEM.
Example 4.47 (Higher-order tautological PDEs) Consider, for instance,
u
t
− D
6
x
u
t
= F[u] ≡
(i, j )
a
i, j
D
i
x
uD
j
x
u, (4.205)
with the quadratic operator and polynomial P(X, Y ) defined as in (1.112), e.g.,
F[u] = αuD
7
x
u + βu
x
D
6
x
u + γ u
xx
D
5
x
u + ... .
The subspace W
2
= L{e
x
, e
−x
} is tautological for the operator in (4.205) iff
P(1, 1) = P(−1, −1) = P(1, −1) = 0.
Therefore, one can define the peakon (4.196) and corresponding multipeakon solu-
tions. Determining the kernel of (I − D
6
x
)
−1
by the inverse Fourier transform,
ω(s) = F
−1
1
1+ξ
6
≡
1
π
∞
0
cosξs dξ
1+ξ
6
,
© 2007 by Taylor & Francis Group, LLC
216 Exact Solutions and Invariant Subspaces
u
c
(x , t)
t
1
t
2
= 0
t
3
t
1
< 0 < t
3
x
λ
λ
π
2
0
−
π
2
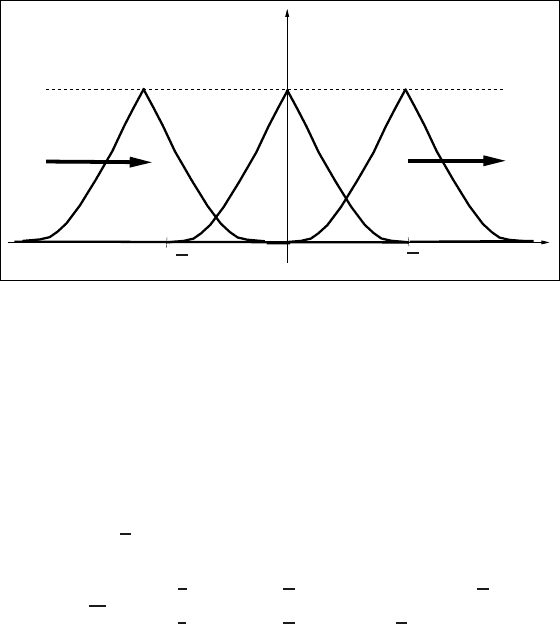
Figure 4.20 Compacton-peakon solution (4.206).
which is an exponentially decaying oscillatory function, we write down (4.205) for
solutions u(·, t) ∈ H
6
(IR ) in the integral form
u
t
= ω ∗ F[u]fort > 0.
The evolutionconsistency of peakonsolutionswill depend on special divergentprop-
erties of the operator F and leads to many difficult
OPEN PROBLEMS.
Example 4.48 (Compacton-peakon) We return to the PDE (4.182) and perform
the shifting in x by ±
π
2
of the increasing and decreasing branches of the compacton
solution(4.184) to create a formal compacton-peakon solution
u
c
(x , t) =
2λ
ρ
cos
2
1
2
(x − λt) +
π
4
for λt ≤ x ≤ λt +
π
2
,
1
2
(x −λt) −
π
4
for λt −
π
2
≤ x <λt,
(4.206)
which is shown in Figure 4.20. The evolution consistency is
OPEN. It would be in-
teresting to create other, possibly higher-order, models with guaranteed patterns like
that in the CP, or FBP settings.
Example 4.49 (Nonlinear dispersion, finite propagation, and oscillatory solu-
tions) We now check a possible character of finite interfaces for the fifth-order PDE.
As a formal extension of our previous study of solutions of changing sign, consider
the following nonlinear dispersion PDE:
u
t
− u
xxt
=
|u|
n
u
xxxxxxx
(n > 0). (4.207)
Similar to the compacton analysis, we describe finite interfaces in the CP by using
the TWs u(x, t) = f (x −λt),where f satisfies
−λf
+ λ f
=
| f |
n
f
(7)
.
Keeping the leading terms near the interface at y = 0, and integrating three times,
yields the fourth-order ODE
| f |
n
f
(4)
= λf for y > 0, f (0) = 0.
The oscillatory behavior close to the interface for λ<0 was studied before (cf.
© 2007 by Taylor & Francis Group, LLC

4 Korteweg-de Vries and Harry Dym Models 217
(4.91)) with the oscillatory component satisfying (4.93). Since the exponent there
was γ =
4
n
→+∞as n → 0
+
,such f (y) can be arbitrarily smooth at the interface
at y = 0forsmalln > 0. We expect that such behavior close to interfaces plays a
role for more general solutions of the PDE (4.207).
Example 4.50 (Hunter–Saxton equation) Neglecting the first term on the right-
hand side of (4.192) gives
u
t
− u
xxt
= 2u
x
u
xx
+ uu
xxx
≡ F
3
[u]inIR × IR . (4.208)
This is a modification of the Hunter–Saxton equation
u
xxt
+ 2u
x
u
xx
+ uu
xxx
= 0, (4.209)
which was proposed as a model for the asymptotic behavior of neumatic fluids crys-
tals [294] and belongs to the Dym hierarchy of integrable PDEs, [358]. Equation
(4.209) is tautological on the subspace of linear functions
W
2
= L{1, x }.
Instead of (4.197), the N-soliton solution takes the form
u(x , t) =
(1≤i≤N)
p
i
(t)|x −q
i
(t)|, with
(1≤i≤N)
p
i
(t) = 0, (4.210)
where the constraint is imposed to guarantee that the function (4.210) is uniformly
bounded. The DS is (4.199) with the Hamiltonian
H
A
( p
i
, q
i
) =
1
2
(i, j )
p
i
p
j
|q
i
− q
j
|.
A unique solution is obtained by using an integro-differential equation imposing a
correct propagation mechanism of weak singularities; see Remarks.
Concerning more general invariant settings, let us point out that the quadratic op-
erator F
3
in (4.208) is now associated with the subspace W
4
= L{1, x , x
2
, x
3
} of
cubic polynomials. This gives the exact solutions
u(x , t) = C
1
(t) + C
2
(t)x + C
3
(t)x
2
+ C
4
(t)x
3
,
C
1
= 4C
2
C
3
+ 6C
1
C
4
+ 84C
3
C
4
,
C
2
= 18C
2
C
4
+ 8C
2
3
+ 252C
2
4
,
C
3
= 42C
3
C
4
,
C
4
= 42C
2
4
.
It follows from the last ODE that C
4
(t) =
1
42
(T − t)
−1
blows up. This determines
the same blow-up rate as t → T
−
of all the other coefficients. The multipeakons on
W
4
can be taken, e.g., in the form of
u(x , t) =
(1≤i≤N)
p
i
|x − q
i
|
3
+r
i
|x − q
i
|
2
+ s
i
|x − q
i
|
,
with necessary constraints for functions {p
i
, q
i
, r
i
, s
i
} that guarantee the uniform
boundednessof these solutions. Existence of multipeakons as propersolutions of the
Cauchy problem for (4.208) is an
OPEN PROBLEM.
Example 4.51 (Schwarzian KdV equation) Some special annihilating properties
© 2007 by Taylor & Francis Group, LLC
218 Exact Solutions and Invariant Subspaces
are exhibited by the operator of the (2+1)-dimensional integrable generalization of
the Schwarzian KdV (SKdV) equation [557]
u
t
= F
∗
[u] ≡−
1
4
u
xxy
+
u
x
u
xy
2u
+
u
xx
u
y
4u
−
(u
x
)
2
u
y
2u
2
+
u
x
8
D
−1
x
(u
x
)
2
u
2
y
,
(4.211)
where D
−1
x
f denotes
f dx . F
∗
annihilates the 1D subspace L{f (x)} for arbitrary
C
2
-functions f ,i.e.,F
∗
[Cf] = 0 for any constant C. This gives a wide family of
separable stationary solutions u(x, y, t) = g(y) f (x ) for any C
1
-function g, includ-
ing localized compactons, see [484]; justification is
OPEN.
Example 4.52 (Green–Naghdi equations) Continuinga reviewof waterwavemod-
els with invariant subspaces, consider the Green–Naghdi (GN) equations
η
t
+ (uη)
x
= 0,
u
t
+ uu
x
+ gη
x
=
1
3η
η
2
((ηu
x
)
t
+ u(ηu
x
)
x
)
x
,
(4.212)
which determine an approximate system of the full water problem, modeling sur-
face wave propagation on an inviscid and incompressible gravity flow, [262]. Here
u is the mean horizontal velocity, η is the surface disturbance, and g is the gravity
acceleration. Invariant solutions of (4.212) are discussed in [21]. The last operator
in (4.212) is quartic, so higher-dimensional subspaces are unlikely. It is curious that
nontrivial dynamics for the GN equations already occur on the elementary subspace
of linear functions W
2
= L{1, x }, where the solutions are
η(x, t) = C
1
(t) + C
2
(t)x , u(x, t) = D
1
(t) + D
2
(t)x ,
C
1
=−C
1
D
2
− C
2
D
1
,
C
2
=−2C
2
D
2
,
D
1
=−D
1
D
2
− gC
2
−
2
1−C
2
2
C
1
C
2
D
2
2
,
D
2
=−
1+C
2
2
1−C
2
2
D
2
2
.
On W
2
, peakon solutions,
η(x, t) = p(t)|x − q(t)| and u(x, t) = r(t)|x −s(t)|,
can be formally defined with unclear evolution properties; an
OPEN PROBLEM.
Example 4.53 (The CDDD equation) Longitudinal strain waves in isotropic cylin-
drical compressibleelastic rods embedded in a viscoelastic medium can bedescribed
by higher-ordermodels containing nonlinear dispersive operators. Consider a gener-
alization of the combined dissipative double-dispersive (CDDD) equation (see, e.g.,
[469])
u
tt
= αu
xxxx
+ βu
xxtt
+ γ(u
2
)
xxxxt
+ δ(u
2
)
xxt
+ ε(u
2
)
t
. (4.213)
The quadratic operator F[u] in this PDE preserves W
3
= L{1, cosx, sin x} of 2π-
periodic solutions iff
16γ − 4δ + ε = 0,
and (4.213) on W
3
is then a sixth-order DS possessing blow-up solutions.
© 2007 by Taylor & Francis Group, LLC
4 Korteweg-de Vries and Harry Dym Models 219
0 2 4 6 8 10
0.01
0.008
0.006
0.004
0.002
0
0.002
0.004
0.006
0.008
0.01
s
Φ(s)
(a) n = 1
0 2 4 6 8 10
0.01
0.008
0.006
0.004
0.002
0
0.002
0.004
0.006
0.008
0.01
s
Φ(s)
(b) n = 10
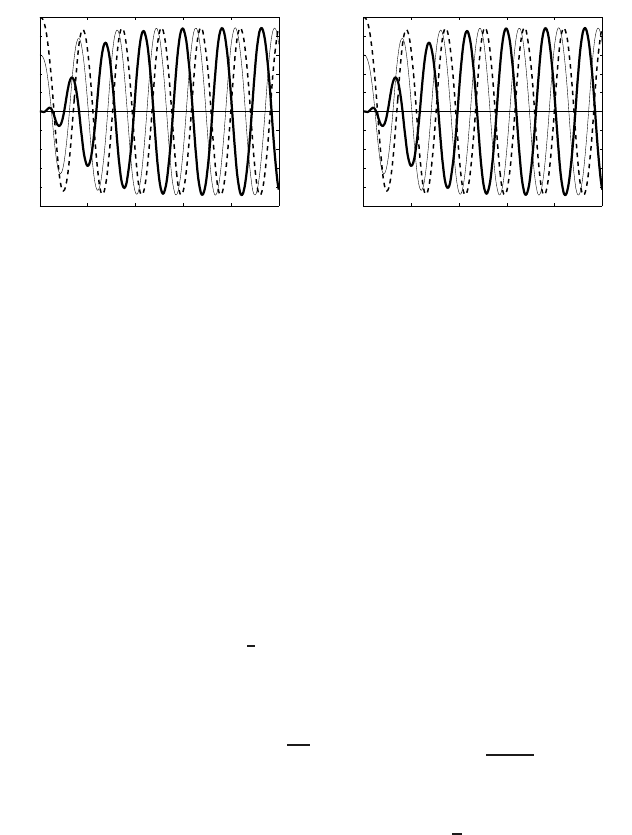
Figure 4.21 Stability of periodic solutions of (4.215) with λ =−1.
Example 4.54 (On a model with finite interfaces)The phenomenon of finite prop-
agation in such models demands a proper monotone extension of nonlinearity u
2
for
negative u < 0, as in the following signed PDE (here, β = δ = ε = 0andγ =−1
for well-posedness):
u
tt
=−
|u|
n
u
xxxxt
(n > 0).
TW solutions u(x, t) = f (x − λt) give the ODE
λ
2
f
= λ(| f |
n
f )
(5)
.
Integrating twice yields the third-order problem
|f |
n
f
− λ f = 0fory > 0, f (0) = 0,
which appeared for TFEs; see Section 3.7. Namely, the behavior near interface at
y = 0 needs introduction of the oscillatory component ϕ by
f (y) = y
3
n
ϕ(s), where s = ln y, (4.214)
where =|ϕ|
n
ϕ solves the third-order ODE
+ 3(µ − 1)
+ (3µ
2
− 6µ + 2)
+µ(µ − 1)(µ − 2) − λ
−
n
n+1
= 0, with µ =
3(n+1)
n
.
(4.215)
Transformation (4.214) shows that the regularity at the interface y = 0 improves as
n → 0
+
. TheODE for (s) with λ<0 is similarto that givenin (3.166) with known
oscillatory properties (recall the difference between γ =
3
n
therein, and µ above).
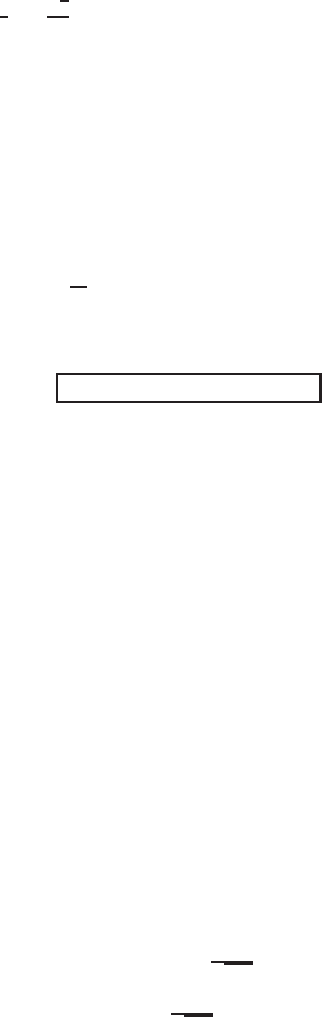
Therefore, solutions of (4.215), with λ =−1, are oscillatory for all n ∈ (0, ∞);see
Figure 4.21.
For λ>0, numerical unstable periodic solutions of (4.215) were not detected. To
confirm this negativeconclusion,using the idea of continuity(homotopy)at n = 0
+
,
we take the linear equation
u
tt
=−u
xxxxt
,
which is the well-posed bi-harmonic PDE in terms of u
t
(see (3.2)). For TWs, the
ODE is
f
− λ f = 0 ⇒ f (y) = e
µy
, where µ
3
= λ.
As above, the solutions are oscillatory at the left-hand interface y =−∞for λ =−1
© 2007 by Taylor & Francis Group, LLC
220 Exact Solutions and Invariant Subspaces
roots are µ
±
=
1
2
± i
√
3
2
and are not for λ = 1 (the only root is µ
0
= 1). The
fundamental solution is always oscillatory, since λ<0 for it by symmetry.
4.6.4 Quasilinear third-order remarkable operator
Recall the definition of the remarkable second-order operator
F
rem
[u] = uu
xx
− (u
x
)
2
,
which exhibits special invariant properties and has been used in the study of the
RPJ equation in Example 1.36. In order to obtain a similar third-order remarkable
operator, we take the derivative of F
rem
,
F[u] =
d
dx
uu
xx
− (u
x
)
2
= uu
xxx
− u
x
u
xx
,
which occurred in several models studied before.
Example 4.55 The quasilinear third-order PDE
u
t
= uu
xxx
− u
x
u
xx
+ µu + ν
possesses solutions
u(x , t) = C
1
(t) + C
2
(t)x +C
3
(t) cos(γ (t)x ) + C
4
(t) sin(γ (t)x), (4.216)
C
1
= µC
1
+ ν, C
2
= µC
2
,
C
3
=−γ
3
C
1
C
4
+ γ
2
C
2
C
3
+ µC
3
,
C
4
= γ
3
C
1
C
3
+ γ
2
C
2
C
4
+ µC
4
,
γ
=−γ
3
C
2
.
4.7 Subspaces, singularities, and oscillatory solutions of Harry Dym-type
equations
4.7.1 PDEs and invariant subspaces
Consider the following third-order PDE:
v
t
= v
n
v
xxx
, (4.217)
where n ∈ IR is a parameter. This equation is posed for nonnegative solutions and,
as usual, v
n
will be replaced by |v|
n
for oscillatory solutions of changing sign. For
n = 3, (4.217) gives the Harry Dym (HD) equation
v
t
= v
3
v
xxx
,
which is one of the most exotic soliton equations. It is associated with the classical
string problem and is linearizable by the inverse spectral transform method; see de-
tails and references in [128]. Setting v =−
1
√
1+q
yields another form of the Harry
Dym equation
q
t
=
2
√
1+q
xxx
,
which, on the complex plane, is relevant to several physical problems, such as the
Hele–Shaw problem,theSaffmane–Taylor problem of the motion of the interface of
© 2007 by Taylor & Francis Group, LLC
4 Korteweg-de Vries and Harry Dym Models 221
two fluids with different viscosities, and the chiral dynamics of closed curves on the
plane.
Let us find those PDEs (4.217) that admit exact solutions on polynomial sub-
spaces. The case n = 1 is excluded, where the quadratic operator
F[v] = vv
xxx
preserves the obvious 4D subspace
W
4
= L{1, x , x
2
, x
3
}.
This is suitable for PDEs, such as
v
t
= vv
xxx
+ βv
x
v
xx
+ µv + ν,
which, on W
4
, reduces to a 4D DS that can describe, e.g., finite-time extinction and
interface propagation in various FBPs.
Looking for other cases, we introduce the “pressure” for the PDE (4.217),
v = u
µ
, with exponent µ =
2
n
.
This yields the following equation with a homogeneouscubic operator:
u
t
= F[u] ≡ u
2
u
xxx
+ 3(µ − 1)uu
x
u
xx
+ (µ − 1)(µ − 2)(u
x
)
3
. (4.218)
The basic subspace for F is trivial, W
2
= L{1, x}. Substituting u = x
2
yields that F
in (4.218) preserves the extended 3D subspace
W
3
= L{1, x , x
2
}, if 12(µ − 1) + 8(µ − 1)(µ − 2) = 0, (4.219)
i.e., for µ = 1(n = 2, the trivial case: u = v and v
xxx
= 0onW
3
)andforµ =
1
2
(n = 4) that gives some applications and extensions.
Example 4.56 (Extinction and interfaces) Consider the Harry Dym-type equation
with absorption
v
t
= v
4
v
xxx
−
1
v
(v ≥ 0). (4.220)
The absorption term is unbounded and singular at v → 0
+
, so that the first question
of PDE theory is to check if (4.220) can admit any nontrivial compactly supported
solution, i.e., a solution v(x, t) ≡ 0. This isnot an easy question,evenfor the second-
order PME with absorption
v
t
= (v
n
)
xx
−
1
v
p
, with n > 0, (4.221)
though the criterion for the existence is known: p < n. It is proved that, for p ≥ n,
any FBP with v = 0 on the interface has the trivial unique proper solution v(x , t) =
limv
ε
(x , t) ≡ 0(i.e.,v is the limit of a family {v
ε
} of smooth global solutions of
the regularizednon-singularequations), regardlessof any nontrivial initial or regular
boundary data. In particular, the heat equation with absorption
v
t
= v
xx
−
1
v
belongs to the nonexistence range, so, for compactly supported initial data v
0
≥ 0,
the unique maximal solution is trivial, v(x , t) ≡ 0. Hence, the same is true for all
© 2007 by Taylor & Francis Group, LLC
222 Exact Solutions and Invariant Subspaces
other non-maximal solutions of any FBPs. For parabolic PDEs, such as (4.221), the
existence-nonexistence criterion is proved by the Sturmian intersection comparison
with TWs, [226, Ch. 7-9]. For higher-order equations, these are
OPEN PROBLEMS.
Let us show that, regardless of such a singular absorption term −
1
v
, equation
(4.220) admits nontrivial exact solutions and we will pose the corresponding FBP.
Setting v =
√
u
µ =
1
2
yields the PDE
u
t
= u
2
u
xxx
−
3
2
uu
x
u
xx
+
3
4
(u
x
)
3
− 2 (4.222)
that possesses solutions u ∈ W
3
,
u(x , t) = v
2
(x , t) = C
1
(t) + C
2
(t)x + C
3
(t)x
2
, (4.223)
C
1
=−3C
1
C
2
C
3
+
3
4
C
3
2
− 2,
C
2
=−6C
1
C
2
3
+
3
2
C
2
2
C
3
,
C
3
= 0.
Setting C
3
(t) ≡ 1 gives the opportunity to study the quenching phenomenon, where
the classical analytic strictly positive solution vanishes as t → T
−
at some x
0
∈ IR .
Vice versa, C
3
(t) ≡−1 describes the single point extinction, when the solution
vanishes identically as t → T
−
.
We consider the extinction phenomenon with C
3
=−1 using typical asymptotic
arguments from thin film analysis (Section 3.2 and 3.10). We need an FBP setting
where the quenching description is similar. We do not solve the DS in (4.223) ex-
plicitly and take orbits such that C
1
(t) = 2(T − t) + ... and C
2
(t) = b(T − t) + ...
(b ∈ IR )ast → T
−
. This gives the pattern
u(x , t) =
2(T − t) + b(T − t)x − x
2
+
+ ... ≡ e
−τ
2 + be
−
τ
2
ξ −ξ
2
+
+ ... ,
where ξ = x/
√
T − t and τ =−ln(T − t) are the standard blow-up rescaled
variables, as in (3.39). In view of (4.223), this shows that, for the original equation
(4.220), the rescaled solution satisfies
v(x , t) =
√
T − t w(ξ, τ) → g(ξ) =
(2 − ξ
2
)
+
as τ →∞, (4.224)
where w solves a singular perturbed PDE of the form
w
τ
=−
1
2
w
ξ
ξ +
1
2
w −
1
w
+ e
−
3
2
τ
w
4
w
ξξξ
for τ 1. (4.225)
Then g(ξ ) is a stationary solution of the limit equation
−
1
2
g
ξ +
1
2
g −
1
g
= 0,
which is (4.225) at τ =+∞. A rigorouspassage to the limit τ →+∞in (4.225) to
proveconvergence(4.224)for a class of the FBP solutions with extinctionat t = T is
adifficult
OPEN PROBLEM. Noticethat (4.220)admits similarity solutions (existence
or nonexistence is
OPEN)
v
s
(x , t) =
√
T − tg(z), z =
x
T −t
⇒ g
4
g
− g
z +
1
2
z −
1
g
= 0.
As usual, using the positive part (·)
+
in the solutions means an FBP. The dynamic
© 2007 by Taylor & Francis Group, LLC
