Ferrarese G. (editor) Wave Propagation

Подождите немного. Документ загружается.

equations

given

Bleustein

and Green

The

equations

morner.tum

and

continuity

278

(later

modi f i ed by Green and Naghdi

[4J).

This

theory

allows

for

additional

dipolar

stress

well

the

normal one .

contrast

with

Newtonian

theory

the

constitutive

varia

bles

include

temperature.

velocity

and

density

gradients.

This

one

sense

generalization

the

Maxwellian

fluid

Truesdell

(see

[2].

§125 and

the

references

therein)

that

dipolar

stress

included

from

the

outset.

although

Truesdell's

Maxwellian

fluid

involves

constitutive

theory

which

includes

density.

temperature

and

velocity

gradients

arbitrary

orders.

pay

particular

attention

the

compressible

dipolar

fluid

since

Truesdell

and

lIoll

[2J

point

out.

the

Maxwel

theory.

• ••

"is

set

such a

~Jay

emphasize

effects

compressibility"

•

The

dipolar

fluid

[3]

are

now

reviewed.

are

(1.1)

0 •

••

pf.,

J1-~J

where

standard

notation

(see

ego [3)

employed

throughout.

However,

the

energy

equation

takes

form

(1.3)

PI' -

peA

70s

i- TS) - q • . +

r.(")kA~

1-~1-

J1-

1-J

J :;)1.

where

Akj'

=1J

••

and

••

and

i j k

are

a symmetrr-Lc

tensor

and

~J1-

J1-

the

dipolar

stress

tensor,

respectively,

the

equat Io-.

(1.4)

'ij

°ij

r.kij~k

+ p(F

i j

- r

i j

)·

Here F

i j

are

components

dipolar

hody

force

and r

i j

th e

dipolar

inertia

which

has

form

(see

Green and Naghdi

[4])

(1.5)

j i

d2(Vi~j

1Ji~k1Jk~j

1Ji~k1Jj~k

1Jk~j1Jk~i)'

being

the

inertia

coefficient.

Furthermore,

the

entropy

inequality

takes

the

form

(l.6)

q.T

peA·

+ S,,-) -

.:!:.-t..:!:..

+ d +

A > 0

'ji

(ij)k

i - •

279

Bleustein

and Green [3]

develop

the

equations

for

compressible,

viscous

dipolar

fluid.

However,

are

primarily

interested

wave mot

ion

shall

derive

the

constitutive

equations

for

compressible

inviscid

dipolar

fluid,

following

the

procedure

given

Bleustein

and Green.

Suppose

then,

that

A, S,

qi'

i j

and

L(ij)k

depend on

the

variables

(1.7)

Using

(3.4)

Bleustein

and Green

[3],

the

entropy

inequality

(1.6)

may be

written

(1.8)

_.-

p--T

. .

••

,tJ

t p

q.T

tT,t

T.-do.

t L(

••

)kA

Y,.,.

1-J

, "-<It

and

the

constitutive

relations

may

then

reduce

with

the

standard

Coleman-Noll

procedure

whereby t he

terms

appearing

linearly

(1.8)

may

chosen

arbitrari1y,

the

momentum

and

energy

equations

being

balanced

suitable

I i

thu

the

coefficients

these

terms

are

zero

. Hence,

(l.9

)

A =A( p , p

T).

, 1-

Since,

[3],

A must be a

hemitropic

funct

ion

i t s

argu-

ments,

(1.9)2

reduces

.10)

where

(1.11)

A =

A(p,v,

T),

280

What remains

the

entropy

inequality

may be

written

(cf

, [3], (3.llf»

(1.12

)

]

(\10

••

+ P .p

d • .

0\1 1,J •

'I-.J

'l-J

q.T

•

~>O

T - •

Like-

This

Thus,

the

term

multiplying

A • 'k must be

zero.

1,J

may

argue

that

the

term

multiplying

d • •

zero.

1,J

the

constitutive

relations

and so we

may

inequality.

Next,

since

and r

are

our

disposal

(1.1)

and

(1.3)

may choose a motion

such

that

particular

~,t

only

the

second

term

(1.12)

non-zero.

Moreover, A"

appears

only

linearly

'1-,1

select

the

motion

such a way

invalidate

the

leads

wise,

0.13)

.14)

'O'k

+ p 'O

'k)'

0\1

.1,

1, '.

For

dipolar

elastic

material

find

easier

with

wave

motion problems

use

the

equations

referred

reference

configuration,

here

taken

be a homogeneous

one.

form

the

equations

are

([lJ,

p.342)

for

the

isothermal

case,

(1.15)

(1.16

)

IT(BA).· = Po

---

•

(1.17)

• .

ax./

where U

the

Lnternal

energy,

•

= 1, aXA' lT

represents

stress

tensor,

the

dipolar

stress

and P

given

(see

Green and Naghdi

[4,5])

(1.18)

= F

• f

281

with

components

dipolar

body

force

and r

the

dipolar

intertia

defined

([4],

ego

(11»

(1.19

)

being

constant

tensor.

these

equations

the

internal

energy

function

A and

AB'

.e.

'Z.~ 'Z.~

(1.20)

u =U(X .

x ,

AB).

'Z.~ 'Z.~

should

remark

this

stage

that

the

constitutive

functions

(1.14)

and

(1.16)

given

only

for

the

symm~tric

part

with

respect

the

first

two

indices

n~i

i j k

•

This

does

not

make any

difference

the

equations

motion,

the

skew

symmetric

part

left

unspecified.

However.

important

considering

boundary

conditions

discussed

[1,3,5]. The

skew-symmetric

part

also

plays

important

role

three-dimen-

sional

wave motion

studies

(see

[6]>,

but

this

article

shall

not

consider

these

matters

an~

only

investigate

the

novel

effects

the

theory

present

one-dimensional

analysis.

pertinent

draw

attention

paper

Mi ndl i n [7]

who

discusses

other

ways

describing

elastic

materials

allowing

for

micro-structure

effects

and

also

includes

detailed

account

linear

wave motion and a

critical

comparison

with

results

from

discrete-type

lattice

theories.

2. Dipolar stress

waves

Restrict

attention

now

the

one-dimensional

form

equations

(1.1).

(1.2).

(1.4).

(1.5).

(1.9).

(1.13)

and

(1.14)

with

••

=0 and suppose P. v,

the

ensit

y and

velocity.

now

depend

'Z-

'Z-J

only

on x and

The

one-dimensional

form

the

stress

tensor.

dipolar

stress

tensor

and

dipolar

inertia

are

denoted

E. r

and

satisfy

the

relation

a = T - Ex ..

prJ

282

where

the

notation

JjJx

signifies

~~,

al!J

1JJ

;:;.

The

constit-

equa

tions

become

<2.2)

= - p -

2p-q,

(2.3)

= -

(2.11 )

( iJ

_ v

)

(2.5)

ere

t he pr es s

( 2. 6 )

( 2

.7)

A =

A(p,px)'

p and

dipolar

= 2 aA

p p ap'

q =

2! .

pressu

are

defi

ned by

The cont i nui t y and momentum

equations

are

( 2. 8)

<2. 9 )

Suppose

(2.8)

and

(2.9)

hold

and p and v

are

contin-

uous l y d

ifferentia

ble

nction

x and t on

P2.

Suppose,

moreove r ,

at t her e

sur

ace

}

for

each

propa-

ating

the

:::-dire

ion

with

eed

un(~

0 ) . The quant

ies

v, iJ

' v

Px' P

assu

med co

i nuous f un

i ons

x , t

on (R , 1:) x R and hav e at mos t jump dis cont

acro

1:.

The jump

a qua

ntit

y P i s d

ined

(

10)

ere

super

scr i pt + s

ign

not

t he

gio

n ahead of t he wave,

a nega

tive

si gn denot i ng t he r

egion

beh

d t he wa

ve.

A s

face

as d

efined

ahove

be a o

ne-

dimens

nal

polar

stress

wave and

the

esponding

wave am

itude

fined

(

2.11

)

<2.1

2 )

B = [

c = rvl-

.:::

283

obtain

the

first

discontinuity

equation,

(2.8)

differ-

entiated

with

respect

x and

limits

either

side

are

taken

find

(2.13)

further

analyse

this

equation

observe

that

for

function

can

be shown

(see

Truesdell

and Toupin

[8])

(2.14)

where

~/~t

the

displv~ement

derivative

defined

(2.15)

and

the

relative

wavespeed

(2.16)

With

the

aid

(2.14),

(2.13)

may

thus

rearranged

(2.17)

+ pC =O.

Until

this

point

our

analysis

has

proceeded

for

third

order

wave

for

classical

perfect

fluid;

now

things

change.

For,

apply

the

jump

conditions

(2.9)

and

use

(2.14)

find

the

resulting

equation

already

involves

the

displacement

derivative

Thus,

necessary

adopt

another

approach

find

suitable

equation

employ in

conjunction

with

(2.17).

instead

adopt

the

approach

which

us ed

with

shock

waves

classical

perfect

fluid

and

use

the

discontinuous

equation

balance

form

(2.9)

(see

Green and Naghdi [9]).

For

this

reason

refer

the

third

order

waves s t udi ed

here

dipolar

stress

waves.

The

appropriate

equation

balance

(2.18)

- U

[w]

= [a].

course,

for

the

waves

considered

here,

the

left

hand

side

this

equation

zero,

and so

(2.19)

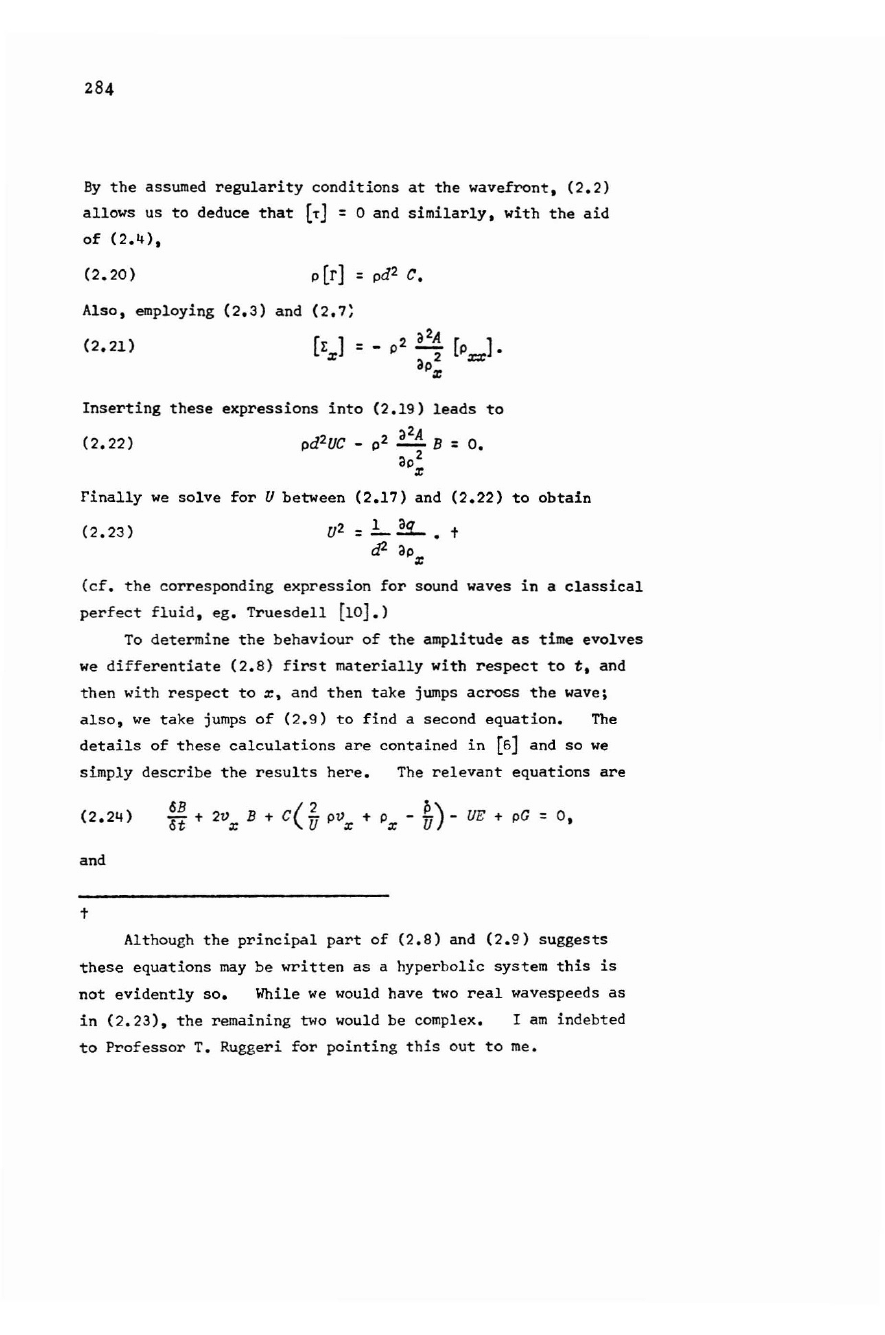
284

the

assumed

regularity

conditions

the

wavefront,

(2.2)

allows

deduce

that

[T]

=0 and

similarly,

with

the

aid

(2.4),

(2.20

)

Also,

employing

(2.3)

and

(2.7:

(2.21)

Inserting

these

expressions

into

(2.19)

leads

p2 a

A B = O.

3P;

finally

solve

for

between

(2.17)

and

(2.22)

obtain

(2.23)

U2 =

.!...

cJ.2

:x:

(cf.

the

corresponding

expression

for

sound waves

classical

perfect

fluid,

ego

Truesdell

[10].)

determine

the

behaviour

the

amplitude

time

evolves

differentiate

(2.8)

first

materially

with

respect

and

then

with

respect

:x:,

and

then

take

jumps

across

the

wave;

also,

take

jumps

(2.9)

find

a second

equation.

The

details

these

calculations

are

contained

[6] and so we

simply

describe

the

results

here.

The

relevant

equations

are

(2.24)

and

6B (2

iIT +

2V:x:

B + C U

PV:x:

P:x:

- U - UE + pG =

Although

the

principal

part

(2.8)

and

(2.9)

suggests

these

equations

may be

written

hyperbolic

system

this

not

evidently

so.

While we would have two

real

wavespeeds

(2.23),

the

remaining

two would be complex. I am

indebted

Professor

Ruggeri

for

pointing

this

out

me.

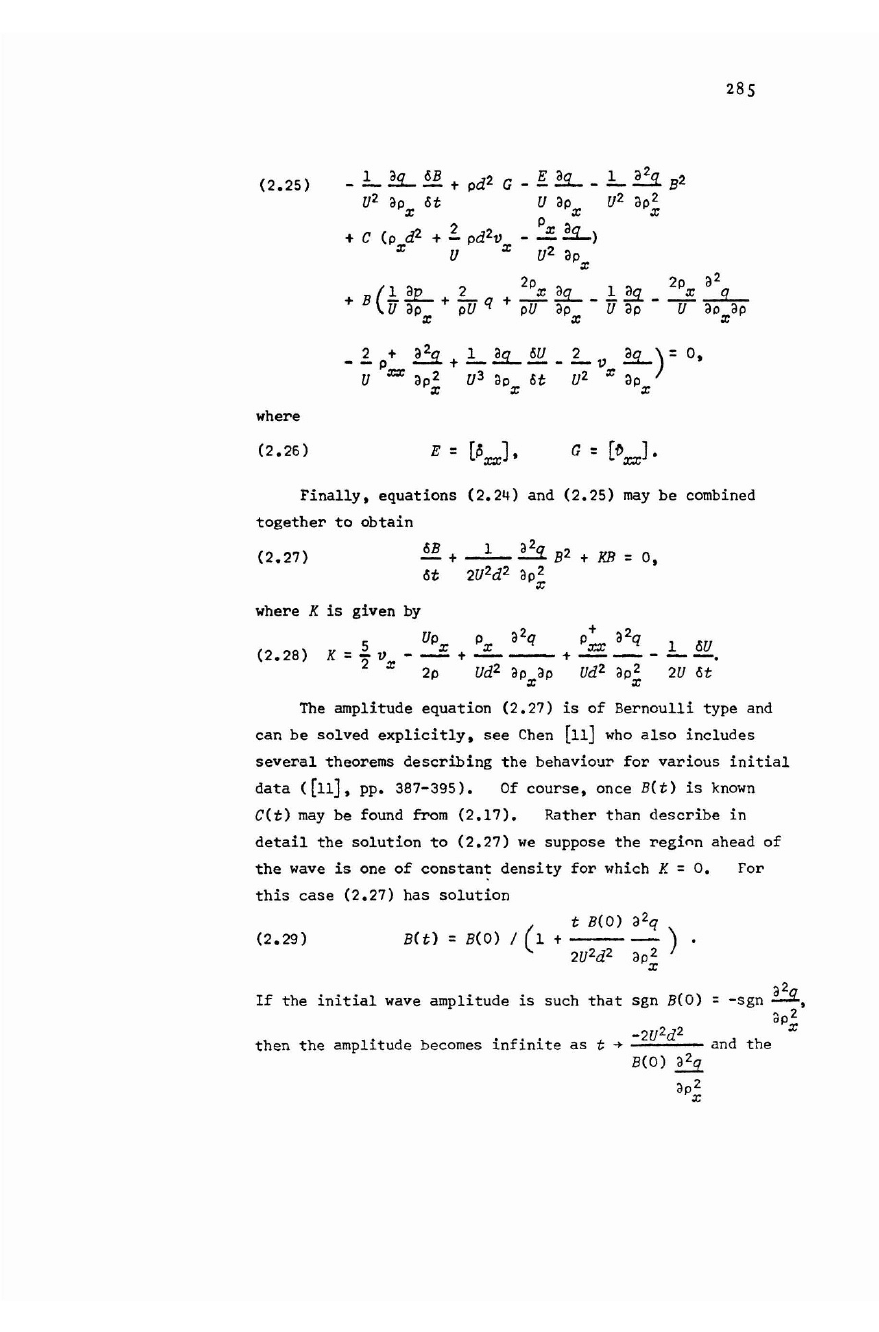
285

(2.25)

2 +

aZn

W 2

an)

--p

.;....;o.+-"'""----V

apZ

x ap

x x

:t:

where

Finally,

equations

(2.24)

and

(2.25)

may be combined

together

obtain

(2.27)

2!.

_1_

BZ + KB = O.

apZ

where

given

Z + 2

UP:t:

P:t:

a q

a q 1

(2.28)

K=-V

--+---+-----.

2 Z 2p

uaz

ap ap

apZ 2U

:t:

The

amplitude

equation

(2.27)

Bernoulli

type

and

can be

solved

explicitly.

see

Chen [11] who

also

includes

several

theorems

describing

the

behaviour

for

various

initial

data

([11],

pp. 387-

395).

course.

once

B(t)

known

C(t)

may be found from

(2.17).

Rather

than

describe

detail

the

solution

(2.27)

we suppose

the

reg

inn

ahead

the

wave

one

cons

tan!

density

for

which K =O. For

this

case

(2.27)

has

solution

(2.29

)

the

initial

wave

amplitude

such

that

sgn B(O)

then

the

amplitude

becomes

infinite

286

dipolar

stress

wave

some

sense

breaks

down.

(Iihile

the

treatment

the

continuity

and

momentum

equations

different

the

usual

procedure

for

acceleration

waves

perfect

fluid.

we have

essentially

used

the

compat-

ibility

relations

the

manner employed by Chen and

his

assoc-

iates.

see

ego

[11].

This

approach would

initially

appear

~ifferent

that

Jeffrey.

see

ego

[12];

but.

the

two

methods

are

fact

equivalent

was shown by

Boillat

and

Ruggeri

[13].)

3. Elastic dipolar

stress

waves.

While

would be

possible

develop a

theory

three

dimensional

elastic

dipolar

waves.

the

spirit

the

work

described

by Chen

[11].

seems more

revealing

this

stage

concentrate

the

novel

effects

caused by

the

presence

strain

gradients.

these

effects

are

present

one-dimen-

sional

wave motion we

restrict

attention

this

case.

this

end,

therefore.

let

rewrite

equations

(1.15)

(1.20)

their

one-dimensional

form.

Let

v and F =

aX/ax

functions

X and t and

supposing

the

body

forces

are

zero

the

equation

motion

then

where

(3.2)

11 =

poU:t

+ Po aF - Po

where

this

and

the

§ a

superposed

dot

denotes

the

partial

time

derivative.

F =

aX/ax.

the

dipolar

inertia

coefficient

and

the

form

(3.1)

clear

that

the

dipolar

theory

leads

differential

equation

fourth

order.

287

elastic

dipolar

stress

wave

defined

be a

surface

across

which

the

third

derivatives

are

~he

firs~

have

finite

discontinuities,

i.e.

x € c

) .

Because

~he

order

(3.1)

clear

that

obtain

the

wavespeed

such a wave we must

consider

the

discontinuity

relation

given

by Green and Naghdi

[9]

(cf.

the

shock wave

equation

(5~16),

Chen

[ll]).

we deno-te

the

wavespeed by V

the

appropriete

halance

relation

POV[.i:]

[11]

Subsequent

analysis

makes

use

the

relation

(equivalent

(2.14»

(3.5)

[I]

v[if]

(3.7)

In view

the

assumed

regularity

z ,

(3.4)

reduces

(3.6

)

[ ] a

[.. ]

- = M "x .

aF2

From

use

(3.5)

this

may

be changed

= H V2

aF2

When

]

which we

are

implicitly

assuming,

<3.7)

leads

immediately

expression

for

the

wavespeed, V,

(3.8)

V2 =

.!.

M aF}

worth comparing

(3.8)

with

the

analogous

expression

for

acceleration

wave

nonlinear

(ordinary)

elastic

material,

see

ego Chen

[11],

equation

(6.12).

derive

expression

for

the

wave

amplitude

now

return

equation

(3.1)

and

take

the

jump

this.

The

result

(3.9)

0 = r.L(l£)l -

rl:....(.2!!...)]

+ n

[~xy]

Lax

sr J

lax