Ferrarese G. (editor) Wave Propagation
Подождите немного. Документ загружается.

266
anti i s
assumed
as
a
criterion
to
pich
u;>
the
phy
sic
a l
sh
ocks
a
mo
ng
the
solutions
of
the
Rankine-Hugoniot
equations.
In
this
section
we
suggest
the
main
steps
of
an
explicitly
covariant
proof
of
the
fact
that
n
is
non
negative
on r.
Let
U; }
a
be a
subcharacteristic
covector
such
that
1
and
a a
covariant
scalar
defined
as
:
a = -
f;a4>a
thm
there
exists
a
space-like
covector
{l;
}
such
that
a
(24)
a
f; l; =
0.
a
(25)
°be
the
equation
of
a
characteristic
hypersurface
which
locally
has
the
same
"direction
of
propagation"
l;a
of
the
shock
surface.
i.e.
(k=1,2
•..••
N)
are
the
solution
of
(26);
these
eigenvalues
(k)
where
IJ
0,
(26)
(27)
are
real
by
the
hyperbolicity
condition.
Now
we
consider
a
solution
of
the
Rankine-Hugoniot
equations
(21)
(shock)
:
(28)
u, U*
being
the
perturbed
and
the
unperturbed
fields
respectively
on
r
(in
the
following
*
will
denote
the
values
of
any
function
of
the
field
computed
for
U = U*) .
Here
we
take
only
k-shocks
according
to
the
following
De
f i ni t i on
of
k-shock
We
shall
say
that
a
shock
is
a
k-shock
if
there
exists
a
number
k
(=1,2
•••.
,N)
such
that
lim
U =
U·
O-+-\J(k)
*
(29)

267
Roughly
speaking
a
k-shock
is
a
shock
that
vanishes
when
the
shock
speed
approaches
to
a
characteristic
velocity
(of
course,
these
shocks
become
weak
shocks
when 0
is
near
to
~~k)
).
We
suppose
to
know
the
solution
(28)
for
a
k-shock
and
replace
it
into
(23):
then
we
get
n
as
function
of
u* and
~a
(30)
By
differentiating
(30)
respect
to
~
a and
taking
into
account
(9),
after
some
calculations
we
obtain
a a
[h
J -
Vhf
[F
]
Thus
(31)
Since
h
is
a convex
function
of
U,
defined
in
a convex domain D, we
have:
w(U,U*)
=
-h(U)
+
h(U*)
+
Vh'(U
- U.. ) >
0,
l/- U
ot
U..
'"
D ,
So
the
r.h.s.
in
(31)
is
equal
to
-\!T,
restricted
to
r . Hence
F;a
an/a~a
< 0
Furthermore,
in
the
frame
S •
in
which
F;
0 =1,
(32)
can
be
written
ani ao > 0
(32)
i
F;
=0,
locally,
condi t i on
(33)
As
an/
ao
is
a
scalar
quantity,
inequality
(33)
is
independent
of
the
frame;
So n
is
a
strictly
increasing
function
of
0
in
any
frame.
Since
our
shock
is
supposed
to
be a
k-shock
we
have
lim
n
=0;
o~(k)
*
hence
we
get
Fop a aonvex
aovaPiant
density
system
and a
k-shoak
one has
when 0
(k)(
}
.::.
Jl*
on
r.
268
ii}
'I
as
generating
function
of
the
shock.
If
'I
i8
a
k1101J
function
of
u· and 'Po'
it
is
easy to
prove
that
the
fol'LoLn.ng
relations
holds
on r
"'here
v· 'I
(34)
V·
., a/au-,
o
A
-.
o
~
(U·)
Eq.
(34)
means
that
if
we know
only
the
scalar
function
'I
(which
is
a
function
of
U·
and
'P
a'
wi
th
~
a
non
characteristic)
we may
find
the
jump
of
U'
and
therefore
of
U; 'I
behaves
like
a
"potential"
for
the
shock.
Of
course,
in
practice,
f\(U·.
'P
a
}
is
computed
when
the
shock
is
known
as
a
solution
of
the
Rankine-Hugoniot
equations.
However
it
is
interesting
the
fact
that,
were
it
possible
to
determine
'I
through
experimental
tests,
we
should
be
able
to
have
all
information
of
the
shock.
iii}
Relativistic
bound
of
the
shock
speed.
The
Rankine-Hugoniot
equations
(35)
provide
N
equations
for
the
perturbed
field
U
if
U·
and
'P
a
are
known.
Eqs.
(35)
are
equations
of
the
kind
(36)
which
always
possess
the
trivial
solution
U ., U*
for
any
~
a They may
have
also
non
trivial
solutions
U
~
U·
(branching
solutions
of
the
trivial
.sot ut aon )
which
in
turn
are
physically
acceptable
only
if
gaa
~
a
~
a
~
0,
so
that
the
speed
of
the
shocks
does
not
exceed
that
of
light,
according
to
relativity
theory.
We
put
now
the
following
question:
does
it
exist
a
set
of
values
of
~
a
such
that
the
function
f
is
globally
invertible
with
respect
to
U
for
a
fixed
'P
a ?
If
the
answer
is
affermative,
then
only
the
trivial
269
solution
U = U* i s
allowe
d.
The
problem
has
be en exami ned by G.
Soillat
and
T. Ru
gge
r
i.
who
proved
[6J
that
non
van
i s
hi
ng
sc
h
oks
t
ake
place
only
if
their
speed
is
greater
than
the
smallest
cha r
ac
t e r i s t i c
speed
and
smaller
than
the
greatest
one.
It
is
possible
to
provide
an
explicitly
covariant
formulation
of
the
proof
given
in
[6J
and
show
that:
For
hyperboUa
convex
covariant
density
systems
the
speed
of
the
non
vani-
shing
shook
fulfiZ
the
condition:
where
m
inf
UeD
m < 0 < M
.
(k)
mln{lJ
},
k
M
sup
UE:
D
(k)
Max {lJ }
k
(37)
As a aonsequence
the
sh~ak
manifolds
are
time-l
ike
or
light-lik
e
if
so
are
the
aharacteristia
manifolds.
In
fact
if
(37)
holds,
and
the
characteristic
man
ifolds
are
time-like
or
light-like
we
have
:
<
sup
U€D
M
{
Cla
(k)
(k)}
axg
qJ lil
<0.
k Cl a
I.
Relativistic
Hydrodynamics
-
Existence
of
a
Convex
Covariant
Density.
The
equations
of
relativistic
hydrodynamics
are
a
(ru
Cl
) = 0
Cl
(38)
(39)
Cla
d
Cl
denoting
the
covariant
derivative
operator
and
T
the
energy-momentum
tensor
Cla c a ClB
T =
rfu
u - p g
(40)
Here
r
is
the
matter
density,
f
the
index
of
the
fluid,
uCl
the
4-veloci
ty
Cl
(u
u
o
one.
1)
and
p
the
pressure.
The
speed
of
light
is
assumed
equal
to
270
By
(38)
and
(39),
taking
into
account
the
thermodynamic
relations,
rf
= p+ p
r a dS
rdf
- dp
(41)
(42)
we
can
prove
the
existence
of
the
supplementary
conservation
law
(43)
where S
is
the
specific
entropy
(entropy
of
the
unit
mass),
a
the
thermo-
dynamic
absolute
temperature
and p
the
proper
energy
density
of
the
fluid.
The
system
(38),
(39)
may be
put
in
the
compact form
(5)
on
choosing:
f _
0;
(Il=O,l.2,3).
The
supplementary
law
(43)
coincides
with
(8)
when
g O.
It
is
possible
to
show
that
the
system
of
relativistic
fluid
dynamics
possesses
a
convex
covariant
density.
In
fact:
i)
there
exist
the
main
field
satisfying
(12)
U'
1
a
(44)
where G = f - e S - 1
is
the
free
enthalpy
(it
is
remarkable
that
the
components
of
the
main
field,
all
independet,
elle
velocity,
the
absolute
temperature
(because
essentially
coincide
with
u
Q
u =1) and
the
free
Q
enthalpy,
Le.
"observables"
of
the
system);
ii)
it
is
possible
to
prove
that
the
covariant
density
is
a convex
function
of
the
field

271
u
(45)
for
any
unity
time-like
covector{(a}
oriented
towards
the
future,
provided
the
usual
thermodynamic
conditions
0;
_
{G
}2>
0
ap
(G
=3G/oa. G =oG/op)
a p
are
satisfied
and
the
Sound
velocity
is
smaller
than
the
velocity
of
light
in
vacuum.
Hence
the
system
of
hydrodynamics
equat.ions
is
a
particular
case
of
the
general
theory.
As a
consequence
we
have:
1)
The
system
of
re
1Ativistia
hydrodynamics
equations
is
syrrunetric hyperbo
ldc
in
the
fieUl. U'
(44)
with
the
four-veator
generatirlfJ
functions
simpLy
given
by
2)
[5]
> 0 on r, when
This
is
a
conseq~ence
of
i)
since
it
is
possible
to
show
that
n =
_r*u
a
~
* a
[5]
=
r*(o_)l*)u
a
(
[5]
•
* a
(46)
where
)1*
is
a
characteristic
speed
of
the
corresponding
material
wave:
o a
IJ* =
(u
1;)
/
(u
( ) •
* 0 * a
3) The knauledqe
of
[5
] as a
function
of
u* and
~
0 detierminee
the
shook,
This
is
a
consequence
of
ii)
and
(46).
4)
The
veLocity
of
propagat.ion
of
the
reZativistia
hydrodynamias
shocks
never
exceeds
the
speed
og
Light.
REMARK
We
have
seen
above
that
the
main
field
U'
of
a
convex
covariant
density
system
is
invariant
under
transformations
of
the
field
U.
and
that
it
in
the
here
h
represents
the
a
conserved
quantity
h
the
a
•
with
respect
to
the
field
U = F
(0
component
of
the
proper
density
of
may be
expressed
as
the
gradient
of
the
convex
covariant
density
h =
=
hat
a
272
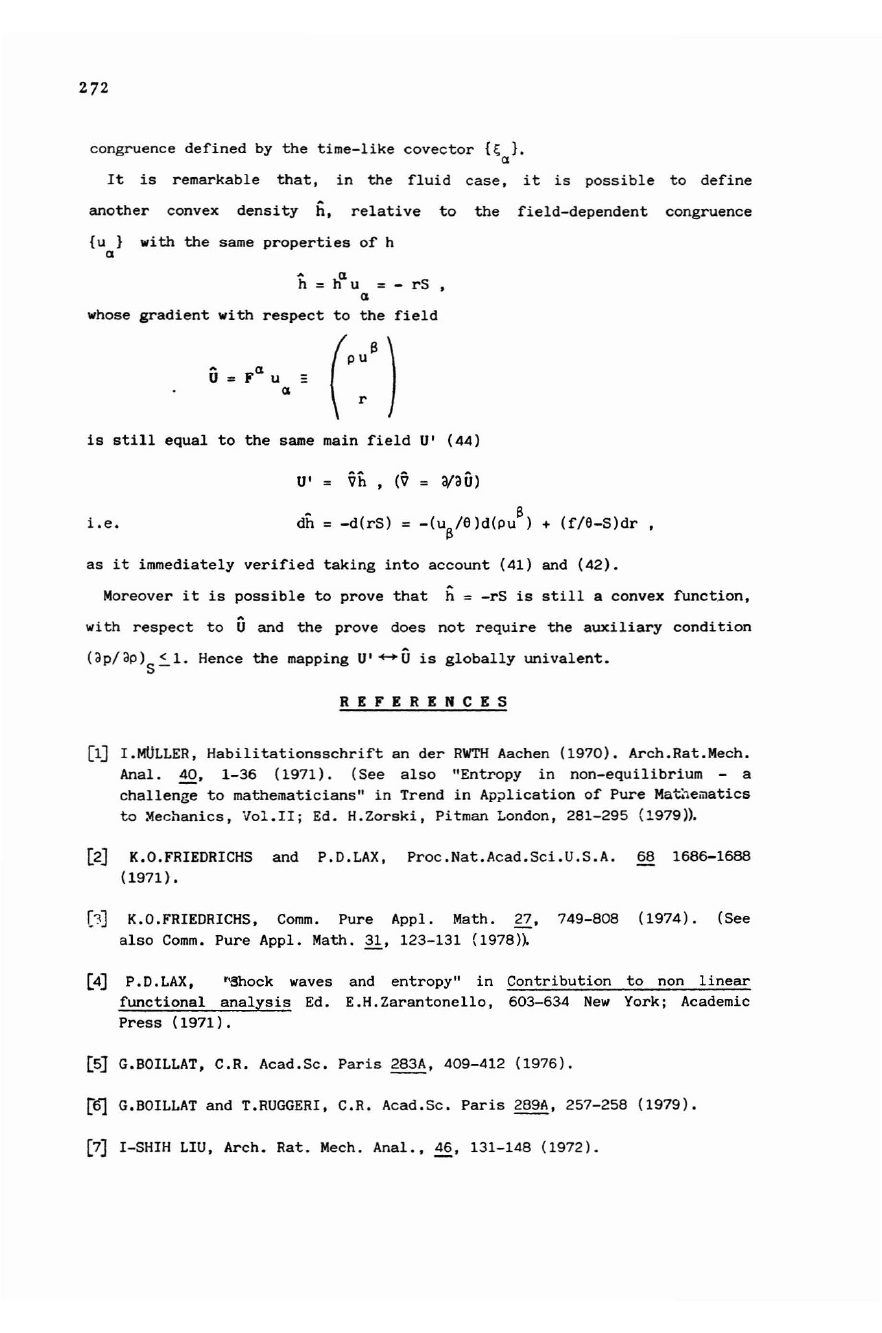
congruence
defined
by
the
time-like
covector
{~
}.
a
It
is
remarkable
that,
in
the
fluid
case,
it
is
possible
to
define
another
convex
density
h,
relative
to
the
field-dependent
congruence
{u}
with
the
same
properties
of
h
a
h =
~u
-
rS
,
a
whose
gradient
with
respect
to
the
field
(:'
)
is
still
equal
to
the
same main
field
U'
(44)
u' Vh,
(V
=
waul
Le.
dh
a
-d{rS)
=
-(ua/e)d(pu
) +
{f/e-S)dr
as
it
immediately
verified
taking
into
account
(41)
and
(42).
Moreover
it
is
possible
to
prove
that
h =
-rS
is
still
a
convex
function,
wi
th
respect
to
U and
the
prove
does
not
require
the
auxiliary
condition
(il
pi
ilp)S
~
1.
Hence
the
mapping U' ..... U
is
globally
univalent.
REF
ERE
N C E 5
[lJ
I.MULLER,
Habilitationsschrift
an
der
RWTH
Aachen
(1970).
Arch.Rat.Mech.
Anal.
40,
1-36
(1971).
(See
also
"Entropy
in
non-equilibrium
- a
challenge
to
mathematicians"
in
Trend
in
Ap~lication
of
Pure
Ma~
1ematics
to
;.Iechanics,
Vol.II;
Ed.
H.Zorski,
Pitman
London,
281-295
(1979)).
[2J K.O.FRIEDRICHS and P.D.LAX,
Proc.Nat.Acad.Sci.U.S.A.
68
1686-1688
(1971)
•
(
,]
K.O.FRIEDRICHS,
Comm.
Pure
Appl.
Math.
27,
749-808
(1974).
(See
also
Comm.
Pure
App1. Math.
31,
123-131
(1978)~
[4J P.D .LAX,
~Shock
waves and
entropy"
in
Contribution
to
non
linear
functional
analysis
Ed. E.H
.Zarantonello,
603-634
New
York; Academic
Press
(1971).
[5)
G.BOILLAT,
C.R.
Acad.Sc.
Paris
283A,
409-412
(1976).
[6J
G.BOILLAT
and
T.RUGGERI,
C.R.
Acad.Sc.
Paris
289A,
257-258
(1979).
[7] I-SHIH LIU,
Arch.
Rat.
Mech.
Anal.,
46,
131-148
(1972) .

273
(8J
M.BERGER
and
M.
BERGER
,
Perspectives
in
nonlinearity
. . W
.A.Benjamin;,
Inc.
New
York,
p.137
(1968).
[9]
T.RUGGERI
and
A.STRUMIA,
"Main
field
and
convex
for
quasi-linear
hyperbolic
systems.
Relativistic
(to
appear).
covar~ant
densi
ty
fluid
dynamics".

CENTRO
INTERNAZIONALE
MATEMATICO
ESTIVO
(C.I.M.E.)
SINGULAR
SURFACES
IN
DIPOLAR
MATERIALS
AND
POSSIBLE
CONSEQUENCES
FOR
CONTINUUM
MECHANICS
B.
STRAUGHAN
CIME
Session
on Wave
Propagation
Bressanone,
June
1980
Department
of
Mathematics,
University
of
Glasgow
University
Gardens,
Glasgow
G12
8QW

Singular surfaces "i n dipolar materials and possible consequences
for continuum mechanics.
B.
Straughan,
University
of
Glasgow.
1. Introduction
In
this
paper
we
study
the
evolutionary
behaviour
of
a
propagating
singular
surface
in two
types
of
nonlinear
dipolar
materials;
a
compressible
inviscid
dipolar
fluid
and an
elastic
dipolar
solid.
The
basic
theory
we
use
was
introduced
by Green and
Rivlin
[1] and from
the
constitutive
theory
viewpoint
essentially
extends
classical
continuum mechanics by
including
gradients
of
the
inde-
pendent
variables
present
in
non-polar
theories.
Gradient
type
theories
were
suggested
earlier
by,
for
example, !1axwell and by
Korteweg,
see
Truesdell
and
Noll
[2],
§125;
in
particular,
Korteweg
developed
an
interesting
theory
of
surface
tension
by
allowing
for
the
possibility
of
rupidly
changing
density
gradients
in
an
interface.
Since
in
a
singular
surface
qu
antities
such
as
density
and
its
gradients
of
various
or
ders
may
change
v
ery
rapidly
a
study
of
wave
motion
in
multi
pol
ar
material
s may
prove
of
value.
for
an
elastic
dipolar
material
the
theory
we empl oy was
derived
by Green and
Rivlin
[1], wh
erea
s
the
c
on
s
t
i
t
u
t
i
v
~
develop-
ment
for
dipolar
fluid
theory
is
due
to
Bl eus t ei n and Green [3]
