Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

1.3. The Diffusion Equation in a Square

extended to a function u(z,, z2, ) holomorphic for z, E C, z2 E C, and

Re t > 0. Any of the partial derivatives can be obtained by term-by-term

differentiation of (1.1.11).

For the proof, it suffices to observe that if I Im z,1, I Im z21 < a, and

Re > E, where a and E are positive, the general term of the series in (1.3.5)

is bounded in absolute value by

lamnIe(m+n)a-K(m2+n2)E

(1.3.14)

where the I amn l are uniformly bounded.

As a consequence of this result we see that the solution u(-) will in

fact be a classical solution (and more) for t > 0 (although the derivative on

the right at t = 0 must be understood in the LP sense).

Another notable property of the heat-diffusion equation is that, due

to the rapidly decreasing multipliers exp(- IC (n 2 + m2 ) t) in the Fourier

series (1.3.5) of a solution, the values of a family of solutions at any fixed

t > 0 tend to "bunch up" even if the family of initial values is widely

dispersed. A precise statement of this phenomenon is:

1.3.3 Lemma. Let (u k) be a sequence in L'(9) with

I I

< C

(n > 1) and let t > 0. Then the sequence (S(t)uk) c Cr(SZ),

the evolution

operator of (1.3.1)) contains a subsequence convergent in the norm of C r (S2 ).

Proof Using Lemma 1.3.2 for the computation of the derivatives

we have, noting that

I amn I < 47r -

211U(0)111 for

any L' solution of (1.3.1):

IIS(t)ukIICr(i), IIDJS(t)ukIICr(5)<C

for k > 1 and some constant C > 0. The second set of bounds and the mean

value theorem are easily seen to imply that the family (uk(t)) is equicontinu-

ous (more precisely, equi-Lipschitz continuous) in S2, thus the result follows

from the Arzela-Ascoli theorem (Dunford-Schwartz [1958: 1, p. 266]).

Considering the relations between the different LP norms among

them and with the norm of Cr, we obtain as a consequence of Lemma 1.3.3

that the propagators S(t) of (1.3.1) in any of the spaces LP or Cr are

compact operators for any t > 0 (see Example 3.10 for definitions and

properties).

A somewhat similar treatment of the heat-diffusion equation can be

given for the boundary condition

D"u(x, y,t)=0 (t>0, (x,y)El'),

(1.3.15)

where v denotes the outer normal vector at the boundary (which is well

defined except at the four corners of 9) and D° is the derivative in the

The Cauchy Problem for Some Equations of Mathematical Physics

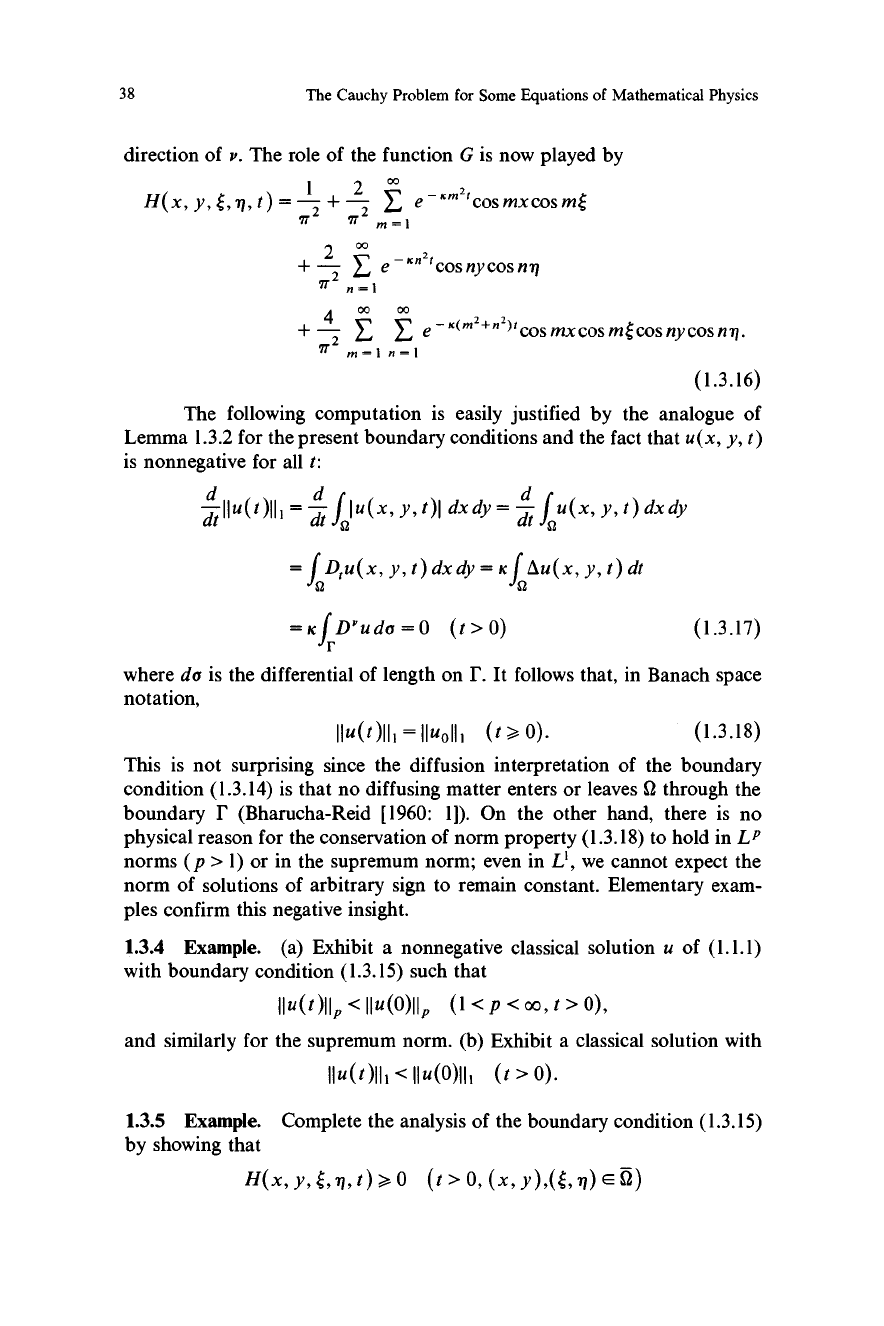
direction of P. The role of the function G is now played by

1 2 00

H (x ,

y, ,1 1, t) _ 2+

Y' e-"`"` c o s MX c o s m

- -

m=1

2 2

+ 2

e-"''cosnycosn1l

n=I

°° °°

+ 2 E E e-

K(,nZ+"Z)

`cos mxcos m cos nycos n q.

m=I n=I

(1.3.16)

The following computation is easily justified by the analogue of

Lemma 1.3.2 for the present boundary conditions and the fact that u(x, y, t)

is nonnegative for all t:

arllu(t)111=at

flu(x,Y,t)I

4 Du (x, y, t) dxdy = KfuAu(x, y, t) dt

K f D'udo=0 (t>0)

(1.3.17)

where do is the differential of length on IF. It follows that, in Banach space

notation,

11U(0111= 11U011'

(t >-0).

(1.3.18)

This is not surprising since the diffusion interpretation of the boundary

condition (1.3.14) is that no diffusing matter enters or leaves 12 through the

boundary I' (Bharucha-Reid [1960: 1]). On the other hand, there is no

physical reason for the conservation of norm property (1.3.18) to hold in LP

norms (p > 1) or in the supremum norm; even in L1, we cannot expect the

norm of solutions of arbitrary sign to remain constant. Elementary exam-

ples confirm this negative insight.

1.3.4

Example. (a) Exhibit a nonnegative classical solution u of (1.1.1)

with boundary condition (1.3.15) such that

Ilu(t)IIp < Ilu(0)IIp

(1 < p < oo, t > 0),

and similarly for the supremum norm. (b) Exhibit a classical solution with

IIu(t)IIl < IIu(0)111

(t>0).

1.3.5 Example.

Complete the analysis of the boundary condition (1.3.15)

by showing that

H(x,Y,E,si,t)>0

(t>0,(x,Y),(,s1)EF2)

1.4. The Schrodinger Equation

and

(t>0,(x,y)ESt).

State and prove the analogues of Lemmas 1.3.2 and 1.3.3.

1.4. THE SCHRODINGER EQUATION

In quantum mechanics, the state of a particle of mass m (say, in three-

dimensional space) under the influence of an external potential U is

described by the equation

h d

- = -

AiP+U'P

(1.4.1)

i dt

where h is Planck's constant and the function I 'P 12 is interpreted as the

probability density of the particle (the probability of finding the particle in

a set e at time t is fe I'P (x, t )12 dx). Clearly this interpretation imposes that

for all t and suggests that L2(683)c is the proper space for the study of

(1.4.1). We shall only consider here the free particle case (U = 0) and carry

out our study in R m for any m >, 1 as no additional difficulties are involved.

(For complete details about the physical situation modelled by (1.4.1) see

Schiff [1955: 1] or Fock [1976: 1].)

We study then the equation

u'(t) = du(t) (1.4.2)

where d is the operator in E = L2 (l ') defined by

dU=iKOU=1K((D')Zu+ +(D-

)2U),

(1.4.3)

K an arbitrary real number. The domain of d consists of all u E L2(R ') such

that Du (understood in the sense of distributions) belongs to L2. The

simplest way to study (1.4.2) is by means of the Fourier-Plancherel trans-

form J (see Section 8). The operator F is an isometric isomorphism from

L2(R

x) onto

L2(R

that transforms the operator d into the multiplication

operator

ltC (a) iKIG12u(a),

D(M) defined as the set of all u E L2(R') such that

I al2u E L2(R');

precisely, u e D((9',) if and only if j u = u E D(6X) and

(¢u) = 6X (Fu).

Hence, if uo E D(( ', ), we obtain a solution of (1.4.2) setting

u(x, t) =

9 -'(exp(- iKIaI2t) Yuo(a))

(1.4.4)

The Cauchy Problem for Some Equations of Mathematical Physics

where F -' is the inverse Fourier transform (verification of this formula is

based on the isometric character of 5 and 6 -' and is left to the reader).

Since D(() is dense in E part (a) of the definition in Section 1.2 is verified.

To check condition (b) we observe that if u E D(Ei ), then (tll',u, u) =

(5 u,'Fu)=iKfIaI2IuI2dasothat

Re(d u, u) = 0. (1.4.5)

Accordingly, if u(.) is a solution of (1.4.2) we have

D IIu(t)II2 = 2Re(u'(t), u(t))

= 2Re(du(t), u(t)) = 0

so that IIu(t)II is constant:

Ilu(t)II = Iiu(0)II.

We note that the preceding arguments can be justified just as well for

t negative as for t positive; in other words, the Cauchy problem for (1.4.2) is

"well posed for all t." This idea will be formalized in the next section; in the

rest of this one we examine the equation (1.4.2) in the space E = LP(Rm),

where 1 < p < oo. The operator d is still defined by (1.4.3) but now we take

D(C) = 5(R') = S, which is dense in LP. If u E S, then (1.4.4) provides a

solution of (1.4.2) in LP; this follows easily from the facts that the Fourier

transform F and its inverse F -' are continuous isomorphisms of 5 onto

itself and that convergence in S implies convergence in any LP space (see L.

Schwartz [1966: 1]). Because of the convolution theorem for Fourier trans-

forms of distributions (L. Schwartz [1966: 1, p. 268]), we can write (1.4.4) in

the form u =9 -'(exp(- iKI al2t)) * uo for uo E 5, or

u(x,

t) ri 1 m/2

ei1X-v12/4Ktuo(y) dy.

(1.4.6)

We examine in detail the case E = L'(Rm). If the Cauchy problem for

(1.4.2) were properly posed in L', then, given t > 0 there would exist a

constant C such that

Ilu(t)Ilt <Cllu0

(1.4.7)

for any function of the form (1.4.6). Assume this is the case, and let {(P7,) be

a 8-sequence (Section 8). Setting uo = (p in (1.4.6) and calling u the

function so obtained, we see that

t) - k(x, t) =

(47riKt)-"'//2exp(ilx

- vl2/4Kt)

for all x. Since I%II

I =1

for all n, it follows from Fatou's theorem that

k(., t) must be in L', which is false. This shows that (1.4.7) cannot hold, and

then that the Cauchy problem for (1.4.2) is not properly posed in L'. Much

more is true. In fact, we have

1.4. The Schrodinger Equation

*1.4.1 Example (Hormander [1960: 1, p. 109]). Let K be a real number,

K * 0. Then the operator

from 5 into S satisfies

Bu=9-I(e"'101 Fu)

IIBuIIP <CIIuIlp

(u E 5)

if and only if p = 2.

However, this sweeping negative result does not mean that LP results

for the Schrodinger equation are totally nonexistent. We shall reexamine the

subject in the next section.

An important difference between the Cauchy problem for the

Schrodinger equation and for the heat-diffusion equation is the following

(another one is pointed out in the next section). While solutions of the heat

equation either in Cr(SZ) or in LP(I) become extremely regular with the

passage of time (Lemma 1.3.2), a solution of the Schrodinger equation has

no tendency to become smoother than its initial value. A concrete formula-

tion of this statement is:

1.4.2

Lemma. Let be a solution of (1.4.2) in L2(Rm), and

assume that

u(to) E HS(R') for some to. Then u(t) E HS(Rm) for

- oo < t < oo ; in particular, u (0) E HS(R ')

To prove this result we only have to observe that (1.4.4) can be

obviously generalized to

u(x,t)= Y -'(exp(-iKlal2(t-to))Juo(o,to)

and take a look at the definition of the spaces HS (see Section 8); we note

that the HS norm of a solution is constant for all t.

1.4.3 Remark. The domain of the operator d in (1.4.3) coincides with

the space H2(Rm) if K

0 (see

Section 8); moreover, the norm

(II uI I + K - 211l? ull2 )1/2 (obviously equivalent to the graph norm II ull + II l u ID is

nothing but the norm 11'112,2 in H2(Rm) (see Section 8).

1.4.4

Remark. The operator d is closed. This can be seen as follows.

Assume

(u -

u -

a subsequence we may

assume that F u -' J u and I a 1 2 j u a.e., thus F v = I a 12 'Fu, and it

follows that u e D(d) and du = v. As the following example shows, this

result holds in much greater generality, although the proof cannot use the

Fourier transform, since p * 2.

1.4.5 Example. Let

du = E AaD"u (1.4.8)

IaI<r

be a differential operator of order r in LP(Rm)° (see Section 7) with

The Cauchy Problem for Some Equations of Mathematical Physics

m, v >_ 1,

1 < p < oo, where the A. are v X v complex-valued matrices and

D(d) is the set of all u E LP(Rm)" such that d u E LP(Rm)" (derivatives

understood in the sense of distributions). Then d is a closed operator.

1.4.6 Example.

Let d be as in Example 1.4.5,p = 2, 6J'(X)

the characteristic polynomial of d,

6Y(X)

XXAa (1.4.9)

jai <r

where x a = X 11.. X . Assume that

6Y(X) commutes with its complex matrix

adjoint

E kAa.

(1.4.10)

aj<r

(Equivalently, assume that AaA' = A'Aa for I a 1, 1$1 < r.) Then li is normal.

To see this we use the Fourier-Plancherel transform Y, still defined by (8.5)

as a vector integral in L2 (R

m )", and having properties similar to those in the

case v = 1. To compute d *, take u E D(d *). Then v - (u, ?v) is a continu-

ous linear functional of v in the L2 norm for v E D(d); in other words,

1/2

f(6y(-ia)'Yu(a),v(a))da<C(fIv(a)I2da) , (1.4.11)

where v indicates a generic element of FD(I?). Since this subspace is dense

in L2(ll

')", it follows that P(- ia)' u(a) belongs to L2(R Q )" and

d *U

= q -1(6J'(- ia)'qu(a)).

(1.4.12)

Conversely, if u E L2(R

)" is such that P(- ia)' u(a) E L2(R a)", then

u E D((*) and (1.4.12) holds. Since d itself can be expressed by

du= j -1(6p(-i(y)ju(a)),

(1.4.13)

the domain ofd consisting of all u E L2(R

)" such that 6(- ia) F u(a) E

L2(R' )", it follows easily from the equality

6J'(X)'Y(X) that

so that d is indeed normal. Obviously, the commutation condition for Y( A )

is automatically satisfied in the scalar case-that is, when v = 1. In view of

the expression (1.4.10) for PP(A)', we see that ¢* can also be defined, in the

same fashion as d, as the operator whose domain consists of all u E L2(Rm )"

such that l?'u E L2(Rm)", where (' is the formal adjoint of d,

(9''= (-1)""AaDau

(1.4.14)

aI<r

with d *u =117u. In particular, if d'= d, the operator ( is self-adjoint.

It follows from the preceding remarks that if d is the Schrodinger

operator (1.4.3), then id is self-adjoint.

1.6. The Maxwell Equations

1.5. THE CAUCHY PROBLEM IN (- oo, oo )

We present here an abstract formulation of the situation encountered in

Section 1.4 in relation to the Schrodinger equation. As in Section 1.2, A is a

densely defined operator in an arbitrary Banach space E.

We say that the Cauchy problem for

u'(t)=Au(t) (-oo<t<oo)

(1.5.1)

is well posed (or properly posed) in - oo < t < oo if and only if (a) and (b) of

Section 1.2 hold with the following modifications: in (a) we replace "there

exists a solution of (1.2.1) in t > 0" by "there exists a solution of (1.5.1) in

- oo < t < oo" and in (b) we assume the existence of a function C(t) such

that C(t) and C(- t) are nondecreasing in t > 0 and

IIu(t)II <C(t)IIu(0)II

(- oo < t < oo). (1.5.2)

In this language, the results of the previous section can be expressed by

stating that the Cauchy problem for the Schrodinger equation is properly

posed in (- oo, oo) with E = L2(R '). We note that this is far from true for

the heat equation considered in Section 1.1; in fact, consider the initial-value

problem (1.1.6), (1.1.7) in the space E = Cr(SZ) with uo(x, y) = un(x, y) _

sin nx sin ny. A solution in (- oo, oo) is given by

u(t)(x, y) = e-2Kn2tun(x, y).

(1.5.3)

However, if t < 0, IIu(t)II = exp(2un2Itl) whereas IIuoII = 1, which shows that

an inequality of the type of (1.5.2) will never hold for t negative. The same

counterexample works of course in LP(I) for any p > 1. We can thus say

that the problem of solving the heat (or diffusion) equation backwards in

time is "improperly posed." We shall study some problems of this type in

Chapter 6.

1.5.1 Example. If A E (E), the Cauchy problem for (1.5.1) is properly posed in

- oo < t < oo; it suffices to notice that the manipulations in Example 1.2.2 make just

as much sense for t < 0 as for t > 0. We note the following analogue of (1.2.13)

(which is similarly proved), expressing R(X) by means of the propagator S(t) for

t < 0: if Re X < -IIAII, then

oo e'1S(-t)dt=-R(X).

(1.5.4)

1.6. THE MAXWELL EQUATIONS

Let S, % be three-dimensional vector functions of x = (xI, x2, x3) and t. We

consider the system

at =

crot`JC

(1.6.1)

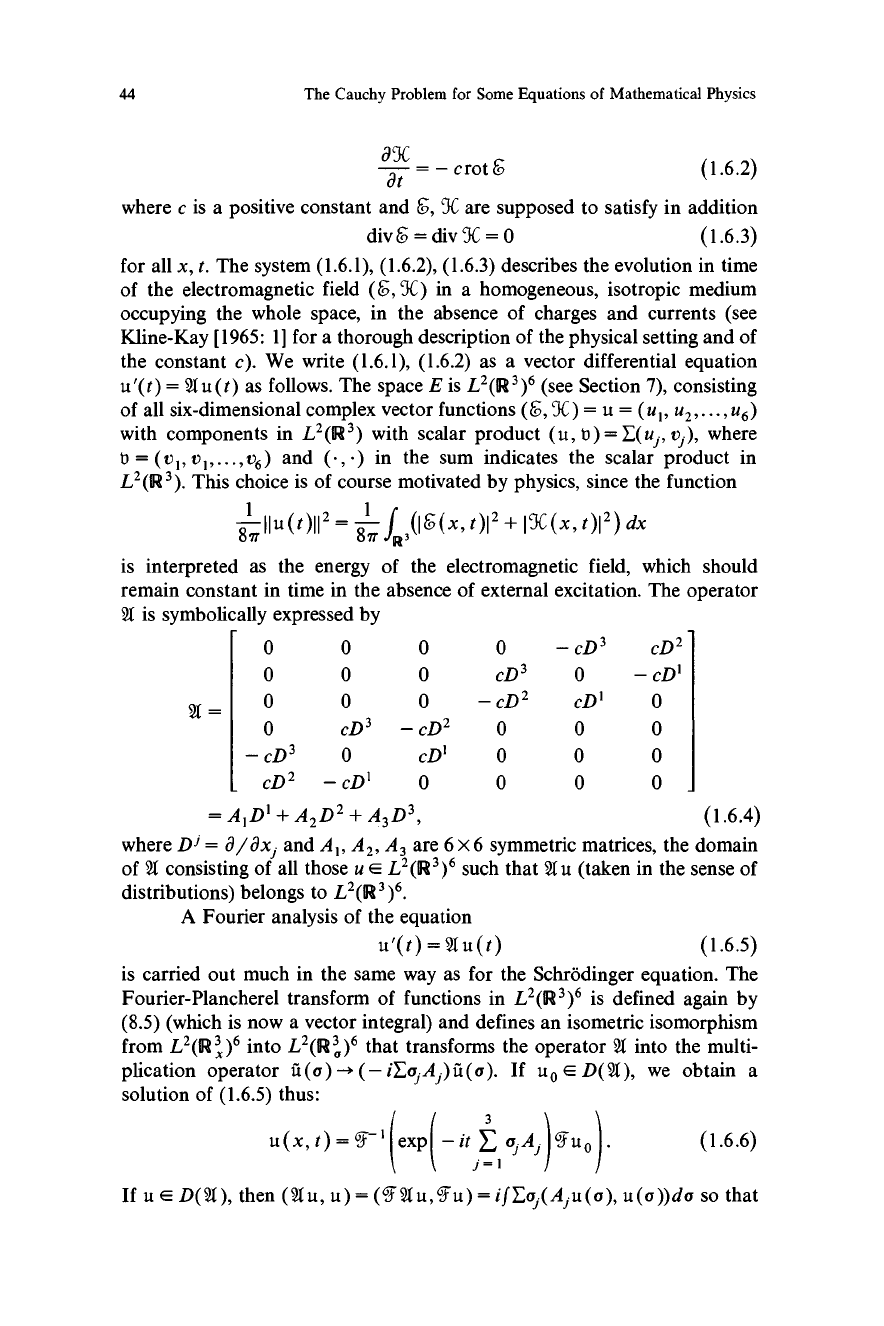
44 The Cauchy Problem for Some Equations of Mathematical Physics

a9C=-crot

(1.6.2)

where c is a positive constant and 6, 9C are supposed to satisfy in addition

div S = div 9C = 0

(1.6.3)

for all x, t. The system (1.6.1), (1.6.2), (1.6.3) describes the evolution in time

of the electromagnetic field (6;,K) in a homogeneous, isotropic medium

occupying the whole space, in the absence of charges and currents (see

Kline-Kay [1965: 1] for a thorough description of the physical setting and of

the constant c). We write (1.6.1), (1.6.2) as a vector differential equation

u'(t) = t u (t) as follows. The space E is L2 (l 3)6 (see Section 7), consisting

of all six-dimensional complex vector functions (&,K) = u = (u,, u2, ... 1U6)

with components in L2 (R 3) with scalar product (u, b) = E(uj, vj ), where

b = (v,, v1,.. . , vv) and ( , ) in the sum indicates the scalar product in

L2 (R 3 ). This choice is of course motivated by physics, since the function

11 IIu(t)112= 87TfR3(16 (x,t)12+19C(x,t)12)dx

is interpreted as the energy of the electromagnetic field, which should

remain constant in time in the absence of external excitation. The operator

9f is symbolically expressed by

0 0 0 0 -cD3

cD2

0 0 0 cD3 0

- cD'

= 0 0 0 - cD2 cD' 0

0 cD3

- cD2

0 0

- cD 3 0 cD' 0 0 0

cD2

- cD'

0 0 0 0

=A,D'+A2D2+A3D3, (1.6.4)

where Dj = 8/dxj and A,, A2, A3 are 6X6 symmetric matrices, the domain

of 1 consisting of all those u E L2(R3)6 such that 9fu (taken in the sense of

distributions) belongs to L2(R3)6

A Fourier analysis of the equation

u'(t)=91u(t)

(1.6.5)

is carried out much in the same way as for the Schrodinger equation. The

Fourier-Plancherel transform of functions in L2(R3)6 is defined again by

(8.5) (which is now a vector integral) and defines an isometric isomorphism

from L2(R3 )6 into L2(R )6 that transforms the operator % into the multi-

plication operator u (a) -(- i Eaj A j) u (a ). If u0 E D (9C ), we obtain a

solution of (1.6.5) thus:

u(x,t)= -' exp -it E ajAj Yuo . (1.6.6)

j=1

If u E D(S11), then (91u, u)= (5 S?tu, Yu) = i f aj(Aju(a), u(a))da so that

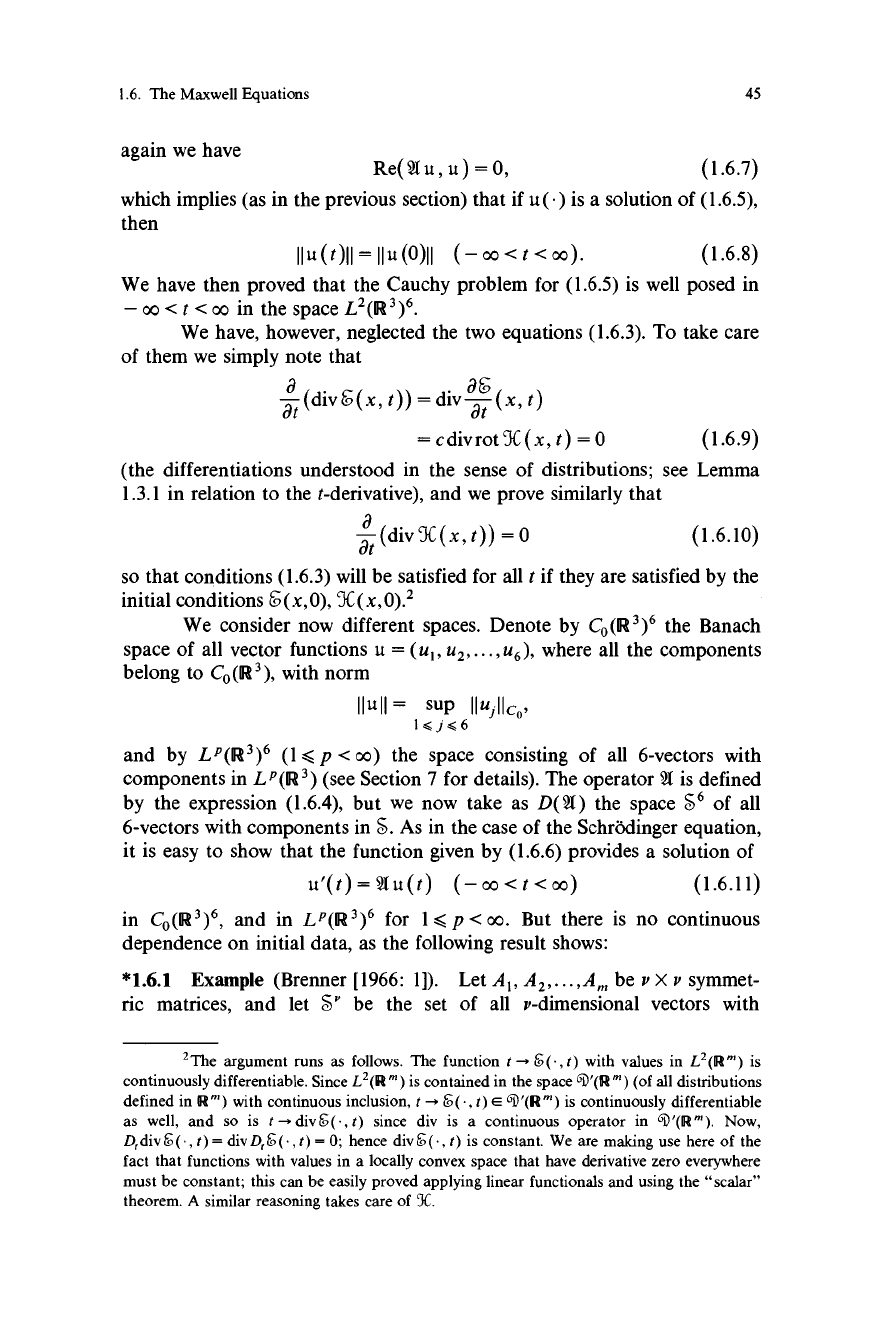
1.6. The Maxwell Equations

again we have

Re(% u, u) =0, (1.6.7)

which implies (as in the previous section) that if u is a solution of (1.6.5),

then

IIu(t)II=IIu(0)II

(-oo<t<oo).

(1.6.8)

We have then proved that the Cauchy problem for (1.6.5) is well posed in

- oo < t < oo in the space OR

3 )6

We have, however, neglected the two equations (1.6.3). To take care

of them we simply note that

at(div6; (x,t))=div

-(x,t)

= c div rot JC (x, t) = 0 (1.6.9)

(the differentiations understood in the sense of distributions; see Lemma

1.3.1 in relation to the t-derivative), and we prove similarly that

d (divXC(x,t))=0 (1.6.10)

so that conditions (1.6.3) will be satisfied for all t if they are satisfied by the

initial conditions G (x, 0), ¶ (x, 0).2

We consider now different spaces. Denote by Co(R3)6 the Banach

space of all vector functions u = (ul, u2,...,u6), where all the components

belong to Co (R 3 ), with norm

Hull=

sup I1ujllc",

I < j < 6

and by LP(R3)6 (1 < p < oo) the space consisting of all 6-vectors with

components in LP(R3) (see Section 7 for details). The operator 9f is defined

by the expression (1.6.4), but we now take as D(9f) the space 56 of all

6-vectors with components in S. As in the case of the Schrodinger equation,

it is easy to show that the function given by (1.6.6) provides a solution of

u'(t)=cu(t) (-oo<t<oo)

(1.6.11)

in CO(R3)6, and in LP(Q83)6 for

1 < p < oo. But there is no continuous

dependence on initial data, as the following result shows:

*1.6.1

Example (Brenner [1966: 1]).

Let A 1, A2,...,A,,, be v X v symmet-

ric matrices, and let 5" be the set of all v-dimensional vectors with

2The argument runs as follows. The function t - G t) with values in L2(R') is

continuously differentiable. Since L2 (R ') is contained in the space 6 '(R "') (of all distributions

defined in R'') with continuous inclusion, t -

t) E nt'(R') is continuously differentiable

as well, and so is

t -> div in

constant. We are making use here of the

fact that functions with values in a locally convex space that have derivative zero everywhere

must be constant; this can be easily proved applying linear functionals and using the "scalar"

theorem. A similar reasoning takes care of %.

The Cauchy Problem for Some Equations of Mathematical Physics

components in 5. The operator

Bu=F-' expIiiaiA,JFu

J=1

satisfies

IIBuIIp 5 Cllullp

(II'IIp is the norm in LP(Rm)9) for p - 2, 1 S p S oo if and only if the

matrices A1,...,Am commute.

However, we cannot discard offhand the possibility that the restrictions

(1.6.3) on the space may improve the situation. This is in fact not the case, but a

little more work is necessary to clarify the point.

1.6.2

Lemma.

Let 9) = (p

1, 9p2, p3)

be a three-dimensional vector function

with components in S. Then we can write

(1.6.12)

where the components of J, 17 are infinitely differentiable and

div ' = 0,

rot q = 0.

(1.6.13)

Moreover,

and r1 belong to LP(R3)3 for every p, 1 <p <oo, and there exists a

constant C = Cp depending only on p such that

II IIp < Cllplip,

INIp < CII9pIIp

(1.6.14)

Proof.

Let S be the (closed) subspace of L2(R3)3 consisting of all solenoidal

vectors 4 (divV = 0 in the sense of distributions).3 Applying the projection theorem

we obtain a decomposition of the type of (1.6.12) with

' E C25, rt E (25 1. Now, if is

an arbitrary vector with components in S, rot E C5; it follows that

f (9,roti;)dx=0

for all such , hence rot r1= 0 in the sense of distributions. Observe next that

Or1= graddiv(g) - 4')-rotrot r1= grad divgp. Define a function 31 by

rl(x)

4,7f II graddivgp(x-y)dy.

(1.6.15)

Then Ij is infinitely differentiable; moreover, i1 = grad div9) (Courant-Hilbert [1962:

1, p. 246]) so that A(rl - ij) = 0 in the sense of distributions.

Let Tl1 be the first component of i . We write the integral in (1.6.15) as the

limit of the integral 3j

1. F

l y I > e and apply the divergence theorem twice. The

3Vectors in Ca need not have first derivatives in L2.