Durst F. Fluid Mechanics: An Introduction to the Theory of Fluid Flows

Подождите немного. Документ загружается.

4 1 Introduction, Importance and Development of Fluid Mechanics

We also want to draw the attention of the reader to the importance of ﬂuid

mechanics in the ﬁeld of chemical engineering, where many areas such as heat

and mass transfer processes and chemical reactions are inﬂuenced strongly

or rendered possible only by ﬂow processes. In this ﬁeld of engineering, it

becomes particularly clear that much of the knowledge gained in the natural

sciences can be used technically only because it is possible to let processes run

in a steady and controlled way. In many areas of chemical engineering, ﬂuid

ﬂows are being used to make steady-state processes possible and to guarantee

the controllability of plants, i.e. ﬂows are being employed in many places in

process engineering.

Often it is necessary to use ﬂow media whose properties deviate strongly

from those of Newtonian ﬂuids, in order to optimize processes, i.e. the use of

non-Newtonian ﬂuids or multi-phase ﬂuids is necessary. The selection of more

complex properties of the ﬂowing ﬂuids in technical plants generally leads

to more complex ﬂow processes, whose eﬃcient employment is not possible

without detailed knowledge in the ﬁeld of the ﬂow mechanics of simple ﬂuids,

i.e. ﬂuids with Newtonian properties. In a few descriptions in the present

introduction to ﬂuid mechanics, the properties of non-Newtonian media are

mentioned and interesting aspects of the ﬂows of these ﬂuids are shown. The

main emphasis of this book lies, however, in the ﬁeld of the ﬂows of Newtonian

media. As these are of great importance in many applications, their special

treatment in this book is justiﬁed.

1.2 Sub-Domains of Fluid Mechanics

Fluid mechanics is a science that makes use of the basic laws of mechanics and

thermodynamics to describe the motion of ﬂuids. Here ﬂuids are understood

to be all the media that cannot be assigned clearly to solids, no matter

whether their properties can be described by simple or complicated material

laws. Gases, liquids and many plastic materials are ﬂuids whose movements

are covered by ﬂuid mechanics. Fluids in a state of rest are dealt with as a

special case of ﬂowing media, i.e. the laws for motionless ﬂuids are deduced

in such a way that the velocity in the basic equations of ﬂuid mechanics is

set equal to zero.

In ﬂuid mechanics, however, one is not content with the formulation of the

laws by which ﬂuid movements are described, but makes an eﬀort beyond

that to ﬁnd solutions for ﬂow problems, i.e. for given initial and boundary

conditions. To this end, three methods are used in ﬂuid mechanics to solve

ﬂow problems:

(a) Analytical solution methods (analytical ﬂuid mechanics):

Analytical methods of applied mathematics are used in this ﬁeld to solve

the basic ﬂow equations, taking into account the boundary conditions

describing the actual ﬂow problem.

1.2 Sub-Domains of Fluid Mechanics 5

(b) Numerical solution methods (numerical ﬂuid mechanics):

Numerical methods of applied mathematics are employed for ﬂuid ﬂow

simulations on computers to yield solutions of the basic equations of ﬂuid

mechanics.

This sub-domain of ﬂuid mechanics uses similarity laws for the trans-

ferability of ﬂuid mechanics knowledge from model ﬂow investigations.

The knowledge gained in model ﬂows by measurements is transferred by

means of the constancy of known characteristic quantities of a ﬂow ﬁeld

to the ﬂow ﬁeld of actual interest.

The above-mentioned methods have until now, in spite of considerable de-

velopments in the last 50 years, only partly reached the state of development

which is necessary to be able to describe adequately or solve ﬂuid mechan-

ics problems, especially for many practical ﬂow problems. Hence, nowadays,

known analytical methods are often only applicable to ﬂow problems with

simple boundary conditions. It is true that the use of numerical processes

makes the description of complicated ﬂows possible; however, feasible solu-

tions to practical ﬂow problems without model hypotheses, especially in the

case of turbulent ﬂows at high Reynold numbers, can only be achieved in

a limited way. The limitations of numerical methods are due to the lim-

ited storage capacity and computing speed of the computers available today.

These limitations will continue to exist for a long time, so that a number of

practically relevant ﬂows can only be investigated reliably by experimental

methods. However, also for experimental investigations not all quantities of

interest, from a ﬂuid mechanics point of view, can always be determined, in

spite of the reﬁned experimental methods available today. Suitable measur-

ing techniques for obtaining all important ﬂow quantities are lacking, as for

example the measuring techniques to investigate the thin ﬂuid ﬁlms shown

in Fig. 1.4. Experience shows that eﬃcient solutions of practical ﬂow prob-

lems therefore require the combined use of the above-presented analytical,

numerical and experimental methods of ﬂuid mechanics. The diﬀerent sub-

domains of ﬂuid mechanics cited are thus of equal importance and mastering

the diﬀerent methods of ﬂuid mechanics is often indispensable in practice.

When analytical solutions are possible for ﬂow problems, they are prefer-

able to the often extensive numerical and experimental investigations. Un-

fortunately, it is known from experience that the basic equations of ﬂuid

mechanics, available in the form of a system of nonlinear and partial diﬀer-

ential equations, allow analytical solutions only when, with regard to the

equations and the initial and boundary conditions, considerable simpliﬁ-

cations are made in actually determining solutions to ﬂow problems. The

validity of these simpliﬁcations has to be proved for each ﬂow problem to be

solved by comparing the analytically achieved ﬁnal results with the corre-

sponding experimental data. Only when such comparisons lead to acceptably

small diﬀerences between the analytically determined and experimentally in-

vestigated velocity ﬁeld can the hypotheses, introduced into the analytical

6 1 Introduction, Importance and Development of Fluid Mechanics

Fig. 1.4 Experimental investigation of ﬂuid ﬁlms

solution of the ﬂow problem, be regarded as justiﬁed. In cases where such a

comparison with experimental data is unsatisfactory, it is advisable to justify

theoretically the simpliﬁcations by order of magnitude considerations, so as

to prove that the terms neglected, for example in the solution of the basic

equations, are small in comparison with the terms that are considered for the

solution.

One has to proceed similarly concerning the numerical solution of ﬂow

problems. The validity of the solution has to be proved by comparing the

results achieved by ﬁnite volume methods and ﬁnite element methods with

corresponding experimental data. When such data do not exist, which may

be the case for ﬂow problems as shown in Figs. 1.5 and 1.6, statements on

the accuracy of the solutions achieved can be made by the comparison of

three numerical solutions calculated on various ﬁne grids that diﬀer from

one another by their grid spacing. With this knowledge of precision, ﬂow

information can then can be obtained from numerical computations that are

relevant to practical applications. Numerical solutions without knowledge of

the numerically achieved precision of the solution are unsuitable for obtaining

reliable information on ﬂuid ﬂow processes.

When experimental data are taken into account to verify analytical or nu-

merical results, it is very important that only such experimental data that can

be classiﬁed as having suﬃcient precision for reliable comparisons are used.

A prerequisite is that the measuring data are obtained with techniques that

allow precise ﬂow measurements and also permit one to determine ﬂuid ﬂows

1.2 Sub-Domains of Fluid Mechanics 7

Fig. 1.5 Numerical calculation of the ﬂow around a train in crosswinds

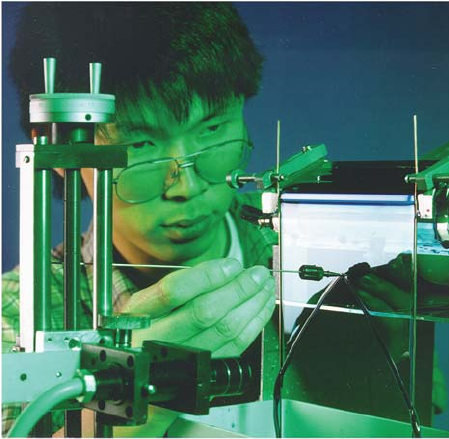
Fig. 1.6 Flow investigation with the aid of a laser Doppler anemometer

by measurement in a non-destructive way. Optical measurement techniques

fulﬁll, in general, the requirements concerning precision and permit mea-

surements without disturbance, so that optical measuring techniques are

nowadays increasingly applied in experimental ﬂuid mechanics (see Fig. 1.6).

In this context, laser Doppler anemometry is of particular importance. It has

developed into a reliable and easily applicable measuring tool in ﬂuid me-

chanics that is capable of measuring the required local velocity information

in laminar and turbulent ﬂows.

Although the equal importance of the diﬀerent sub-domains of ﬂuid me-

chanics presented above, according to the applied methodology, has been

outlined in the preceding paragraphs, priority in this book will be given to

analytical ﬂuid mechanics for an introductory presentation of the methods for

solving ﬂow problems. Experience shows that it is better to include analytical

solutions of ﬂuid mechanical problems in order to create or deepen with their

8 1 Introduction, Importance and Development of Fluid Mechanics

help students’ understanding of ﬂow physics. As a rule, analytical methods

applied to the solution of ﬂuid ﬂow problems, are known to students from lec-

tures in applied mathematics. Hence students of ﬂuid mechanics bring along

the tools for the analytical solutions of ﬂow problems. This circumstance

does not necessarily exist for numerical or experimental methods. This is the

reason why in this introductory book special signiﬁcance is attached to the

methods of analytical ﬂuid mechanics. In parts of this book numerical solu-

tions are treated in an introductory way in addition to presenting results of

experimental investigations and the corresponding measuring techniques. It

is thus intended to convey to the student, in this introduction to the subject,

the signiﬁcance of numerical and experimental ﬂuid mechanics.

The contents of this book put the main emphasis on solutions of ﬂuid

ﬂow problems that are described by simpliﬁed forms of the basic equations

of ﬂuid mechanics. This application of simpliﬁed equations to the solution

of ﬂuid problems represents a highly developed system. The comprehensible

introduction of students to the general procedures for solving ﬂow problems

by means of simpliﬁed ﬂow equations is achieved by the basic equations being

derived and formulated as partial diﬀerential equations for Newtonian ﬂuids

(e.g. air or water). From these general equations, the simpliﬁed forms of the

ﬂuid ﬂow laws can be derived in a generally comprehensible way, e.g. by the

introduction of the hypothesis that ﬂuids are free from viscosity. Fluids of

this kind are described as “ideal” from a ﬂuid mechanics point of view. The

basic equations of these ideal ﬂuids, derived from the general set of equations,

represent an essential simpliﬁcation by which the analytical solutions of ﬂow

problems become possible.

Further simpliﬁcations can be obtained by the hypothesis of incompress-

ibility of the considered ﬂuid, which leads to the classical equations of

hydrodynamics. When, however, gas ﬂows at high velocities are considered,

the hypothesis of incompressibility of the ﬂow medium is no longer justiﬁed.

For compressible ﬂow investigations, the basic equations valid for gas dynamic

ﬂows must then be used. In order to derive these, the hypothesis is introduced

that gases in ﬂow ﬁelds undergo thermodynamic changes of state, as they

are known for ideal gases. The solution of the gas dynamic basic equations is

successful in a number of one-dimensional ﬂow processes. These are appropri-

ately dealt with in this book. They give an insight into the strong interactions

that may exist between the kinetic energy of a ﬂuid element and the internal

energy of a compressible ﬂuid. The resulting ﬂow phenomena are suited for

achieving the physical understanding of one-dimensional gas dynamic ﬂuid

ﬂows and applying it to two-dimensional ﬂows. Some two-dimensional ﬂow

problems are therefore also mentioned in this book. Particular signiﬁcance

in these considerations is given to the physical understanding of the ﬂuid

ﬂows that occur. Importance is also given, however, to representing the ba-

sics of the applied analytical methods in a way that makes them clear and

comprehensible for the student.

1.3 Historical Developments 9

1.3 Historical Developments

In this section, the historical development of ﬂuid mechanics is roughly

sketched out, based on the most important contributions of a number of

scientists and engineers. The presentation does not claim to give a complete

picture of the historical developments: this is impossible owing to the con-

straints on allowable space in this section. The aim is rather to depict the

development over centuries in a generally comprehensible way. In summary,

it can be said that already at the beginning of the nineteenth century the

basic equations with which ﬂuid ﬂows can be described reliably were known.

Solutions of these equations were not possible owing to the lack of suitable

solution methods for engineering problems and therefore technical hydraulics

developed alongside the ﬁeld of theoretical ﬂuid mechanics. In the latter area,

use was made of the known contexts for the ﬂow of ideal ﬂuids and the in-

ﬂuence of friction eﬀects was taken into consideration via loss coeﬃcients,

determined empirically. For geometrically complicated problems, methods

based on similarity laws were used to generalize experimentally achieved ﬂow

results. Analytical methods only allowed the solution of academic problems

that had no relevance for practical applications. It was not until the second

half of the twentieth century that the development of suitable methods led to

the numerical techniques that we have today which allow us to solve the basic

equations of ﬂuid mechanics for practically relevant ﬂow problems. Parallel to

the development of the numerical methods, the development of experimental

techniques was also pushed ahead, so that nowadays measurement techniques

are available which allow us to obtain experimentally ﬂuid mechanics data

that are interesting for practical ﬂow problems.

Some technical developments were and still are today closely connected

with the solution of ﬂuid ﬂows or with the advantageous exploitation of ﬂow

processes. In this context, attention is drawn to the development of naviga-

tion with wind-driven ships as early as in ancient Egyptian times. Further

developments up to the present time have led to transport systems of great

economic and socio-political signiﬁcance. In recent times, navigation has been

surpassed by breathtaking developments in aviation and motor construction.

These again use ﬂow processes to guarantee the safety and comfort which

we take for granted nowadays with all of the available transport systems. It

was ﬂuid mechanics developments which alone made this safety and comfort

possible.

The continuous scientiﬁc development of ﬂuid mechanics started with

Leonardo da Vinci (1452–1519). Through his ingenious work, methods were

devised that were suitable for ﬂuid mechanics investigations of all kinds. Ear-

lier eﬀorts of Archimedes (287–212 B.C.) to understand ﬂuid motions led to

the understanding of the hydromechanical buoyancy and the stability of ﬂoat-

ing bodies. His discoveries remained, however, without further impact on the

development of ﬂuid mechanics in the following centuries. Something similar

holds true for the work of Sextus Julius Frontinus (40–103), who provided the

10 1 Introduction, Importance and Development of Fluid Mechanics

basic understanding for the methods that were applied in the Roman Empire

for measuring the volume ﬂows in the Roman water supply system. The work

of Sextus Julius Frontinus also remained an individual achievement. For more

than a millennium no essential ﬂuid mechanics insights followed and there

were no contributions to the understanding of ﬂow processes.

Fluid mechanics as a ﬁeld of science developed only after the work of

Leonardo da Vinci. His insight laid the basis for the continuum principle for

ﬂuid mechanics considerations and he contributed through many sketches of

ﬂow processes to the development of the methodology to gain ﬂuid mechanics

insights into ﬂows by means of visualization. His ingenious engineering art

allowed him to devise the ﬁrst installations that were driven ﬂuid mechani-

cally and to provide sketches of technical problem solutions on the basis of

ﬂuid ﬂows. The work of Leonardo da Vinci was followed by that of Galileo

Galilei (1564–1642) and Evangelista Torricelli (1608–1647). Whereas Galileo

Galilei produced important ideas for experimental hydraulics and revised the

concept of vacuum introduced by Aristoteles, Evangelista Torricelli realized

the relationship between the weight of the atmosphere and the barometric

pressure. He developed the form of a horizontally ejected ﬂuid jet in connec-

tion with the laws of free fall. Torricelli’s work was therefore an important

contribution to the laws of ﬂuids ﬂowing out of containers under the inﬂuence

of gravity. Blaise Pascal (1623–1662) also dedicated himself to hydrostatics

and was the ﬁrst to formulate the theorem of universal pressure distribution.

Isaac Newton (1642–1727) laid the basis for the theoretical description of

ﬂuid ﬂows. He was the ﬁrst to realize that molecule-dependent momentum

transport, which he introduced as ﬂow friction, is proportional to the velocity

gradient and perpendicular to the ﬂow direction. He also made some addi-

tional contributions to the detection and evaluation of the ﬂow resistance.

Concerning the jet contraction arising with ﬂuids ﬂowing out of containers,

he engaged in extensive deliberations, although his ideas were not correct in

all respects. Henri de Pitot (1665–1771) made important contributions to the

understanding of stagnation pressure, which builds up in a ﬂow at stagnation

points. He was the ﬁrst to endeavor to make possible ﬂow velocities by dif-

ferential pressure measurements following the construction of double-walled

measuring devices. Daniel Bernoulli (1700–1782) laid the foundation of hy-

dromechanics by establishing a connection between pressure and velocity,

on the basis of simple energy principles. He made essential contributions to

pressure measurements, manometer technology and hydromechanical drives.

Leonhard Euler (1707–1783) formulated the basics of the ﬂow equations

of an ideal ﬂuid. He derived, from the conservation equation of momentum,

the Bernoulli theorem that had, however, already been derived by Johann

Bernoulli (1667–1748) from energy principles. He emphasized the signiﬁcance

of the pressure for the entire ﬁeld of ﬂuid mechanics and explained among

other things the appearance of cavitations in installations. The basic princi-

ple of turbo engines was discovered and described by him. Euler’s work on

the formulation of the basic equations was supplemented by Jean le Rond

1.3 Historical Developments 11

d’Alembert (1717–1783). He derived the continuity equation in diﬀerential

form and introduced the use of complex numbers into the potential theory.

In addition, he derived the acceleration component of a ﬂuid element in ﬁeld

variables and expressed the hypothesis, named after him and proved before

by Euler, that a body circulating in an ideal ﬂuid has no ﬂow resistance. This

fact, known as d’Alembert’s paradox, led to long discussions concerning the

validity of the equations of ﬂuid mechanics, as the results derived from them

did not agree with the results of experimental investigations.

The basic equations of ﬂuid mechanics were dealt with further by Joseph

de Lagrange (1736–1813), Louis Marie Henri Navier (1785–1836) and Barre

de Saint Venant (1797–1886). As solutions of the equations were not success-

ful for practical problems, however, practical hydraulics developed parallel

to the development of the theory of the basic equations of ﬂuid mechan-

ics. Antoine Chezy (1718–1798) formulated similarity parameters, in order

to transfer the results of ﬂow investigations in one ﬂow channel to a second

channel. Based on similarity laws, extensive experimental investigations were

carried out by Giovanni Battista Venturi (1746–1822), and also experimental

investigations were made on pressure loss measurements in ﬂows by Gotthilf

Ludwig Hagen (1797–1884) and on hydrodynamic resistances by Jean-Louis

Poiseuille (1799–1869). This was followed by the work of Henri Philibert

Gaspard Darcy (1803–1858) on ﬁltration, i.e. for the determination of pres-

sure losses in pore bodies. In the ﬁeld of civil engineering, Julius Weissbach

(1806–1871) introduced the basis of hydraulics into engineers’ considerations

and determined, by systematic experiments, dimensionless ﬂow coeﬃcients

with which engineering installations could be designed. The work of William

Froude (1810–1879) on the development of towing tank techniques led to

model investigations on ships and Robert Manning (1816–1897) worked out

many equations for resistance laws of bodies in open water channels. Similar

developments were introduced by Ernst Mach (1838–1916) for compressible

aerodynamics. He is seen as the pioneer of supersonic aerodynamics, provid-

ing essential insights into the application of the knowledge on ﬂows in which

changes of the density of a ﬂuid are of importance.

In addition to practical hydromechanics, analytical ﬂuid mechanics devel-

oped in the nineteenth century, in order to solve analytically manageable

problems. George Gabriel Stokes (1816–1903) made analytical contributions

to the ﬂuid mechanics of viscous media, especially to wave mechanics and

to the viscous resistance of bodies, and formulated Stokes’ law for spheres

falling in ﬂuids. John William Stratt, Lord Rayleigh (1842–1919) carried out

numerous investigations on dynamic similarity and hydrodynamic instability.

Derivations of the basis for wave motions, instabilities of bubbles and drops

and ﬂuid jets, etc., followed, with clear indications as to how linear instabil-

ity considerations in ﬂuid mechanics are to be carried out. Vincenz Strouhal

(1850–1922) worked out the basics of vibrations and oscillations in bodies

through separating vortices. Many other scientists, who showed that applied

mathematics can make important contributions to the analytical solution

12 1 Introduction, Importance and Development of Fluid Mechanics

of ﬂow problems, could be named here. After the pioneering work of Lud-

wig Prandtl (1875–1953), who introduced the boundary layer concept into

ﬂuid mechanics, analytical solutions to the basic equations followed, e.g. so-

lutions of the boundary layer equations by Paul Richard Heinrich Blasius

(1883–1970).

With Osborne Reynolds (1832–1912), a new chapter in ﬂuid mechanics

was opened. He carried out pioneering experiments in many areas of ﬂuid

mechanics, especially basic investigations on diﬀerent turbulent ﬂows. He

demonstrated that it is possible to formulate the Navier–Stokes equations

in a time-averaged form, in order to describe turbulent transport processes

in this way. Essential work in this area by Ludwig Prandtl (1875–1953) fol-

lowed, providing fundamental insights into ﬂows in the ﬁeld of the boundary

layer theory. Theodor von Karman (1881–1993) made contributions to many

sub-domains of ﬂuid mechanics and was followed by numerous scientists who

engaged in problem solutions in ﬂuid mechanics. One should mention here,

without claiming that the list is complete, Pei-Yuan Chou (1902–1993) and

Andrei Nikolaevich Kolmogorov (1903–1987) for their contributions to turbu-

lence theory and Herrmann Schlichting (1907–1982) for his work in the ﬁeld of

laminar–turbulent transition, and for uniting the ﬂuid-mechanical knowledge

of his time and converting it into practical solutions of ﬂow problems.

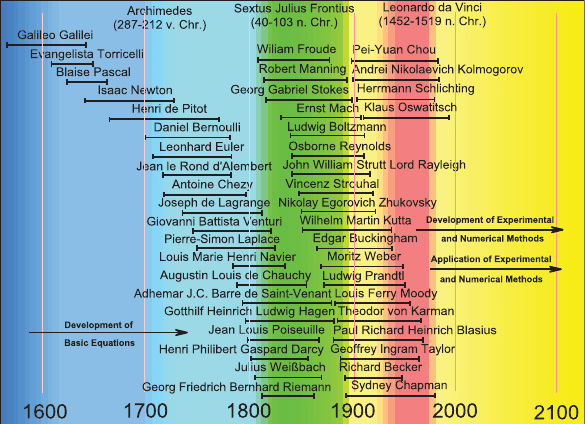
The chronological sequence of the contributions to the development of ﬂuid

mechanics outlined in the above paragraphs can be rendered well in a diagram

as shown in Fig. 1.7. This information is taken from history books on ﬂuid

Fig. 1.7 Diagram listing the epochs and scientists contributing to the development

of ﬂuid mechanics

1.3 Historical Developments 13

mechanics as given in refs [1.1] to [1.6]. On closer examination one sees that

the sixteenth and seventeenth centuries were marked by the development

of the understanding of important basics of ﬂuid mechanics. In the course

of the development of mechanics, the basic equations for ﬂuid mechanics

were derived and fully formulated in the eighteenth century. These equations

comprised all forces acting on ﬂuid elements and were formulated for sub-

stantial quantities (Lagrange’s approach) and for ﬁeld quantities (Euler’s

approach). Because suitable solution methods were lacking, the theoretical

solutions of the basic equations of ﬂuid mechanics, strived for in the nine-

teenth century and at the beginning of the twentieth century, were limited to

analytical results for simple boundary conditions. Practical ﬂow problems es-

caped theoretical solution and thus “engineering hydromechanics” developed

that looked for ﬂuid mechanics problem solutions by experimentally gained

insights. At that time, one aimed at investigations on geometrically simi-

lar ﬂow models, while conserving ﬂuid mechanics similarity requirements, to

permit the transfer of the experimentally gained insights by similarity laws

to large constructions. Only the development of numerical methods for the

solution of the basic equations of ﬂuid mechanics, starting from the middle

of the twentieth century, created the methods and techniques that led to

numerical solutions for practical ﬂow problems. Metrological developments

that ran in parallel led to complementary experimental and numerical so-

lutions of practical ﬂow problems. Hence it is true to say that the second

half of the twentieth century brought to ﬂuid mechanics the measuring and

computational methods that are required for the solution of practical ﬂow

problems. The combined application of the experimental and numerical meth-

ods, available today, will in the twenty-ﬁrst century permit ﬂuid mechanics

investigations that were not previously possible because of the lack of suitable

investigation methods.

The experimental methods that contributed particularly to the rapid ad-

vancement of experimental ﬂuid mechanics in the second half of the twentieth

century were the hot-wire and laser-Doppler anemometry. These methods

have now reached a state of development which allows their use in local ve-

locity measurements in laminar and turbulent ﬂows. In general, one applies

hot wire anemometry in gas ﬂows that are low in impurities, so that the

required calibration of the hot wire employed can be conserved over a long

measuring time. Reliable measurements are possible up to 10% turbulence in-

tensity. Flows with turbulence intensities above that require the application

of laser Doppler anemometry. This measuring method is also suitable for

measurements in impure gas and liquid ﬂows.

Finally, the rapid progress that has been achieved in the last few decades

in the ﬁeld of numerical ﬂuid mechanics should also be mentioned. Con-

siderable developments in applied mathematics took place to solve partial

diﬀerential equations numerically. In parallel, great improvements in the

computational performance of modern high-speed computers occurred and

computer programs became available that allow one to solve practical ﬂow