Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics
Подождите немного. Документ загружается.

Electron–Ion and Ion–Ion Recombination 54.3 Macroscopic Methods 805
and the recombination coefficient is
ˆ
α = k
c
P
S
=k
c
ρ
∗
ν
s
τ
d
= Kρ
∗
ν
s
. (54.44)
Microscopic Generalization
From (54.167), the microscopic generalizations of rate
(54.40) and probability (54.43c) are, respectively,
ˆ
α =
v
∞
0
εe
−ε
dε
b
0
0
2πb dbP
S
(ε, b; R
0
), (54.45a)
P
S
(ε, b; R
0
) =
R
0
R
i
ρ
i
(R)ν
b
i
(R) dt ≡
ρν
s
τ
d
,
(54.45b)
where ρ
i
(R) = n(ε, b; R)/
˜
n(ε, b; R); ν
(b)
i
is the fre-
quency (54.164a)of( A–B)–M continuum-bound
collisional transitions at fixed A–B separation R, R
i
is the pericenter of the orbit,
|
i
≡
|
ε, b
,and
b
2
0
= R
2
0
[
1−V(R)/E
]
,ε= E/k
B
T , (54.45c)
ˆ
α ≡ k
c
P
S
ε,b
, v =(8k
B
T/πM
AB
)
1/2
, (54.45d)
k
c
=
πR
2
0
[
1−V(R
0
)/k
B
T
]
v
. (54.45e)
where M
AB
is the reduced mass of A and B.
Low Gas Densities
Here ρ
i
(R) = 1forE > 0,
P
S
(ε, b; R
0
) =
R
0
R
i
ν(t) dt =
R
0
R
i
ds/λ
i
. (54.46)
λ
i
=(Nσ)
−1
is the microscopic path length towards the
(A–B)–M reactive collision with frequency ν = Nvσ .
For λ
i
constant, the rate (54.45a) reduces at low N to
ˆ
α = (vσ
0
N )
R
0
0
1−
V(R)
k
B
T
4πR
2
dR (54.47)
which is linear in the gas density N.
54.3.3 Working Formulae
for Three-Body Collisional
Recombination at Low Density
For three-body ion–ion collisional recombination of the
form A
+
+B
−
+M in a gas at low density N,setV(R) =
−e
2
/R .Then(54.47) yields
ˆ
α
c
(T ) =
8k
B
T
πM
AB
1/2
4
3
πR
3
0
1+
3
2
R
e
R
0
(σ
0
N ),
(54.48)
where R
e
=e
2
/k
B
T , and the trapping radius R
0
, deter-
mined by the classical variational method, is 0.41R
e
,in
agreement with detailed calculation. The special cases
are:
(a) e
−
+ A
+
+ e
−
Here, σ
0
=
1
9
πR
2
e
for ( e
−
− e
−
) collisions for scattering
angles θ ≥ π/2sothat
ˆ
α
c
ee
(T ) = 2.7×10
−20
300
T
4.5
n
e
cm
3
s
−1
(54.49)
in agreement with Mansbach and Keck [54.3].
(b) A
+
+ B
−
+ M
Here, σ
0
v ≈ 10
−9
cm
3
s
−1
, which is independent of T
for polarization attraction. Then
ˆ
α
3
(T ) = 2×10
−25
300
T
2.5
N cm
3
s
−1
. (54.50)
(c) e
−
+ A
+
+ M
Only a small fraction δ =2m/M of the electron’s en-
ergy is lost upon (e
−
−M ) collision so that (54.45a)for
constant λ is modified to
ˆ
α
eM
=σ
0
N
R
0
0
4πR
2
dR
E
m
0
˜
n(R, E)v dE
(54.51a)
=v
e
σ
0
N
R
0
0
4πR
2
dR
ε
m
0
1−
V(R)
E
εe
−ε
dε
(54.51b)
where ε = E/k
B
T ,andE
m
= δe
2
/R = ε
m
k
B
T is the
maximum energy for collisional trapping. Hence,
ˆ
α
eM
(T
e
) =4πδ
8k
B
T
e
πm
e
1/2
R
2
e
R
0
[
σ
0
N
]
(54.52a)
≈
10
−26
M
300
T
2.5
Ncm
3
s
−1
, (54.52b)
where the mass M of the gas atom is now in u.
This result agrees with the energy diffusion result of
Pitaevski˘ı [54.4]whenR
0
is taken as the Thomson radius
R
T
=
2
3
R
e
.
Part D 54.3
806 Part D Scattering Theory
54.3.4 Recombination
Influenced by Diffusional Drift
at High Gas Densities
Diffusional-Drift Current
The drift current of A
+
towards B
−
in a gas under an
A
+
–B
−
attractive potential V(R) is
J(R) =−D∇n(R) −
K
e
∇V(R)
n(R)
(54.53a)
=−
D
˜
N
A
˜
N
B
e
−V(R)/k
B
T
∂ρ
∂R
ˆ
R.
(54.53b)
Relative Diffusion and Mobility Coefficients
D = D
A
+D
B
,
K = K
A
+K
B
, De = K(k
B
T ), (54.54)
where the D
i
and K
i
are, respectively, the diffusion and
mobility coefficients of species i in gas M.
Normalized Ion-Pair R-Distribution
ρ(R) =
n(R)
˜
N
A
˜
N
B
exp
[
−V(R)/k
B
T
]
.
(54.55)
Continuity Equations for Currents and Rates
∂n
∂t
+∇· J =0 , R ≥ R
0
(54.56a)
ˆ
α
RN
(R
0
)ρ(R
0
) =
ˆ
αρ(∞) (54.56b)
The rate of reaction for ion-pairs with separations
R ≤ R
0
is α
RN
(R
0
).Thisistherecombinationrate
that would be obtained for a thermodynamic equilib-
rium distribution of ion pairs with R ≥ R
0
,i.e.for
ρ(R ≥ R
0
) =1.
Steady-State Rate of Recombination
ˆ
α
˜
N
A
˜
N
B
=
∞
R
0
∂n
∂t
dR =−4πR
2
0
J(R
0
). (54.57)
Steady-State Solution
ρ(R) = ρ(∞)
1−
ˆ
α
ˆ
α
TR
(R)
, R ≥ R
0
(54.58a)
ρ(R
0
) =ρ(∞)
ˆ
α/
ˆ
α
RN
(R
0
)
. (54.58b)
Recombination Rate
ˆ
α =
ˆ
α
RN
(R
0
)
ˆ
α
TR
(R
0
)
ˆ
α
RN
(R
0
) +
ˆ
α
TR
(R
0
)
(54.59a)
→
ˆ
α
RN
, N → 0
ˆ
α
TR
, N →∞.
(54.59b)
Diffusional-Drift Transport Rate
ˆ
α
TR
(R
0
) =4πD
∞
R
0
e
V(R)/k
B
T
R
2
dR
−1
. (54.60)
With V(R) =−e
2
/R ,
ˆ
α
TR
(R
0
) =4πKe
1−exp(−R
e
/R
0
)
−1
, (54.61)
where R
e
=e
2
/k
B
T provides a natural unit of length.
Langevin Rate
For R
0
R
e
, the transport rate
ˆ
α
TR
→
ˆ
α
L
=4πKe , (54.62)
tends to the Langevin rate which varies as N
−1
.
Reaction Rate
When R
0
is large enough that R
0
-pairs are in
E, L
2
equilibrium (54.167),
ˆ
α
RN
(R
0
) =v
∞
0
εe
−ε
dε
b
0
0
2πb dbP
S
(ε, b; R
0
)
(54.63a)
≡v
∞
0
εe
−ε
dε
πb
2
0
P
S
(ε; R
0
)
(54.63b)
≡vπb
2
max
P
S
(R
0
), (54.63c)
where
b
2
0
= R
2
0
[
1−V(R
0
)/E
]
,ε= E/k
B
T , (54.64a)
v = (8kT/πM
AB
)
1/2
, (54.64b)
b
2
max
= R
2
0
1−
V(R
0
)
k
B
T
.
(54.64c)
The probability P
S
and its averages over b and (b, E)
for reaction between pairs with R ≤ R
0
is determined in
(54.63a–c) from solutions of coupled master equations.
P
S
increases linearly with N initially and tends to unity
Part D 54.3

Electron–Ion and Ion–Ion Recombination 54.4 Dissociative Recombination 807
at high N. The recombination rate (54.59a) with (54.63a)
and (54.61) therefore increases linearly with N initially,
reaches a maximum when
ˆ
α
TR
≈
ˆ
α
RN
and then decreases
eventually as N
−1
, in accord with (i).
Reaction Probability
The classical absorption solution of (54.157)is
P
S
(E, b; R
0
) =1−exp
−
R
0
R
i
ds
i
λ
i
.
(54.65)
With the binary decomposition λ
−1
i
=λ
−1
iA
+λ
−1
iB
,
P
S
= P
A
+ P
B
− P
A
P
B
. (54.66)
Exact b
2
–Averaged Probability
With V
c
=−e
2
/R for the A
+
–B
−
interaction in
(54.63b), and at low gas densities N,
P
A,B
(E, R
0
) =
4R
0
3λ
A,B
1−
3V
c
(R
0
)
2E
i
[
1−V
c
(R
0
)/E
i
]
(54.67)
appropriate for constant mean free path λ
i
.
(E,b
2
)–Averaged Probability
P
S
(R
0
) in (54.63c) at low gas density is
P
A,B
(R
0
) = P
A,B
(E = k
B
T, R
0
). (54.68)
Thomson Trapping Distance
When the kinetic energy gained from Coulomb attrac-
tion is assumed lost upon collision with third bodies,
then bound A −B pairs are formed with R ≤ R
T
.Since
E =
3
2
k
B
T −e
2
/R ,then
R
T
=
2
3
e
2
k
B
T
=
2
3
R
e
. (54.69)
Thomson Straight-Line Probability
The E →∞limit of (54.65)is
P
T
A,B
(b; R
T
) =1−exp
−2
R
2
T
−b
2
/λ
A,B
.
(54.70)
The b
2
-average is the Thomson probability
P
T
A,B
(R
T
) =1−
1
2X
2
1− e
−2X
(1+2X)
(54.71a)
for reaction of (A −B) pairs with R ≤ R
T
.AsN → 0
P
T
A,B
(R
T
) →
4
3
X
1−
3
4
X +
2
5
X
2
−
1
6
X
3
+···
(54.71b)
and tends to unity at high N. X = R
T
/λ
A,B
= N(σ
0
R
T
).
These probabilites have been generalized [54.5]toin-
clude hyperbolic and general trajectories.
Thomson Reaction Rate
ˆ
α
T
=πR
2
T
v
P
T
A
+ P
T
B
− P
T
A
P
T
B
→
4
3
πR
3
T
λ
−1
A
+λ
−1
B
, N → 0
πR
2
T
v, N →∞.
(54.72)
54.4 Dissociative Recombination
54.4.1 Curve-Crossing Mechanisms
Direct Process.
Dissociative recombination (DR) for diatomic ions can
occur via a crossing at R
X
between the bound and repul-
sive potential energy curves V
+
(R) and V
d
(R) for AB
+
and AB
∗∗
, respectively. Here, DR involves the two-stage
sequence
e
−
+ AB
+
(v
i
)
k
c
ν
a
(AB
∗∗
)
R
ν
d
−→ A + B
∗
. (54.73)
The first stage is dielectronic capture whereby the free
electron of energy ε = V
d
(R) −V
+
(R) excites an elec-
tron of the diatomic ion AB
+
with internal separation R
and is then resonantly captured by the ion, at rate k
c
,to
form a repulsive state d of the doubly excited molecule
AB
∗∗
, which in turn can either autoionize at probability
frequency ν
a
, or else in the second stage predissoci-
ate into various channels at probability frequency ν
d
.
This competition continues until the (electronically ex-
cited) neutral fragments accelerate past the crossing
at R
X
. Beyond R
X
the increasing energy of relative sep-
aration reduces the total electronic energy to such an
extent that autoionization is essentially precluded and
the neutralization is then rendered permanent past the
stabilization point R
X
. This interpretation [54.6]hasre-
mained intact and robust in the current light of ab initio
quantum chemistry and quantal scattering calculations
for the simple diatomics
O
+
2
,N
+
2
,Ne
+
2
,etc.
. Mechan-
ism (54.73) is termed the direct process which, in terms
Part D 54.4

808 Part D Scattering Theory
of the macroscopic frequencies in (54.73), proceeds at
the rate
ˆ
α = k
c
P
S
=k
c
[
ν
d
/(ν
a
+ν
d
)
]
, (54.74)
where P
S
is probability for A − B
∗
survival against
autoionization from the initial capture at R
c
to the cross-
ing point R
X
. Configuration mixing theories of this
direct process are available in the quantal [54.7]and
semiclassical-classical path formulations [54.8].
Indirect Process
In the three-stage sequence
e
−
+ AB
+
(v
+
i
) →
AB
+
(v
f
) − e
−
n
→(AB
∗∗
)
d
→ A +B
∗
(54.75)
the so-called indirect process [54.7] might contribute.
Here the accelerating electron loses energy by vibra-
tional excitation
v
+
i
→v
f
of the ion and is then
resonantly captured into a Rydberg orbital of the bound
molecule AB
∗
in vibrational level v
f
, which then inter-
acts one way (via configuration mixing) with the doubly
excited repulsive molecule AB
∗∗
. The capture initially
proceeds via a small effect – vibronic coupling (the ma-
trix element of the nuclear kinetic energy) induced by the
breakdown of the Born-Oppenheimer approximation –
at certain resonance energies ε
n
= E(v
f
) −E
v
+
i
and,
in the absence of the direct channel (54.73), would there-
fore be manifest by a series of characteristic very narrow
Lorentz profiles in the cross section. Uncoupled from
(54.73) the indirect process would augment the rate. Vi-
bronic capture proceeds more easily when v
f
=v
+
i
+1
so that Rydberg states with n ≈ 7−9 would be involved
for H
+
2
v
+
i
= 0
so that the resulting longer periods of
the Rydberg electron would permit changes in nuclear
motion to compete with the electronic dissociation. Re-
combination then proceeds as in the second stage of
(54.73), i. e., by electronic coupling to the dissociative
state d at the crossing point. A multichannel quantum
defect theory [54.9] has combined the direct and indirect
mechanisms
Interrupted Recombination
The process
e
−
+ AB
+
(v
i
)
k
c
ν
a
(AB
∗∗
)
d
ν
d
→ A +B
∗
ν
nd
ν
dn
AB
+
(v) − e
−
n
(54.76)
proceeds via the first (dielectronic capture) stage of
(54.73) followed by a two-way electronic transition
with frequency ν
dn
and ν
nd
between the d and n states.
All (n,v)Rydberg states can be populated, particularly
those in low n and high v since the electronic d −n inter-
action varies as n
−1.5
with broad structure. Although
the dissociation process proceeds here via a second or-
der effect (ν
dn
and ν
nd
), the electronic coupling may
dominate the indirect vibronic capture and interrupt the
recombination, in contrast to (54.75) which, as written
in the one-way direction, feeds the recombination. Both
dip and spike structure has been observed [54.10].
54.4.2 Quantal Cross Section
The cross section for direct dissociative recombination
e
−
+ AB
+
v
+
i
AB
∗∗
r
−→ A +B
∗
(54.77)
of electrons of energy ε, wavenumber k
e
and spin
statistical weight 2, for a molecular ion AB
+
(v
+
i
) of
electronic statistical weight ω
+
AB
in vibrational level v
+
i
is
σ
DR
(ε) =
π
k
2
e
ω
∗
AB
2ω
+
a
Q
2
=
h
2
8πm
e
ε
ω
∗
AB
2ω
+
a
Q
2
. (54.78)
Here ω
∗
AB
is the electronic statistical weight of the dis-
sociative neutral state of AB
∗
whose potential energy
curve V
d
crosses the corresponding potential energy
curve V
+
of the ionic state. The transition T-matrix
element for autoionization of AB
∗
embedded in the
(moving) electronic continuum of AB
+
+ e
−
is the
quantal probability amplitude
a
Q
(v) = 2π
∞
0
V
∗
dε
(R)
ψ
+∗
v
(R)ψ
d
(R)
dR
(54.79)
for autoionization. Here ψ
+
v
and ψ
d
are the nuclear
bound and continuum vibrational wave functions for
AB
+
and AB
∗
, respectively, while
V
dε
(R) =φ
d
|H
el
(r, R(t))|φ
ε
(r, R)
r,
ˆ
ε
= V
∗
εd
(R) (54.80)
are the bound-continuum electronic matrix elements
coupling the diabatic electronic bound state wave func-
tions ψ
d
(r, R) for AB
∗
with the electronic continuum
state wave functions φ
ε
(r, R) for AB
+
+ e
−
. The matrix
element is an average over electronic coordinates r and
Part D 54.4
Electron–Ion and Ion–Ion Recombination 54.4 Dissociative Recombination 809
all directions
ˆ
of the continuum electron. Both con-
tinuum electronic and vibrational wave functions are
energy normalized (Sect. 54.8.3), and
Γ(R) = 2π
V
∗
dε
(R)
2
(54.81)
is the energy width for autoionization at a given nu-
clear separation R.GivenΓ(R) from quantum chemistry
codes, the problem reduces to evaluation of continuum
vibrational wave functions in the presence of autoioniza-
tion. The rate associated with a Maxwellian distribution
of electrons at temperature T is
ˆ
α =
v
e
εσ
DR
(ε) e
−ε/k
B
T
dε/(k
B
T )
2
(54.82)
where v
e
is the mean speed (Sect. 54.9).
Maximum Cross Section and Rate
Since the probability for recombination must remain less
than unity,
a
Q
2
≤1 so that the maximum cross section
and rates are
σ
max
DR
(ε) =
π
k
2
e
ω
∗
AB
2ω
+
=
h
2
8πm
e
ε
(2 +1),
(54.83)
where ω
∗
AB
has been replaced by 2(2 +1)ω
+
under the
assumption that the captured electron is bound in a high
level Rydberg state of angular momentum ,and
ˆ
α
max
(T ) = v
e
σ
max
DR
(ε =k
B
T ) (54.84a)
≈5×10
−7
300
T
1/2
(2 +1) cm
3
/s .
(54.84b)
Cross section maxima of 5(2 +1)(300/T ) ×10
−14
cm
2
are therefore possible, being consistent with the rate
(54.84b).
First-Order Quantal Approximation
When the effect of autoionization on the continuum vi-
brational wave function ψ
d
(R) for AB
∗
is ignored, then
a first-order undistorted approximation to the quantal
amplitude (54.79)is
T
B
(v
+
) =2π
∞
0
V
∗
dε
(R)
ψ
+∗
v
(R)ψ
(0)
d
(R)
dR
(54.85)
where ψ
(0)
d
is ψ
d
in the absence of the back reaction of
autoionization. Under this assumption, (54.78) reduces
to
σ
c
(ε, v
+
) =
π
k
2
e
ω
∗
AB
2ω
+
T
B
v
+
2
, (54.86)
which is then the cross section for initial electron cap-
ture since autoionization has been precluded. Although
the Born T -matrix (54.85) violates unitarity, the cap-
ture cross section (54.86) must remain less then the
maximum value
σ
max
c
=
π
k
2
e
ω
∗
AB
2ω
+
=
h
2
8πm
e
ε
ω
∗
AB
2ω
+
,
(54.87)
since
a
Q
2
≤1. So as to acknowledge after the fact the
effect of autoionization, assumed small, and neglected
by (54.85), the DR cross section can be approximated
as
σ
DR
ε, v
+
=σ
c
ε, v
+
P
S
, (54.88)
where P
S
is the probability of survival against autoion-
ization on the V
d
curve until stabilization takes place at
some crossing point R
X
.
Approximate Capture Cross Section
With the energy-normalized Winans–Stückelberg vibra-
tional wave function
ψ
(0)
d
(R) =
V
d
(R)
−1/2
δ(R − R
c
), (54.89)
where R
c
is the classical turning point for (A − B
∗
)
relative motion, (54.86) reduces to
σ
c
(ε, v
+
) =
π
k
2
e
ω
∗
AB
2ω
+
[
2πΓ(R
c
)
]
ψ
+
v
(R
c
)
2
|V
d
(R
c
)|
!
(54.90)
where the term inside the braces in (54.90) is the effec-
tive Franck–Condon factor.
Six Approximate Stabilization Probabilities
(1) A unitarized T -matrix is
T =
T
B
1+
1
2
T
B
2
, (54.91)
so that P
S
=
|
T
|
2
/
|
T
B
|
2
to give
P
S
(low ε)
=
1+
1
4
|
T
B
|
2
−2
=
1+π
2
∞
0
V
∗
dε
(R)
ψ
+∗
v
(R)ψ
(0)
d
(R)
dr
2
−2
(54.92a)
Part D 54.4
810 Part D Scattering Theory
which is valid at low ε when only one vibrational
level v
+
, i. e., the initial level of the ion is repopulated
by autoionization.
(2) At higher ε, when population of many other ionic
levels v
+
f
occurs, then
P
S
(ε) =
1+
1
4
f
T
B
v
+
f
2
−2
, (54.92b)
where the summation is over all the open vibrational
levels v
+
f
of the ion. When no intermediate Rydberg
AB
∗
(v) states are energy resonant with the initial
e
−
+ AB
+
v
+
state, i. e., coupling with the indirect
mechanism is neglected, then (54.88) with (54.92b)is
the direct DR cross section normally calculated.
(3) In the high-ε limit when an infinite number of
v
+
f
levels are populated following autoionization, the
survival probability, with the aid of closure, is then
P
S
=
1+π
2
R
X
R
c
V
∗
dε
(R)
2
ψ
(0)
d
(R)
2
dR
−2
.
(54.93)
(4) On adopting in (54.93) the JWKB semiclassical
wave function for ψ
(0)
d
,then
P
S
(high ε) =
1+
1
2
R
X
R
c
Γ(R)
v(R)
dR
−2
=
1+
1
2
t
X
t
c
ν
a
(t) dt
−2
, (54.94)
where v(R) is the local radial speed of A −B relative
motion, and where the frequency ν
a
(t) of autoionization
is Γ/
.
(5) A classical path local approximation for P
S
yields
P
S
=exp
−
t
X
t
c
ν
a
(t) dt
, (54.95)
which agrees to first-order for small ν with the expansion
of (54.94).
(6) A partitioning of (54.73) yields
P
S
=ν
d
/(ν
a
+ν
d
) =(1+ν
a
τ
d
)
−1
, (54.96)
on adopting macroscopic averaged frequencies ν
i
and
associated lifetimes τ
i
= ν
−1
i
. The six surivival prob-
abilities in (54.92a,b), (54.93–54.96) are all suitable for
use in the DR cross section (54.88).
54.4.3 Noncrossing Mechanism
The dissociative recombination (DR) processes
e
−
+H
+
3
→H
2
+H
→H+H +H
(54.97)
at low electron energy ε,and
e
−
+HeH
+
→He+H(n =2) (54.98)
have spurred renewed theoretical interest because they
both proceed at respective rates of
2×10
−7
to 2×10
−8
cm
3
s
−1
and 10
−8
cm
3
s
−1
at 300 K. Such rates are gen-
erally associated with the direct DR, which involves
favorable curve crossings between the potential energy
surfaces, V
+
(R) and V
d
(R) for the ion AB
+
and neu-
tral dissociative AB
∗∗
states. The difficulty with (54.97)
and (54.98) is that there are no such curve crossings,
except at ε ≥ 8eVfor(54.97). In this instance, the pre-
vious standard theories would support only extremely
small rates when electronic resonant conditions do not
prevail at thermal energies. Theories [54.11,12]arecur-
rently being developed for application to processes such
as (54.97).
54.5 Mutual Neutralization
A
+
+B
−
→ A +B . (54.99)
Diabatic Potentials
V
(0)
i
(R) and V
(0)
f
(R) for initial (ionic) and final (cova-
lent) states are diagonal elements of
V
if
(R)=Ψ
i
(r, R)|H
el
(r, R)|Ψ
f
(r, R)
r
, (54.100)
where Ψ
i, f
are diabatic states and H
el
is the electronic
Hamiltonian at fixed internuclear distance R.
Adiabatic Potentials for a Two-State System
V
±
(R) = V
0
(R) ±
∆
2
(R) +
V
if
(R)
2
1/2
,
(54.101a)
V
0
(R) =
1
2
V
(0)
i
(R) +V
(0)
f
(R)
, (54.101b)
∆(R) =
V
(0)
i
(R) −V
(0)
f
(R)
. (54.101c)
Part D 54.5
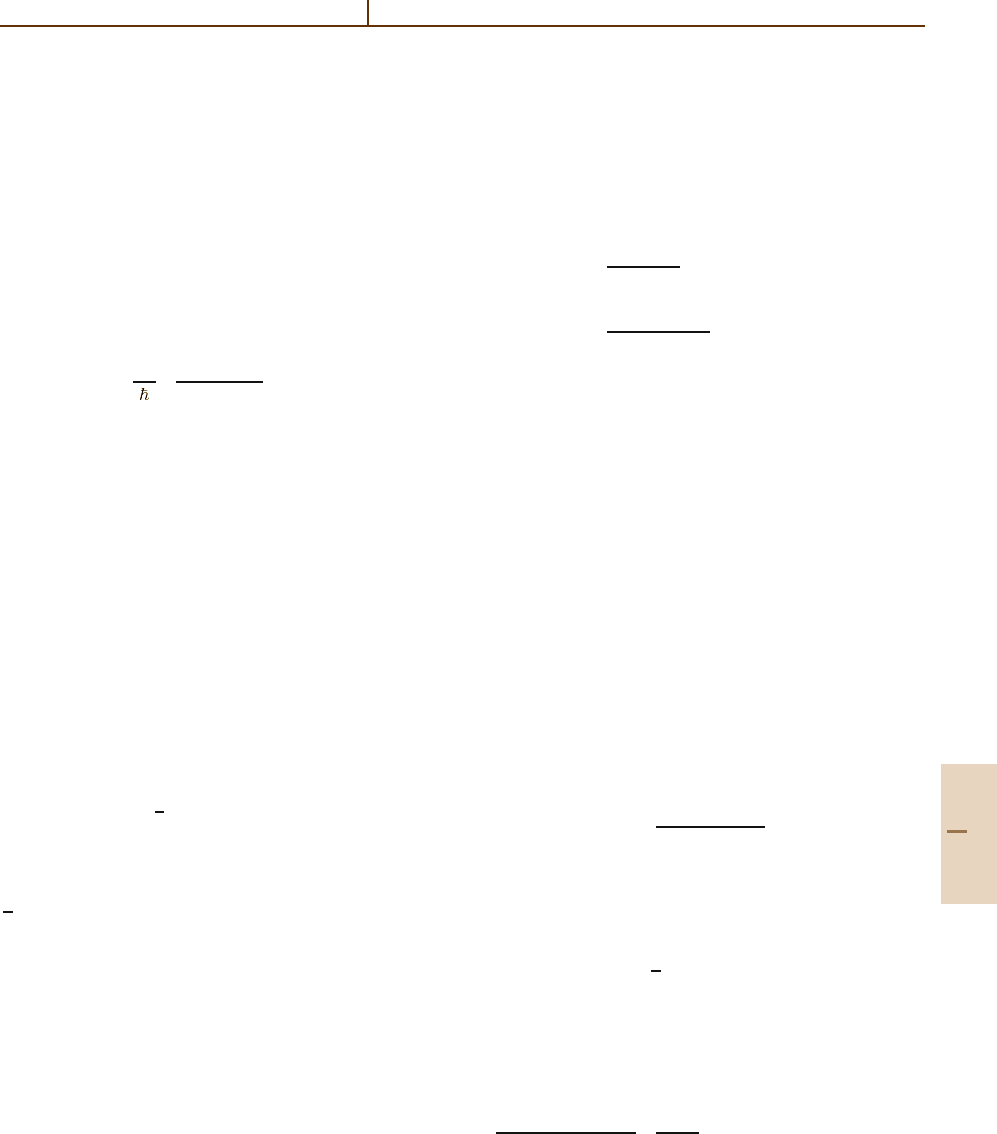
Electron–Ion and Ion–Ion Recombination 54.6 One-Way Microscopic Equilibrium Current, Flux, and Pair-Distributions 811
For a single crossing of diabatic potentials at R
X
then
V
(0)
i
(R
X
) = V
(0)
f
(R
X
) and the adiabatic potentials at R
X
are,
V
±
(R
X
) = V
(0)
i
(R
X
) ±V
if
(R
X
) (54.102)
with energy separation 2V
if
(R
X
).
54.5.1 Landau–Zener Probability for Single
Crossing at R
X
On assuming ∆(R) =(R −R
X
)∆
(R
X
), where ∆
(R) =
d∆(R)/ dR, the probability for single crossing is
P
if
(R
X
) =exp
[
η(R
X
)/v
X
(b)
]
(54.103a)
η(R
X
) =
2π
V
if
(R
X
)
2
∆
(R
X
)
(54.103b)
v
X
(b) =
1−V
(0)
i
(R
X
)/E −b
2
/R
2
X
1/2
.
(54.103c)
Overall Charge-Transfer Probability
From the incoming and outgoing legs of the trajectory,
P
X
(E) = 2P
if
(1−P
if
). (54.104)
54.5.2 Cross Section and Rate Coefficient
for Mutual Neutralization
σ
M
(E) = 4π
b
X
0
P
if
(1−P
if
)b db
= πb
2
X
P
M
, (54.105a)
πb
2
X
= π
1−
V
(0)
i
(R
X
)
E
R
2
X
= π
1+
14.4
R
X
Å
E(eV )
R
2
X
. (54.105b)
P
M
is the b
2
-averaged probability (54.104) for charge-
transfer reaction within a sphere of radius R
X
.
The rate is
ˆ
α
M
=(8k
B
T/πM
AB
)
1/2
∞
0
σ
M
() e
−
d (54.106)
where = E/k
B
T .
54.6 One-Way Microscopic Equilibrium Current, Flux,
and Pair-Distributions
Notation:
M reduced mass M
A
M
B
/(M
A
+M
B
)
R internal separation of A −B
E orbital energy
1
2
Mv
2
+V(R)
L orbital angular momentum
L
2
2MEb
2
for E > 0
v
R
radial speed |
˙
R|
v mean relative speed (8kT/πM
AB
)
1/2
ε normalized energy E/k
B
T
n
i
pair distribution function n
+
i
+n
−
i
n
±
i
component of n
i
with
˙
R > 0 (+) and
˙
R < 0 (−).
All quantities on the RHS in the expressions (a)–(e)
below are to be multiplied by
˜
N
A
˜
N
B
[
ω
AB
/ω
A
ω
B
]
where the ω
i
denote the statistical weights of
species i which are not included by the density of
states associated with the E, L
2
orbital degrees of
freedom.
Case (a).
|
i
≡
R, E, L
2
.
Current: j
±
i
(R) = n
±
(R, E, L
2
)v
R
≡n
±
i
v
R
Flux: 4πR
2
j
±
i
(R) dE dL
2
=
4π
2
e
−E/k
B
T
(2πMk
B
T )
3/2
dE dL
2
.
(54.107)
This flux is independent of R. For dissociated pairs
E > 0,
4πR
2
j
±
i
(R) dE dL
2
=
vεe
−ε
dε
[
2πbdb
]
.
(54.108)
R, E, L
2
-Distribution:
n
R, E, L
2
dRdE dL
2
=
8π
2
/v
R
e
−E/k
B
T
(2πMk
B
T )
3/2
dR
4πR
2
dE dL
2
. (54.109)
Part D 54.6
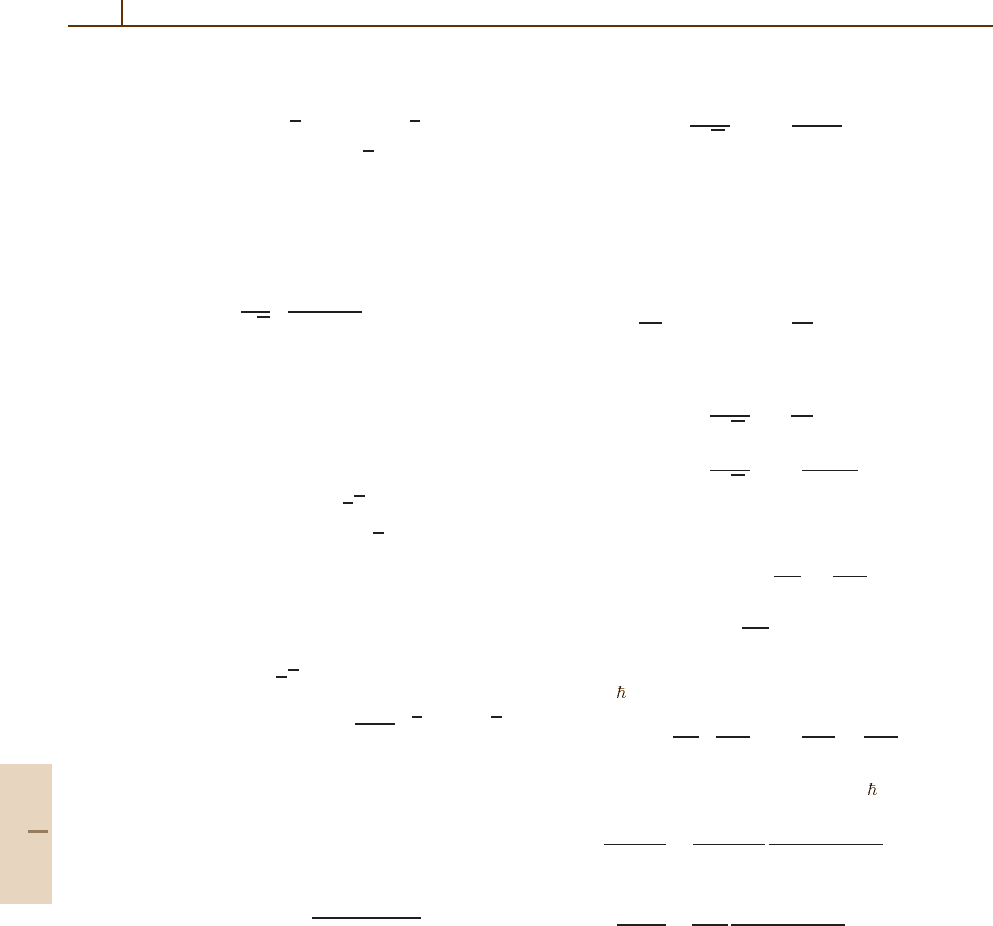
812 Part D Scattering Theory
Case (b).
|
i
≡
|
R, E
; L
2
-integrated quantities.
Current: j
±
i
(R) =
1
2
vn
±
(R, E) ≡
1
2
vn
±
i
, (54.110)
Flux: 4πR
2
j
±
i
(R) dE =
vεe
−ε
dε
πb
2
0
,
(54.111a)
πb
2
0
=πR
2
[
1−V(R)/E
]
,
(54.111b)
(R, E)-Distribution:
n(R, E) dRdE
=
2
√
π
E −V(R)
k
B
T
1/2
e
−ε
dεdR
≡ G
MB
(E, R)dR, (54.112)
which defines the Maxwell–Boltzmann velocity vistri-
bution G
MB
in the presence of the field V(R).
Case (c).
E, L
2
-integrated quantities.
Current: j
±
(R) =
1
4
v e
−V(R)/k
B
T
, (54.113)
Flux: 4πR
2
j
±
(R) = πR
2
v e
−V(R)/k
B
T
, (54.114)
Distribution: n(R) = e
−V(R)/k
B
T
. (54.115)
When E-integration is only over dissociated states
(E > 0), the above quantities are
j
±
d
(R) =
1
4
v
[
1−V(R)/k
B
T
]
, (54.116)
4πR
2
j
±
d
(R) = πR
2
1−
V(R)
k
B
T
v ≡ πb
2
max
v,
(54.117)
n(R) =
[
1−V(R)/k
B
T
]
. (54.118)
Case (d).
E, L
2
-distribution. For Bound Levels
n(E, L
2
)dE dL
2
=
4π
2
τ
R
(E, L)
(2πMk
B
T )
3/2
e
−E/k
B
T
dE dL
2
,
(54.119)
where τ
R
=
,
dt = (∂J
R
/∂E) is the period for bounded
radial motion of energy E and radial action
J
R
(E, L) = M
,
v
R
dR.
Case (e). E-distribution. For bound levels
n(E)dE =
2e
−ε
√
π
dε
R
A
0
E −V
k
B
T
1/2
dR , (54.120)
where R
A
is the turning point E = V(R
A
).
Example. For electron–ion bounded motion, V(R) =
−Ze
2
/R , R
A
= Ze
2
/|E|, R
e
= Ze
2
/k
B
T , ε = E/k
B
T .
Then τ
R
=2π(m/Ze
2
)
1/2
(R
A
/2)
3/2
,
R
A
0
R
e
R
−
|
ε
|
1/2
dR =
π
2
4
R
5/2
A
R
1/2
e
, (54.121)
and
n
s
(E) dE =
2e
−ε
√
π
dε
π
2
4
R
5/2
A
R
1/2
e
(54.122)
=
2e
−ε
√
π
dε
π
2
R
3
e
4
|
ε
|
5/2
.
(54.123)
For closely spaced levels in a hydrogenic e
−
− A
Z+
system,
n
s
( p,)= n
E, L
2
dE
dp
dL
2
d
(54.124a)
n
s
( p) = n(E)
dE
dp
.
(54.124b)
Using E =−
2p
2
−1
Z
2
e
2
/a
0
and L
2
= ( +
1/2)
2 2
for level ( p,)then
τ
R
(E, L)
dE
dp
dL
2
d
=
dJ
R
dp
dL
2
d
(54.125)
= h
(2 +1)
2
(54.126)
n
s
( p,)
n
e
N
+
=
2(2 +1)
2ω
+
A
h
3
(2πm
e
k
B
T )
3/2
e
I
p
/k
B
T
,
(54.127a)
n
s
( p)
n
e
N
+
=
2p
2
2ω
+
A
h
3
(2πm
e
k
B
T )
3/2
e
I
p
/k
B
T
, (54.127b)
in agreement with the Saha ionization formula (54.16)
where N
+
is the equilibrium concentration of A
Z+
ions
in their ground electronic states. The spin statistical
weights are ω
eA
= ω
e
=2.
54.7 Microscopic Methods for Termolecular Ion–Ion Recombination
At low gas density, the basic process
A
+
+B
−
+M → AB +M (54.128)
is characterized by nonequilibrium with respect to E.
Dissociated and bound A
+
–B
−
ion pairs are in equilib-
rium with respect to their separation R, but bound pairs
Part D 54.7

Electron–Ion and Ion–Ion Recombination 54.7 Microscopic Methods for Termolecular Ion–Ion Recombination 813
are not in E-equilibrium with each other. L
2
-equilibrium
can be assumed for ion–ion recombination but not for
ion–atom association reactions.
At higher gas densities N, there is nonequilibrium
in the ion-pair distributions with respect to R, E and L
2
.
In the limit of high N, there is only nonequilibrium with
respect to R. See [54.13] and the appropriate reference
list for full details of theory.
54.7.1 Time Dependent Method:
Low Gas Density
Energy levels E
i
of A
+
–B
−
pairs are so close that they
form a quasicontinuum with a nonequilibrium distribu-
tion over E
i
determined by the master equation
dn
i
(t)
dt
=
∞
−D
n
i
ν
if
−n
f
ν
fi
dE
f
, (54.129)
where n
i
dE
i
is the number density of pairs in the interval
dE
i
about E
i
,andν
if
dE
f
is the frequency of i-pair
collisions with M that change the i-pair orbital energy
from E
i
to between E
f
and E
f
+ dE
f
. The greatest
binding energy of the A
+
–B
−
pair is D.
Association Rate
R
A
(t) =
∞
−D
P
S
i
dn
i
dt
dE
i
(54.130a)
=
ˆ
αN
A
(t)N
B
(t) −kn
s
(t), (54.130b)
where P
S
i
is the probability for collisional stabiliza-
tion (recombination) of i-pairs via a sequence of energy
changing collisions with M. The coefficients for C →
recombination out of the C-block with ion concentra-
tions N
A
(t), N
B
(t) (in cm
−3
) into the block of total
ion-pair concentrations n
s
(t) and for →C dissociation
are
ˆ
α
cm
3
s
−1
and k
s
−1
, respectively.
One-Way Equilibrium Collisional Rate
and Detailed Balance
C
if
=
˜
n
i
ν
if
=
˜
n
f
ν
fi
=C
fi
, (54.131)
where the tilde denotes equilibrium (Saha) distributions.
Normalized Distribution Functions
γ
i
(t) =n
i
(t)/
˜
n
S
i
,γ
s
(t) =n
s
(t)/
˜
n
B
s
(t), (54.132)
γ
c
(t) = N
A
(t)N
B
(t)/
˜
N
A
˜
N
B
, (54.133)
where
˜
n
S
i
and
˜
n
B
are the Saha and Boltzmann distribu-
tions.
Master Equation for γ
i
(t)
dγ
i
(t)
dt
=−
∞
−D
γ
i
(t) −γ
f
(t)
ν
if
dE
f
. (54.134)
Quasi-Steady State (QSS) Reduction
Set
γ
i
(t) = P
D
i
γ
c
(t) + P
S
i
γ
s
(t)
t→∞
−→ 1 (54.135)
where P
D
i
and P
S
i
are the respective time-independent
portions of the normalized distribution γ
i
which ori-
ginate, respectively, from blocks C and . The energy
separation between the C and blocks is so large
that P
S
i
= 0 (E
i
≥ 0, C block), P
S
i
≤ 1(0> E
i
≥−S,
E block), P
S
i
= 1(−S ≥ E
i
≥−D, block). Since
P
S
i
+ P
D
i
=1, then
dγ
i
(t)
dt
=−
[
γ
c
(t) −γ
s
(t)
]
∞
−D
P
D
i
− P
D
f
C
if
dE
f
.
(54.136)
Recombination and Dissociation Coefficients
Equation (54.135)in(54.130a) enables the recombin-
ationratein(54.130b) to be written as
ˆ
α
˜
N
A
˜
N
B
=
∞
−D
P
D
i
dE
i
∞
−D
P
D
i
− P
D
f
C
if
dE
f
.
(54.137)
The QSS condition (dn
i
/dt = 0inblockE )isthen
P
D
i
∞
−D
ν
if
dE
f
=
E
−D
ν
if
P
D
f
dE
f
, (54.138)
which involves only time independent quantities. Under
QSS,(54.137) reduces to the net downward current
across bound level −E,
ˆ
α
˜
N
A
˜
N
B
=
∞
−E
dE
i
−E
−D
P
D
i
− P
D
f
C
if
dE
f
,
(54.139)
which is independent of the energy level (−E)inthe
range 0 ≥−E ≥−S of block E .
The dissociation frequency k in (54.130b)is
k
˜
n
s
=
−E
−D
dE
i
∞
−E
P
S
i
− P
S
f
C
if
dE
f
, (54.140)
Part D 54.7
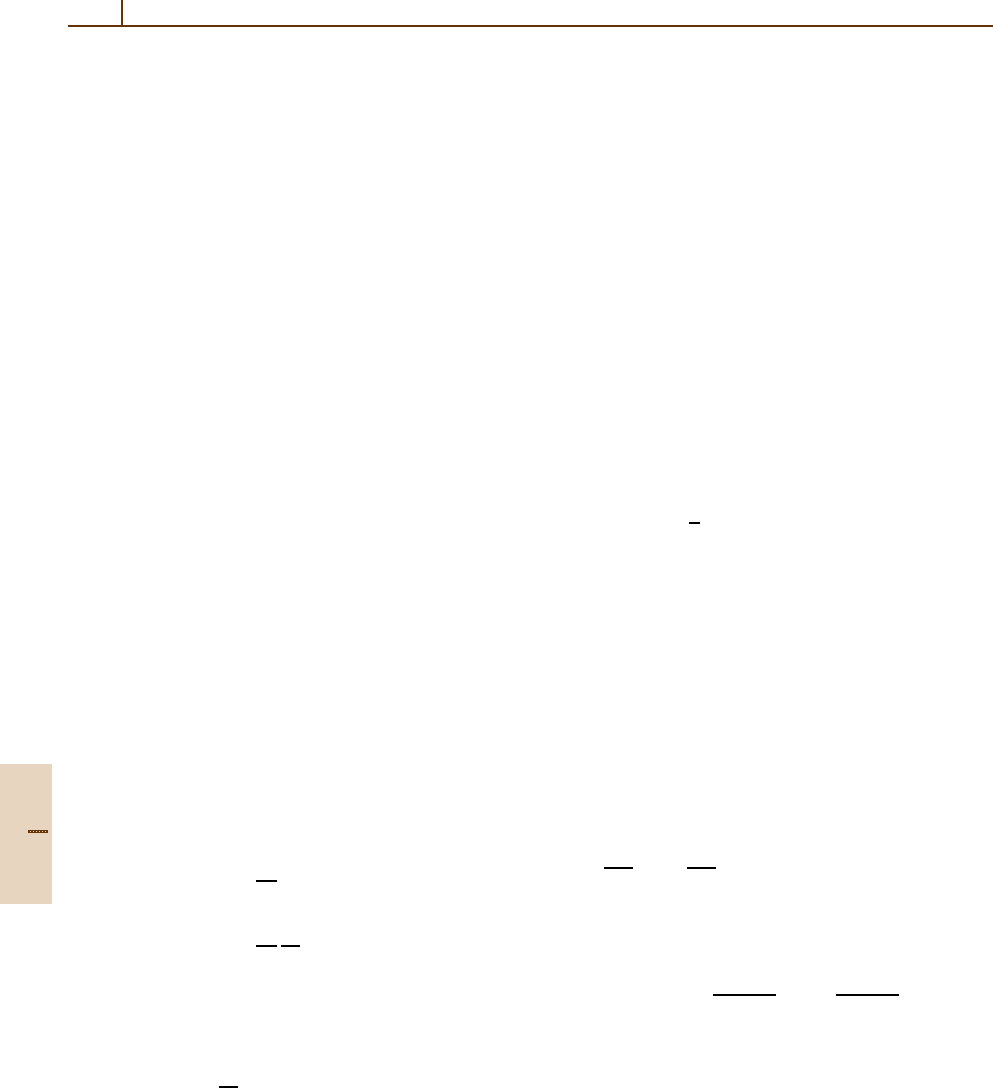
814 Part D Scattering Theory
and macroscopic detailed balance
ˆ
α
˜
N
A
˜
N
B
=k
˜
n
s
is
automatically satisfied.
ˆ
α is the direct (C →) colli-
sional contribution(small) plus the (much larger) net col-
lisional cascade downward contribution from that frac-
tion of bound levels which originated in the continuum
C. k
d
is the direct dissociation frequency (small) plus the
net collisional cascade upward contribution from that
fraction of bound levels which originated in block .
54.7.2 Time Independent Methods:
Low Gas Density
QSS-Rate. Since recombination and dissociation (ion-
ization) involve only that fraction of the bound state
population which originated from the C and blocks,
respectivel,y recombination can be viewed as time inde-
pendent with
N
A
N
B
=
˜
N
A
˜
N
B
, n
s
(t) =0 , (54.141a)
ρ
i
=n
i
/
˜
n
i
≡ P
D
i
(54.141b)
ˆ
α
˜
N
A
˜
N
B
=
∞
−E
dE
i
−E
−D
ρ
i
−ρ
f
C
if
dE
f
. (54.141c)
QSS Integral Equation.
ρ
i
∞
−D
ν
if
dE
f
=
∞
−S
ρ
f
ν
if
dE
f
(54.142)
is solved subject to the boundary condition
ρ
i
=1(E
i
≥0), ρ
i
= 0(−S ≥ E
i
≥−D).
(54.143)
Collisional Energy-Change Moments.
D
(m)
(E
i
) =
1
m!
∞
−D
(E
f
−E
i
)
m
C
if
dE
f
, (54.144)
D
(m)
i
=
1
m!
d
dt
(∆E)
m
.
(54.145)
Averaged Energy-Change Frequency. For an equilib-
rium distribution
˜
n
i
of E
i
-pairs per unit interval dE
i
per
second,
D
(1)
i
=
d
dt
∆E
.
Averaged Energy-Change per Collision.
∆E=D
(1)
i
/D
(0)
i
.
Time Independent Dissociation. The time independent
picture corresponds to
n
s
(t) =
˜
n
s
,γ
c
(t) =0,ρ
i
=n
i
/
˜
n
i
≡ P
S
i
,
(54.146)
in analogy to the macroscopic reduction of (54.38a,b).
Variational Principle
The QSS-condition (54.135) implies that the fraction P
D
i
of bound levels i with precursor C are so distributed
over i that (54.137)for
ˆ
α is a minimum. Hence P
D
i
or ρ
i
are obtained either from the solution of (54.142) or from
minimizing the variational functional
ˆ
α
˜
N
A
˜
N
B
=
∞
−D
n
i
dE
i
∞
−D
ρ
i
−ρ
f
ν
if
dE
f
(54.147a)
=
1
2
∞
−D
dE
i
∞
−D
ρ
i
−ρ
f
2
C
if
dE
f
(54.147b)
with respect to variational parameters contained in a trial
analytic expression for ρ
i
. Minimization of the quadratic
functional (54.147b) has an analogy with the prin-
ciple of least dissipation in the theory of electrical
networks.
Diffusion-in-Energy-Space Method
Integral Equation (54.142) can be expanded in terms of
energy-change moments, via a Fokker–Planck analysis
to yield the differential equation
∂
∂E
i
D
(2)
i
∂ρ
i
∂E
i
=0 ,
(54.148)
with the QSS analytical solution
ρ
i
(E
i
) =
0
E
i
dE
D
(2)
(E)
0
−S
dE
D
(2)
(E)
−1
(54.149)
of Pitaevski˘ı [54.4]for(e
−
+ A
+
+M) recombination
where collisional energy changes are small. This distri-
bution does not satisfy the exact QSS condition (54.142).
When inserted in the exact non-QSS rate (54.147b),
highly accurate
ˆ
α for heavy-particle recombination are
obtained.
Part D 54.7
