Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics

Подождите немного. Документ загружается.

Electron–Atom, Electron–Ion, and Electron–Molecule Collisions 47.1 Electron–Atom and Electron–Ion Collisions 711

then we can show from (47.50) that in the neighborhood

of the resonance

sum

(

)

= δ

0,sum

(

)

+ tan

−1



i,r

− E



(47.54)

where δ

0,sum

is the slowly varying background eigen-

phase sum obtained by replacing S by S

in (47.52)and

(47.53). It follows from (47.54) that the eigenphase sum

increases by π radians in the neighborhood of the res-

onance energy. If there are m resonances, that may be

overlapping, (47.54) generalizes to

sum

(

)

= δ

0,sum

(E) +



i=1

tan

−1



i,r

− E



(47.55)

Thisresulthasprovedtobeveryusefulinanalyz-

ing closely spaced resonances to obtain the individual

resonance positions and widths.

47.1.4 Multichannel Quantum Defect Theory

When an electron scatters from a positive ion, the op-

erator QHQ in (47.45) has an inﬁnite series of bound

states, supported by the long-range attractive Coulomb

potential, converging to the lowest energy threshold of

QHQ. Multichannel quantum defect theory (MQDT),

developed by Seaton[47.6,19,20], relates the S-matrices

and K-matrices above and below thresholds using gen-

eral analytic properties of the Coulomb wave function.

In this way the cross sections above and below threshold

can be related and whole Rydberg series of resonances

rather than individual resonances can be predicted.

When all the channels are open, the S-matrix is

related to the K-matrix by (47.17), rewritten here as

(

iI− K

)(

iI+ K

)

−1

. (47.56)

In the energy region where some channels are closed,

Seaton showed that the S-matrix can be written as

(

iI− K

)(

tI+ K

)

−1

, (47.57)

where t is a diagonal matrix with nonzero matrix elem-

ents given by

= i , open channels k

≥ 0

= tan πν

, closed channels k

< 0 (47.58)

where ν

is deﬁned in the closed channels by

=−z

/ν

. (47.59)

When some channels are closed, Rydberg series of res-

onances occur because of the terms tan πν

.Wenow

introduce the matrix

χ =

(

iI− K

)(

iI+ K

)

−1

, (47.60)

which is analytic through the thresholds. When all the

channels are open then clearly S= χ. Let us partition

the S-matrix and the χ-matrix into open (subscript o)

and closed (subscript c) channel matrices as follows:





, χ =





(47.61)

Then eliminating K between (47.57)and(47.60)and

using (47.61), the open–open submatrix of S is

= χ

− χ



− e

−2πiν



−1

. (47.62)

This is an expression for the open channel S-matrix in

terms of quantities that can be analytically continued

through the thresholds.

A similar expression can be obtained for the open

channel, or contracted, K-matrix K

deﬁned in analogy

with (47.56)by



iI− K



iI+ K



−1

. (47.63)

Partitioning the K-matrix into open and closed channels

K =





(47.64)

and substituting into (47.57) yields the expression

= K

− K

(

+ tan πν

)

−1

. (47.65)

This equation enables cross sections and the resonance

parameters in an energy region where some channels are

closed to be predicted by calculating K at a few energies

where all channels are open and then extrapolating it to

the region where some channels are closed.

A simple one channel example has already been dis-

cussed following (47.28). In this case, it follows from

(47.22)thatK = tan δ



. Also, from (47.57), a pole in the

S-matrix occurs below threshold when

tan πν + K = 0 .

(47.66)

Since from (47.59), the bound state energies correspond

to ν = n − µ,wheren is an integer and µ is the quantum

defect, then (47.66)showsthatδ



extrapolates below

thresholdtogiveπµ. This result agrees with (47.28)

since 1 − exp

(

2πη

)

≈ 1 close to threshold.

Part D 47.1

712 Part D Scattering Theory

The above theory has been extended by Gaili-

tis [47.21] who showed that the collision strengths

deﬁned by (47.20) can also be extrapolated through

thresholds. Provided that the separation between the

resonances is large compared with their widths, then

the collision strength averaged over resonances below

a threshold denoted by

Ω

(

i, f

)

, can be written as

Ω

(

i, f

)

= Ω

(

i, f

)



Ω

(i, j)Ω

(

j, f

)



Ω

(

j, k

)

(47.67)

where Ω

are the collision strengths determined above

the threshold and extrapolated to energies below this

threshold. Also in (47.67), j is summed over the de-

generate closed channels of the new threshold and k is

summed over all open channels. This result is particu-

larly useful in applications to plasmas where it is often

only the collision strength averaged over a Maxwellian

distribution of electron energies that is required.

Multichannel quantum defect theory has been ex-

tended to molecules by Fano [47.22], where it has

proved to be important in the analysis of resonances

occuring in partial cross sections involving rovibra-

tional and dissociating channels as well as electronic

channels [47.23, 24].

47.1.5 Solution of the Coupled

Integrodifferential Equations

This section considers methods that have been developed

for solving the coupled integrodifferential equations

(47.10). These equations arise in low-energy electron–

atom and electron–ion collisions. Section 47.2.2 shows

that similar equations also arise in low energy electron–

molecule collisions in the ﬁxed-nuclei approximation.

Thus the methods discussed in this section are also

applicable for electron–molecule collisions.

R-Matrix Method

This method was ﬁrst introduced in nuclear

physics [47.25, 26] in a study of resonance reactions. It

has now been applied to wide range of atomic, molecular

and optical processes, as reviewed in [47.27].

This method starts by partitioning conﬁguration

space into two regions by a sphere of radius a, chosen

so that the direct potential has achieved its asymptotic

form given by (47.12) and the exchange and correlation

potentials are negligible for r ≥ a. The objective is then

to calculate the R-matrix R

(E), which is deﬁned by

(

)



=1

i

(

)



 j

− b



 j



r=a

(47.68)

by solving (47.10) in the internal region.

The collision problem is solved in the internal region

by expanding the wave function, in analogy with (47.8),

in the form

(

N+1

)

= A





,... ,x

;

N+1



× r

−1

N+1

(

N+1

)

ijk



(

,... ,x

N+1

)

, (47.69)

where the u

are radial basis functions deﬁned over the

range 0 ≤ r ≤ a. For radial basis functions u

satisfying

arbitrary boundary conditions at r = a, the Hamiltonian

N+1

deﬁned by (47.3) is not Hermitian in the internal

region due to the kinetic energy operators. It can however

be made Hermitian by adding the Bloch operator [47.28]



i=1





δ(r − a)



−

− 1







(47.70)

to H

N+1

,whereb

is an arbitrary parameter. The

Schrödinger equation (47.2) then becomes

(

N+1

+ L

− E

)

= L

, (47.71)

which can be formally solved, giving

(

N+1

+ L

− E

)

−1

. (47.72)

Next, expand the Green’s function (H

N+1

+ L

− E)

−1

in terms of the basis Ψ

, where the coefﬁcients a

ijk

and b

in (47.69) are chosen to diagonalize H

N+1

+ L

giving





N+1

+ L







= E



. (47.73)

Equation (47.72) can then be written as













− E





(47.74)

Finally, we project this equation onto the channel func-

tions

, and evaluate it at r = a. Assuming Ψ

is given

Part D 47.1

Electron–Atom, Electron–Ion, and Electron–Molecule Collisions 47.1 Electron–Atom and Electron–Ion Collisions 713

by (47.8), we retrieve (47.68), where the R-matrix can

be calculated from the expansion

(

)



− E

(47.75)

and where we have introduced the surface amplitudes



(

)

ijk

. (47.76)

The main part of the calculation involves setting up and

diagonalizing the matrix given by (47.73). This has to

be carried out once to determine the R-matrix for all

energies E.

In the external region r ≥ a,(47.10) reduces to

coupled differential equations, coupled by the po-

tential V

(r) which has achieved its asymptotic

form (47.12).These equations can be integrated out-

wards from r = a for each energy of interest, subject to

the boundary conditions (47.68), to yield the K-matrix

givenby(47.15), and hence the S-matrix and collision

cross sections.

Kohn Variational Method

The application of variational methods in electron atom

collision theory has been reviewed by Nesbet [47.29].

An S-matrix or complex Kohn version of this method

has been developed [47.30], which has eliminated sin-

gularities present in earlier K-matrix versions of the

theory. This new method has been particularly important

in electron–molecule collision calculations [47.31].

This approach starts from the basic expansion given

by (47.8), where the reduced radial functions are chosen

to satisfy the T-matrix asymptotic boundary conditions

∼

r→∞

−



sin θ

(

)

−1

iθ



(47.77)

which follows by taking linear combinations of the

solutions deﬁned by (47.15) and using (47.17). We

then deﬁne the integral

=−

∞







dr , (47.78)

where L is the integrodifferential operator given by

(47.10) with all terms taken onto the left-hand side of

the equation.

Now consider variations of the integral I

resulting

from arbitrary variations δF

of the functions F

about

the exact solution of (47.10), where these solutions sat-

isfy the boundary conditions (47.77), and the variations

satisfy the boundary condition

δF

∼

r→∞

(

)

−1

−

iθ

δT

. (47.79)

The corresponding variation in I

to ﬁrst-order is

δI

=−

∞







δF







LδF



dr ,

(47.80)

which after some manipulation yields

δI

= (4i)

−1

δT

. (47.81)

It follows that the functional





= T

− 4i I

(47.82)

is stationary for small variations about the exact solution.

This is the complex Kohn variational principle.

This variational principle can be used to solve

(47.10) by representing the reduced radial functions F

by the expansion

(

)

= w

(

)

(

)

−1

(

)



(

)

ijk

, (47.83)

where w

and w

are zero at the origin and have the

asymptotic forms

(

)

∼

r→∞

−

sin θ

; (47.84)

(

)

∼

r→∞

−

exp iθ

, (47.85)

while φ

are square integrable basis functions. The co-

efﬁcients c

ijk

and the T-matrix elements T

can then be

determined as variational parameters in the variational

principle (47.82).

Schwinger Variational Method

This method has been used to calculate electron–

molecule collision cross sections by McKoy and

co-workers [47.32]. The method starts from the

Lippmann–Schwinger integral equation, which cor-

responds to the integrodifferential equations (47.10),

together with the K-matrix or T-matrix boundary con-

dition deﬁned by (47.15)or(47.77) respectively. The

T-matrix form of the Lippmann–Schwinger integral

equation is

(

)

= w

(

)



k

∞



∞



(

)



r, r





× U

k





, r





 j











(47.86)

Part D 47.1

714 Part D Scattering Theory

where U

k

represents the sum of the potential terms



k

+ K

k

+ X

k



in (47.10), and the multichannel

outgoing wave Green’s function G

Γ(+)

is deﬁned as-

suming all channels are open by

(

)



r, r











−w

(

)







, r < r



−w

(

)







, r ≥ r



(47.87)

where w

and w

are solutions of the Coulomb equation



−



(



+ 1

)

(

Z − N

)

+ k



(

)

= 0

(47.88)

that satisfy the asymptotic boundary conditions (47.84)

and (47.85), respectively.

An integral expression for the T-matrix can be ob-

tained by comparing (47.77)and(47.86). This gives

=−2i



∞



∞



(

)



r, r











drdr



(47.89)

or, rewriting this equation using Dirac bracket notation,

T =−2i





(+)



(47.90)

where the plus sign indicates that F

(+)

satisﬁes the

outgoing wave boundary condition (47.77), and where

for convenience we have suppressed the superscript Γ .

In a similar way, the Lippmann–Schwinger equation

corresponding to the ingoing wave boundary condition

∼

r→∞

−



sin θ

−

(

)

−1

−iθ

Γ ∗



(47.91)

can be introduced, from which follows the integral

expression

T =−2i



(

−

)





(47.92)

where F

(−)

satisﬁes the ingoing wave boundary con-

dition (47.91). A further integral expression for the

T-matrix is obtained by substituting for w

in (47.92)

from (47.86)giving

T =−2i



(

−

)



U − UG

(+)



(+)



(47.93)

Hence, a combination of (47.90), (47.92)and(47.93)

yields the functional

[

]

=−2i





(+)







(−)



U − UG

(+)



(+)





−1



(−)





(47.94)

This functional is stationary for small variations of F

(+)

and F

(−)

about the exact solution of (47.10) satisfying

the boundary conditions (47.77)and(47.91) respec-

tively, and forms the basis of numerical calculations.

Linear Algebraic Equations Method

In this method, the integrodifferential (47.10)are

reduced directly to a set of linear algebraic (LA) equa-

tions [47.33], or alternatively, (47.10) are ﬁrst converted

to integral form, given by (47.86), which are then re-

duced to a set of linear algebraic equations [47.34].

A direct approach [47.33] has been widely used for

electron–atom and electron–ion collisions. As in the

R-matrix method, conﬁguration space is ﬁrst divided

into two regions. A mesh of N points is then used to

span the internal region r ≤ a where

= 0; r

k−1

< r

, k = 1,... ,N; r

= a .

(47.95)

In addition, two further mesh points r

N+1

and r

N+2

are

introduced to enable the solution in the internal region to

be matched to the solution in the external region r ≥ a.

In the external region, (47.10) reduces to coupled differ-

ential equations that can be solved by one of the same

methods as adopted in the R-matrix approach.

The n functions F

(

)

, i = 1,... ,n,in(47.10)are

represented by their values at the mesh points r

.Using

a ﬁnite difference representation of the differential and

integral operators, (47.10) then reduces to a set of linear

algebraic equations for the unknown values F

(

)

the internal region. These equations can be solved using

standard methods for each linearly independent solu-

tion j = 1,... ,n

deﬁned by the asymptotic boundary

conditions (47.15).

47.1.6 Intermediate and High Energy

Elastic Scattering and Excitation

For electron–atom and electron–ion collisions at elec-

tron impact energies greater than the ionization threshold

of the target, an inﬁnite number of channels is open so

that they cannot all be included explicitly in the ex-

pansion of the total wave function. Several approaches

have been developed to treat collisions at these ener-

gies, such as extensions of low energy methods based

on expansion (47.8) to intermediate energies, the devel-

opment of optical potentials that take account of loss

of ﬂux into the inﬁnity of open channels in some aver-

age way, and extensions of the Born approximation to

lower energies by including higher-order terms in the

Born Series.

Part D 47.1

Electron–Atom, Electron–Ion, and Electron–Molecule Collisions 47.1 Electron–Atom and Electron–Ion Collisions 715

Pseudostate Methods

In this approach, expansion (47.8) is extended by

including a set of suitably chosen square-integrable

pseudostates Φ

that are orthogonal to the eigenstates Φ

retained in expansion (47.8). These pseudostates are usu-

ally deﬁned by diagonalizing the target Hamiltonian H

in a square-integrable basis yielding an equation analo-

gous to (47.4), viz







= ω

, (47.96)

where the energies ω

lie just below the ionization

threshold or in the continuum. The pseudostates Φ

thus represent in an average way the high lying Rydberg

states and the continuum states of the target rather than

just the low lying target bound states as in (47.4). This

enables loss of ﬂux into the continuum states to be repre-

sented enabling accurate excitation cross sections to be

calculated at intermediate energies. In addition, by cal-

culating the amplitudes for exciting these pseudostates

accurate ionization cross sections can be determined, as

discussed in Sect. 47.1.7.

A proposal to include pseudostates in expansion

(47.8) for e–H scattering was ﬁrst made by Burke

and Schey [47.35]. Also a modiﬁcation of this expan-

sion to include polarized pseudostates which allowed

for the long-range dipole and quadrupole polarizabil-

ity in e–H scattering was proposed by Damburg and

Karule [47.36]. Later detailed e–H scattering calcu-

lations which included pseudostates were carried out

by a number of authors [47.37–40] with considerable

success showing the validity of this approach.

More recently the R-matrix method, discussed in

Sect. 47.1.5, has been extended to include pseudostates

giving rise to the intermediate energy R-matrix (IERM)

method [47.41–44]andtheR-matrix with pseudostates

(RMPS) method [47.45–48], the latter approach be-

ing applicable to multi-electron atoms and ions. Both

of these methods have enabled excitation and ioniza-

tion cross sections to be accurately calculated above the

ionization threshold.

Methods have also been developed in which the

pseudostates are represented by Sturmian functions. An

expansion of this type was ﬁrst proposed by Roten-

berg[47.49] and in a more recent major development the

convergent close coupling (CCC) method has been de-

veloped by Bray, Stelbovics and co-workers [47.50–56]

which has been applied with considerable success for

both electron impact excitation and ionization.

As an example of the CCC method, we consider

−

–H scattering. In this case the radial basis of the target

states are expanded in the complete Laguerre basis

k

(r) =





(k− 1)!

(2 + 1 + k)!



1/2

× (λ



+1

exp(λ



r/2)L

2+2

k−1

(λ



r), (47.97)

where L

2+2

k−1

(λ



r) are associated Laguerre polynomials

and k ranges from 1 to the basis size N



which depends

on the angular momentum . The target states Φ

i

are

expanded for each angular momentum as follows

i





k=1

k

(r)c

ki

, i = 1,...,N



, (47.98)

where the coefﬁcients c

ki

are obtained by diagonalizing

the target Hamiltonian H

as follows

Φ

i

|Φ

j

=w

i

. (47.99)

The states Φ

i

corresponding to the lowest energies w

i

provide an accurate representation of the lowest bound

eigenstates of the target for each , while the states

corresponding to the higher energies w

i

correspond

to pseudostates representing the high lying Rydberg

states and the continuum. As N



is increased for ﬁxed

range paramenter λ



in (47.98), more bound target eigen-

states are accurately represented while at the same time

the states corresponding to the higher energies provide

a denser and more accurate representation of the con-

tinuum. The scattering amplitude is written in the close

coupling approximation as

ψ

− E



S+



ψ

|I(H

− E)



1 + (−1)





S+



(47.100)

where P is the space exchange operator which ensures

that the total wave function has the correct symmetry for

each total spin S, I is the projection operator onto the

target states Φ

i

retained in the calculation and ψ

| is an

eigenstate of the asymptotic Hamiltonian. The scattering

amplitude is then obtained by solving the close coupling

equations in momentum space [47.50].

Optical Potential Methods

An approach which is also capable in principle of in-

cluding the effect of all excited and continuum states is

the optical potential method [47.57]. Adopting Feshbach

projection operators P and Q as in (47.40), an optical po-

tential V

is deﬁned by (47.44), where now P projects

onto those low-energy channels that can be treated ex-

actly, for example by solving equations (47.10), and

Part D 47.1

716 Part D Scattering Theory

Q allows for the remaining inﬁnity of coupled channels,

including the continuum.

At sufﬁciently high energies, it is appropriate to

make a perturbation expansion of V

, where the second-

order term is given by

(

)

= PVQ

E − T

− H

+ i

QVP,

(47.101)

V being the electron–atom (ion) interaction poten-

tial, T

the kinetic energy operator of the scattered

electron, and H

the target Hamiltonian. Byron and

Joachain [47.58] converted the lowest-order terms of

perturbation theory into an ab initio local complex po-

tential for the elastic scattering of electrons and positrons

from a number of atoms.

The optical potential calculated in second-order has

also been used by Bransden et al. [47.59, 60] to describe

−

–H collisions, while McCarthy [47.61] has studied an

optical-potential approximation that goes beyond sec-

ond order, and also makes allowance for exchange. This

method is called the coupled channels optical (CCO)

model. Finally, Callaway and Oza [47.62] have con-

structed an optical potential using a set of pseudostates

to evaluate the sum over intermediate states and have

obtained encouraging results for elastic scattering and

excitation of the n = 2 states in e

−

–H collisions.

Born Series Methods

In the high energy domain, which can usually be as-

sumed to extend from several times the ionization

threshold of the target upwards, methods based on the

Born series give reliable results. Ignoring electron ex-

change for the moment, the Born series for the direct

scattering amplitude can be written as [47.63, 64]

f =

∞



n=1

n,B

, (47.102)

where the nth Born term f

n,B

contains the interaction V

between the scattered electron and the target atom or ion

n times, and the Green’s function

(+)

(

+ T

− E − i

)

−1

(47.103)

(n − 1) times, where T

and H

are deﬁned following

(47.101).

It is important to retain consistently all terms in the

Born series with similar energy and momentum-transfer

∆ =|k

− k

| dependencies. For elastic scattering, the

scattering amplitude converges to the ﬁrst Born approx-

imation for all ∆, but at lower energies it is necessary to

include Re( f

3,B

),aswellasthesecondBornterms,to

obtain the cross section correct to k

−2

. In the forward di-

rection, convergence to the ﬁrst Born approximation is

slow because of the contribution from Im( f

2,B

) which,

from the optical theorem, corresponds to loss of ﬂux into

all open channels.

For inelastic scattering, the ﬁrst Born approximation

does not give the correct high energy limit for large ∆.

Instead this comes from Im( f

2,B

). Physically, this can

be understood by noting that inelastic scattering at large

angles involves two collisions: a collision of the incident

electron with the nucleus to give the large scattering

angle, followed or preceeded by an inelastic collision

with the bound electrons to give excitation. Again, in

the forward direction, it is necessary to retain Re( f

3,B

)

to obtain the cross section correct to k

−2

Since the third Born term is difﬁcult to calculate, By-

ron and Joachain [47.65] suggested that to third-order,

the scattering amplitude should be calculated using the

eikonal Born series (EBS) approximation

EBS

= f

1,B

+ f

2,B

+ f

3,G

+ g

och

, (47.104)

where f

3,G

is the third-order term in the expansion of

the Glauber amplitude [47.66]inpowersofV,which

can be more easily calculated than f

3,B

,andg

och

the Ochkur electron exchange amplitude [47.67]. The

EBS method has been very successful when perturbation

theory converges rapidly; namely, at high energies, at

small and intermediate scattering angles, and for light

atoms.

Distorted Wave Methods

Distorted wave methods are characterized by a separa-

tion of the interaction into two parts, one which is treated

exactly and the other which is treated in ﬁrst-order. The

usual approach is based on the integral expressions for

the T-matrix given by (47.90)or(47.92). The distorted

wave approximation is obtained by replacing the exact

solution of (47.10), denoted by F

(+)

and F

(−)

in (47.90)

and (47.92) respectively, by approximate solutions, usu-

ally obtained by omitting all channels except the ﬁnal or

initial channels of interest. In addition, the potential in-

teraction U is often approximated by just the direct term

in (47.10).

This method becomes more accurate at intermediate

energies as Z − N increases, and as the angular momen-

tum of the scattered electron becomes large. However,

it gives poor results at low energies where the coupling

between the channels in (47.10) is strong, and where res-

onances are often important. It has proved to be a very

useful way of calculating electron–ion total and differ-

ential cross sections at intermediate and high energies

well removed from threshold.

Part D 47.1

Electron–Atom, Electron–Ion, and Electron–Molecule Collisions 47.1 Electron–Atom and Electron–Ion Collisions 717

47.1.7 Ionization

This section discusses processes where electrons are

ejected from the target during the collision, giving rise to

ionization. The main focus is single ionization, or (e, 2e)

processes given by

−

(

)

+ A

→ A

+ e

−

(

)

+ e

−

(

)

(47.105)

where E

, E

and E

are the incident, scattered and

ejected electron energies respectively. They are related

through the energy conservation relation

= E

+ E

+ ε, (47.106)

where ε is the binding energy of the ejected atomic

electron. The threshold behavior of the ionization cross

section leading to the Wannier threshold law is treated

in Chapt. 52.

The scattering amplitude for the direct ionization

process is [47.68]

(

, k

)

=−

(

2π

)

iχ

(

)



(

N+1

− E

)



(+)



(47.107)

where Ψ

(+)

is an exact solution of (47.2) satisfying plane

wave plus outgoing wave boundary conditions given by

(47.5), and where Φ is a solution of the equation

(

N+1

− V − E

)

Φ = 0 (47.108)

satisfying ingoing wave boundary conditions. Also,

and k

are the momenta of the two outgoing electrons

deﬁned in terms of E

and E

= E

. (47.109)

In practice the potential V is chosen so that Φ has

a simple form. Thus, for electron scattering by H-like

ions with nuclear charge Z, V is often chosen as

V =−

Z − Z

−

Z − Z

, (47.110)

so that

(

, r

)

= Ψ

(−)

(

, k

, r

)

(

−

)

(

, k

, r

)

(47.111)

where the Ψ

(−)

are Coulomb wave functions satisfying

ingoing-wave boundary conditions, and Z

and Z

are

the effective charges seen by the two electrons. In order

that the scattering amplitude does not contain a divergent

phase factor, Z

and Z

must satisfy

−

− k

(47.112)

and the phase factor in (47.107) is then given by

(

, k

)

+ k

(47.113)

The exchange ionization amplitude g

(

, k

)

can be

obtained from the Peterkop theorem [47.69]

(

, k

)

= f

(

, k

)

(47.114)

This result follows from the fact that the amplitudes

(

, k

)

and g

(

, k

)

describe the same physical

process where the electron at r

N+1

has momentum k

and the electron at r

has momentum k

. In practice, the

Peterkop theorem is not valid if approximate wave func-

tions are used to calculate the ionization amplitude. For

this reason, a relative phase τ

(

, k

)

between these

two amplitudes is sometimes introduced by

(

, k

)

= e

iτ

(

)

(

, k

)

(47.115)

This adds an element of arbitrariness into the calculation

since different choices of this phase leads to different

cross sections [47.70].

The ionization cross sections can be obtained di-

rectly from the scattering amplitudes. For random

electron spin orientations, the triple differential cross

section (TDCS) for ionization of a target with one ac-

tive electron from an initial state denoted by |i is given

dΩ



| f

+ g

| f

−g



(47.116)

By integrating the TDCS with respect to dΩ

, dΩ

or dE, we can form three different double differen-

tial cross sections, and three different single differential

cross sections. The total ionization cross section ob-

tained by integrating (47.116) over all outgoing electron

scattering angles and energies is

E/2



dEk



dΩ



dΩ

| f

+ g

| f

− g

(47.117)

Part D 47.1

718 Part D Scattering Theory

where the upper limit of integration over the energy

variable is E/2 because the two outgoing electrons are

indistinguishable.

We conclude this general discussion of the theory of

electron impact ionization by mentioning recent work

in which an integral representation for the ionization

amplitude has been developed [47.71] which is free from

the ambiguity and divergence problems associated with

earlier work [47.68, 69, 72, 73]. An important aspect of

this new development is that it has a form which can be

used for practical calculations.

We now consider several approaches which have

been used to obtain accurate ionization cross sections

commencing, as in electron impact excitation with low

energy methods and concluding with Born and distorted

wave methods.

Pseudostate Methods

An important recent development is the realization that

accurate ionization cross sections close to threshold

can be obtained by representing the ionization contin-

uum by suitably chosen square-integrable pseudostates.

As already discussed when we considered pseudostate

methods in Sect. 47.1.6 several methods including the

intermediate energy R-matrix method [47.41–44], the

R-matrix with pseudostates method [47.45–48]andthe

convergent close coupling method [47.50–56] have been

used to obtain accurate ionization cross sections in this

energy range.

Time-Dependent Close Coupling Method

Electron impact excitation and ionization ampli-

tudes and cross sections can also be determined by

solving the time-dependent Schrödinger equation di-

rectly [47.74–77]. In the case of electron scattering by

atomic hydrogen or by an atom or atomic ion with one

electron outside a closed inert shell, the total wave func-

tion can be expanded for each conserved LSπ symmetry

, r

, t) =





)

−1



, r

, t)

× Y







(47.118)

where Γ is deﬁned by (47.9) and the coupled spherical

harmonics Y



are deﬁned by









(



|



)

× Y



(θ

,φ



(θ

,φ

). (47.119)

Substituting (47.118) into the time-dependent Schrödin-

ger equation and projecting onto the coupled spherical

harmonics Y



then yields the following coupled

differential equations

∂P



, r

, t)

∂t

= T



, r



, r

, t)



















, r

, t)P









, r

, t),

(47.120)

where



, r

) =−

∂

∂r

−

∂

∂r

+ V



) + V



). (47.121)

In this equation V



is an -dependent pseudopotential

representing the interaction of the valence or scattered

electrons with the closed shell core and V









are the

radial coupling potentials obtained by taking the ma-

trix elements of the r

−1

interaction between the valence

and scattered electrons. Equations (47.120)aresolvedon

a two-dimensional grid using an explicit time propagator

which can be readily implemented on parallel comput-

ers. Commencing at time t = 0 with a wave function

which is constructed as the appropriately symmetrized

product of an incoming radial wave packet for one elec-

tron and the lowest energy bound stationary state for the

other electron, the time-dependent equations (47.120)

are integrated forward in time. Electron impact excita-

tion and ionization amplitudes and cross sections are

determined by projecting the time-evolved radial wave

function P



, r

, t) onto a complete set of target

states.

Exterior Complex Scaling Method

In this approach, developed by Rescigno et al. [47.78–

80], the time-independent Schrödinger equation for

three charged particles is solve numerically on

a two-dimensional grid without explicitly imposing

asymptotic boundary conditions for three-body break-

up. In the case of electron hydrogen atom scattering, the

total wave function is partioned into the sum of an ap-

propriately symmetrized unperturbed wave function ψ

describing a free electron with momentum k

incident on

the target ground state and a scattered wave function Ψ

which is expanded in the form

, r

) =



L



−1



, r







(47.122)

Part D 47.1

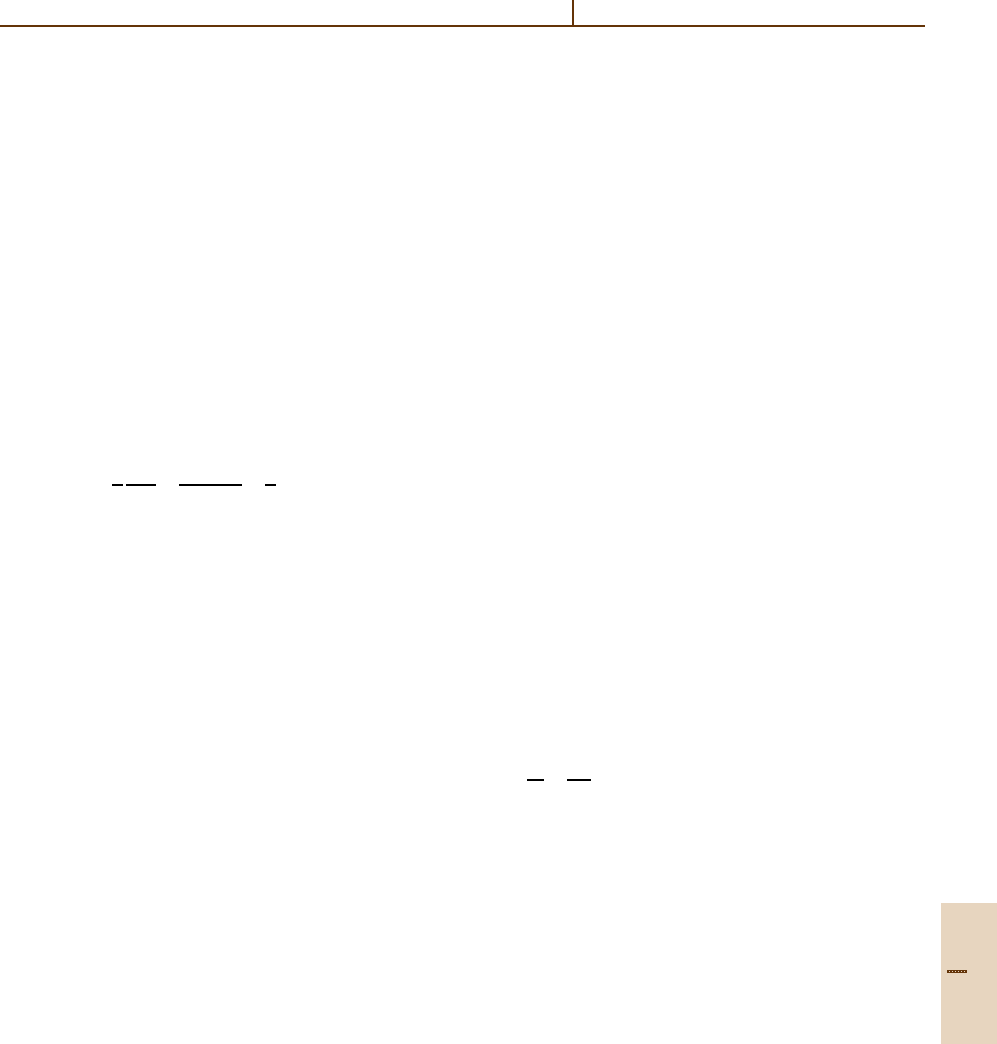
Electron–Atom, Electron–Ion, and Electron–Molecule Collisions 47.1 Electron–Atom and Electron–Ion Collisions 719

Since the z-axis is deﬁned to lie along the incident beam

direction then M

in the coupled spherical harmonics

deﬁned by (47.119) is zero. Also, since parity is con-

served the summation in (47.122) is limited to terms for

which L + 

+ 

is even.

Substituting the expression for the total wave func-

tion into the Schrödinger equation then yields the

following set of coupled two-dimensional equations for

the radial functions ψ



for each L and S:

(E − H



) − H



))ψ



, r

)

−



















, r

)ψ









, r

)

= χ



, r

). (47.123)

In this equation H



(r) is the radial hydrogenic Hamilto-

nian



(r) =−

( + 1)

−

(47.124)









,asin(47.120), are the radial coupling po-

tentials obtained by taking the matrix elements of the

electron–electron interaction r

−1

and the inhomoge-

neous term χ



, r

) arises from the partial wave

expansion of the incident wave term.

The coupled differential equations (47.123)are

solved on a two-dimensional grid using exterior com-

plex scaling (ECS) boundary conditions which avoid

imposing detailed asymptotic boundary conditions. The

ECS transformation is taken as a mapping r → z(r) of

all radial coordinates to a contour

z(r) ≡

r, r ≤ R

+ (r − R

iη

r > R

, (47.125)

that is real for r ≤ R

but is rotated into the upper half of

the complex plane for r > R

. This transformation has

the desirable property that any outgoing wave evaluated

on this contour dies exponentially as the coordinate be-

comes large. Thus the ECS procedure transforms any

outgoing wave into a function that falls off exponen-

tially outside R

but is equal to the inﬁnite range wave

over the ﬁnite region of space where the coordinates are

real. Producing meaningful ionization cross sections at

energies several eV above the ionization threshold re-

quires R

to be at least 100 a

. The grid must extend

beyond R

far enough to allow the complex scaled ra-

dial function to decay effectively to zero at the edge of

the grid requiring grids that extend an additional 25 a

beyond R

. A detailed discussion of this method is given

by McCurdy et al. [47.80].

Born and Distorted Wave Methods

The integral expression (47.107) for the direct ionization

amplitude provides a starting point for the calculation

of cross sections at higher energies. Both the Born se-

ries methods and distorted wave methods, which were

described in Sect. 47.1.6 when we considered interme-

diate and high energy elastic scattering and excitation,

have been used to obtain ionization amplitudes.

Recently an important development of the dis-

torted wave method has been made by Jones and

Madison [47.81–83]. This new approach entitled the

continuum distorted wave with eikonal initial state

(CDW-EIS), commences from the two-potential expres-

sion for the transition amplitude given by Gell-Mann and

Goldberger [47.84]



−







−



− W





(47.126)

In the ﬁrst term in this equation Ψ

is the exact scat-

tering wave function developed from the initial state

satisfying outgoing wave boundary conditions, χ

−

a distorted wave corresponding to the ﬁnal state sat-

isfying incoming wave boundary conditions and the

corresponding perturbation W

is the adjoint of the

operator W

and operates to the left. In the second term,

is the unperturbed initial state which in the case of

electron hydrogen atom scattering is

= (2π)

−3/2

exp(ik

)ψ

), (47.127)

and V

is the initial state interaction potential given in

this case by

=−

. (47.128)

An eikonal approximation is made for the initial state

wave function Ψ

in (47.126) and the ﬁnal state wave

function χ

−

is represented by a CDW wave func-

tion [47.85]. In this way distortion effects are included

in both initial and ﬁnal state wave functions. Results us-

ing the CDC-EIS approximation have been compared

with ECS calculations for electron hydrogen atom triple

ionization cross sections at 54.4 eV [47.86]. The two cal-

culations are generally in very good agreement for equal

energy sharing between the outgoing electrons which

was considered in this work.

A further development of the distorted wave method

for ionization has been made when the incident electron

is fast and interacts weakly with the target atom or ion

and the ejected electron is slow and interacts strongly

with the residual ion [47.87, 88]. In this case (47.107)

is applicable where the ionizing electron is represented

Part D 47.1

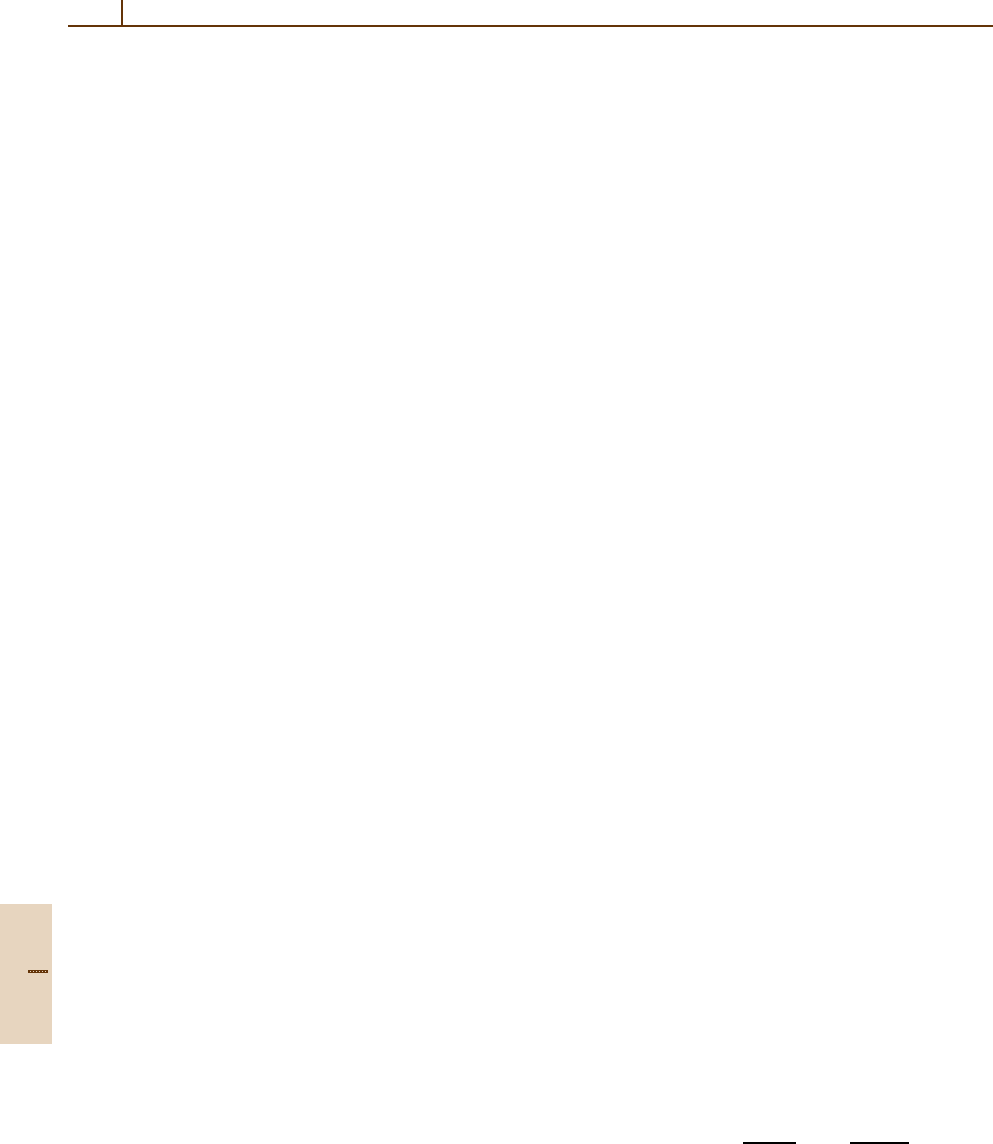
720 Part D Scattering Theory

by plane waves or distorted waves while the initial tar-

get state and the ejected electron and residual ion state

are both represented by the close coupling expansion

(47.8). This approximation is particularly useful when

the ejected electron can be captured into an autoionizing

state of the target atom or ion which then decays giv-

ing rise to the following excitation-autoionization (EA)

process

−

+ A

→





+ e

−

→



(q+1)+



+ 2e

−

(47.129)

In this equation the bracket indicates a resonance state,

while q is the charge on the atom A. This process

together with related resonant excitation double autoion-

ization (REDA) process

−

+ A

→



(q−1)+



→





+ e

−

→



(q+1)+



+ 2e

−

, (47.130)

and the resonant excitation auto-double ionization

(READI) process

−

+ A

→



(q−1)+



→



(q+1)+



+ 2e

−

(47.131)

have attracted considerable experimental and theoreti-

cal interest [47.89–93]. However, while the EA process

can be accurately treated using a distorted wave method

if the incident electron is fast, both the REDA and

READI processes involve capture of the incident elec-

tron into a resonant state and a strong coupling approach

is required.

An Example and Conclusions

We conclude this section by mentioning a recent

comparison that has been made between theory and ex-

periment for electron impact ionization of hydrogen at

17.6 eV [47.94]. At this energy, which is only 4 eV above

the ionization threshold, strong coupling effects between

the incident and ejected electrons and the residual proton

are important and hence Born series and distorted wave

methods are not applicable. This comparison thus pro-

vides a stringent test of theoretical calculations. In this

work triple-differential cross section measurements with

coplanar outgoing electrons both having 2 eV energy

were compared with exterior complex scaling (ECS)

and convergent close coupling (CCC) calculations. The

two calculations show excellent overall agreement both

with the shape and the magnitude of the experiment for

a wide range of scattering angles.

It is clear that a detailed theoretical understand-

ing of electron hydrogen atom ionization has now been

obtained over a wide range of energies. Although fur-

ther work is required to predict accurate cross sections

involving highly excited states of interest in plasma

physics and astrophysical applications, for example in

astrophysical H II regions [47.95], methods have been

developed which should enable these cross sections to

be accurately determined.

Good progress has also been made in the study of

electron impact ionization of multi-electron atoms and

ions. However major problems still remain both due

to the need to obtain accurate target states, which also

applies to elastic scattering and excitation, and due to

fundamental difﬁculties in carrying out accurate cal-

culations for REDA and READI processes deﬁned by

(47.130)and(47.131) respectively. In the latter case

major theoretical difﬁculties still arise in the accurate

treatment of resonance states which decay with the

emission of more than one electron.

47.2 Electron–Molecule Collisions

47.2.1 Laboratory Frame Representation

The processes that occur in electron collisions with

molecules are more varied than those that occur in elec-

tron collisions with atoms and atomic ions because of

the possibility of exciting degrees of freedom associ-

ated with the motion of the nuclei. In addition, the

multicenter and nonspherical nature of the electron mol-

ecule interaction considerably complicates the solution

of the collision problem by reducing its symmetry and

by introducing multicenter integrals that are more dif-

ﬁcult to calculate than those occurring for atoms and

ions.

We ﬁrst consider the derivation of the equations

describing the collision in the laboratory frame of ref-

erence discussed by Arthurs and Dalgarno [47.96]. The

Schrödinger equation describing the electron–molecule

system is

(

+ T

+ V

)

Ψ = EΨ, (47.132)

where H

is the molecular Hamiltonian, T

is the kinetic

energy operator of the scattered electron and V is the

electron–molecule interaction potential

V(R, r

, r) =



r −r

−



r − R

(47.133)

Part D 47.2