Douce A.P. Thermodynamics of the Earth and Planets

Подождите немного. Документ загружается.

588 Non-equilibrium thermodynamics

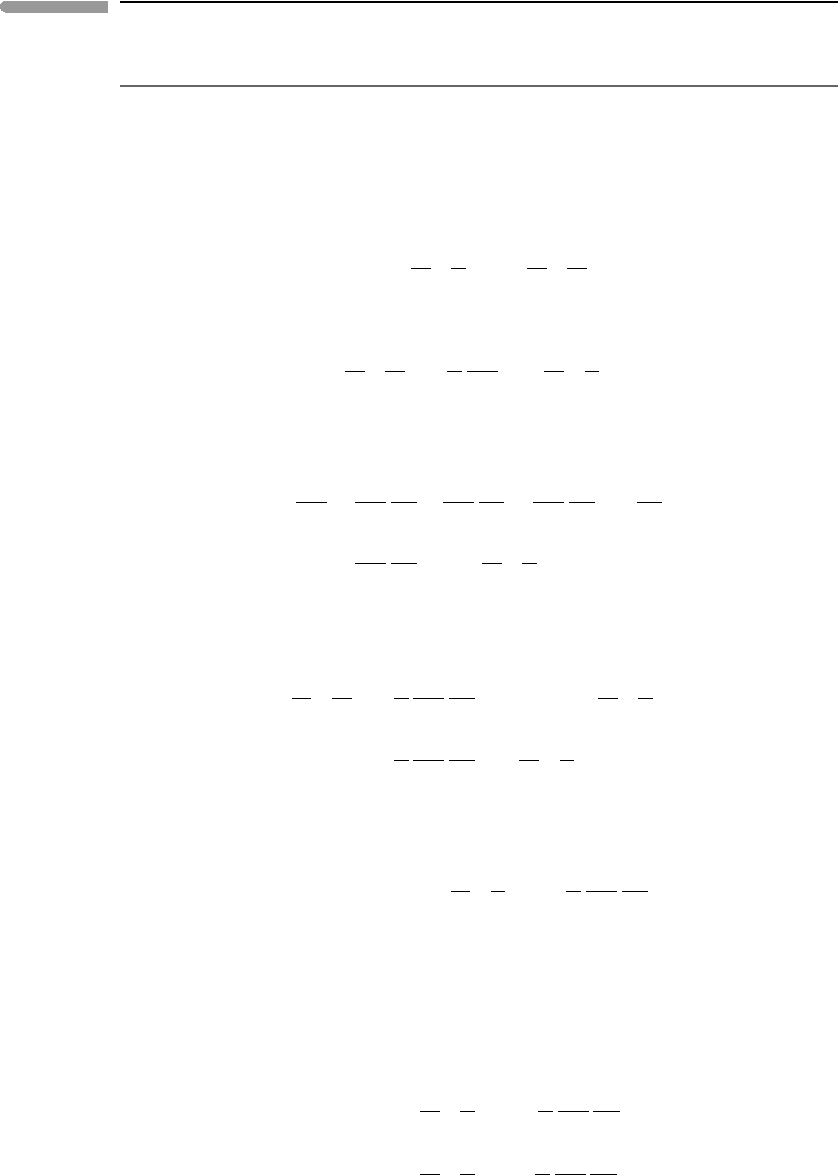
where D

is the interdiffusion coefficient of species 1 and 2 in a binary mixture.

Note that identity (12.38) does not mean that the interdiffusion coefficient is constant:

equations (12.37) show that it is a function of the concentration product c

. Because the

phenomenological coefficient L is a constant, however, it is in principle possible to calcu-

late the interdiffusion coefficient for any arbitrary composition from measurements of tracer

diffusion coefficients of one of the two species. From this we can infer that interdiffusion

coefficients are at most of the same order of magnitude as tracer diffusion coefficients. For

example, if we take species 1 as the trace component we have (equation (12.25)):

1,tr

, (12.39)

where c

1,tr

is a trace concentration of 1, and D

1,tr

is the corresponding tracer diffusion

coefficient. Using 12.37 we calculate the interdiffusion coefficient for c

1,tr

, (12.40)

which is at most of the same order as D

1,tr

, and generally smaller. It follows that the

conclusions that we reached in Worked Example 12.1 are valid for chemical diffusion in

general, not just tracer diffusion.

Consider now a slightly different case, in which rather than balancing the number of

particles, as in equation (12.31), the opposing flows of matter keep the volume of the

system constant. Equation (12.31) is then modified as follows:

=0, (12.41)

where v

, v

are the partial molar volumes of the two species. In this case we find that the

diffusion coefficients are given by:

(12.42)

(note that the dimension of L is not the same as in equations (12.37), which of course follows

from the fact that we are now considering volume fluxes rather than particle fluxes). The

interdiffusion coefficient is now given by:

, (12.43)

which, if the two species have the same partial molar volume, as is the case for ideal gases,

collapses to (12.38).

The fact that binary diffusion can be fully described with a single phenomenological

coefficient (equations (12.37)or(12.42)) means that there are no “cross-flow” terms, or,

in other words, that the Onsager reciprocal relationship does not apply to binary diffusion.

This is so because the chemical potential gradient of each component is unequivocally

determined by the concentration gradient of the other. Reciprocal matter flow terms appear

in systems of three or more components (Exercise 12.1), in which there is at least one

concentration that can vary independently.

589 12.2 Chemical diffusion

12.2.4 Coupling of mass and heat transfer

Let us consider a system in which there are gradients in temperature and composition. For

simplicity we will assume that a compositional gradient exists in only one component, i.e.

we consider tracer diffusion, and we restrict the treatment to one spatial dimension. Using

for heat flux and J

for matter flux of component i, the rate of entropy production σ is

(see equations (12.5), (12.6) and (12.20)):

σ = J

∂

∂z





−J

∂

∂z





. (12.44)

We have:

∂

∂z





∂µ

∂z

+µ

∂

∂z





(12.45)

but chemical potential is a function of both composition and temperature, both of which

vary, so:

∂µ

∂z

∂µ

∂c

∂z

∂µ

∂T

∂z

∂µ

∂c

∂z

−s

∂T

∂z

∂µ

∂c

∂z

∂

∂z





(12.46)

where s

is the partial molar entropy of component i, and c

its concentration, which we

choose to express in mols per unit volume. Substituting in (12.45):

∂

∂z





∂µ

∂c

∂z



T +µ



∂

∂z





∂µ

∂c

∂z

∂

∂z





(12.47)

where h

is the partial molar enthalpy of i. We now substitute (12.47)in(12.44) and group

terms as follows:

σ =



−h



∂

∂z





−J

∂µ

∂c

∂z

. (12.48)

The product h

, known as the heat of transport, is the thermal energy content of the matter

flow. Defining the reduced heat flux, J

q,r

as:

q,r

≡J

−h

(12.49)

we write the linear phenomenological relationships, (12.8), as follows:

q,r

∂

∂z





−L

∂µ

∂c

∂z

∂

∂z





−L

∂µ

∂c

∂z

(12.50)

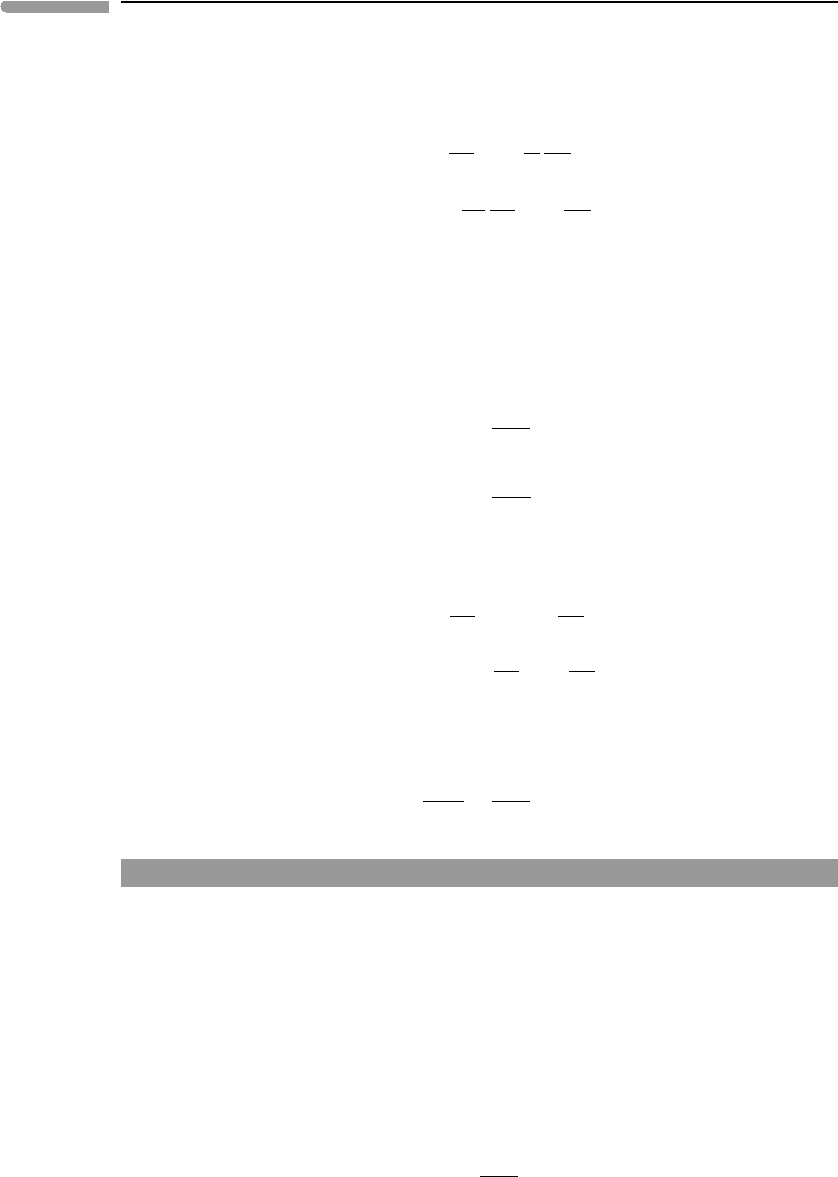
590 Non-equilibrium thermodynamics

or, using the definitions of heat conductivity k (equation (12.13)) and chemical diffusivity

(equation (12.25)), and identity (12.23):

q,r

=−k

∂T

∂z

−L

∂c

∂z

=−L

∂T

∂z

−D

∂c

∂z

(12.51)

The two cross terms are the heat flux carried by matter flow, which is known as the

Dufour effect, and the flow of matter driven by the temperature gradient, which is known

as the Soret effect, or also as thermal diffusion. Defining the Dufour coefficient, D

, and

the coefficient of thermal diffusion, D

, as follows (do not confuse D

with the thermal

diffusivity, κ!):

D,i

≡

T ,i

≡

(12.52)

equations (12.51) become:

q,r

=−k

∂T

∂z

−c

D,i

∂c

∂z

=−c

T ,i

∂T

∂z

−D

∂c

∂z

(12.53)

in which we have from the Onsager reciprocal relationship, L

D,i

T ,i

. (12.54)

Worked Example 12.2 Soret and Dufour diffusion in planetary processes

The derivations in this section make for some rather elegant, if elementary, algebra (even

more so in three dimensions). They lead to equation (12.54), which suggests an experimental

test of the Onsager reciprocal relation. But how important are these effects in planetary

processes? During the 1980s it was thought that Soret diffusion could be an important,

perhaps even dominant, mechanism of igneous differentiation (see Walker & DeLong,

1982; Lesher, 1986; Lesher & Walker, 1991). This is now known not to be the case, except

perhaps in very specific and localized situations, and then only over minuscule lengthscales.

Let us see why.

It is convenient to define the Soret coefficient, s

, as the ratio of thermal to chemical

diffusivity:

≡

T ,i

. (12.55)

591 12.2 Chemical diffusion

We note that s

has dimension of [T ]

−1

, i.e. K

−1

. The matter flux equation becomes:

=−s

∂T

∂z

−D

∂c

∂z

. (12.56)

The condition of no matter flow, i.e. J

= 0, results if the thermal and chemical gradients

have opposite signs and their effects exactly balance each other out. Thus, by setting J

we get an estimate of the magnitude of the compositional gradient that can be caused by

a thermal gradient. It is convenient to do this in terms of mol fraction rather than molar

concentration, so using the relationship X

V we write:

−

∂T

∂z

−

∂X

∂z

=0 (12.57)

which simplifies to:

=−s

. (12.58)

Thus, approximately:

δX

∼−s

δT . (12.59)

The key is, of course, the value of s

. Soret coefficients are poorly understood functions of

composition, temperature and temperature gradient (Lesher & Walker, 1991; Huang et al.,

2010). For a wide range of materials, including silicate melts, aqueous electrolyte solutions

and gases, s

is of order 10

−2

to 10

−3

−1

. This means that we can expect Soret diffusion

to be a significant effect only in settings in which there are strong temperature differences,

of order 100 K or more. Such temperature differences could exist, for example, across the

thermal boundary layer of a convective magma chamber. Soret diffusion could be important

in that instance (Sonnenthal, 2004).

This is not the whole story, however, as the rate of mass transfer is still determined by the

chemical diffusivity, regardless of whether the driving force is a gradient in composition

or temperature. Given that thermal diffusivity, κ ∼10

−6

−1

is typically six orders of

magnitude greater than chemical diffusivity in silicate melts, D

∼ 10

−12

−1

(see

Worked Example 12.1), thermal gradients decay much faster than chemical gradients. The

question is, then, how far can Soret diffusion extend in the time that it takes for a thermal

gradient to dissipate? Using λ

for the thermal diffusion lengthscale and λ

for the chemical

diffusion lengthscale we have, from equations 3.16 and 12.30:

∼





1/2

∼10

−3

. (12.60)

Suppose that a thermal gradient of ∼100 K exists across a 10-m thick thermal boundary

layer of a magma chamber. Soret diffusion would cause a significant compositional change

over a thickness of only ∼1 cm. An essentially identical result was obtained by Cygan and

Carrigan (1992) by means of a rigorous numerical solution of the mass flow equations (i.e.

(12.56)). Soret diffusion is not important in nature, although it could have noticeable effects

on isotopic fractionation during high-temperature experiments (Richter et al., 2008, 2009),

and also has technical applications.

592 Non-equilibrium thermodynamics

The Dufour effect is smaller than the Soret effect. Using (12.54) and (12.55) in the heat

flow equation (12.53), and setting J

q,r

=0, we find:

δT ∼−

δX

. (12.61)

Substituting typical values we find δT ∼ 10

−4

δX

. We can interpret this result as meaning

that the Dufour effect is equivalent to the heat flow driven by a temperature difference

of order 10

−4

K times the mol fraction difference. It is unlikely that there are natural

environments where this is a significant effect.

12.3 Rate of chemical reactions

12.3.1 Fundamental concepts

Chemical reactions displace systems towards equilibrium. Therefore, chemical reactions

take place while a system is not at equilibrium. The study of the rate of chemical reactions is

a huge field and we can only cover some of the basic concepts. In particular, we will focus

only on chemical reactions in homogeneous systems, e.g. a gas phase or a liquid solution.

We begin by considering elementary reactions. These can be defined as reactions that go

from reactants to products without any intermediate steps. At a molecular level, elementary

reactions take place by collisions between the actual reactant molecules. Most chemical

reactions are not elementary. Rather, they are combinations of elementary steps. Here we

focus on elementary reactions in homogeneous systems only, whether or not I state this

explicitly.

We can expect on physical grounds that there are likely to be some simple constraints

on the nature of elementary reactions, in particular with regards to the number of partic-

ipating molecules. This number is known as the molecularity of the reaction. Elementary

reactions are known to have molecularities of 1, 2 or 3 only, and are designated unimolecu-

lar, bimolecular or termolecular, respectively. Of these, bimolecular reactions are the most

straightforward, as they simply require a collision between two reactant molecules, for

example A and B:

A +B →products.

In contrast to this simple case, unimolecular and termolecular reactions each present prob-

lems of their own, and it is arguable whether they are rigorously elementary. Let us focus

on termolecular reactions first, which we can schematize as:

A +B +C → products.

The probability that three different molecules will converge on the exact same spot at exactly

the same time is very low, and in fact termolecular elementary reactions are much rarer than

bimolecular ones. The simplest picture of how termolecular reactions take place is that they

consist of two collisions in rapid succession, such that one of the collisions produces a

593 12.3 Rate of chemical reactions

temporary “agglutination” of two of the reactant molecules, for example:

A +B →AB,

AB +C → products.

An important point is that A and B remain stuck for only a very short period of time. If they

collide with C within this time then the products form; if not A and B separate. The fact that

termolecular reactions become more common with increasing pressure is consistent with

this model, as higher molecular density makes it more likely that C will collide with the AB

cluster before it has time to break down. We can expect that reactions with molecularities

higher than three must be exceedingly rare, and indeed there is no experimental evidence

for such reactions taking place.

Unimolecular reactions correspond to:

A →products

and they present a different problem. If a reaction is truly unimolecular, then what makes

a molecule react? Some fraction of the reactant molecules must acquire higher energy,

sufficient to cause the energized molecules to break apart into the product molecules (if

all reactant molecules were energized then the reactant would disappear instantaneously

and we would not have to worry about chemical kinetics). One way in which this can

happen is by irradiation with photons of the appropriate wavelength – this is known as

photodissociation (Section 12.4.2). But unimolecular reactions that are not photo-activated

also exist. The path of unimolecular decomposition in this case is known as the Lindemann

mechanism and can be schematized as follows:

A +M → A

∗

+M → A +M

∗

→products.

The first step, known as the activation step, involves a collision with another molecule,

M, known as a collision partner. M could be another molecule of A, it could be a product

molecule, or it could be a molecule of some other species. The important point is that as

a result of the collision some of the energy of M is transferred to A, which becomes the

activated molecule A

∗

. This molecule can lose its energy excess either by colliding with

another collision partner in a deactivation step (second step) or by breaking apart into the

products (third step). The reaction is not truly unimolecular, but if we add up the first and

third steps it looks unimolecular.

One can legitimately argue whether a unimolecular reaction of this kind is an elementary

reaction, and the same is true of termolecular reactions which consist of two collisions

in rapid succession. Such arguments may not be all that important, however, as what we

observe macroscopically is not the molecularity but a related quantity, known as the order

of the reaction. In the simple examples discussed above the molecules that participate in

elementary reactions were labeled A, B and C, but they do not necessarily have to be

different. We can therefore write a generalized elementary reaction as follows:



→products, (12.62)

594 Non-equilibrium thermodynamics

where ν

is the stoichiometric coefficient of reactant species Y

. We can expect that the rate of

an elementary reaction must be proportional to the number of molecules that are available to

react, which in the simplest case means the concentration of each of the reactant molecules.

A thermodynamically more rigorous choice would be their activities (see Section 12.3.2),

but concentration is universally used in kinetic studies, because it is a directly observable

quantity, whereas activity is not. We therefore write the rate law of reaction (12.62) as:

r =k



[

]

, (12.63)

where r is the reaction rate expressed in units of number of (reacting) mols per unit of

volume per unit of time, and [Y

] is the concentration of reactant Y

in mols per unit

volume. This notation for concentration is different from what we have used so far, but

is standard in the literature of chemical kinetics, and convenient too. The parameter k is

called the rate constant for the reaction. It is important to understand that the rate constant is

not a thermodynamic quantity, and that it in fact encapsulates aspects of chemical kinetics

that cannot be addressed by thermodynamics. We shall have more to say about this in later

sections, but we note at this point that the rate constant is a measure of the fraction of

molecules that are reactive. Owing to the statistical distribution of molecular energies (e.g.

Section 1.14) we can expect that there will always be some fraction of the total ensemble of

molecules that do not carry enough energy for the molecular bonds to be broken during a

collision. Those molecules that are energetic enough are the reactive molecules. The fraction

of reactive molecules, and thus the rate constant, increases with temperature in the case of

thermally activated reactions (Section 12.4.1), but molecules can become activated by non-

thermal processes as well, such as absorption of photons within a specific wavelength. This

is the basis of photochemical reactions (Section 12.4.2).

Reaction (12.63) is said to be of order ν

in species Y

, and the order of the reaction as a

whole is the sum



. At first sight the order of an elementary reaction is the same as its

molecularity, and indeed elementary reactions can be of first, second or third order only, but

the detailed microscopic picture is a bit different. It is clear that bimolecular reactions must

be of order two. Regardless of whether an elementary termolecular reaction occurs by a

single triple collision or by two collisions in rapid succession, we may expect it to be of order

three, because in either case the rate should vary with the product of the concentrations of the

three species. The point is that what is actually measured is the order of the reaction, which

is a macroscopic variable, and the molecularity is one possible microscopic interpretation

of this observation. Finally, it must be noted that the overall order of a compound reaction,

composed of an assemblage of elementary steps, is defined by equation (12.63), but in such

case there is no connection with any simple microscopic picture, and the order need not be

1, 2, or 3, in fact not even an integer (Logan, 1996; Houston, 2006).

An important part of the study of chemical kinetics focuses on the rate laws for reactions

of the various orders, and on how these laws combine in non-elementary reactions. We

discuss these topics below, but before doing that it is important to clarify some of the

relationships between thermodynamics and kinetics.

12.3.2 Thermodynamics, kinetics and entropy production by chemical reactions

All of the arguments that we made in the preceding section are strictly kinetic. By writ-

ing an elementary reaction, for example as A +B → products, we are eschewing any

thermodynamic content, as we are implying that the reaction proceeds in only one direction,

595 12.3 Rate of chemical reactions

and that there is no possibility of equilibrium between reactants and products. Thermody-

namics tells us that this is not the case, and that in fact there is always the possibility, at least

in principle, that the free energies of products and reactants will be the same, and equilib-

rium will be attained. The key to understanding the relationship between thermodynamics

and kinetics can be found by focusing on equilibrium situations, and more specifically on

how a chemical reaction approaches equilibrium.

Let us write a somewhat different version of reaction (12.62) as follows:



→

←



, (12.64)

where now ν

is the stoichiometric coefficient of reactant species Y

, and ν

is the stoi-

chiometric coefficient of product species Z

. The implication of equation (12.64) is that

there are two elementary reactions taking place simultaneously: the forward reaction (Y s

going to Zs) and the reverse reaction (Zs going to Y s). At equilibrium the rates of the two

reactions are the same, and in fact this is one possible definition of chemical equilibrium,

but in general this need not be so. We also define a variable, ξ , called the extent of reaction

or progress variable, as follows:

dξ ≡

=−

, all i, j , (12.65)

where dn

is the number of mols of species Zj that are produced, dn

is the number

of mols of species Yi that are consumed, and ν

, ν

are the corresponding stoichiometric

coefficients. By defining the progress variable in this way we avoid any ambiguities about

the extent of reaction that might arise from the variable stoichiometric coefficients. Note

that the progress variable is always a positive quantity. We also define the net rate of reaction

(12.64), r

, as follows:

≡

dξ

(12.66)

with dimension of mols per unit volume per unit time. If the reaction rates of the forward

and reverse reactions in (12.64) are r

and r

, respectively, then the net rate of the reaction,

, must be equal to the difference between the forward and reverse rates, i.e.:

−r

. (12.67)

We now seek to relate kinetics to thermodynamics, so we will write the rate laws

(equation (12.63)) in terms of activity rather than concentration. One way to think about

this is that in (12.63) the activity coefficients are subsumed in the rate constants, whereas

now we include them in the activities. We therefore write the forward and reverse reaction

rates as follows:













(12.68)

where k

, k

are the rate constants for the forward and reverse reactions.

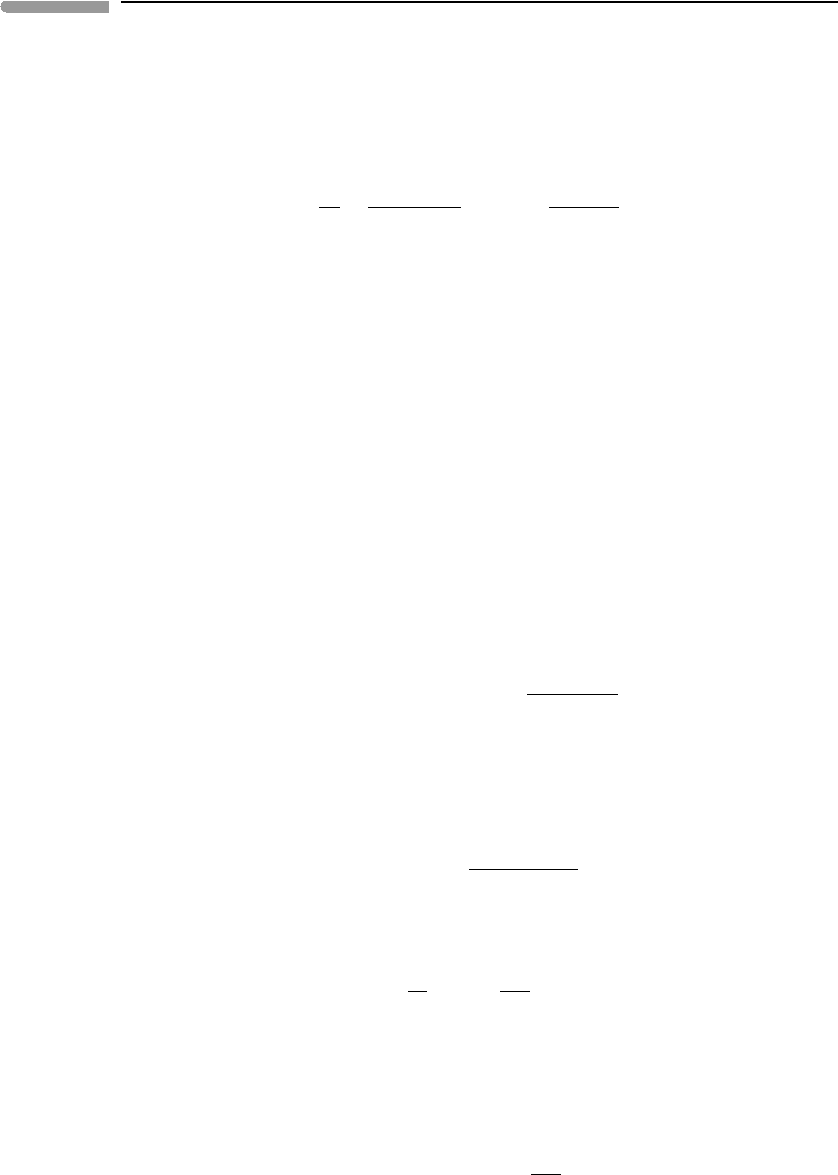
596 Non-equilibrium thermodynamics

The double arrow in equation (12.64) is always true, but we commonly think of a reaction

as “proceeding to the right” or “proceeding to the left”. What these statement refer to is

to the relative magnitudes of the forward and reverse rates. In particular, we can define

chemical equilibrium as the condition for which r

=0. From (12.68) this implies:













=exp



−



P ,T



, (12.69)

where I have added the subscript eq to specify that these are the activities at equilibrium. Up

until this point we have only dealt with equilibrium situations, so that this notation was not

necessary, but this is no longer the case. If the rate laws given by equations (12.68) are valid,

then equation (12.69) says that the ratio between the forward and reverse rate constants is

determined by equilibrium thermodynamics, and is in fact equal to the equilibrium constant.

What thermodynamics cannot do is provide the values of the individual rate constants.

We now seek a thermodynamic function that gives the distance of a chemical reaction

from equilibrium. This function is called the affinity, which I will represent with E .Itis

defined as the difference between the sum of the chemical potentials of the reactants and

those of the products. Using the notation of equation (12.64) we have:

E ≡



−



. (12.70)

If the affinity is positive then reaction (12.64) proceeds from left to right, whereas E = 0

corresponds to equilibrium. We can also write (12.70) as follows:

E =−

P ,T

+RT ln

















, (12.71)

where in this case the activities are the actual values, i.e. the values as the chemical reaction

is taking place, and not necessarily the equilibrium values. Using (12.69) we see that the

affinity is also given by:

E = RT ln

















(12.72)

or, from (12.68):

=exp





, (12.73)

which should be compared to (12.69): the ratio between the rate constants is a constant (the

equilibrium constant), but the ratio between the reaction rates varies with the affinity, or,

in other words, with the progress of the reaction. Using (12.73) we can write (12.67)as

follows:



1 −exp



−



, (12.74)

597 12.3 Rate of chemical reactions

which shows that the net rate of the reaction approaches a maximum value, equal to the

rate of the forward reaction, when E →∞, i.e. when the products are infinitely dilute.

The net reaction rate decreases exponentially as equilibrium is approached and E → 0,

but equation (12.74) yields a value for the net reaction rate only if r

is known. This in

turn requires that we know the value of the rate constant k

(equation (12.68)), which

is something that thermodynamics cannot supply. Equation (12.74) does say that the net

reaction rate increases exponentially with distance from the equilibrium state, but it does

not say that the rates of different reactions can be compared on the basis of their affinities,

as the rate constants for different reactions are generally different, and not predictable

from thermodynamics. Equations (12.69) and (12.74) encapsulate the relationship between

thermodynamics and kinetics.

We can calculate the rate of entropy production by a chemical reaction. Using

equation (12.65) we expand (12.16) as follows:

S =−







−







dξ (12.75)

or:

S =

dξ (12.76)

from which the rate of entropy production per unit volume (equation (12.4)) is:

σ =

dξ

. (12.77)

Note that this is always positive, i.e. chemical reactions always produce entropy. Comparing

with equations (12.5) and (12.66) we identify the thermodynamic flow with the net reaction

rate, i.e.:

J =

dξ

. (12.78)

We can think of a chemical reaction as a mass transfer process in which chemical components

migrate from the reactant species to the product species, and in which the net reaction rate

is the mass transfer rate. The thermodynamic potential gradient is given by:

F =

(12.79)

so that the linear phenomenological relationship (12.7) implies:

. (12.80)

This appears to be at odds with (12.74), in which we found a non-linear relationship between

the thermodynamic flow and the potential gradient. The apparent contradiction arises from

the fact that (12.74) is valid in general, whereas the linear relation (12.80) is valid only

close to equilibrium. This is explored further in Exercise 12.2.