Doktorov E.V., Leble S.B. A Dressing Method in Mathematical Physics
Подождите немного. Документ загружается.


5.2 Goursat and binary Goursat transformations 149
5.2 Goursat and binary Goursat transformations
An analogy of the Moutard transformation for the Goursat equation was
studied by Ganzha [169]. Such a Goursat transformation is valid without
a reduction restriction and redu ction equations. Many useful details can be
found in the textbook o f Ganzha and Tsarev [171], where the transformation
is defined via two solutions of (5.9). The transformed function ψ[1] and the
potential λ[1] are extracted by quadratures [169, 197].
Theorem 5.5. L et the transform ψ[1] be introduced by the relations
(z
1
ψ[1]/ψ
1
)
x
= z
1
(ψ
2
/ψ
1
)
x
, (5.25)
(z
1
ψ[1]/ψ
1
)
y
=[z
1
z
1xy
− 2z
1x
z
1y
/z
1xy
](ψ
2
/ψ
1
)
y
,
where z
1,2
are solutions of (5.9) and ψ
1,2
=
√
z
1,2x
solve (5.10). Then ψ[1] is
a solution of the (transformed ) equation (5.10) with the potential
λ[1] = λ − (ln z
1
)
xy
and the transform z[1] is found by a quadrature from
z[1]
x
= ψ
2
[1],z[1]
y
=(ψ[1]
y
)
2
/λ[1]. (5.26)
This transformation preserves the form of the Laplace–Goursat equation
(5.10), e.g., possesses the covariance property. Below we introduce a twofold
eDT for the Goursat equaton with the same property.
We introduce new variables ξ = x + y and η = x −y and rewrite (5.10) in
matrix form,
Ψ
η
= σ
3
Ψ
ξ
+ UΨ. (5.27)
Here
Ψ =
ψ
1
ψ
2
χ
1
χ
2
,U=
√
λσ
1
, (5.28)
where ψ
k
= ψ
k
(ξ,η)andχ
k
= χ
k
(ξ,η), k =1, 2 are particular solutions of
(5.10) with some λ(ξ, η), and σ
1,3
are the Pauli matrices. Let Ψ
1
be some
solution of (5.27) and Ψ = Ψ
1
. We define a matrix function σ ≡ Ψ
1,ξ
Ψ
−1
1
.
Equation (5.27) is covariant with respect to the classical DT:
Φ[1] = Φ
ξ
− σΦ, U[1] = U +[σ
3
,σ]. (5.29)
It is a particular case of the general classical non-Abelian formula from Chap.
2, the Matveev Theorem 2.19.
Remark 5.6. It is not difficult to check that the DT (5.29) is the superposition
formula for two simpler DTs given by (5.4) and (5.5).

150 5 Dressing in 2+1 dimensions
Remark 5.7. Equation (5.27) is the spectral problem for the DS equation
[13, 277]. The LT produces explicitly invertible B¨acklund autotransformations
for the DS equation. It is shown in [459] that these transformations permit
solutions to the DS equation to be constructed that fall off in all directions in
the plane according to exponential and algebraic laws.
Next we consider a closed 1 -form
dΩ =dξΦΨ+dηΦσ
3
Ψ, Ω =
dΩ,
where a 2 × 2 matrix function Φ solves the equatio n
Φ
η
= Φ
ξ
σ
3
− ΦU. (5.30)
Let us apply the DT. It can be verified by immediate substitution that (5.30)
is covariant with respect to the transformation
Φ[+1] = Ω(Φ, Ψ
1
)Ψ
−1
1
.
We can alternatively affect U (5.28) by the following transformation:
U[+1, −1] = U +[σ
3
,Ψ
1
Ω
−1
Φ].
The particular solution of (5.30) has the form
Φ
1
=
s
1
ψ
1
+ s
2
ψ
2
−s
1
χ
1
− s
2
χ
2
s
3
ψ
1
+ s
4
ψ
2
−s
3
χ
1
− s
4
χ
2
, (5.31)
where s
k
=const(k =1,...,4). It is convenient to choose Φ
1
in the form
Φ
1
= Ψ
T
1
σ
3
, (5.32)
where the sup erscript T stands for the transpose. Equation (5.32) is the par-
ticular case of (5.31). In this case
U[+1, −1] = U − 2A
F
, (5.33)
where A
F
is the off-diagonal part of the matrix A = Ψ
1
Ω
−1
Ψ
T
1
, Ω = Ω(Φ
1
,Ψ
1
)
and
A
T
F
= A
F
= fσ
1
. (5.34)
Here f = f(ξ,η) is some function. Using (5.29), (5.33), and (5.34), we see that
U[+1, −1] has the same form as for the initial matrix U ,
U[+1, −1] ≡
0
λ[+1, −1]
λ[+1, −1] 0
=
0
√
λ − 2f
√
λ − 2f 0
;
thus, the reduction restriction is vali d without the reduction equations.
5.2 Goursat and binary Goursat transformations 151
The new function Φ[+1, −1] has the form
Φ[+1, −1] = Φ − Ω(Φ, Ψ
1
)[Ω(Φ
1
,Ψ
1
)]
−1
Φ
1
, (5.35)
where Φ is an arbitrary solution of (5.30).
Using the twofold DT (5.33) and (5.35), we can construct a new solution
of the Goursat equaton by means of dressing a particular solution. As a result,
we get the following theorem (returning to the former variables x and y):
Theorem 5.8. Let
ψ
k,y
=
√
λχ
k
,χ
k,x
=
√
λψ
k
,
α
k,y
= −
√
λβ
k
,β
k,x
= −
√
λα
k
,
where k =1, 2. Then new functions
α
′
1
= α
1
−
A
1
ψ
1
+ A
2
ψ
2
D
,β
′
1
= β
1
+
A
1
χ
1
+ A
2
χ
2
D
are solutions of the equations
α
′
1,y
=
√
λ
′
β
′
1
,β
′
1,x
=
√
λ
′
α
′
1
,
where
√
λ
′
= −
√
λ +
ψ
1
χ
1
Ω
22
+ ψ
2
χ
2
Ω
11
− (ψ
1
χ
2
+ ψ
2
χ
1
)Ω
12
D
and
Ω
11
=
dxψ
2
1
+dyχ
2
1
,Ω
12
= Ω
21
=
dxψ
1
ψ
2
+dyχ
1
χ
2
,
Ω
22
=
dxψ
2
2
+dyχ
2
2
,D= Ω
11
Ω
22
− Ω
2
12
,
Λ
11
=
dxα
1
ψ
1
+dyβ
1
χ
1
,Λ
12
=
dxα
1
ψ
2
+dyβ
1
χ
2
,
Λ
21
=
dxα
2
ψ
1
+dyβ
2
χ
1
,Λ
22
=
dxα
2
ψ
2
+dyβ
2
χ
2
,
A
1
= Λ
11
Ω
22
− Λ
12
Ω
12
,A
2
= Λ
12
Ω
11
− Λ
11
Ω
12
.
Here
=
Γ
,whereΓ is an arbitrary path of integration in the plane. The
explicit expressions for the functions α
′
2
and β
′
2
are obtained by the direct
picking up of the relations indicated.
Thus the twofold eDT allows us to construct explicit solutio ns of the Goursa t
equation without solving the reduction equation.
152 5 Dressing in 2+1 dimensions
5.3 Moutard transformation
The Moutard transformation [340, 341] is a map of the DT type: it connects
solutions and the coefficient u(x, y) of the equation (5.7) so that if ϕ and ψ
are different solutions of (5.7), then the solution of the twin equation with
ψ → ψ[1] and u(x, y) → u[1](x, y) can be constructed by the solution of the
system
(ψ[1]ϕ)
x
= −ϕ
2
(ψϕ
−1
)
x
, (ψ[1]ϕ)
y
= ϕ
2
(ψϕ
−1
)
y
.
In other terms,
ψ[1] = ψ − ϕΩ(ϕ, ψ)/Ω(ϕ, ϕ), (5.36)
where Ω is the integral of the exact differential form
dΩ = ϕψ
x
dx + ψϕ
y
dy. (5.37)
The transformed coefficient (potential in mathematical physics) is given by
u[1] = u − 2(log ϕ)
xy
= −u + ϕ
x
ϕ
y
/ϕ
2
.
The pro of is straightforward; see [298] for details.
The important feature of the Moutard transformation is general for the
DTs: the transform is parameterized by a pair of solutions of the equation
and the transform vanishes if the solutions coincide. The Moutard equa-
tion is obviously transformed to the two-dimensional Schr¨odinger equation
and studied in connection with the central problems of classical differential
geometry [197].
In the soliton theory the Moutard equation enters the Lax pairs for non-
linear equations such as the KP equation [35, 168, 298, 430] (see Chaps. 9, 10
for more details).
5.4 Iterations of Moutard transformations
Analysis of the iteration sequences for the transformations of the form (5.36),
where, in accordance with (5.37),
Ω(ϕ, ϕ)=
∆
2
(ϕ, ϕ)dx
i
+ c
φ
= φ
2
/2 (5.38)
by the appropriate choice of the constant c
φ
, is performed similarly to the
algorithm given in [324] for the classical DT. S up pose the result of N iterations
is a linear combination of the integrals Ω(ϕ
i
,ψ) of (5.37):
ψ[N]=ψ +
i
s
i
Ω(ϕ
i
,ψ). (5.39)
5.5 Two-dimensional KdV equation 153
This formula is proved by induction. The main property of the Mourtard
transformation can be written as
ϕ
k
+
i
s
i
Ω(ϕ
i
,ϕ
k
) = 0 (5.40)
and gives
s
i
= ∆
i
/∆ (5.41)
by Kramer’s rule. Denoting
Ω
i
≡ Ω(ϕ
i
,ψ)andΩ
ik
≡ Ω(ϕ
i
,ϕ
k
),
we get ∆ =det[Ω
ik
], and ∆
i
is obtained from ∆ by the known rule of action
with the ith row. Hence, the results of the iterations can be presented in the
compact determinant form as in the classical Crum case [324].
Differentiating (5.39) yields
ψ
xy
[N]=ψ
xy
+(s
i
Ω
i
)
xy
= −u[N]ψ[N] (5.42)
= −uψ +(s
ix
Ω
i
+ s
i
Ω
ix
)
y
= −u[N ](ψ + s
i
Ω
i
),
and using the definition of the determinant ∆ together with the properties
Ω
ix
= ϕ
i
ψ
x
, s
ix
= −s
i
ln
x
ϕ
i
gives the DT for the iterated potential
u[N]=u +6(ln∆)
xx
, (5.43)
that is used for multikink (see the next section) and multidromions [145, 146]
construction.
5.5 Two-dimensional KdV equation
Applications of the Moutard transformations for solution of the KP and DS
equations are well known [324]; for the Nizhnik–Veselov–Novikov equation see
[278]. Here we follow [145] concerning the equation
m
ty
=(m
xxy
+ m
y
m
x
)
x
, (5.44)
which is the 2+1 version [281] of the KdV-like Hirota–Satsuma equation
[211]. Equation (5.44) was integrated by inverse spectral transform in [58, 65].
Details of multisoliton (multikink) construction a nd asymptotic behavior are
given in the next section. We also use this example in Sect. 7.3 to show how
the singular manifold method generates the Moutard transformation.

154 5 Dressing in 2+1 dimensions
5.5.1 Moutard transformations
Here we consider the asymptotic behavior of iterated solutions and the
simplest example of repeated iterations from the zero seed potential that
demonstrates the interaction of kinks. The formula for the N -times iterated
solution is
m =6(ln∆)
x
, (5.45)
where, again, ∆ =det[∆
ik
] and, like [277], the one-step transform was
performed,
∆
ik
=
dΩ(φ
k
,φ
i
)+C
ik
,C
ik
+ C
ki
= φ
k
(0)φ
i
(0),
Ω(φ
k
,φ
i
)=−2
[δ
1
dx + δ
2
dy + δ
3
dt], (5.46)
δ
1
= φ
k
φ
ix
,δ
2
= φ
ky
φ
i
,δ
3
= φ
k
φ
it
− φ
kx
φ
ixx
+ φ
kxx
φ
ix
.
This way we fix the constants of integration. A similar combination of solutions
leads to multidromions [145], the localized solitons in two dimensions (first
appeared in [62]).
5.5.2 Asymptotics of multikink solutions of two-dimensional
KdV equation
To demonstrate the possibilities of the technique in 2+1 dimensions, we con-
sider the example of kink interaction and cho ose the seed Lax pair solution
as
φ
k
= A
k
exp(a
k
x + a
3
k
t)+B
k
exp(b
k
y). (5.47)
Introducing the notations
α
ik
=
a
i
a
i
+ a
k
,β
ik
=
b
i
b
i
+ b
k
,
ξ
k
= a
k
x + a
3
k
t, ξ
i0
= a
i
x
0
+ a
3
i
t
0
,A
i
/B
i
= p
i
,
we perform integration from x
0
, y
0
, t
0
to x, y, t and obtain
∆
ik
= C
ik
+ α
ik
p
i
p
k
[exp(ξ
i
+ ξ
k
) −exp(ξ
i0
+ ξ
k0
)]+ (5.48)
+p
i
[exp(ξ
i
+ b
k
y) − exp(ξ
i0
+ b
k
y
0
)] + β
ik
[exp(b
i
+ b
k
)y − exp(b
i
+ b
k
)y
0
] .
We would stop at kinks within the choice a
i
> 0, b
i
> 0forx
0
,y
0
,t
0
→−∞;
hence,
∆
ik
=[α
ik
p
i
p
k
exp(χ
i
+ χ
k
)+p
i
exp(χ
i
)+β
ik
]exp[(b
i
+ b
k
)y]+C
ik,
(5.49)

5.5 Two-dimensional KdV equation 155
where χ
i
= a
i
x + a
3
i
t −b
i
y. Notice that it is impossible to represent ∆
ik
as a
sum of two exponents with the opposite powers like for the multisoliton de-
terminant r epresentation for the KP equation [324]. Hence, we should develop
an asymptotic calculation technique.
Let us consider the case
0 < Re(a
2
1
) <...<Re(a
2
N
)
and g o to the reference frame of the sth kink that means fixing the phase χ
s
.
Running at the level y, we shall derive the asymptotic at t →±∞.Weshall
also put C
ik
=0and∆
′
ik
= ∆
ik
exp(b
i
+ b
k
)y and account for the relation
(ln ∆)
′
x
=(ln∆)
x
. Finally, let us investigate
∆
′
ik
= α
ik
exp(χ
′
i
+ χ
′
k
)+exp(χ
′
i
)+β
ik
, (5.50)
where
x = −a
2
s
t + b
s
y/a
s
+ χ
s
/a
s
, (5.51)
χ
′
k
= a
k
(a
2
k
− a
2
s
)t +(a
k
b
s
/a
s
− b
k
)y + χ
k
/a
s
+ ln[p
k
].
Therefore, at t →∞and χ
s
=const,
χ
k
=
−∞,k<s
+∞,k>s
and the elements of the determinant matrix have the following asymptotic
values:
1. ∆
ik
→ β
ik
,i,k<s
2. ∆
ik
→ α
ik
exp(χ
′
i
+ χ
′
k
),i,k>s
3. ∆
ik
→ exp χ
′
i
,i>s,k<s
4. ∆
ik
→ α
ik
exp(χ
′
i
+ χ
′
k
)+β
ik
,i<s,k>s
It can be shown that only the first term contributes to the determinant
asymptotic. We list below the special cases:
i = s
k<s, ∆
sk
=exp(χ
s
)+β
sk
,
k = s, ∆
ss
=[α
ss
exp(χ
s
)+1]exp(χ
s
)+β
sk
,
k>s, ∆
sk
= α
sk
exp(χ
′
s
+ χ
′
k
).
k = s
i<s, ∆
is
= β
is
,
i>s, ∆
is
= α
is
[exp(χ
s
)+1]exp(χ
i
)exp(χ
i
).
It is convenient to present the explicit form of the determinant via the super-
matrix

156 5 Dressing in 2+1 dimensions
k<s k = s k>s
i<s β
ik
β
ik
α
ik
exp(χ
′
i
+ χ
′
k
)
i = s ∆
sk
=exp(χ
s
)+β
sk
∆
ss
α
sk
exp(χ
s
+ χ
k
)
i>s exp(χ
i
) exp(χ
i
)[α
is
exp(χ
s
)+1] α
ik
exp(χ
i
+ χ
k
)
In this asymptotic determinant it is possible to extract exp χ from rows i>s
and from columns k>s, i.e.,
∆ =exp
n
i=1
χ
i
− χ
s
∆
1
. (5.52)
Then
∆
1
=
β
ik
β
is
0
exp(χ
s
)+β∆
ss
α
sk
χ
s
1 α
is
exp(χ
s
)+1 α
ik
,
where 0 and 1 are matrices with zero a nd unit elements. O bviously, it follows
from (5.45) that
m =
#
n
i=1
χ
i
− χ
s
$
x
+(ln∆
1
)
x
=
n
i=1
a
i
− a
s
+(ln∆
1
)
x
. (5.53)
A Lagrange expansion by the row of number s,
[0,...,0,α
ss
exp(2χ
s
), 0,...,0] + [1,...,1,α
s,s+1
exp(χ
s+1
), 1,...,1] + ...
+(β
s1
,...,β
ss
, 0,...,0)
allows us to present the result for the asymptotic ∆
1
in a “kink” form:
∆
1
= α
ss
exp(2χ
s
)
β
ik
β
is
0
010
1 α
is
exp(χ
s
)+1α
ik
+exp(χ
s
)
β
ik
β
is
0
11α
sk
1 α
is
exp(χ
s
)+1α
ik
+
β
ik
β
is
0
β
sk
β
ss
0
1 α
is
exp(χ
s
)+1α
ik
.
(5.54)
The first determinant is arranged via a sum of the columns with the num-
ber s terms:
β
ik
1
1
+
0
0
α
is
exp(χ
s
)
.
The second determinant is zero because it has a zero row. Finally,
∆
a
=exp(2χ
s
)(α
ss
∆
1
+ ∆
2
)+exp[χ
s
](∆
3
+ ∆
4
)+∆
5
, (5.55)

5.5 Two-dimensional KdV equation 157
where
∆
1
=
β
ik
β
is
0
010
1
0
1
0
α
ik
,∆
2
=
β
ik
00
1
0
1 α
sk
1
0
α
is
α
ik
, (5.56)
∆
3
=
β
ik
β
is
0
1
0
1 α
sk
1
0
1
0
α
ik
,∆
4
=
β
ik
β
is
0
β
sk
β
ss
α
sk
1
0
α
is
α
ik
, (5.57)
∆
5
=
β
ik
β
is
0
β
sk
β
ss
0
1
0
1
0
α
ik
. (5.58)
The determination of the phase of the sth kink is performed in the following
way. If we introduce the phase χ and rewrite ∆
a
as
∆
a
=(expχ + a)
2
+ b, (5.59)
then
m =(ln∆
a
)
x
= ∆
a
x
/∆
a
=2[expχ + a]α/[(exp χ + a)
2
+ b], (5.60)
where α = χ
x
.Asaresult,
m =0,χ→∞, (5.61)
m =2aα/(a
2
+ b),χ→−∞. (5.62)
Equating powers of exponential terms,
2χ =2χ
k
+ln(α
ss
∆
1
+ ∆
2
), (5.63)
2a exp
(
1
2
ln(α
ss
∆
1
+ ∆
2
)
)
= ∆
3
+ ∆
4
, (5.64)
a
2
+ b = ∆
s
, (5.65)
we immediately determine the phase χ and asymptotic value of the sth kink
taking into account (5.53), (5.60), and (5.45).
Concluding, though this note is rather technical, it contains ideas about
a development of asymptotic construction in the “dromionic” case of 2+1
equations, as well as symmetry reductions of explicit solutions or the two-
step equation reduction. It follows from Sects. 5.1 and 5.2 that there exists a
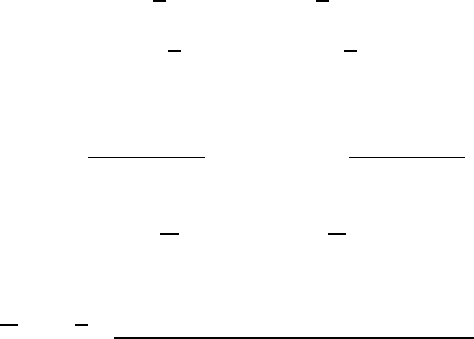
direct possibility to construct solutions of (5.10) or (5.7) via forms like (5.37).
More general asymptotic behavior can b e analyzed similarly. For example,
equating the phases of (5.53) and (5.48) and linear combinations of ξ and η
of the form (5.50) and (5.51) with Y =constyields
a
i
x + a
3
i
t − b
i
y = A
i
ξ + B
i
η,
A
i
= a
i
c
2
,B
i
= a
i
c
2
− a
3
i
T = Yb
i
.
The three-phase solutions are possible with one determinant condition on the
parameters a
i
and b
i
,andsoon.
158 5 Dressing in 2+1 dimensions
5.6 Generalized Moutard transformation
for two-dimensional MKdV equations
In this section we generate solutions of the two-dimensional MKdV equations,
giving one more example of efficient applications of the technique which ex-
ploits the generalized Moutard transformation.
5.6.1 Definition of generalized Moutard transformation
and covariance statement
The Lax pair for the two-dimensional MKdV equations (5.23) has the form
ψ
xy
=
u
x
u
ψ
y
+ u
2
ψ, (5.66)
ψ
t
= ψ
xxx
+ ψ
yyy
− 3
u
y
u
ψ
yy
+
(
3
!
u
y
u
"
2
− u
2
− B
)
ψ
y
+
A − u
2
ψ
x
+
1
2
A − u
2
x
ψ.
Ganzha [169] studied one type of the Moutard transformation for the
Goursat equation. To use this tra n sformation for obtaining exact solutions
of (5.23), we should complete the definition of the Moutard transformation.
It is easy to do that. Let φ be the second solution of (5.66) (the support
function). Then we have a closed 1-form
dθ =dxθ
1
+dyθ
2
+dtθ
3
,θ=
dθ,
where
θ
1
= φ
2
,θ
2
=
φ
y
u
2
,θ
3
=(A − u
2
)φ
2
− φ
2
y
− φ
2
x
+2φφ
xx
+
+u
−4
(2φ
3y
φ
y
− φ
2
yy
− Bφ
2
y
)u
2
− 2uφ
y
(u
y
φ
y
)
y
+3(u
y
φ
y
)
2
.
We define the generalized Moutard transformation in the following way:
u → ˜u = u −
*
(ln θ)
x
(ln θ)
y
,A→
˜
A = A − (∂
x
∂
y
− 3∂
2
x
)lnθ,
B →
˜
B = B +(∂
x
∂
y
− 3∂
2
y
)lnθ, ψ →
˜
ψ =
φQ
θ
,
(5.67)
where
Q ≡
dQ, dQ = dx Q
1
+dyQ
2
+dtQ
3
,
