Doktorov E.V., Leble S.B. A Dressing Method in Mathematical Physics

Подождите немного. Документ загружается.

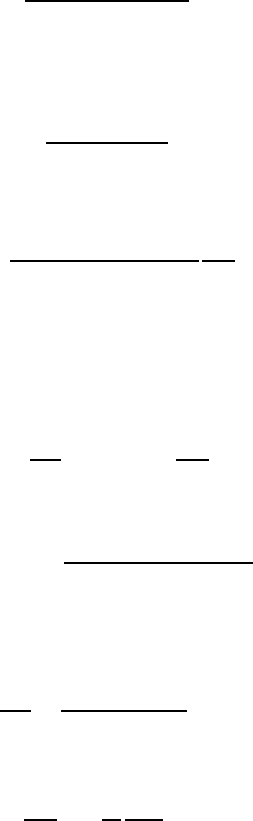
4.8 Darboux coordinates 129

4.8 Darboux coordinates

We continue to study the case N =2g + 1. Diﬀerentiation of the equation for

the spectral curve (4.54) in x yields

′

(λ

− Q

(λ

)

(λ

)

. (4.70)

The general conservation law P

′

(λ

) = 0 is taken into account. Equation

(4.66) for λ

may be rewritten as

′

− Q(λ

−1

′

(λ

)

. (4.71)

Let us solve the spectral curve equation (4.65) with the plugged P (λ

)for



j=1

+ Q(λ

−1

− I

(λ

)

∂b

∂λ

. (4.72)

This gives a new interpretation of the conserved q u antities I

as functions of

the double set of variables λ

,...,λ

and z

,...,z

. The Darboux coordinates

[103] are λ

and log z

, as follows from [423].

Proposition 4.7. Equations (4.71) and (4.70) c a n b e considered as the Hamil-

tonian system

′

= z

∂H

∂z

′

= z

∂H

∂λ

(4.73)

with the Hamiltonian

H = I



j=1

+ Q(λ

−1

− I

(λ

)

. (4.74)

For a proof, (4.71) is checked by the diﬀerentiation of (4.74) in z

and λ

.The

ﬁrst diﬀerentiation gives

∂H

∂z

− Q(λ

−1

(λ

)

and the second one yields

∂H

∂λ

= −

∂I

∂λ

The derivatives (∂I

/∂λ

) can be extracted from the result of diﬀerentiating

the spectral curve (4.54) in λ

,ifthevariablesz

are considered a s functions

of I

via (4.72).

130 4 Dressing chain equations

The diﬀerentiation of I

from (4.72) and use of (4.65) gives

∂I

∂λ



j=1

[−P (λ

+ Q

′

(λ

)]δ

(λ

)

(−b

), (4.75)

the link b

= −b

(λ

+ ... + λ

) is also used, while

∂b

∂λ

= −b

and (4.75) yield the second part of (4.73).

4.9 Operator Zakharov–Shabat problem

In this section we discuss the non-Abelian ZS problem. We formulate the DT

and the correspo nd in g dressing chain equations. As an e xample, we consider

the operator nonlinear Schr¨odinger (NLS) equation.

4.9.1 Sketch o f a general algorithm

We consider a general equation with non-Abelian entries,

Ψ + a

DΨ = Ψ

, (4.76)

that has the (ﬁrst order in D) form of the operator L with Ψ being treated as a

matrix or, more generally, as an operator. The p otential a

may be expressed

in terms of s from (4.17),

+[a

]=−Da

− [a

or, in terms of ad

=[s, .],

=(D − ad

)

−1

{−Da

− [a

}. (4.77)

The existence of the inverse operator in (4.77) imposes some restriction for

the expression in curly brackets. Namely, the expression should be outside the

kernel of the operator D +ad

. The DT is simple in this case. Namely, a

not transformed owing to (4.13) but

i+1

= a

+[a

]. (4.78)

Substituting (4.77) for i and i + 1 into (4.78) gives the chain equations. One

could also express the matrix elements of a

in terms of the elements of the

matrix s and plug them into the Darboux transform (4.78) separately. Similar

tricks give results in the case of the alternative DT (see [324]).

4.9 Operator Zakharov–Shabat problem 131

4.9.2 Lie algebra realization

Let us reformulate the general scheme to derive the dressing chain in the

non-Abelian case [270], starting again from the evolution (4.76),

uΨ + JDΨ = Ψ

, (4.79)

with the polynomial operator L(D). This case provides the nontrivial example

of a general equation (4.10) with operator entries J and u (y is changed to

t ). As a result, the form of the evolution operator (Hamiltonian) is ﬁxed in

the form JD + u. Within this scheme, the one-dimensional Dirac equa tion

arises that can be applied for consideration of a multilevel system interacting

with a quantum ﬁeld [254]. We treat Ψ (and the other solution Φ necessary

to construct the DT) as operators. The Dirac equation in the form of the ZS

spectral problem enters the Lax pair of some integrable nonlinear equations

as the NLS and Manakov equations.

The potential u is expressible in terms of σ (4.14) that we rewrite as

−σ

+ Jσ

+[Jσ,σ]=[σ, u]. (4.80)

The structure of this equation determines the algebraic properties of the ad-

missible dressing construction.

For the x-independent version, when u

= σ

= 0, (4.80) yields

−σ

+[Jσ,σ]=[σ, u], (4.81)

which means [tr(σ)]

= 0 and the choice of traceless σ. The structure of σ

implies also the restriction

det σ =detM =



. (4.82)

Namely, introducing the iteration index i,wehavethelink

=(ad

− D)

−1

(DJ +[J, σ

]σ

). (4.83)

In the subspace ker(D − ad

) = 0, where the Lie product is zero, (4.83)

trivializes. The DT (4.78) is reproduced as

i+1

= u

+[J, σ

]. (4.84)

Note that J is not changed under the DT; see (4.12). Substituting (4.83) for

i and i + 1 into (4.84), we arrive at the chain equations. We can also express

matrix elements of u in terms of the entries of σ and plug them into the

Darboux transform (4.84) separately.

Let us give more details of the construction in the stationary case, re-

stricting ourselves to DJ =0;notethatΨ and Φ correspond to λ and μ,

respectively. There are two possibilities for stationary equations that follow

from the non-Abelian equation (4.79): either Ψ

= λΨ or Ψ

= Ψλ. The ﬁrst of

132 4 Dressing chain equations

the possibilities leads to the e ssentially trivial connection between solutions

and potentials from the point of view of DT theory [321]. In the second case

we write

σ = Φ

−1

= J

−1

(ΦμΦ

−1

− u)

and the DT takes the following form in terms of s

i+1

= J

−1

J − J

−1

,J],

where s = ΦμΦ

−1

; hereafter iteration number indices are omitted. The p oten-

tials u

can be excluded from (4.14) for this case:

= Dr +[r, σ],

with

r = Jσ + u = s. (4.85)

The stationary case, after plugging u

from (4.85) and r eentering ind ices, gives

i+1

= s

+ Jσ

i+1

− σ

J, (4.86)

while the relation

+[s

,σ

] = 0 (4.87)

links the derivative of s and the internal derivative of σ. The formal transfor-

mation that leads to the chain equations is similar to (4.83) and follows after

substitution of σ

= −ad

−1

into (4.86).

Further progress in the explicit realization of this program is connected

with the choice of the additional algebraic structure over the diﬀerential ring

we consider. For example, if the elements s

and σ

belong to a Lie algebra

with structure constants C

αβ

, then we introduce the expansion (summation

over the Greek indices is implied) in the basis elements e

for s

= ξ

and

= η

. Plugging into (4.87) gives the diﬀerential equation

Dξ

+ C

γβ

=0.

In terms of the matrix B,

βα

= C

γβ

, (4.88)

we have for vectors outside of the kernel of B

= −B

−1

βα

Dξ

. (4.89)

By the deﬁnition of the Cartan subalgebra C the corresponding subspace does

not contribute to the Lie product of (4.87).

Proposition 4.8. Let J belongs to a module over the Lie algebra, Je

βα

, and let there exist an external involutive automorphism τ [e. g.,

(ab)

= b

]. Then the chain equation for the variables ξ

takes the form

i+1

= ξ

− B

−1

βγ

(Dξ

i+1

βα

+(J

αβ

−1

βγ

Dξ

)

4.9 Operator Zakharov–Shabat problem 133

where the matrix B is deﬁned by (4.88) and the components e

lie outside the

Cartan subalgebra C. Otherwise,

Dξ

=0,

if e

∈ C.

This proposition demonstrates in fact the DT in the form of (4.86) written in

the basis of e

, in which (4.89) is used. The subspace of C gives the second

case. The system of diﬀerential equations is hence nonlinear as the matrix B

depends on ξ

4.9.3 Examples of NLS equations

Split NLS dressing chai n

The split NLS equation is associated with the 2 ×2 matrix spectral problem

= i



λp

q −λ



under the reduction p =¯q and the choice J = σ

.Let

σ = η

,u= u

+ u

∈ sl(2, C)

with the Pauli matrices σ

[σ

,σ

]=2iε

iks

as generators of the algebra sl(2, C). The “Miura” connection (4.81) is spec-

iﬁed by

Jσ = iσ

− iσ

+ η

Inserting this result, as well as u and σ into (4.81), we arrive at the equations

′

+2η

=2ıη

,η

′

+2η

= −2ıη

, (4.90)

′

− 2η

=2ıη

− 2ıη

It follows from (4.82) that

− η

, (4.91)

in accordance with (4.90). Hence, the chain equations ar e

i+1

= u

− 2iη

i+1

= u

+2iη

, (4.92)

where

= −(η

′

/η

+2η

)/2i, u

=(η

′

/η

+2η

)/2i,

and η

is the function (4.91). The structure of the matrix elements of the

potential u is such that q = u

+ iu

, so the reduction to the NLS case means

the rea l ity of both u

. The repulsive NLS equation corre sponds to p = −¯q.

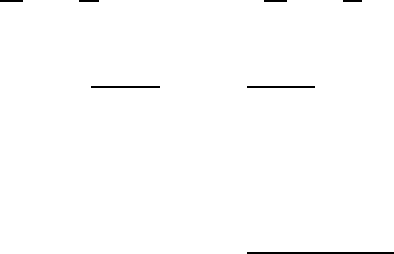
134 4 Dressing chain equations

The simplest chain closures and solutions

The simplest version of a periodic closure is

i+1

= α

,η

= x, η

= y, η

= z,

with the conditions

−μ

= x

+ y

+ z

, −μ

= α

+ α

providing restrictions for the constants

−μ

= x

+ y

− (μ

− α

)/α

= α

,μ

= μ

Hence,

= ±α

,α

= ±α

. (4.93)

Equations (4.92) yield



− 1



=2(α

+1)y,



− 1



=2(α

+1)x.

Excluding z,

− α

1+α

(ln y)

− α

1+α

(ln x)

gives nontrivial conditions for constants (4.93),

= −α

,α

= −α

In this case (ln y)

= −(ln x)

or y = c/x,

/x = −(1 − α

)

−μ

− x

+ y

which is again solved in elliptic functions [208]. If the coeﬃcients a re chosen as

)

= −(1 − α

)(−μ

− x

− c

)=(1− x

)(k

+ k

′2

then x =cn(t, k) is the Jacobi elliptic function.

The t-chains are obtained in a way similar to that in Sect. 4.3 using the

second Miura map.

4.10 General polynomial in T operator chains 135

4.10 General polynomial in T operator chains

4.10.1 Stationary equations as eigenvalue problems and chains

Let again A be an operator ring with the automorphism T . If for any two

elements f,g ∈ A

T (fg)=T (f )T (g),

general formulas for the DT for p olynomial in T operators exist [321]; see also

[271]. Here we continue to study the versions of the ZS problem. We call the

operator T a shift operator but it could be general as deﬁned above.

The stationary equation that corresp onds to the evolution generated by a

polynomial in the automorphism T (see the previous chapter) appears when

the solutions of the constraint equations ψ

(x, t)=λψ and ψ

(x, t)=ψλ are

considered, or, in the ﬁrst case,



m=−M

ψ = λψ . (4.94)

Hence, there are two versions of the scheme depending on the position

of λ. Let us rewrite the ﬁrst version of the Miura equation fr om Sect. 4.2,

omitting the index + which denotes the ﬁrst version:



m=−M

(σ) σ − σT (U

) B

m+1

(σ) σ] . (4.95)

The derivative is written as

= ϕ

(Tϕ)

−1

− ϕ(Tϕ)

−1

(Tϕ)

−1

= μσ − σμ. (4.96)

It is zero if σ and μ commute. Recall that ϕ ∈{ψ

λ=μ

The connection between potentials and σ follows as a consequence of (4.95)

in the stationary case of (4.96). For a similar link in the case of diﬀerential

operators see Sect. 4.2. When the reduction is such that all the coeﬃcients in

(4.94) are functions of some unique potential u, this connection allows us in

principle to express the potential u as a function of σ. This connection has the

same form for the dressed potential. If we take connections for both potentials

with the corresponding elements σ

and plug the result into the DT formulas,

the chain equations result.

This algorithm is illustrated next for particular examples.

136 4 Dressing chain equations

4.10.2 Nonlocal operators of the ﬁrst order

Let us take the general equation (4.94) for N =1:

(x, t)=(J + UT) ψ. (4.97)

There ar e two types of DT in this case [271], denoted by the indices ±;see

Chap.3.TheDToftheﬁrstkind(+)leavesJ unchanged. We rewrite the

transform of U as

= σ

(TU)



Tσ



−1

, (4.98)

where σ

= φ(Tφ)

−1

; further the superscript + is omitted.

For the spectral problem corresponding to (4.97), the nontrivial trans-

formations appear if in the stationary equation we introduce the constant

element μ that does not commute with ϕ and σ:

(J + UT) ϕ = ϕμ. (4.99)

The formula for the potential is then changed to

U = ϕμ(Tϕ)

−1

− Jσ. (4.100)

Let us derive the identity that links the potential U and σ,doingsoina

diﬀerent manner from that in [271] or in Sect. 4.6. We start from

T (σ)T

(ϕ)=T (ϕ),

and insert it into the shifted equation (4.99):

T (U)T

(ϕ)=T (ϕ)μ − JT(ϕ).

One has a Miura-like link

σT(U )σ = U +[J, σ], (4.101)

where T (σ)=σ

−1

is accounted for. Comparing with (4.98) yields a new form

of the DT that coincides with

U +[J, σ]=U

Direct use of (4.99) for expressing U in terms of τ = ϕμϕ

−1

and σ gives

U = τσ − Jσ. (4.102)

The e lement τ is useful as well, for

T (U)=σ

−1

τ − Jσ

−1

. (4.103)

Inserting (4.103) and (4.102) into (4.101), we arrive at the identity. The al-

gorithm of the explicit deriva tion of the chain equations begins from (4.101)

4.10 General polynomial in T operator chains 137

solved with respect to U in an appropriate way. For matrix rings, it may be

a system of equations for matrix elements, which could be eﬀective in low

matrix dimensions of the “Miura” link (4.101).

The role of σ

can be played also by the function s = ϕμ(Tϕ)

−1

.Equa-

tion (4.100) connects U and σ. Let us rewrite (4.101) and the DT in terms

of s, excluding U from (4.100) and denoting the number of iterations by

index n:

U[n]=s

− Jσ

Equation (4.101) reduces to

= σ

T (s

)σ

. (4.104)

This result gives for the DT

n+1

− s

= Jσ

n+1

+ σ

Then, solving (4.104) with respect to s, we obtain the chain system. This

could be done similarly to the method in the previous section by means of the

Lie a lgebra representation.

Let us mention that the chain equations for the classical ZS problem and

two types of the DT were introduced in [396]. The closure of the chain equa-

tions speciﬁes cla sses of solutions.

4.10.3 Alternative spectral evolution equation

Let us take (4.94) in the alternative version of the spectral problem (left

position of the parameter μ):

+ U

T ) ϕ = μϕ. (4.105)

The connection b etween the unique potential U

and σ

(recall that + denotes

the ﬁrst version considered in 4.10.1) is obtained from (4.95) and (4.96), or

directly from ( 4.105) ,

=(μ − U

)σ

. (4.106)

Introducing the number of iterations n for U

and n +1 forU

yields

[n +1]=(μ

− U

)σ

[n +1]=σ

[n] T

(μ

− U

)σ

[n)

'

Tσ

[n]



−1

(4.107)

This should be the chain equation for the generalized ZS problem. We rewrite

the chain equation (4.107) in a more compact form changing the notations as

follows U

→ J, U

→ U, σ

[n] → σ

and supposing that T (J)=J.We

arrive at

n+1

=(μ

− J)

−1

(μ

− J).

This dressing is, however, almost trivial. Su ch a phenomenon is well known for

the diﬀerential operators [324]. The alternative and eﬀective transformations

138 4 Dressing chain equations

appear if in the stationary equation (4.105) we introduce the element μ that

does not commute with σ and changes the order of the elements μ and ϕ on

the rig ht-hand side. (It is the second case mentioned at the beginning of this

section). We get

(J + UT)ϕ = ϕμ.

The formula for the potential is changed to

U = ϕμ(Tϕ)

−1

− Jσ (4.108)

and the role of σ

is played by the function s = ϕμ(Tϕ)

−1

. Equation (4.95)

connects U and σ:

Jσ + U − σJσ + σT(U)(Tσ)

−1

=[μ, σ]. (4.109)

The algorithm of the explicit derivation of the chain equations begins from

(4.109) solved with respect to U in the appropriate way. For matrix rings, it

may be a system of equations for matrix elements that could be eﬀective in

low matrix dimensions of (4.109), as in [396]. Otherwise it leads to a problem

of the adequate choice of basis. Let us rewrite (4.109) and the DT (4.107) in

terms of s, excluding U from (4.108) and denoting the number of iterations

by the index n: U[n]=s

− Jσ

. Equation (4.109) is transformed as

s − σJσ + σT (s)(Tσ)

−1

+ σJ =[μ, σ].

This result gives for the DT

n+1

− s

= Jσ

n+1

+ σ

Jσ

− [μ, σ

]. (4.110)

Then, taking (4.110) for two indices (e. g ., they could b e n and n +1) we

have the chain system. In the next section we give the explicit example for

the bilinear Hirota equation .

4.11 Hirota equations

In this section we build dressing chain equations for the non-Abelian analog

of the Hirota equations. Performing periodic closure, we obtain a solution of

the equations.

4.11.1 Hirota equations chain

Let us return to the Hirota equations and to their non-Abelian analog from

Sect. 2.10. Excluding the transformed potential from (2.127) and (2.128), we

arrive at the equation that links the potential and the function σ

−

(r):

−1

−

(r) − σ

−

(r − 1) u =



−

(r − 1) − σ

−

(r)



−1

−

(r) . (4.111)