DiBenedetto E. Degenerate Parabolic Equations

196

VII.

Harnack

estimates and extinction profile

for

singular equations

t

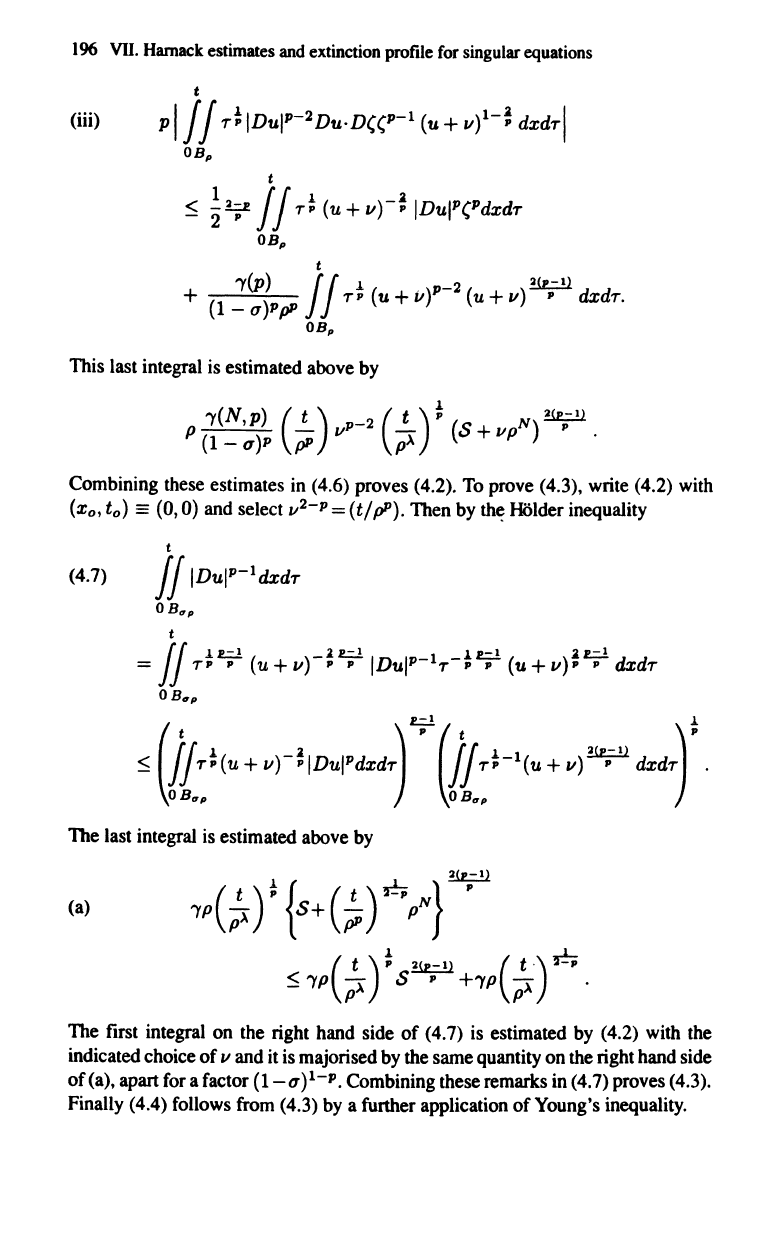
(iii)

p I I I

'T~

I

Du

l,,-2

Du·D((,,-l

(U

+

1I)1-~

dxd'Tl

OBp

t

~

~¥

I I

'T~

(U

+

II)-~

IDul"("dxd'T

OB

p

t

+

(1

2~;"pI'

11'1';

(u + v),,-2

(u

+

II)

2('1'-1)

dxd'T.

OBp

This last integral is estimated above by

p "Y(N,p)

(.!.-)

11,,-2

(.!.-)

~

(8

+ IIpN)

2(,;1)

•

(1

-

U)"

pi'

p>'

Combining these estimates in (4.6) proves (4.2). To prove (4.3), write (4.2) with

(x

o

,

to)

==

(0,0) and select

11

2

-"

=

(t/

PP).

Then by

th~

HOlder inequality

t

(4.7)

IIIDUI,,-ldxd'T

OB"p

t

{f

H=1

12=1

.12=1

12=1

=

JJ

'TP

I'

(U+II)-"

I'

IDul,,-l'T-

p

I'

(U+II)"

"

dxd'T

OB"p

2=1

.1

,; (ijT*(U+

v)-iIDUIPdzdT)

I'

(il'T~_l(U

+

II)~

dxd'T)

I'

~OB"p

OB"p

The

last integral is estimated above by

(a)

(

t)f;{

(t)~

}~

"YP

P>'

8+

pi' pN

(

t )

~!1£=.ll

(

t.)

~

"YP

-X 8

I'

+"YP

-X

.

P P

The first integral

on

the right hand side

of

(4.7) is estimated by (4.2) with the

indicated choice

of

II

and it is majorised by the same quantity

on

the right hand side

of

(a),

apart

for a factor

(l-U)I-

P

. Combining these remarks in (4.7) proves (4.3).

Finally (4.4) follows from (4.3) by a further application

of

Young's ineqUality.

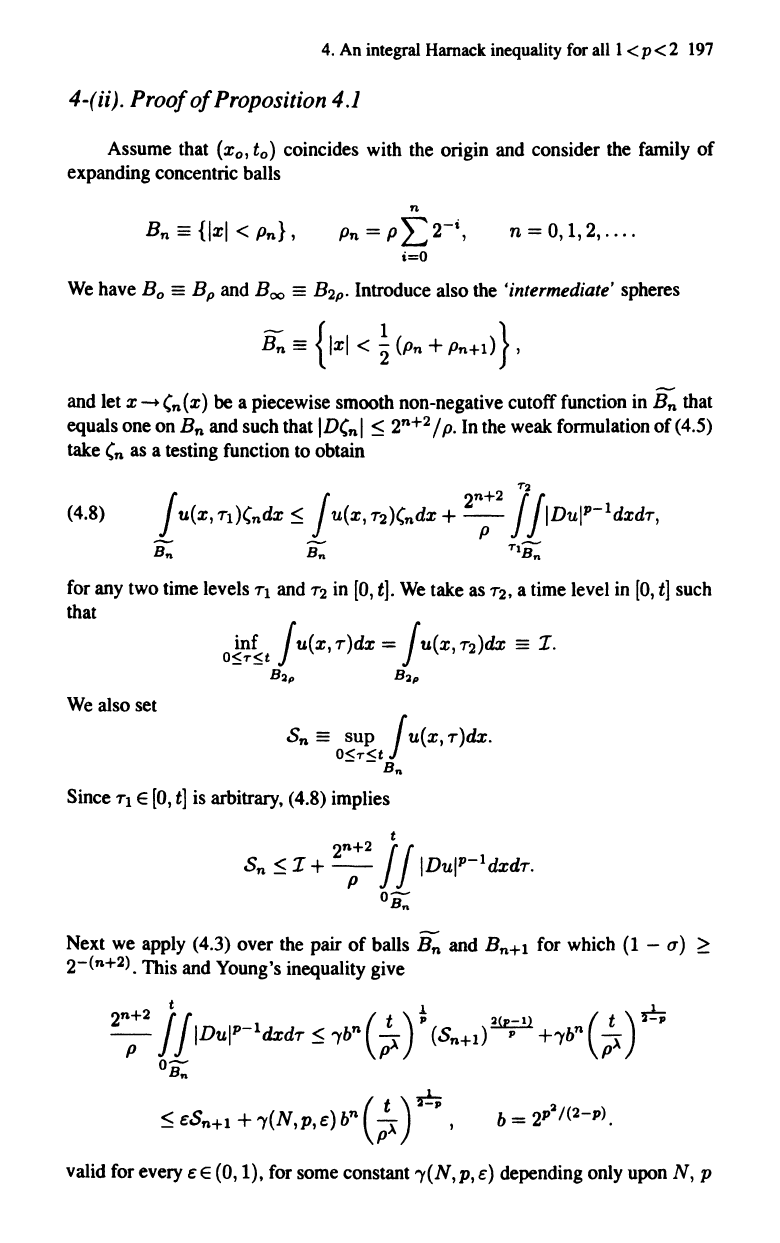
4.

An

integral Harnack inequality for all 1 < p < 2 197

4-0i). Proof

of

Proposition

4.1

Assume that

(X

o

,

to)

coincides with the origin and consider the family

of

expanding concentric balls

n

Pn

= P L

2

-

i

,

i=O

n =

0,1,2,

....

We have Bo

==

Bp and

Boo

==

B2p' Introduce also the 'intermediate' spheres

and let x

-+

'n

(x)

be

a piecewise smooth non-negative cutoff function in

Bn

that

equals one on

Bn

and such that

ID'nl

~

2

n

+

2

/

p.

In the weak formulation

of

(4.5)

take

'n

as a testing function to obtain

for any two time levels

'Tl

and'T2 in

[0,

tJ.

We

take as

'T2,

a time level in

[0,

tJ

such

that

We also set

Sn

==

sup

ju(x,

'T)dx.

O<T<t

- -

Bn

Since'Tl E

[0,

tJ

is arbitrary, (4.8) implies

Next we apply

(4.3) over the pair

of

balls

Bn

and

BnH

for which

(1

- u)

~

2-(n+2). This and Young's inequality give

t

.1

6

2n;2

j jIDuIP-1dxd'T

~

",b

n

(;)

P

(SnH)

2(,;1)

+",b

n

(;l)

-P

0-

Bft

(

t)J!;

~eSnH+",(N,p,e)bn

pl

'

valid for every

eE

(0, I), for some constant

",(N,p,

e) depending only upon

N,

p

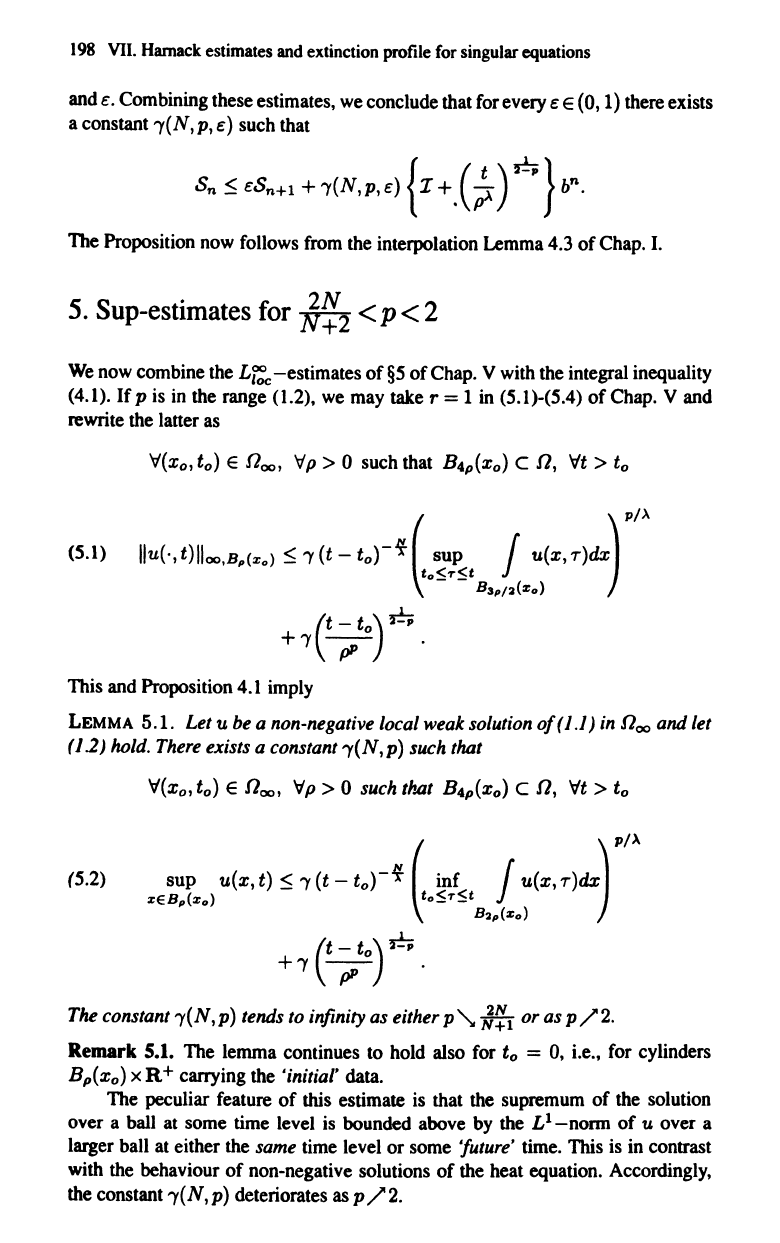
198

VII.

Harnack

estimates

and

extinction profile

for

singular

equations

and

E. Combining these estimates,

we

conclude that

for

every E E (0,1) there exists

a constant

'Y(

N, p,

E)

such that

s..

!>

<Sft+! + 1(N,p,<)

{z

+.(~)

r-.}

b".

The Proposition

now

follows

from

the

interpolation

Lemma

4.3 of

Chap.

I.

5.

Sup-estimates

for

&~2

< p < 2

We

now

combine

the

Ll::

-estimates of

§5

of

Chap.

V

with

the integral inequality

(4.1).

If

p

is

in

the range (1.2),

we

may

take r = 1

in

(5.1)-(5.4)

of

Chap. V and

rewrite the latter

as

(5.1)

lIu(·,

t)lIoo,Bp(zo)

~

'Y

(t -

to)-~

( sup J u(x,

T)dx~

pI)..

to~.,.~t

B

Sp

/

2

(zo)

+'YC~to)

~.

This and Proposition 4.1

imply

LEMMA 5.1. Let u

be

a non-negative local weak solution

of

(1.1)

in

noo

and let

(1.2)

hold.

There

exists a constant 'Y(N,p) such that

V(xo,

to)

E n

oo

,

Vp>

° such that

B4p(X

o

)

c

n,

"It>

to

(5.2) sup u(x, t)

~

'Y

(t -

to)-~

(inf

J u(x,

T)dx~

pI"

zeBp(zo)

to~"'9

B2p(Zo)

+'Y

e~to)~.

The

constant

'Y(

N,

p) tends to infinity as either p

'\,

J~

1 or as p /

2.

Remark

5.1. The lemma continues

to

hold

also

for

to

=

0,

i.e.,

for

cylinders

Bp(x

o

)

x

R+

carrying the 'initial' data.

The peculiar feature

of

this estimate

is

that

the

supremum of the solution

over a

ball

at

some

time

level

is

bounded above

by

the

Ll-nonn

of

u over a

larger ball

at

either

the

same

time

level or some 1uture'

time.

This

is

in

contrast

with the behaviour of non-negative solutions

of

the heat equation. Accordingly,

the

constant

'Y(

N, p) deteriorates

as

p /

2.

6.

Local

subso1utions

199

5-(i). A special/orm 0/(5.2)

We will use this fact in the following form. Let u be a non-negative weak

solution

of

the p.d.e. in (1.1) in some space-time domain and let p be in the range

(1.2). Let

R>O

and assume that the cylinder

Q4R

==

B4RX{-4,

O}

is all contained in the domain

of

definition

of

u.

Then

6. Local subsolutions

As in the degenerate case, the proof

of

Theorem

1.1

is based on expanding the

positivity set

of

the solution u by means

of

suitable comparison functions. Let

b,

k,

JL

be positive parameters satisfying

(6.1)

Consider the cylindrical domain with annular cross section

(6.2)

Q(8)

==

{:-1

k

P

-

2

<

Ixl

P

<

I}

X

{O,8},

and the function

(6.3)

LEMMA 6.1. Assume that p

is

in

the

range (1.2), i.e.,

A

==

N(P

-

2)

+ p >

O.

Then

the

constant

b=b(N,p)

can

be

chosen a priori only dependent

upon

Nand

p,

so

that

Vk

> 0, V

I'

> 0 > satisfying (6.1),

(6.4)

Wt

- div(lDwl

p

-

2

Dw)

~

0

a.e.

in

Q(8),

8=min{

(~)P-l

JLj

k2-P}.

Remark

6.1. The proof below shows that the constant b> 1 is • stable' as p / 2.

200

YD.

Harnack

estimates

and

extinction profile

for

singular equations

e

o

Figure

6.1

PROOF

OF

LEMMA 6.1: The function

x-!li(x,t}

is

radial and decreasing with

respect to

Ixl.

so that writing (6.4) in polar coordinates

we

have

where

We

write

C(!Ii}

==

!lit - div(ID!liI,,-2 D!Ii}

= !lit + ( N ;

1)

(_!li,},,-l _ (p _ 1)( _!li,),,-2!1i",

p=lxl,

!Ii' =

~!Ii

;

dp

(6.5) ( _!li,)2-"

C(!Ii)

= (_!li,)2-"!lit +

'R.(!Ii)

,

'R.(!Ii)

==

N - 1

(-!Ii')

- (p - 1 } !Ii" ,

p

and calculate

'R.(!Ii)

as

follows. First

we

set

~

(IXI")~

IIzll

=kFIb

-t

;

F = 1 +

IIzll

k

W=--l

FF-;

11

=

(1

-lxI

2

);!r.

Then by direct calculation

6. Local subsolutions

201

(6.6)

We calculate the expressions

1/1'

=

w'v

+

wv'

and

1/1"

=

w"

v + 2w'v' +

wv"

from

(6.6) and combine them into

'R.(I/I)

to obtain

(6.7)

'R.(I/I)

:5

-P-(I

-

p2);;!-r

(w)

M

{N

- 1

2-p

p2

:F

- (p -

1)

- - + 1 +

p-

[

P

IIzll

] 1 }

2-p:F

:F

+

2pw(I-

p2)~

{(N

-

1)

_

~

M +

I}.

p-I

2-p:F

Rewrite the first factor in braces on the right-hand side

of

(6.7) as

{

...

}=(N--

P

M).

2-

p

:F

We

will impose on

/I

z

II

to

be

so

large that

(6.8)

N-_p_M<o.

2

-p

:F

This is possible since N(p - 2) + p

==

A>

O.

The second term in braces on the

right hand side

of

(6.7) is negative

if

we choose

IIzll

to satisfy (6.8).

If

N =

1,2,

this is a direct consequence

of

(6.8).

If

N

~

3,

(P_I){N-I

_

~M

+I}

=

(N-

_P_

M)

p-I

2-p:F

2-p

:F

+

-p-

(3

-

2p)M

+

(p-

2).

2-p

:F

The first term is negative in view

of

(6.8) and the second is negative since p >

;:~l

>

~

if

N

~

3. We drop the last negative term

on

the right hand side

of

(6.7)

and estimate

202

VII.

Harnack

estimates

and

extinction profile

for

singular equations

(6.9)

'R.(lli)

~

_P-v (

W)

J!.:!l

[N

_ _

P_

J!.:!l]

.

2-p

p

2

:F

2-p

:F

We return

to

(6.5) and estimate above the term ( -lli,)2-"lli

t

•

First using (6.6)

, w ( 2p

p)

(w)

-lli

~

P p _ 1 + 2 _ p

==

'Y

P .

Also

lli

t

=

_1_

vw

J!.:!l

~.

2-p

:F

t

Therefore

(-lli')2-"lli

t

~

(~)2-"

vw.ll:l!.~.

2-p

p

:F

t

We combine this with (6.9) into (6.5) and set

to obtain

C*(lli) =

(-lli')2-"[lli

t

_

div(lDllil,,-2 Dlli)]

(2

- p):F

p2

vwllzll

C*(lli)

~

'YW2-"pP

+ P

[N

-

-p-

.ll:l!.]

.

t

2-p

:F

From the defmition

of

w and

II

z

II

w

2

-"pP

= (

IIzll

),,-1

6

1

-"

<

_I_

t 1 +

IIzll

-

11'-1

and

.c*(lli) < ...:L +

_P_

[-,\

+

1!..]

.

-

11'-1

2 - P

:F

We will choose

II

z

II

so

large that

~

~,and

then select 6 from

'Y

p'\

-----=0

11'-1

2 - P 2 .

We will have

~

==

I41T

~

if

for example

IIzll

>

¥,

i.e.

if

k

2

-"

V-I

Ixl"

(~)

,,-1'

> t >

O.

From the construction

of

the cylinder

Q(

fJ)

in

(6.2),

we

have

k

2

-"

V-I

Ixl"

~

",.

Therefore

to

prove the lemma

it

suffices to take

(

,\),,-1

o < t

~

fJ

= 2p

",.

7.

Time

expansion of positivity

203

7.

Time expansion of positivity

The next subsolution

of

(1.1) will be employed to expand the set

of

positivity

of

u

in the direction

of

increasing

t.

Set

(7.1)

k~

{ (

Ix

I"

);2:r}2

~(x;t)

==

Re(t)

1-

R(t)

+'

R(t)

==

k

P

-

2

t +

pP,

F

==

l-lIzlI;2:r,

IIzll

==

:(1:).

Here k and p

are

positive parameters and

e>

1 is a number to be chosen indepen-

dent

of

k and

p.

For t = 0 the function

~(.,

0) is supported in the ball B p and for

t > 0 the support

of

x-~(x,

t)

is the 'expanding' ball

We

will consider

~

only within the domain

The function

~

is continuous in V{k,e}. vanishes on the 'lateral' boundary

of

V{k,e} and it is

of

class

Coo

in the interior ofV{k,e}.

LEMMA

7.1. The number e =

e(

N,

p) can be determined a priori only in terms

of

N

and

p so that

Remark

7.1. The constant e is 'stable' as p

/'

2.

PROOF

OF

LEMMA

7.1: By direct calculation within V{k,e} we have

ek"-l~

2 2

k"-l~

-L

~t

= - Re+l(t) F +

p-l

~+l(t)Fllzllp-l,

2p

k~

(IXI);2:r x

D~=

-

p-l

~(t)F

R(t) lxi'

ID~I,,-2D~

= _

(~)P-l

[k~

F],,-l

-=-

p - 1 Re(t)

R(t)

,

-div

1~1,,-2D~

=

(~)"-l

_1

[k~

F]"-l

(N

_

pIlZII;2:r)

.

p-l

R(t)

~(t)

F

Setting

204

VU.

Harnack

estimates

and

extinction profile

for

singular equations

the previous calculations give

(7.3)

C·

(4))

=

{-

f.F +

_2_lIzll~

p-l

(

~)P-l

[~

]p-2(

_

IIZIl~)}

+ p _ 1

R£.(t)

F N P F .

Introduce the two sets

£1

==

{(X,t) E

V{k.£.}

I:F

< 6},

£2

==

{(X,t) E

V{k.£.}

I F

~

6}.

Here 6 is a small positive number to be determined so that, within

£1,

the last term

on

the right hand side

of

(7.3) is negative, i.e.,

With such a choice we have in

£1

(7.4)

c-

(4))

~

_2_

+

(~)p-l

(N

_

~)

,

p-l p-l

6

where in estimating the term containing R(t)

on

the right hand side

of

(7.3) we

have used the fact that 1

< p <

2.

We

determine 6 so that the right hand side

of

(7.4) is

non-positive

and observe that such a choice can

be

made independent

of

f..

Next, having determined 6, within

£2

we have

(7.5)

c-

(4))

~

-f.6 +

_2_

+ N

(~)p-l

[nt.(t)

~]2-p

p-l

pPt.

6

Within

the

range (7.2)

of

t we estimate

[

nt.(t)

1]2-1'

(

1)£.(2-1')

-2

(e)2-p

---

< 1 + -

61'

< - .

,.6

-

f.

-6

We

substitute this estimate in (7.5) and

choo~e

f.

so large that the right hand side

is non-positive.

8.

Space-time configurations

Locally bounded weak solutions

of

(1.1) are locally

R;Ider

continuous in the in-

terior

of

their domain

of

definition, "ripE (0,1). This is

the

content

of

Theorem 1.1

of

Chap.

IV.

The proof consists

of

controlling the essential oscillation

of

a local

solution over a family

of

nested and shrinking cylinders. Such a control is estab-

lished in Proposition 2.1

of

Chap.

IV,

by working with cylinders whose 'space

dimensions' are rescaled in terms

of

the solution itself. As observed in Remark 2.2

8.

Space-time

configurations

205

of Chap.

IV,

such a geometry is not the only possible. A version of Proposition

2.1 holds for

an

intrinsic parabolic geometry where the scaling occurs

in

the 'time

dimension'.

We

restate the proposition for such a geometry

in

the context of (1.1)

and

in

a

form

convenient for the proof of

the

Harnack inequality. Let

1.£

be

a local

weak solution of

(1.1). Fix (x, t) E n

T

and

suppose that

we

can

fmd

a cylinder of

the type

(8.1)

[(x,

t) + Q (aoR",

R)]

==

{Ix -

xl

<

R}

x

{l-

aoR"} , a

o

==

(~)

2-",

where A

is

an absolute constant, R is so small that

[(x,

t) + Q (aoR",

R)]

c

nT,

and w

is

any

positive number satisfying

(8.2)

sup

11.£1

:5

w.

[(z,l)+Q(ooRr>

,R)]

PROPOSITION

8.1. There exist constants eo,

1/

E (0,1)

and

C, A > 1 that can

be determined a priori depending only upon N and p, satisfying the following.

Construct the sequences

Ro =

R,

WO

= W

Rn

=C-nR,

Wn+l =

TJWn,

n = 1,2,

...

,

and the boxes

_

(

Wn)2-"

Q(n)

==

{Ix -

xl

<

Rn}

x

{l-

anR~,

l},

an

- A .

Thenfor all

n=O,

1,2,

...

and

essosc

1.£

:5

W

n

·

Q(n)

A consequence

is

the

HOlder

continuity of

1.£

at (x, t). A particular case

is

LEMMA

8.1. There exist constants

"'(

> 1

and

a E (0,

1)

that can be determined a

priori only in terms

of

the data, such that for all

0<

p

:5

R

essosc u(x, t)

:5

"'(Wo

(RP)Q

.

Ix-zl<p

Remark

8.1. This

is

a version of Lemma

2.1

of Chap.

IV,

stated for a 'fixed time'

l.

Remark

8.2. The constants A and C depend only

upon

N and p and

are

indepen-

dent

of

u.

Moreover they

are

'stable'

as

p

/2.

This follows

from

the remarks of

§3-(I) of Chap.

IV.

DiBenedetto E. Degenerate Parabolic Equations

Подождите немного. Документ загружается.