DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.

216 VIn. Degenerate and singular parabolic systems
for some a E (0, 1). This
is
the
focal
point
of
the theory.
Weak
solutions
of
elliptic
systems
in
general are not continuous everywhere within their domain
of
defini-
tion.
We
refer
to
[48J
for counterexamples and
an
account
of
the
theory.
Solutions
of
(1.1) are regular everywhere in
nT
because
of
the
special nature
of
the sys-
tem.
If
u solves (1.1), then the function
IDul
2
is a non-negative subsolution
of
a parabolic p.d.e. (1)
It
is
precisely such a property, which for elliptic systems
is
called 'quasi-subharmonicity'
,(2)
that permits one to prove (1.4) everywhere
in
nT.
These estimates can
be
extended
up
to t = °
if
the system
in
(1.1)
is
associated
with a
smooth initial datum
110.
They also carry over to the lateral boundary
of
nT
if (1.1)
is
associated with homogeneous either Dirichlet or Neumann data
on
ST
==
an
x
(0,
T). If the data are not homogeneous, the theory
is
fragmented and
incomplete.
In
the case
of
non-homogeneous Dirichlet data,
we
will show that
Ui
EC
6
(nx
(e,
T»
for arbitrary 6 E (0,1),
'rIe
E
(0,
T),
provided p > max
{I;
;~2}'
However the
key
estimate (1.4)
is
not known to
hold in such a case, and it
is
a major open problem
in
the theory.
The C
1
,o
regularity (1.4) requires a preliminary estimation ofthe type
(1.5)
IIDulloo.K:
:5
const,
IC
a compact subset
of
nT.
The
degenerate case
p>
2 and the singular case
pE
(1,
2)
are rather different with
respect
to
such an estimate. The function class
in
(1.1) implies
that(3)
(1.6)
If P >
2,
such integrability suffices to establish (1.5). If 1 < p <
2,
the
sup-bound
(1.5) can
be
derived only if further 'integrability'
is
assumed
on
lui.
Precisely,
(1.7)
lui
E
L1oc(n
T
),
where r
~
2 satisfies
Ar==N(p
-
2)
+ rp >
0.
This
is
analogous to the condition imposed
in
Theorem
5.1
of Chap.
V.
It implies
(1.4) and
in
addition
(1.8)
l-(i). Aboutthe singular
case
l<p<2
In
the degenerate case p >
2,
the behaviour
of
the solutions
of
(1.1)
is
entirely
a
local
fact.
In
particular the sup-bound (1.5) and the estimate (1.4) are a sole con-
sequence
of
u being a weak solution of (1.1).
If
1 < p < 2 due
to
the singular
(1)
See (3.3) in
the
Preface
or
(1.8)
of
Chap.
IX.
(2)
We refer
to
Meier
[77]
for some sufficient conditions for
an
elliptic system
to
be
quasi-subharmonic.
(3) See Proposition 3.1
of
Chap.
I.

1.
Introduction 217
nature
of
the p.d.e. some global infonnation is needed. This is not related to sys-
tems. Indeed it occurs also in Theorem 5.1
of
Chap. V to establish a sup-bound for
solutions
of
a single equation. Since our estimates involve u and
DU
t
the global
infonnation needed regards both the solution and its space gradient. Let r
~
2 sat-
isfy (1.7) and let U be a local weak solution
of
(1.1) for p E (1,2).
We
assume
that
{
U
can be constructed as the weak limit in L[oc(f1T)
ofa
(1.9) sequence
of
bounded subsolutions
{Un
her
of
(1.1)
satisfying in addition
IDunl
E
L~oc(f1T)'
We
stress however that all our estimates will depend only upon the quantities
lIull
r
,K;,
IIDull"K;,
x:;
a compact subset
of
f1
T
.
Such an assumption is not restrictive in view
of
the available existence theory(l)
and the special fonn
of
(1.1).
1-(ii). General structures
We will develop the theory for the homogeneous system (1.1). The same re-
sults however continue to hold for the following general class
of
quasilinear sys-
tems
(1.10)
~1J,'
-
div
A
(i)
(x t
Du)
=
B(i)
(x t u
Du)
in
nT,
at
I , , , , ,
i = 1,2,
...
,m,
where the functions
A(i)=(A(i)
A(i)
A(i»).
rl
xR
Nm
--+RN
-
l'
2 ,
...
, N .
UT
,
B(i)
:
f1
T
xRxR
Nm
--+
R,
i = 1,2,
...
,m,
satisfy the structure condition
(S3)
(1) See
Lions
[73).

218
vm.
Degenerate
and
singular
parabolic
systems
m
(8
5
)
2:
IB(i)1
~
C11Dul
p
-
1
+
!P2,
i=1
where C
i
,
i
=0,1.
are given positive constants and !Pi, i =
0,1,2,
are given non-
negative functions satisfying
(Ss)
#r
2 q
(t"l
) N
+2
!Po
+
!PI
+
!P2
E L
,oc
UT,
q >
-2-'
Remark
1.1. The structure condition
(8
2
)
is somewhat fonnal since there is no
stipulation that
Ut.z/cz;
have meaning at all. More correctly it should be written
with
Ut,z/cz;
and
DUi,Zi
replaced by tensors
~t,k,j.
Neverthless we prefer the for-
mal but suggestive fonn
of
(8
2
).
We will develop the main points
of
the theory for the model system (1.1) and
indicate later how to modify the arguments to include (1.10).
2.
Boundedness of
weak
solutions
We
will use the notation of§3
of
Chap. II. Thus Q (0,
p)
is the cylinder with 'vertex'
at the origin. Its cross sections are the cubes Kp and its height is
O.
The cylinder
[(xo,
to)
+ Q
(0,
p)]
has the 'vertex' at
(xo,
to)
and is congruenttoQ
(0,
p).
With (
we denote a piecewise smooth non-negative cutoff function in
Q
(0,
p)
vanishing
on the parabolic boundary
of
Q
(0,
p).
THEOREM
2.1
(THE
CASEp>2).
Letubealocalweaksolutionof{l.1).and
let p >
2.
Then for all e E (0,
21
there exists a constant
'Y
depending only upon
N,p,mande.
such thatfor every cylinder [(x
o
,
to)
+ Q
(O,p)1
C
flT
andforever
CTE(O,I).
(2.1) sup lui <
'Y
(Ojpp)IIE
(f!
l
u
IP
-
2
+£dXdT)
liE
[(zo,t
o
)+Q(u9,up») -
(1
- CT)(N+p)/E
(zo,t
o
)+Q(9,p»)
A
(~);!J
.
THEOREM
2.1
(THE
CASE 1 < p <
2).
Let u
be
a local weak solution
of
(1.1)
for 1 < p <
2.
Assume moreover that .
(2.2) lui E L
,oc
(flT),
r
~
1
Ar
-=N(P
-
2)
+
rp
>
0,

2.
Boundedness
of
weak
solutions
219
and that (1.9)
holds.
There
exists a constant
'Y
depending only
upon
N, p, m and
r
such
that/or every cylinder
[(xo,
to) + Q
(fJ,
p)]
c
flT
and/or every
CTE
(0,
I),
(2.3)
2-(i).
An
auxiliary proposition
The arguments are similar to
the
proof of local boundedness of solutions of a
single equation and are based on local energy inequalities which
we
derive
next.
We
set
(2.4)
lul=w.
PROPOSITION
2.1. Let u be a local
weak
solution
0/
the
system (1.1)
in
nT,
and let f(·}
be
a non-negative, bounded, Lipschitz function
in
R+.
There
exists a
constant
'Y='Y(N,p,
m},
such
that
(2.5) V(xo,
to}
E n
T
Vp,
fJ
> 0
such
that
[(xo,
to)
+ Q
(fJ,
p)]
C n
T
sup !
(1~f(8)d8)
("(x,
t}dx
to-9~t~O
0
(zo+K,.)
+ ! !IDw
l
"
f(w)("dxdr
+
!!IDUI,,-2IDwI2wf'(w)("dxdr
(zo,t
o
)+Q(9,p»)
(zo,t
o
)+Q(9,p»)
:5;
'Y
!!
w" f(w}ID(I"dxdr + 'Y!!
(1~f(8)dS)
(,,-l(t
dxdr
.
(zo,t
o
)+Q(9,p») (:I!o,t
o
)+Q(9,p»)
PROOF:
The weak fonnulation (1.2) can be rewritten
in
tenns of Steklov aver-
ages,as
(2.6)
!{!
Ui,htpi
+
[IDul,,-2
DUi]h·Dtpi}
dxdr =
0,
Vh E
(O,T),
n
VO<
t:5;T -
h,
V
tpi
E
W~'''(n)
n
L2(n)
i = 1,2,
...
, m
Since
/eUi,h
E
L?oA
n
T
),
this implies
(2.6)' !
Ui,h
- div
[lDul,,-2
DUi]
h = 0 a.e.
in
nT·
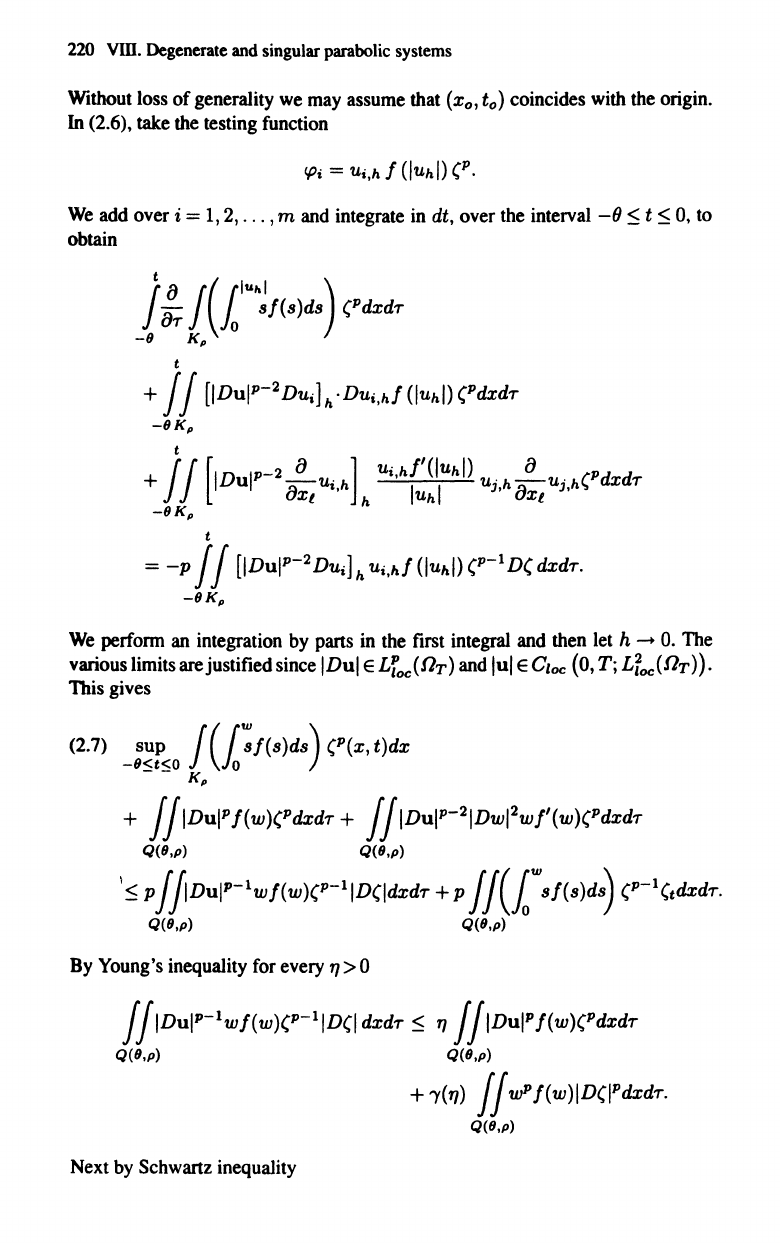
220
VID.
Degenerate
and
singular
parabolic
systems
Without loss
of
generality
we
may assume that (x
o
,
to)
coincides with the origin.
In
(2.6), take the testing function
We add over
i =
1,2,
...
, m and integrate in
dt,
over the interval
-()
$ t $ 0, to
obtain
j!
f(t;/(3)ds)
,'dxdT
-8
Kp
t
+ J J
[lDulp-2Dudh·Dui,h!(luhl)
(PdxdT
-8K
p
t
+ J J
[IDU
1P
-
2
a~l
Ui,h]
h
Ui'hr~~IIUhl)
U;,h
a~l
u;,h(PdxdT
-8K
p
t
=
-p
J J
[lD
u
l
p
-
2
DUi]
h
Ui,h!
(luhD
(p-l
D(
dxdT.
-8K
p
We perform an integration by parts in the
rust
integral and then let h
-t
O.
The
various limits are justified since
IDul
E
Lfoc(lh)
and
lui
EC,oc
(0,
T;
L~oc(l1T».
This gives
(2.7)
sup
J(
f~J(S)dS)
(P(x, t)dx
-8<t<O
10
- -
Kp
+ J
JIDu
lP
!(w)(PdxdT + JJIDuIP-2IDwI2W!'(w)(PdxdT
Q(8,p) Q(8,p)
'$
P JfiDuIP-IW!(W)(P-IID(ldxdT + p
!!(1wS!(S)dS)
(P-l(t
dxdT
.
Q(8,p) Q(8,p)
By
Young's inequality for every
1]
> 0
!
!IDuIP-1w!(w)(P-1ID(1
dxdT
$
1]
!!IDu
IP
!(w)(PdxdT
Q(8,p) Q(8,p)
+
-Y(1])
J J
wP
!(w)ID(IPdxdT.
Q(8,p)
Next by Schwartz inequality
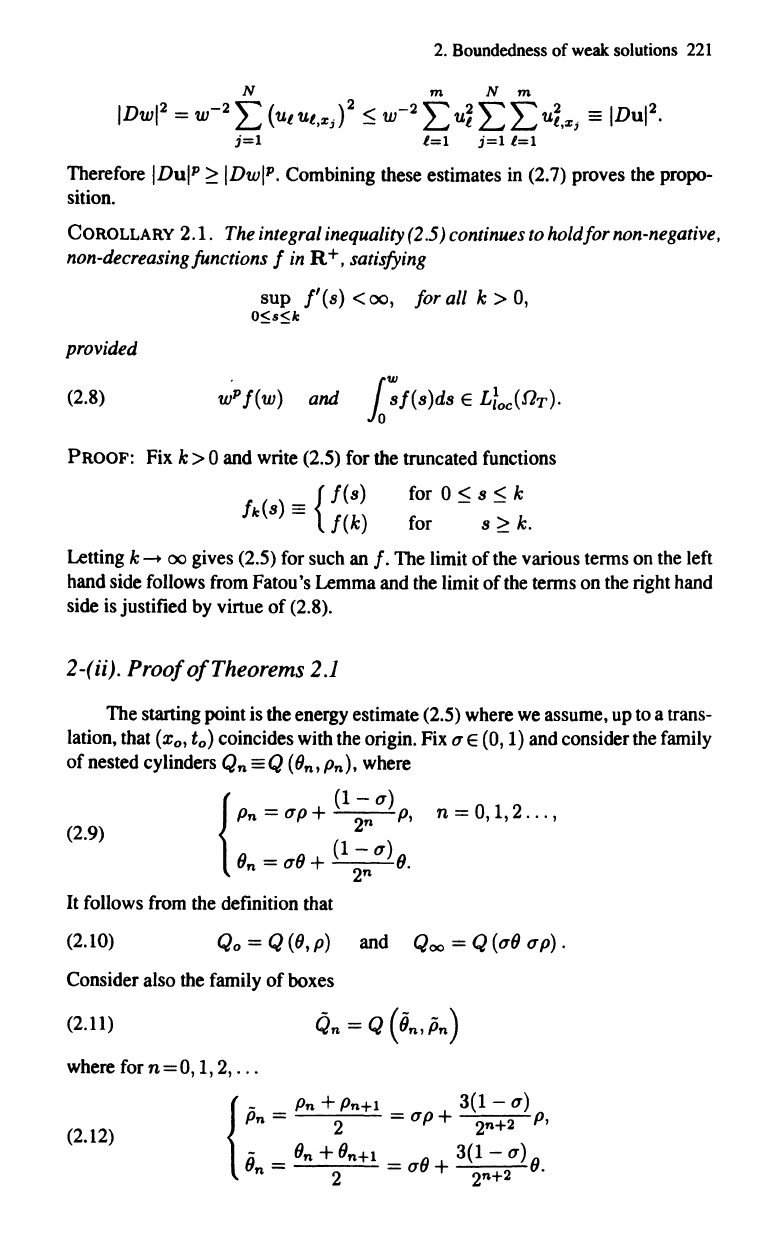
2.
Boundedness
of
weak
solutions
221
N m N m
IDwl2
=
w-
2
L
(Ul
Ul,z;)
2
$
W-
2
L
U~
L L
U~,z;
==
IDuI
2
•
;=1
l=1
;=1
l=1
Therefore
IDul
P
~
IDwI
P
•
Combining these estimates
in
(2.7) proves
the
propo-
sition.
COROLLARY
2.1.
The
integral inequality (2.5) continues
to
hold for non-negative,
non-decreasing Junctions f
in
R
+,
satisfying
provided
(2.8)
sup
/'(8)
<00,
forall k > 0,
O~s~k
PROOF:
Fix
k>
° and write (2.5) for the truncated functions
{
/(s)
fk(S)
==
f(k)
forO$s$k
for s
~
k.
Letting k
-+
00
gives (2.5) for such
an
f. The limit of the various terms
on
the left
hand side follows
from
Fatou's Lemma and
the
limit of
the
terms on
the
right hand
side
is
justified by virtue of (2.8).
2-(ii).
Proof
of
Theorems
2.1
The starting point is the energy estimate (2.5) where
we
assume.
up
to
a trans-
lation, that
(xo, to) coincides with the origin. Fix
uE
(0,1) and consider
the
family
of nested cylinders Qn
==Q
(6
n
,
Pn). where
{
Pn
=
up
+
(1;:
u)
p,
n =
0,
1,2
...
,
(2.9)
(1
_ u)
6
n
= u6 + 2
n
6.
It follows
from
the
definition that
(2.10)
Qo
= Q (6, p) and
Qoo
= Q (u6
up)
.
Consider also
the
family of boxes
(2.11)
where
forn=O,
1,2,
...
(2.12)
{
_
Pn
+ Pn+l 3(1 - u)
Pn
= 2 =
up+
2n+2
p,
8 = 6
n
+ 6
n
+l
= 6 3(1 - u) 6
n 2 u + 2
n
+
2
•

222 VIll. Degenerate and singular parabolic systems
For these boxes
we
have the inclusion
Qn+l
C
Qn
C Qn
Introduce the sequence of increasing levels
n =
0,1,2,
....
(2.13)
k
k
=k--
n 2
n
where k is a positive number to
be
chosen.
We
will
work
with
the
inequalities (2.5)
written for the functions
(u
- kn+l) +, over
the
boxes Qn. The cutoff function
(n
is
taken to satisfy
(2.14)
Set
(2.15)
{
(n
vanis~es
~n
the parabolic boundary of Qn
(n
==
1 m Qn
2
n
+
2
2
n
+
2
ID(nl
~
(1
_
(1)p'
0
~
(n,t
~
(1
- (1)6.
if
(8
- kn+l)
~
e
if 0 <
(8
-
kn+l)
< e
if
(8
- kn+d
~
0,
and
as
a function few) take
f~
[(w -
kn+l)+].
We
put these choices
in
(2.5)
and
neglect the non-negative term involving IDul
p
-
2
since f;(8)
~
O.
Letting e - 0
we
obtain
We
estimate the two integrals on the right hand side
as
in
(7.2)-(7.5) of Chap.
V.
This gives
the
inequalities
(2.16) sup
j(W-kn+d!(x,t)dx+
ffID(w-kn+1)+IPdxdT
-8
..
<t<O
J J
Kp.. Q
..
~
(1'Y~n;)p
(PPk
I6
_
p
+
6k!-2)
ff(w
-
kn)~
dxdT,
Q
..
valid for all 6
~
max
{Pi
2}.
If
P >
2,
the proof
is
now
concluded
as
in the
proof
of
Theorem
4.1
in
§
12
of Chap.
V.
If 1 < P <
2,
we
may
take 6 = r
in
(2.16)
and

3.
Weak
differentiability
of
IDulEy!
Du
and
energy
estimates
for
IDul
223
obtain the analog
of
the recursive integral inequalities (10.3)
of
Chap.
V.
The
proof
of
Theorem
2.1
for the singular case 1 < p < 2
is
now
concluded
as
in
the proof of
Theorem
5.1
in
§
16
of
Chap.
V.
3.
Weak
differentiability of
IDuI
P
;2
Du
and
energy
estimates
for
I Dul
The main tool
in
investigating
the
local behaviour of
the
of the space-gradient of
the
solutions of (1.1) are certain local energy estimates for
Ui,zj'
These are derived
by
first I differentiating' (1.1)
and
then
by
taking testing functions roughly speaking
of
the type
ipi
= Ui,zj f(lDul),
up
to
some
localising cutoff function.
Here
f(·) is a non-negative Lipschitz
func-
tion
in
R+.
In
this section
we
discuss a rigorous
way
of
carrying the indicated
calculations.
PROPOSITION
3.1
(THE
DEGENERATE CASE
p>
2). Let u
be
a
local
weak
solution
in
fiT
of
the
degenerate system (1.1).
Then
IDul2j!Ui,z;
EL1oc(o,
Tj
W1!;;(fi»)
,
i=I,2,
...
,m,
j=l,
2,
...
, N,
and
there
exists a constant 'Y='Y(N,p),
such
that
(3.1) j jIDuIP-2ID2UI2dxdT
[(zo,t
o
)+Q(119,l1p)]
where
Moreover
$
(I..?
0")2
[p-2
+ 0-1J j J (1+IDulfI)
dxdT
[(zo,t
o
)+Q(9,p)]
m N
ID
2
ul
2
==
L L
u~,z;z.·
i=1
j,k=1
(3.2)
Ui,zj
ECloc(O,
Tj
L1oc(n))
,
i=l,
2,
...
,
m,
j=
1,
2,
...
, N.
PROPOSITION
3.1
(THE
SINGULAR CASE 1 < p < 2). Let u
be
a
local
weak
solution
of
the singular system (1.1)
in
fiT and let
the
approximation assumption
(1.9)
hold.
Then

224
vm.
Degenerate
and
singular parabolic systems
E.jl 2
r.
12
~
. .
IDul
Ui,ZjELloc\O.TjWlo'c{fl);.
'=1.2
•...•
m.
J=1.2
•...•
N,
and
there
exists a constant 'Y='Y(N.p), such that
(3.3)
IIIDuIP-2ID2uI2dxdr
where
[(zo ,to
)+Q(
178,17
p»)
:5
(1
.?
0')2
[p-2 +
0-
2
]
(1
+
M;)
11(1
+
IDulP)
dxdr.
[(zo,t
o
)+Q(8,p»)
Moreover
Ui,z,
E
Lfoc
(0.
Tj
w,!;:(n))
.
and there exists a constant
'Y
=
'Y(
N.
p)
such
that
(3.4)
IIID
2
u
1P
dxdr
Finally
[(Zo ,to
)+Q(
0'8,0' p l)
:5
(1
.?
O')p
[p-P
+
O-P]
(1
+
M:)
11(1
+
IDuIP)
dxdr.
[(zo,t
o
)+Q(8,p»)
(3.5)
Ui,z,
EC1oc(O.T;L?oC<fl»).
i=1.2
•...•
m.
j=1.2
•...
,N.
This local regularity pennits to derive local energy estimates for
Du.
To simplify
the symbolism we set
(3.6)
v=IDul·
Given a cylinder [(x
o
• to) + Q
(0.
p)]
C flT we
let'
denote a non-negative piece-
wise smooth cutoff function in
[(x
o
• to) + Q (9.
p)]
that vanishes
on
the boundary
ofthe
cube
[xo
+ K
p].
In particular we
are
not requiring in general that , vanishes
for
t=to-O.

3.
Weak
differentiability of
IDul
~
Du
and
energy
estimates
for
IDul
225
PROPOSITION 3.2 (LOCAL ENERGY ESTIMATES). Let u
be
a local
weak
so-
lution
of
(1.1) for p >
1.
1n
the
singular
case
1 < p < 2
assume
in
addition that
the
approximation
assumption (1.9)
be
in
force.
Let
also
/ (
.)
denote anon-negative,
nOR-decreasing
Lipschitz function
in
R + .
There
exists a constant
'Y
=
'Y(
N,
p)
such
that
(3.7)
't/
(xo,
to)
E
nT,
't/
[(Xo,
to)
+ Q (9,
p)]
c n
T
t
sup ! (
r:/(S)dS)
(2
(X,
t)dx
to-9~t~O
J
o
t 9
[zo+K~)
0-
+ ! I vP-
2
1D
2
u1
2
/(v)(2dxdT
+ II vP-
1
1
Dv
1
2
/,(v)(2dxdT
[(zo,t
o
)+Q(9,p»)
[(zo,t
o
)+Q(9,p»)
+
(p
-
2)
t II vP-3IDv.DuiI2/'(v)(2dxdT
1=1
[(zo,t
o
)+Q(9,p»)
$
'Y
I I
vI'
/(v)ID(1
2
dxdT +
'Y
! I
(l:/(s)dS)
"t
dxdT
.
[(Zo,t
o
)+Q(9,p») [(Zo,t
o
)+Q(9,p»)
COROLLARY 3.1.
The
integral inequalities (3.7)
continue
to
holdfor
non-negative,
non-decreasing
functions /
in
R + , satisfying
provided
(3.8)
sup
/,(s)
<00,
forall k > 0,
O~.~k
PROOF:
Analogous to that
of
Corollary 2.1.
3-(i).
Taking
discrete derivatives
of
(1.1)
For a function
FE
Lfoc(nT) and T/ER\{O}. we introduce the discrete derivative
with respect to the
Xj
variable
CjF(x,t)==T/-l{F
(Xl,
...
,X; +
11,
..
.
,xN)-F(Xb'"
,X;,
..
"XN)}'
This is defined for
x E
nl'll
==
{x
En
I
dist(x,
an)
>
IT/I}
,
where we let
1111
be so small that
nl'll
is not empty.
We
also let
cF
denote the
discrete gradient
of
F ,i.e.,
