DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.

176
VI.
Harnack
estimates:
the
case
p>
2
Remark
9.1. The estimates above show that 'Y='Y(N,p)
/00.
as p\.,2.
Remark
9.2. The proof is independent
of
the fact that the initial datum is
of
com-
pact support and that
v is a solution in the whole 1:
00
• The lemma continues to hold
for every non-negative solution in
1:T
for some T >
O.
provided the quantities
lIu(·,
T)lIoo,B~
sup sup 2
O<?<r-E
p>r
ppl(p-)
are finite for all E E (0, T). The conclusion will hold for all times
(9.1)'
0<
t
~
'Y.llullr,T-E.
Remark
9.3. Lemma 9.1 is independent
of
the homogeneous structure
of
the
p.d.e. Indeed it continues to hold, in the same form, for equations with homoge-
neous structure as in (2.8)-(2.9)
of
Chap.
V,
provided the analog
of
Corollary 8.1
is
in force. A version
of
this Corollary can be proved, by essentially the same tech-
nique, for solutions
of
equations with general structure such as (2.1)
of
Chap.
V.
Remark
9.4. The functional dependence upon t on the right hand side
of
(9.1)
is
optimal. as shown by the following example.
The
family
(9.2)
{
...L..}~
I
I
p-l
.>.
-NI.>. X
Br(x,t)=(t+r)
1-'Y
p
(
11'>')
,
(t+r'>']
+
(
1);6
p-2
'Yp
= X
-p-'
p>
2;
t,
r > 0,
solves (8.1) with initial data B
r
{-,
0) supported in the ball B
r
•
By calculation we
have, for all
p
~
r
t
! !IDBrIP-ldxdT = 'Y(N,p)
[t
+
r'>']
11'>'
- r,
o
B2(1
where
'Y(
N,
p) is an explicit constant independent
of
rand
t.
The assertion follows
by letting r
-+
O.
Let
Eo
denote the integral average
of
the initial datum. i.e.
(9.3)
By the conservation
of
mass (8.4)
10. Proof
of
Proposition
8.1
continued 177
Ilvll
r
= sup sup
p-~fv(x,t)dx
teR+
p?r
B"
$ sup sup
p-~
f v(x, t) dx
teR+
p>r
-
RN
-r-~E
-
o·
Therefore Lemma 9.1 can be rephrased as
LEMMA
9.2. There exists a constant "Y="Y(N,p) such that
(9.4)
r
P
for
all 0 < t <
"Y.
EC-
2
'
andfor
all p
~
r
(9.5)
t 1
~
fflDvlP-ldxdT
$"Y
(:p)X
(;)~
E~+~.
OB"
1
O.
Proof of Proposition
8.1
continued
Let ( be the standard cutoff function in
B2r
that equals one on B
r
. In the weak
formulation
of
(8.1) take x
-+
(P
(x) as a testing function and integrate over B
2r
X
(0,
to), where
(10.1)
and e is a small positive constant to be chosen. Making use
of
(9.5) we obtain
to
f v(x,
to)
dx
~
2-
N
f
Vo
dx -
~
f f
IDvl
p
-
1
dx
B2.. B.. OB2
..
for the choice
We
summarise:
~
2-
N
Eo
-
"Y
(e"Y.)l/~
Eo
-
2-(N+l)
E
-
0,
178
VI. Harnack estimates: the case
p>
2
LEMMA
10.1.
There
exist a constant
c.
that
can
be
determined a priori
only
in
terms
of
N
and
p.
such
that
f v(x,
to)
dx
~
2-(N+l)
Eo,
B2r
Since v
is
continuous,
there
exists
some
x E
B2r
such that
(10.2)
Next
we
apply
the
Harnack estimate
of
Theorem
2.1.
For this, construct the cylin-
der
Q _ B
(-)
{ 4C
(6r)"
4C
(6r)"
}
=
46r
X X
to
- [v(x, t
o
)],,-2 '
to
+ [v(x, t
o
)],,-2 '
where
C
is
the
constant determined
in
Theorem
2.1
and
6 > 0
is
to
be
chosen. Such
a
box
is
contained
in
1:00
if
t >
4C(6r)"
0_[(
)],,2·
v x,to
Using (10.1)
and
(10.2)
we
see
that this is the case if
r"
4C(6r)"2(N+l)(p-2)
E'II
-->
,.
E~-2
-
g-2
Therefore Q c
1:00
for
the
choice
It follows
from
Theorem
2.1
that
"{Ix -
xl
<
6r}
_
4C(6r)"
at
the
time
level t =
to
+ " 2
[v(x,
to))
(10.3)
v(x, t)
~
-y-1
v(x,
to)
~
2-(N+1)-y-1
Eo
==
coEo·
Therefore
we
have located a ball or radius
6r
about x and at the time level f
where
v
is
bounded
below
by
eoEo.
In
view
of(10.1) and (10.2) the time level
tis
bounded
above
by
(10.4)
11.
Proof of
Proposition
8.1
concluded
179
11.
Proof of Proposition
8.1
concluded
Let
(J
> 0
be
fixed.
We
may
assume that
(11.1)
Indeed otherwise
(
8);;!-'
Eo:5 B r
P
,
and (8.2) becomes trivial.
We
will
expand the bound below
on
v given
by
(10.3),
up
to
the
time
level 8 over
the
ball B
r
•
Consider the 'fundamental solution'
8/c,p
introduced
in
(3.1)-(3.2),
with
pole at
(x,
t) and
p=6r,
By
the
cQmparison
principle,
(11.2)
vex,
t)
~
8
co
E
o
.6r
(x,
tj
x,
t) , V x E R
N
,
Vt
~
f.
Let
us
estimate below the right
hand
side of
(11.2)
at
the
time
level
t=8.
First
by
(10.4) and (11.1)
- 1
8-t>-8
- 2 '
therefore the support of
X-+8
co
E
o
.6r (x,
8j
x,
t)
will
cover
the
ball B
4r
about
the
origin if
8(8) = b(eoEo)p-2 (6r)N(p-2)
(8
- t) + (6r)'\
~
~
(c
o
E
o)P-2
(6r)N(p-2) 8
~
(8r)".
This will occur if
(11.3)
We
may
assume that (11.3)
is
in
force and estimate above
8(8)
:5
b [eo6Nt-2 E:-
2
r
N
(p-2)
8 + 6'\r
N
(p-2)
r
P
:5
{b
[eo
6N
t-
2
+
~:}
~-2
r
N
(p-2)
8
==
6
1
E:-
2
r
N
(p-2)
8.
We
return
to
(11.2).
These estimates imply that for
all
x E
Br
for t = 8

180 VI. Harnack estimates: the case
p>
2
Therefore
f
(
0 ) N III [ ]
>'111
Eo
==
Vo
dx
:$
6;>'lp r
P
W!
v(·,
6}
,
Br
and
the Proposition follows with
B=max{(2Bl}~;
BF;
6;>'11I}.
12. The Cauchy problem with compactly supported
initial data
1be proof
of
(7.2) is based on comparing u with the unique solution
of
the Cauchy
problem
(12.1)
{
V
E C
(R+;
L2(RN}) n V
(R+;
Wl'II(RN»
,
Vt
-
div
IDvllI-2Dv = 0 in
!Joe
==
RN
xR+,
(
)
{
EC(Br}
forsomer>O,
v
,,0
=
Vo
~
0 and _ .
RN\B
= 0
10
r.
Such a problem plays a role also in the theory
of
Harnack estimates for non-
negative weak solutions
of
(1.1) in the singular case 1 < p <
2.
The Cauchy
problem for general initial data in
Lloc
(R
N)
and all p > 1 will
be
studied in
Chaps.
XI
and
XII.
To
render the theory
of
Harnack inequalities self-contained
we briefly discuss the unique solvability
of
(12.1) for all p >
1.
First, the notion
of
solution is:
(a) For every compact subset
JC
c
RN
and for every T >
0,
u is a local solutions
of
the p.d.e.
in
JC
x
(0,
T), in the sense
of
(1.2)-(1.4)
of
Chap.
II.
(b) v(·,
t}
-+
Vo
in L2(RN}.
PROPOSITION
12.1.
There
exists a
unique
solution
to
(12.1) for all p >
1.
PROOF:
For n =
1,
2,
...
let Bn
be
the ball
of
radius n about the origin and con-
sider the boundary value problems
{
Vn
E C
(R+;
L2(Bn»
n V
(R+;
W:'II(Bn»
(12.2)
Vn,t
- div IDv
n
lp-2
DV
n
= 0 in Bn x (0, n),
vn(-,O} =
Vo
E L2(Bn}.

12.
The Cauchy problem with compactly supported initial data
181
The functions
Vn
vanish in the sense
of
the traces on
Ixl
= n.
We
regard them as
defined in the whole
Eoe
by extending them to zero for
Ixl
> n. The problems
(12.2) can be uniquely solved by a Galerkin(l) procedure and give solutions
Vn
satisfying
(12.3)
The sequence
{v
n
}n6N
is equibounded(2) in E
oe
,
and uniformly Holder continu-
OUS(3) in
Ex
(e,
00)
for all e >
O.
In the weak formulation
of
(12.2), we take the
testing function
(v
n
+ e )p-2
Vn
, modulo a Steklov average. Letting e - 0 gives
(12.4)
VneN.
Therefore
Vn
e
LP
(R+; W1,P(R
N
))
uniformly in n.
A subsequence can be selected and relabelled with n such that
Vn
- v uniformly
on compact subsets
of
Eoe
and weakly in
LP
(R+; Wl'P(RN)). The limit v is
in the function space specified by
(12.1), it is
HOlder
continuous in
Ex
(e,
00)
for all e > 0, and it satisfies the p.d.e. weakly in
Eoe.
To
prove this
we
select a
compact subset
Ie
c
RN
and some T >
O.
Then
if
n is so large that
Ie
C B
n
,
we
write
(12.2) weakly against testing functions supported in
lex
(0,
T). The limiting
process can be carried on the basis
of
the previous compactness and the non-linear
term is identified by means
of
Minty's Lemma. (4)
It
remains to show that v takes
the initial
data
Vo
in the sense
of
L2(RN). Let
1/
e
(0,
1)
be arbitrary and let
VO,'l
be a mollification
of
Vo
such that
!lvo
- V
O
,'l!l2,RN
--+
0 as
1/
'\.
O.
In the weak formulation
of
(12.1), take the testing function
Vn
-
vo,'l
modulo a
Steklov average.
If
n is so large that
supp[VO,'l]
C B
n
,
we obtain
t
jlvn
- VO,'l12(t)dx
:::;
!lvo
-
VO''lIl~,RN
+
'Y
j jIDVo''lIPdxdT,
"It>
0,
RN
ORN
for a constant
'Y
depending only upon p. Letting n -
00,
t
IIv(·,
t) -
voll~,K:
:::;
211vo
-
VO''lIl~,RN
+
'Y
j jIDVo''lIPdXdT,
"It>
0,
ORN
(1) See J.L.Lions
[73]
or
Ladyzhenskaja-Solonnikov-Ural'tzeva
[67].
(2)
By
the
weak maximum principle
of
Theorem 3.3
of
Chap.
V.
(3)
By
the
HOlder
estimates of Theorem 1.2
of
Chap.
III
and
Theorem 1.2
of
Chap. IV.
(4) See G. Minty
[78].

182
VI.
Harnack
estimates:
the
case
p>
2
for all compact subsets
IC
eRN.
From this,
]~
IIv("
t)
- V
o
Il2,K;
=
211vo
- V
o
,,,1I2,RN,
for all
'1
E (0,1).
To
prove uniqueness
we
first write the p.d.e. satisfied by the difference W =
Vl
-
V2
of two possibly distinct solutions originating from
the
same
initial datum
vo,
i.e.,
{
wE
C
(R+;L2(RN»)
nLP
(R+;Wl,P(R
N
»),
(12.5)
We
- div
(IDVIlp-2
DVI
-
IDV2l
p
-
2
DV2)
= 0,
in
1:
00
,
w(',O) =
0,
in
L~oc(RN).
In
the
weak
formulation of (12.5), take the testing function
w(,
modulo a Steklov
average,
where
x
-+
«x)
is
a non-negative piecewise smooth cutoff function
in
the ball
B2R
that equals one on
BR
and such that
ID(I
~
1/
R.
This gives, for all
t>O,
t
~/IWI2(t)dx
+ / /<IDVIIP-2
DVl
-IDV2lp-2Dv2,Dvl -
DV2)
(dxdT
Bil
OB2Il
e
= - / !<IDVIIP-2DVI-IDV2IP-2DV2,D()WdxdT.
OB21l
1be second integral on
the
left hand side is non-negative(l) and it
is
discarded.
Therefore
! IwI2(t) dx
~
IIwll
p
,l;oo
(IIDVlllp,l;; +
IIDV2l1p,l;oo
)P-l
Bil
Uniqueness follows letting R
-+
00.
A similar argument proves the following weak comparison principle.
LEMMA
12.1. Let
Vi,
i = 1,2. be
two
weak solutions
to
(12.1) originating
from
bounded and compactly supported initial data
Vo,i,
i=
1,
2.
satisfying
Vo,l
~Vo,2'
Then
Vl
~
V2
in
Eoo.
PROOF:
In
the weak formulation of
the
difference w =
VI
-
V2,
take the testing
function
w+(
modulo a Steklov average.
(1) See Lemma
4.4
of
Chap.
I.
13.
Bibliographical
notes
183
13. Bibliographical notes
In the classical work
of
Moser [81,82,83],
~e
HOlder continuity is implied by the
Harnack estimate. Conversely we use the HOlder estimates
of
Chaps.
III
and N
to establish a Harnack inequality. This point
of
view, even though not explicitly
stated, is already present in the work
of
Krylov and Safonov [64]. The results
of
§2 have been established in [40]. A version
of
these holds for non-negative weak
solutions
of
the porous medium equations
(13.1)
{
u
E C'
oc
(0,
Tj
L~oc(fi»)
,
urn
E
L~oc
(0,
Tj
W,!;;(fi») ,
! u -
.::lu
rn
= 0 in fiT, m >
1.
In particular the intrinsic Harnack estimate takes the form
THEOREM
13.1. Let u
be
a non-negative weak solution
0/
(13.1). Fix any
(xo,
to)
E fiT and assume that u(xo,
to)
>
O.
There
exist constants
'Y
> 1 and
C>
1, depending only
upon
Nand
m, such that
(13.2)
provided
the
cylinder
Q4p(9)
==
{Ix -
xol
< 4p} x
{to
-
49,
to
+
49}
is contained
in
fiT.
A version
of
Corollary
2.1
for (13.1) appears in [6]. For the remaining results
we refer to [40]. The 'fundamental solutions'
Blc,p
are due to Barenblatt [8]. The
comparsion function
(ilc,p
for p close
to
2, is introduced in [40]. The technical
device
of
the family
of
expanding cylinders
Q.,.
in §4 appears in Krylov
-Safonov
[64]. The regularising effects
of
Proposition 6.1 are due to Benilan and Crandall
[9].

VII
Harnack
estimates
and
extinction
profile
for
singular
equations
1.
The Harnack inequality
We
will investigate the local behaviour of non-negative solutions of
the
singular
p.d.e.,
{
u
E
C,oc
(0,
T;
L~oc(n»)
n
Lfoc
(0,
T;
W,!:(n)),
1
<p<2
(1.1)
Ut
- div IDulp-2
Du
=
0,
in
UT.
Weak
solutions of (1.1) exhibit
an
intriguing behaviour. Even though
in
general
they are not locally bounded,(I) they might become extinct after a finite time. It
turns out however that the Harnack inequality of Theorem
2.1
of Chap.
VI
contin-
ues
to hold provided p satisfies the further restriction
(1.2)
2N
N + 1
<p
<
2.
We
will show that such a range of p
is
optimal for a Harnack estimate to hold. The
extinction
in
finite time,
the
Harnack inequality
and
the
LOO-estimates are linked
by
the
range (1.2) of the parameter p.
THEOREM
1.1. Let u be a non-negative weak solution
of
(
1.1
) and let (1.2) hold.
Fix
any (x
o
,
to)
E
nT
and assume that u(x
o
,
to)
>
O.
There exist constants
'Y>
1
and cE
(0,
1). depending only upon
Nand
p. such that
(1.3)
(I)
See §5-(IV) of Chap.
V.
1.
The Harnack inequality
185
where
(1.4)
provided the cylinder
(1.5)
Q4p(0)
==
{Ix
-
xol
<
4p}
x
{to
-
40,
to
+
40}
is contained in
nT.
Remark
1.1. The statement
of
Theorem
1.1
is the same as that
of
Theorem
2.1
of
Chap. VI except that now the constant c is 'relatively small'; that is, the positivity
of
u(xo,
to)
spreads over the ball Bp(x
o
) but is preserved only for the 'relatively
small' time c
[u(xo,
t
o
)]2-
P
pp.
Remark
1.2. As p
'\.
J~l'
the constant
"Y
tends to infinity and c tends to zero.
However these constants are 'stable' as p
/'
2. i.e.,
lim "Y(N,p),
c-
1
(N,p) =
"Y(N,
2),
c-
1
(N,2) <
00.
p/2
Therefore the classical Harnack inequality for non-negative solutions
of
the heat
equation can be recovered by letting p
/'
2 in (1.3). The limiting process can be
made rigorous by the
C,7/:
(n
T
)
estimates
of
Chap. IX.
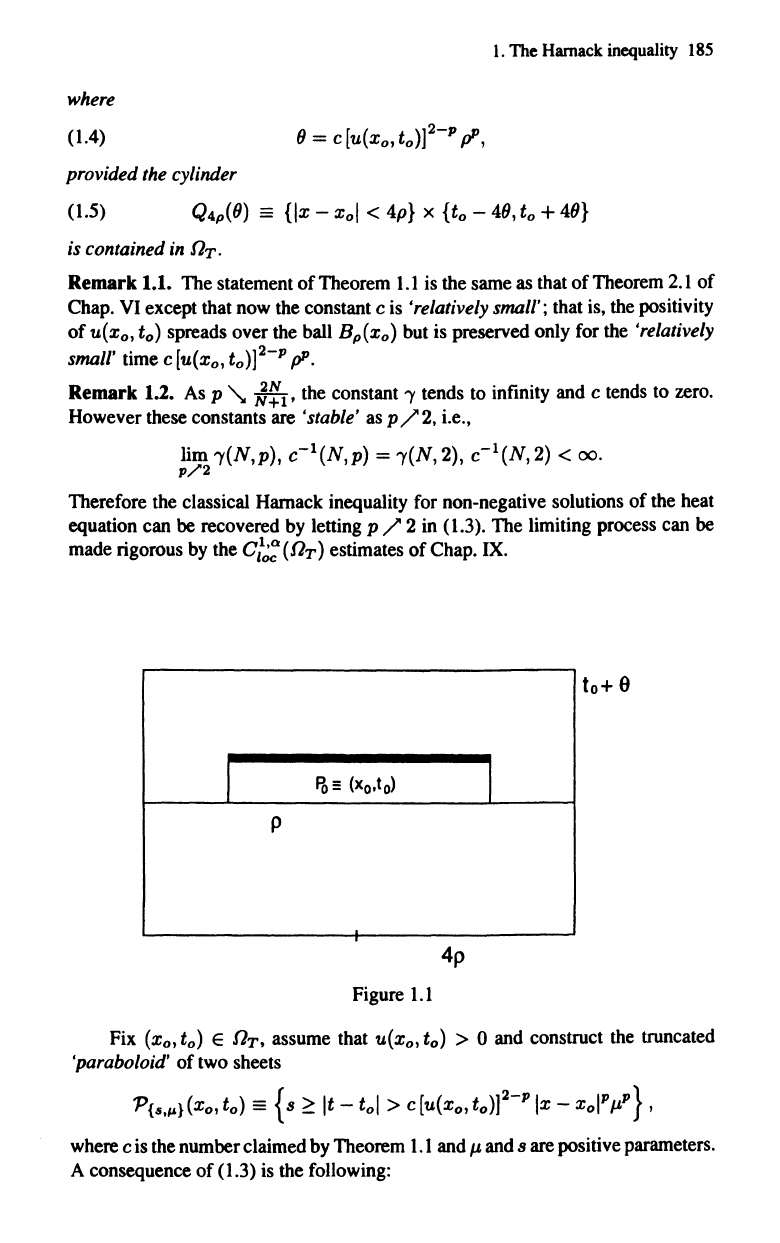
t
o
+ a
I
I
p
4p
Figure
1.1
Fix
(xo,
to)
E
nT,
assume that u(x
o
,
to)
> 0 and construct the truncated
'paraboloid'
of
two sheets
"{s.,,}
(x
o
,
to)
==
{ s
~
It
-
tol
> c
[u(xo,
t
o
)]2-
P
Ix -
xol
P
IJP
} ,
where c is the number claimed by Theorem
1.1
and
IJ
and s are positive parameters.
A consequence
of
(1.3) is the following:
