DiBenedetto E. Degenerate Parabolic Equations
Подождите немного. Документ загружается.


VI
Harnack estimates: the case p > 2
1.
Introduction
We
will establish a Harnack-type estimate
for
non-negative
weak
solutions of
de-
generate parabolic equations of
the
type
{
U
E
Gloc
(0,
Tj
L~oc(ll})
n
Lfoc
(0,
Tj
WI!;:(ll)) ,
p>
2,
(1.1)
Ut
- div
IDulp-2
Du
=
0,
in
llT.
Since
the
equation
is
invariant
by
the scaling x - hx, t - hPt, h > 0. it
may
seem
plausible
that
the Harnack estimate of Hadamard [50]
and
Pini [86],(1) would hold
in
the geometry of the cylinders
(1.2)
This
is
not
the
case,
as
one
can verify
for
the explicit solution (x, t) -
8(x,
t)
in-
troduced
in
(4.7) of
Chap.
v.
Let
(xo, to)
be
a point of
the
free boundary
{t=
Ixl>'},
and let
p>
1.
Then if
to
is
sufficiently large, the ball Bp(x
o
)
taken
at
the
time
level
to
-
pP
intersects the support of x - 8 (x,
to
-
PP)
in
a open
set.
Therefore
sup
8(x,to
-
PP)
> °
Bp(zo)
and
This reveals a
gap
between the elliptic theory
and
the corresponding parabolic
theory.
Indeed non-negative
weak
solutions of
(1) See (2.2) in
the
Preface.

2. The intrinsic Harnack inequality 157
div
IDul
p
-
2
Du
= 0, u E W,!;:(fl), p > 1,
satisfy the Harnack inequality,
(2) whereas solutions
of
the corresponding parabolic
equation (1.1) in general do not.
Let
u
be
a non-negative local solution
of
the heat equation in flT. Then for all
e> 0 there exists a constant
"Y
depending only upon N and
e,
such that for every
cylinder
Qp(x
o
,
to)
C flT and for every
uE
(0, 1),
(1.3)
sup
u <
"Y
N 2 ( ff u
E
dxdr )
~
,
Q .. ,,(Xo,t
o
) -
(1
-
u).;¥
Q,,(xo,t
o
)
where Qp(x
o
,
to)
is defined by (1.2) with
p=2.
This local sup-bound
of
the solu-
tion in tenns
of
the integral average
of
a
small
power
of
u,
is a key fact in Moser's
proof
of
the Harnack estimate. An estimate
of
this kind does not hold for solutions
of
(1.1) and it is replaced by the more structured inequality (4.1)
of
Chap.
V.
A
study
of
[83] however reveals that (1.3) continues to hold for sufficiently smooth
solutions
of
(1.4)
With this in mind one may heuristically regard (1.1) as it were (1.4) written in
a time scale intrinsic to the solution itself and, loosely speaking,
of
the order
of
t [u(x, t)]2-
p
.
Next we observe that (2.2) in the Preface is equivalent to
(1.5)
The Harnack estimate
of
Krylov and Safonov [64] for non-divergence parabolic
equations is given precisely in this fonn.
This suggests that the number
[u(xo,
t
o
)]2-
P
is the intrinsic scaling factor and
leads
to
conjecture that non-negative solutions
of
(1.1) will satisfy the Harnack
inequality with respect to such an intrinsic time scale.
2. The intrinsic Harnack inequality
The following theorem makes rigorous the heuristic remarks
of
the previous sec-
tion.
THEOREM
2.1. Let u
be
a non-negative weak solution
off
1.1). Fix
any
(xo,
to)
E
flT and assume that u(xo,
to)
>
o.
There
exist constants
"Y
> 1 and C >
1,
depend-
ing
only
upon
N and
p,
such
that
(2.1)
(2) See [82,92,96).
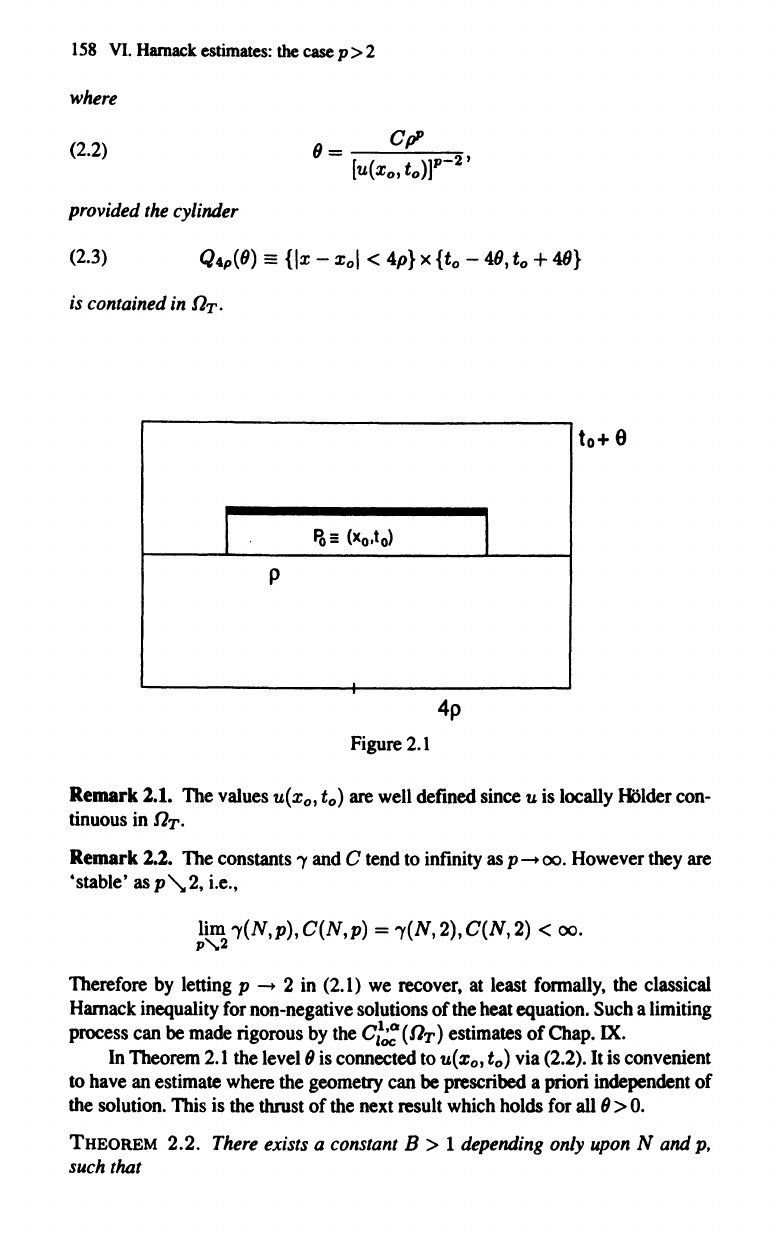
158
VI.
Harnack
estimates:
the
case
p>
2
where
(2.2)
provided
the
cylinder
(2.3)
Q4p(9)
==
{Ix
-
xol
< 4p} x
{to
-
49,
to
+
49}
is
contained
in
n
T
•
t
o
+ 0
I .
J
p
4p
Figure
2.1
Remark
2.1.
The
values
u(xo,
to)
are
well
defined
since
u
is
locally
Wider
con-
tinuous
in
nT.
Remark
2.2.
The
constants
"'(
and
C
tend
to
infinity
as
p -
00.
However
they
are
'stable'
asp'\.2,
i.e.,
lim
",(N,p),
C(N,p)
= "'(N, 2),
C(N,
2)
<
00.
",,"2
Therefore
by
letting p _ 2
in
(2.1)
we
recover.
at
least fonnally,
the
classical
Harnack
inequality
for
non-negative
solutions
of
the
heat
equation.
Such
a
limiting
process
can
be
made
rigorous
by
the
C,~
(nT ) estimates of
Chap.
IX.
In
Theorem
2.1
the
level
9
is
connected
to
u(
%0'
to)
via
(2.2). It
is
convenient
to
have
an
estimate
where
the
geometry
can
be
prescribed a priori
independent
of
the
solution.
This
is
the
thrust
of
the
next
result
which
holds
for
all
9 >
O.
THEOREM
2.2.
There
exists a constant B > 1
depending
only
upon
N and
p.
such
that

3.
Local
comparison
functions
159
(2.4) V (xo,
to)
E nT.
Vp,
(J
> 0
such
that
Q4p((J)
c nT.
u(xo,
to)
~
B {
(~)
~
+
(;)
NIp
[B!?!o)
u(·,to +
(J)f/J],
where
(2.5)
.\
= N(p -
2)
+ p.
Remark
2.3. Inequality (2.4) holds for all p E (2,00), but the constant B
is
not
'stable' as p
'\,2,
i.e.,
lim
B(N,p)
= 00.
p'\,2
In
(2.4) the positivity
of
u(x
o
,
to)
is
not required and
(J
> 0
is
arbitrary so
that
Theorems 2.1 and 2.2
may
seem
markedly different. In
fact
they
are
equivalent,
i.e.,
PROPOSITION
2.1.
Theorem2.1
<=>
Theorem
2.2.
In view'of Remark 2.3, the equivalence
is
meant
in
the sense that
(2.1)
implies
(2.4)
in
any case
and
(2.4) implies (2.1)
with
a constant
"(
= ,,((N,p)
which
may
not be 'stable' as
p'\,2.
A consequence of Theorem
2.2
is
COROLLARY 2.1.
There
exists a constant B > 1
depending
only
upon
N and p,
such
that
(2.6)
V(xo,
to)
E nT, V
p,
(J
> 0
such
that
Q4p((J)
c nT,
ju(z,t.)dx"
B{
(~t
+
(;
t
P
[U(Z.,t.+9)J
VP
}
B,.(zo)
2-(i).
Generalisations
All
the
stated results remain valid if
the
right hand side of (1.1) contains a
forcing term
f, provided
(2.7)
q>
(N
+p)/p
and f
is
non-negative.
We
wiD
indicate later
how
to
modify the proofs to include
such a case.
3.
Local comparison functions
Let
p> 0 and k > 0 be fixed and consider the following 'fundamental solution' of
(1.1) with pole at
(x,
t):
160
VI.
Harnack
estimates:
the
case
p>
2
~
_ kpN { (
Ix
-
xl
)
~
}
p-
Bk,p
(x,
tj
x,
l)
==
SN/>'(t) 1 - Sl/>'(t)
+'
(3.1)
where
A
is
defined
in
(2.5)
and
(3.2)
S(t) = b(N,p)kP-
2
p
N(p-2)(t
-l)
+
p>',
t
2:
f,
( )
P-l
b(N,p) = A
p~
2
By
calculation,
one
verifies that
Bk,p
(x, tj
x,
l)
is
a
weak
solution of (1.1)
in
RN
x
{t > l}. Moreover
for
t = f
it
vanishes outside
the
ball B p (x)
and
for t > f the
function
x-Bk,p
(x,
tj
x,
l)
vanishes,
in
a
Cl
fashion, across the boundary of
the
ball
{Ix
-
xl
< Sl/>'(t)}.
One
also verifies that
Bk,p
(x,
tj
x,
l)
~
k,
and that
for
f
~
t
~
t*,
the
support of
Bk,p
(x,
tj
x,
l)
D*
==
{IX -
xl
~
SI/>'(t)} x [f,
t*J,
is
contained
in
the cylindrical domain
Q*
==
BS1/1I(t.)(x)
x [f,
t*J.
If
u
is
a non-negative
weak
solution of (1.1)
in
Q*
satisfying
u(x,l)
2:
k
for
Ix
-
xl
< p,
then
u(x,
t)
2:
Bk,p
(x,
tj
x,
l)
,
'v'(X,t)EQ*.
This
is
a consequence of
the
following comparison principle.
LEMMA
3.1. Let u
and
v
be
two solutions
of
(1.1) in
nT
satisfying
{
u,v
E C (O,Tj L
2
(n»)
n £P (O,Tj W
1
,p(n»
n C
(liT)
u
2:
v on the parabolic boundary
of
nT.
Then
u2:v
in n
T
.
PROOF:
We
write
the
weak
form
of (1.1) for u
and
v
in
terms of
the
Steklov-
averages,
as
in
(1.5) of
Chap.
II,
against the testing function
[
t+h
1
[(v
-
U)hJ+
(x, t) =
*!
(v
- u)(x,
'T)d'T
+'
h E
(0,
T),
t E
[0,
T - h).
Differencing
the
two
equations and integrating over
(0,
t)
giv\
's
3. Local
comparison
functions
161
j[(v
- U)h]! {x, t)dx -
j[{V
- U)h]! (x,
O)dx
n n
=
-2//
[lDvl
p
-
2
Dv
-IDuI
JI
-
2
Du] h·D
[{v
-
U)h]+
dxdr.
n.
As
h
-+
0 the second tenn
on
the left hand side tends to zero since
(v
- u) + E
C (liT). Applying also Lemmas
3.2
and 4.4 of Chap. I
we
arrive at
/{v
-
u)~{x,
t)dx
n
=
-2
/ / (IDvIJl-2 Dv -IDuI
JI
-
2
DU)
·D{v - u)dxdr
:5
O.
n.n(v>u)
3-(i). Local comparison/unctions: the case p near 2
The next comparison function
is
a subsolution of (1.1) for p > 2
and
for p < 2
provided p
is
close enough to
2.
For definiteness let
us
assume p E [2,5/2]
and
consider the function
(3.3)
(3.4)
t
~
f,
where the positive numbers
II
and
~(II)
are
linked
by
(3.5)
~(II)
= 1 - v(p -
2)
.
P
Introduce
the
number
(3.6)
p{v) = 4(1 + 2v)/{1 + 4v),
and
observe that
(3.7)
1 1
4
:5
~(v)
:5
2'
for p E
[2,
p(v)].
LEMMA
3.2. The number
v>
1 can be determined a priori only in terms
of
N
aTul
independent
ofpE
[2,
5/2], such that
Qk,p
is a classical subsolution
of
!Qk,P - div
(I
DQk,p
I
JI
-
2
Dgk,P)
:5
0 in
aN
x {t > t}.
PROOF:
For (x, t) E
aN
x {t>t}, set
162
VI.
Harnack estimates:
the
case
p>
2
IIzll
==
t(~)
~~)
,
:F
==
(1
-
IIzll;!r
) + ' a
==
(p
~
1)
2.
Then. by calculation.
(3.8) £*
(g/c,p)
=
-v:F;!r
+ NaP-1:F
- -LaP-1Ilzll;!r +
~(v)a:F;!r
IIzlI;!r.
p-l
Introducing
the
set
[
--Z..-
1 (
N(P-l»)]
£1
==
IIzlIFT
~
2
1+
N(P-l)+p
,
we
have
:F
< p
in
£1,
- 2[N(P - 1) +
p]
and therefore by (3.5)
r:
(g/c,p)
:5
-v:F;!r
+
Na
P
-
1
+
~(II)a:F;!r
- a
P
-
1
(N
+
_P-)
IIzll;!r
p-l
:;;
...-1
[-\(v)
(N(P
-"1) +
p)
o!t
-
2(P~
1)
1
:;;
.p--l
[H
N(P -"1) + p t -
-::-:2(p......;;~~I)
1
:5
a
P
-
1
(~
-
2(P~
1»)
<
O.
Within
the
set
[
;!y
1 ( N(P -
1)
)]
£2
==
IIzll
p-
< 2 1 + N(P _
1)
+ p ,
we
have
:F> P
-
2[N(P-l)+p]
It
follows
from
(3.5)
and
(3.7) that
(
p
);!r
£*
(g/c,p)
:5
-v
2[N(P _ 1) +
p]
+ a
P
-
1
N +
~(II)a
(
p
);!r
a
-2
:5
-II
2[N(P _
1)
+
p]
+ p
[NpaP
+
1]
.
4. Proof
of
Theorem
2.1
163
Choosing
(3.9)
V==
max
~
[NpaP-2+1]
(2[N(P-l)+P])~,
pe[2,5/2) P P
we have in either case
in
RN
x{t
> t}.
One verifies that for t = t
(hc,p
(XI
tj
X,
f)
$ k,
and that for t $ t $ t*, the support
of
(hc,p,
'R,*
==
{Ix
-
xl
<
L'''(II)
(t) } x [t I t*],
is contained in the cylindrical domain
C*
==
{Ix
-
xl
< L'''(II)(t*)} x [t I t*].
Therefore
if
u is a solution
of
(1.1) in C* such that
U(X,
f)
~
k
then
U(X,
t)
~
(h:,p (x,
tj
ft,
f)
in C*.
Remark 3.1. The same proof shows that
gkr
is a
sub-solution
of
0.1)
also for
p<2,
providedp
is close to 2. Precisely
if
pE
(4 -
p(v),2)
..
4.
Proof of Theorem
2.1
Let (xo, to) E n
T
and p > 0
be
fixed, assume that U(XOI to) > 0 and consider the
box
where
C is a constant to
be
detennined later. The change
of
variables
X-Xo
x----
P I
maps Q
4p
into the box Q
==
Q+
U
Q-
, where
Q+
==
B4
X
[0,
4C), Q-
==
B4
X
(-4C
,
0].
164 VI. Harnack estimates: the case
p>
2
(0,0)
I
I
,
•
---.----------.-----~
c/o
1
Figure
4.1
We
denote again
with
x
and
t
the
new
variables. and observe that the rescaled
function
1 (
tpp)
v(x,t)
= (
t)
U xo+px, t
o
+ [ (
)IP-2
U x
o
, 0 U xo,to
is
a bounded non-negative weak solution of
{
Vt
- div
(lDvlp-2
Dv)
= 0
in
Q
v(O,O)
= 1.
To
prove
the
Theorem it suffices
to
find
constants
'Yo
E (0,11
and
C>
1 depending
only
upon
N and p such that
ill!
v(x, C)
~
'Yo·
Construct the
family
of
nested
and
expanding
boxes
and
the
numbers
M.,.
==
sup v,
N.,.
==
(1-
T)-fJ,
Q
..
T E
(0,
I],
T E [0,1),
where
{J
> 1
will
be
chosen
later.
Let
To
be
the
largest root of the equation
M.,.
=
N.,..
Such a root
is
well defined since
Mo
=
No.
and
as
T
/1.
the numbers
M.,.
remain
bounded and
N.,.
/00.
By
construction
Since v
is
continuous
in
Q there exists within
Q.,."
at
least one point.
say
(x, t).
such that
v (x, t) =
N.,."
=
(1
- To)-fJ.
The next arguments are intended
to
establish that within a small ball about x
and
at
the same time-level t
the
function v is of
the
same
order of
(1-
To)-fJ. For this
we
make
use
of the R)}der continuity of v
and
more specifically
of
Lemma
3.1
of
Chap.
III.
Set
R =
1-
To
2 '
4.
Proof of
Theorem
2.1
165
and consider
the
cylinder
with
'vertex' at
(x,
t)
[(x,t) + Q (RP,R)]
==
{Ix
-
xl
< 1
~
To
} X
{t
_
(1;
To
)
P,
t}.
By
construction
[(x,
t) + Q
(RP,
R)] c Q!:tfa
and
therefore
sup
v $ N!:tfa =
2.8(1-
T
o
)-.8
==
w.
[(z,i)+Q(RP
,R»)
If
A
is
the
number determined
by
Proposition
3.1
of
Chap.
Ill,
we
may
choose
{J> 1
so
large that
(2.8
fA)
>
1.
Therefore
the
cylinder
[(x,
t) + Q
(aoRP,
R)]
,
:0
==
(~r-2
=
[2.8(1
~TO)-.8r-2
> 1
is
contained in
[(x,
t) + Q
(RP,
R)],
and
osc
v <
w.
[(z,i)+Q(ooRP,R») -
It
follows that
[(x,
t) + Q
(aoRP,
R)]
can
be
taken
as
the starting
box
in
Lemma
3.1
of Chap.
III.
We
conclude that there exist constants
"(
> 1
and
0:,
Co E (0,1)
such that for
all
r E
(0,
R].
We
let r = u R and then choose u
so
small that
for
all
{Ix
- x I < u
R},
(4.1)
v(x,
t)
~
tJ
(x,
t) -
2.8+1"((1
- T
o
)-.8
u
Ot
=
(1
-
2.8+1"(uOt)
(1
- T
o
)-.8
1
=
"2(1
- T
o
)-.8.
The
various constants appearing
in
Proposition
3.1
and
Lemma
3.1
of
Chap.
III,
in
our context, depend only
upon
N and p and
are
indePendent of
V,(l)
therefore the
number
u can
be
determined a priori only
in
terms of N, p
and
(J.
We
summarise:
(1) See §3-(I) of Chap. III.
