Davidson K.R., Donsig A.P. Real Analysis with Real Applications
Подождите немного. Документ загружается.

13.4 Convergence in the Open Disk 433
Now we extend this argument to apply to the various partial derivatives of u.
This procedure justifies term-by-term differentiation under appropriate condi-
tions on the convergence.
13.4.2. LEMMA. Suppose that u
n
(x, y) are C
1
functions on an open set R for
n ≥ 0 such that
∞
P
n=0
u
n
(x, y) converges uniformly to u(x, y) and
∞
P
n=0
∂
∂x
u
n
(x, y)
converges uniformly to v(x, y). Then
∂
∂x
u(x, y) = v(x, y).
PROOF. It is enough to verify the theorem on a small square about an arbitrary
point (x
0
, y
0
) in R. By limiting ourselves to a square, the whole line segment from
(x
0
, y) to (x, y) will lie in R. We define functions on this small square by
w
n
(x, y) =
n
X
k=0
u
k
(x, y) =
n
X
k=0
u
k
(x
0
, y) +
Z
x
x
0
∂
∂x
n
X
k=0
u
k
(t, y) dt
and
w(x, y) = u(x
0
, y) +
Z
x
x
0
v(t, y) dt.
Then since the integrands
∂
∂x
n
P
k=0
u
n
(t, y) converge uniformly to v(t, y), Corol-
lary 8.3.2 shows that w
n
(x, y) converges uniformly to w(x, y). But this limit is
u(x, y). Therefore, by the Fundamental Theorem of Calculus,
∂
∂x
u(x, y) =
∂
∂x
w(x, y)
=
∂
∂x
³
u(x
0
, y) +
Z
x
x
0
v(t, y) dt
´
(x, y) = v(x, y).
¥
We may apply this to our function u(r, θ).
13.4.3. THEOREM. Let f be an absolutely integrable function with associated
function u(r, θ) = A
0
+
P
∞
n=1
A
n
r
n
cosnθ + B
n
r
n
sinnθ. Then u satisfies the
heat equation ∆u(r, θ) = 0 in the open disk D.
PROOF. Let u
n
(r, θ) = A
n
r
n
cosnθ + B
n
r
n
sinnθ. Then
∂
∂r
u
n
(r, θ) = nA
n
r
n−1
cosnθ + nB
n
r
n−1
sinnθ
∂
2
∂r
2
u
n
(r, θ) = n(n − 1)A
n
r
n−2
cosnθ + n(n − 1)B
n
r
n−2
sinnθ
∂
∂θ
u
n
(r, θ) = −nA
n
r
n
sinnθ + nB
n
r
n
cosnθ
∂
2
∂θ
2
u
n
(r, θ) = −n
2
A
n
r
n
cosnθ − n
2
B
n
r
n
sinnθ.
We will apply the M-test to the series
∞
P
n=0
u
n
(r, θ) and to each series of partial
derivatives. Indeed, on the disk
D
R
for 0 ≤ R < 1, each of the preceding terms is

434 Fourier Series and Physics
uniformly bounded by 4kfk
1
n
2
R
n−2
. Apply the Ratio Test (Exercise 3.2.I) to the
series
∞
P
n=0
4kfk
1
n
2
R
n−2
:
lim
n→∞
4kfk
1
(n + 1)
2
R
n−1
4kfk
1
n
2
R
n−2
= R < 1.
Thus this series converges; and therefore it follows from the Weierstrass M-test
that each series of partial derivatives converges uniformly on
D
R
.
Therefore, by Lemma 13.4.2, the partial derivative of the sum equals the sum
of the partial derivatives. This means that
∂
∂r
u(r, θ) =
∞
X
n=0
∂
∂r
u
n
(r, θ)
∂
2
∂r
2
u(r, θ) =
∞
X
n=0
∂
2
∂r
2
u
n
(r, θ)
∂
∂θ
u(r, θ) =
∞
X
n=0
∂
∂θ
u
n
(r, θ)
∂
2
∂θ
2
u(r, θ) =
∞
X
n=0
∂
2
∂θ
2
u
n
(r, θ).
This convergence is uniform on every disk
D
R
for R < 1.
Using ∆u = u
rr
+
1
r
u
r
+
1
r
2
u
θθ
, we deduce from Lemma 13.4.2 that
∆u(r, θ) =
∞
X
n=0
∆u
n
(r, θ)
and that this convergence is uniform on any disk
D
R
. However, we constructed the
functions r
n
cosnθ and r
n
sinnθ as solutions of ∆u = 0. Thus the right-hand side
of this equation is zero. Hence ∆u(r, θ) = 0 everywhere in the open disk D. ¥
13.4.4. DEFINITION. A function u such that ∆u = 0 is called a harmonic
function. The function u(r, θ) determined by the Fourier series of a 2π-periodic f
is called the harmonic extension of f.
Exercises for Section 13.4
A. Find the Fourier series of f (θ) = θ
2
for −π ≤ θ ≤ π.
(a) Show that the series for u(r, θ) converges uniformly on the closed disk D.
(b) Show that the series for u
θθ
does not converge uniformly on D.
B. Let A
n
and B
n
be the Fourier coefficients of a continuous function f (θ).
(a) Show that v
t
(r, θ) = u(rt, θ) = A
0
+
P
∞
n=1
A
n
(rt)
n
cosnθ + B
n
(rt)
n
sinnθ
converges uniformly on the closed disk D for 0 < t < 1.
(b) Show that v
t
converges uniformly to u on D as t → 1
−
if and only if u extends to
a continuous function on D.

13.5 The Poisson Formula 435
C. (a) Suppose that a 2π-periodic function f is C
1
(i.e., f
0
is continuous), then the Fourier
coefficients of f satisfy |A
n
| + |B
n
| ≤ Cn
−1
for some constant C.
HINT: Integrate by parts and compare with Lemma 13.3.1.
(b) Show by induction that if f is C
k
, then |A
n
|+ |B
n
| ≤ Cn
−k
for some constant C.
D. Suppose f ∼ A
0
+
P
n≥1
A
n
cosnθ + B
n
sinnθ is a 2π-periodic function and that
P
n≥1
|A
n
| + |B
n
| < ∞. Prove that the Fourier series converges uniformly to f.
E. Suppose f ∼ A
0
+
P
n≥1
A
n
cosnθ + B
n
sinnθ is a 2π-periodic function and that
P
n≥1
n|A
n
| + n|B
n
| < ∞. By modifying the proof of Lemma 13.4.2, establish that
P
n≥1
−nA
n
sinnθ + nB
n
cosnθ converges uniformly to f
0
.
F. The Fourier coefficients of a 2π-periodic function f satisfy |A
n
| + |B
n
| ≤ Cn
−k
for
an integer k ≥ 2 and some constant C. Show that f is in the class C
k−2
.
HINT: Use term-by-term differentiation and the M-test.
G. Show that u(r, θ) has partial derivatives
∂
j+k
∂r
j
∂θ
k
u of all orders. Hence show that u is
C
∞
.
HINT: First verify this for the functions u
n
(r, θ). Then show that the sequence of
partial derivatives converges uniformly on each D
R
.
H. Give necessary and sufficient conditions for A
0
+
P
∞
n=1
A
n
cosnθ + B
n
sinnθ to be
the Fourier series of a C
∞
function.
HINT: Combine Exercises C and E.
13.5. The Poisson Formula
The next step is to find a formula for u(r, θ) in terms of the boundary function
f(θ). The basic idea is to substitute the formula for each Fourier coefficient and in-
terchange the order of the summation and integration. This again requires uniform
convergence.
Compute u(r, θ) for a bounded integrable function f:
u(r, θ) = A
0
+
∞
X
n=1
A
n
r
n
cosnθ + B
n
r
n
sinnθ
=
1
2π
Z
π
−π
f(t) dt +
1
π
∞
X
n=1
Z
π
−π
f(t) cosnt dt r
n
cosnθ+
Z
π
−π
f(t) sinnt dt r
n
sinnθ
=
1
2π
Z
π
−π
f(t) dt +
∞
X
n=1
1
π
Z
π
−π
f(t)r
n
¡
cosnt cosnθ + sin nt sinnθ
¢
dt
=
1
2π
Z
π
−π
f(t) dt +
∞
X
n=1
1
π
Z
π
−π
f(t)r
n
cosn(θ − t) dt.

436 Fourier Series and Physics
Yet again, we apply the M-test. Since
kf(t)r
n
cosn(θ − t)k ≤ kfk
∞
R
n
for 0 ≤ r ≤ R,
and
∞
P
n=0
kfk
∞
R
n
= kfk
∞
/(1 − R) < ∞, the series converges uniformly on
D
R
.
Therefore, by Theorem 8.3.1, we can interchange the order of the summation and
the integral
u(r, θ) =
Z
π
−π
f(t)
1
2π
³
1 + 2
∞
X
n=1
r
n
cosn(θ − t)
´
dt
=
Z
π
−π
f(t)P (r, θ − t) dt =
Z
π
−π
f(θ − u)P (r, u) du.
We have introduced the function
P (r, θ) =
1
2π
³
1 + 2
∞
X
n=1
r
n
cosnθ
´
for 0 ≤ r < 1, θ ∈ R.
Notice that the last step is a change of variables in which we make use of the fact
that both f and P are 2π-periodic in θ.
The function P (r, θ) is known as the Poisson kernel. The purpose of this
section is to develop its basic properties.
We will use the fact that exponentiation and trigonometric functions are related
using complex variables. (See Appendix 13.10.) In particular, e
it
= cost + i sint
and consequently
cosnt =
e
int
+ e
−int
2
and sinnt =
e
int
− e
−int
2i
.
13.5.1. THE POISSON FORMULA.
Let f be a bounded integrable function on [−π, π]. Then for 0 ≤ r < 1 and
−π ≤ θ ≤ π, the harmonic extension of f is given by
u(r, θ) =
Z
π
−π
f(θ − t)P (r, t) dt,
where P (r, t) =
1
2π
1 − r
2
1 − 2r cost + r
2
.
PROOF. From the discussion preceding the theorem, it only remains to evaluate the
series for P (r, t). Changing the cosines to complex exponentials allows us to sum
a geometric series.
2πP (r, t) = 1 + 2
∞
X
n=1
r
n
cosnt = 1 +
∞
X
n=1
r
n
(e
int
+ e
−int
)
= 1 +
∞
X
n=1
(re
it
)
n
+
∞
X
n=1
(re
−it
)
n

13.5 The Poisson Formula 437
= 1 +
re
it
1 − re
it
+
re
−it
1 − re
−it
=
(1 − re
it
− re
−it
+ r
2
) + (re
it
− r
2
) + (re
−it
− r
2
)
(1 − re
it
)(1 − re
−it
)
=
1 − r
2
1 − r(e
it
+ e
−it
) + r
2
=
1 − r
2
1 − 2r cost + r
2
¥
The Poisson kernel has a number of very nice properties. The most important
is that P (r, t) is positive and integrates to 1. Hence u(r, θ) is a weighted average
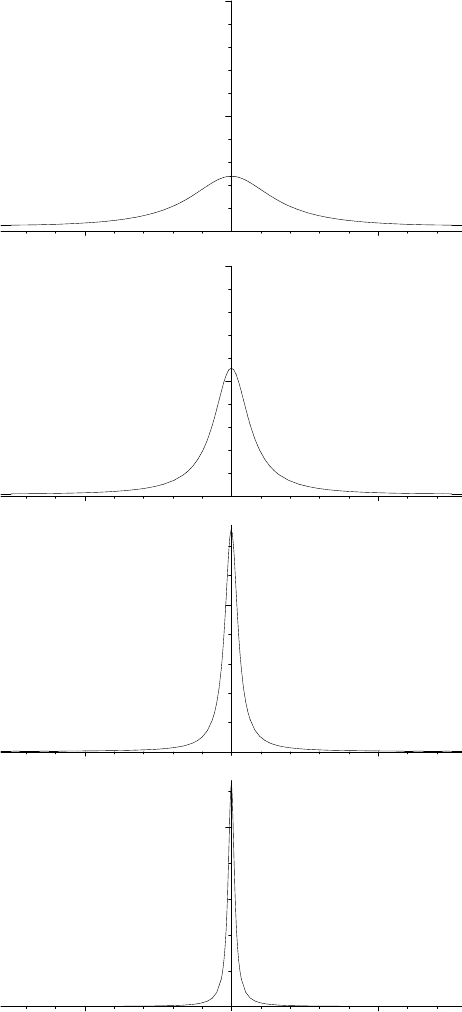
of the values f(θ − t). The function P (r, t) peaks dramatically at t = 0 when r is
close to 1, and thus eventually u(r, θ) depends mostly on the values of f(u) for u
close to θ. See Figure 13.2.
13.5.2. PROPERTIES OF THE POISSON KERNEL.
For 0 ≤ r < 1 and −π ≤ t ≤ π,
(1) P (r, t) > 0.
(2) P (r, −t) = P (r, t).
(3)
Z
π
−π
P (r, t) dt = 1.
(4) P (r, t) is decreasing in t on [0, π] for fixed r.
(5) For any δ > 0, lim
r→1
−
max
δ≤|t|≤π
P (r, t) = 0.
PROOF. Statements (1) and (2) are routine. For (3), take f = 1. The Fourier series
of f is A
0
= 1 and A
n
= B
n
= 0 for n ≥ 1. Hence u(r, θ) = 1. Plugging this into
the Poisson formula, we obtain
1 = u(r, θ) =
Z
π
−π
P (r, t) dt.
For fixed r, the numerator 1 − r
2
of P (r, t) is constant while the denominator
1 − 2r cost + r
2
is monotone increasing on [0, π]; whence P (r, t) is monotone
decreasing in t. This verifies (4). Finally, from (2) and (4), we see that
lim
r→1
−
max
δ≤|t|≤π
P (r, t) = lim
r→1
−
P (r, δ) = lim
r→1
−
1 − r
2
1 − 2r cosδ + r
2
=
0
2(1 − cos δ)
= 0.
¥
In the next chapter, we will develop two other integral kernels, the Dirichlet
kernel and the Fej
´
er kernel. The reader may want to compare their properties and
compare Figures 13.2, 14.2, and 14.4.
438 Fourier Series and Physics
0
1
2
–2 2
0
1
2
–2 2
2
–2 2
5
–2 2
FIGURE 13.2. Graphs of P (r, t) for r = 1/2, 3/4, 9/10, and 19/20.

13.6 Poisson’s Theorem 439
Exercises for Section 13.5
A. Compute the Fourier series of f (θ) = P (s, θ) for 0 ≤ s < 1.
B. Find an explicit value of r < 1 for which
Z
.01
−.01
P (r, t) dt > 0.999.
C. Prove that
1 − r
1 + r
≤ 2πP (r, θ) ≤
1 + r
1 − r
.
D. Let f be a positive continuous 2π-periodic function with harmonic extension u(r, θ).
(a) Prove that u(r, θ) ≥ 0.
(b) Prove Harnack’s inequality:
1 − r
1 + r
u(0, 0) ≤ u(r, θ) ≤
1 + r
1 − r
u(0, 0).
HINT: Use the previous exercise.
E. Suppose that f is a 2π-periodic continuous function such that L ≤ f (θ) ≤ M for
−π ≤ θ ≤ π. Let u(r, θ) be the Poisson extension of f. Show that L ≤ u(r, θ) ≤ M
for 0 ≤ r ≤ 1 and −π ≤ θ ≤ π.
F. Show that if f(θ) is absolutely integrable on [−π, π] and g
n
(θ) are continuous func-
tions that converge uniformly to g(θ) on [−π, π], then
lim
n→∞
Z
π
−π
f(θ)g
n
(θ) dθ =
Z
π
−π
f(θ)g(θ) dθ.
HINT: Look at the proof of Theorem 8.3.1.
G. Use the previous exercise to show that the Poisson formula is valid for absolutely
integrable functions on [−π, π].
H. Prove that
Z
π
−π
P (r, θ − t)P (s, t) dt = P (rs, θ).
HINT: Use the series expansion of the Poisson kernel.
I. Let f(θ) be a continuous 2π-periodic function. Let u(r, θ) be the harmonic extension
of f. Let 0 < s < 1, and define g(θ) = u(s, θ). Prove that the harmonic extension of
g is u(rs, θ).
HINT: Use the Poisson formula twice to obtain theharmonic extension of g as a double
integral, and interchange the order of integration.
13.6. Poisson’s Theorem
Using the properties of the Poisson kernel, it is now possible to show that
u(r, θ) approaches f(θ) uniformly as r tends to 1. This means that our proposed
solution to the heat problem is continuous on the closed disk and has the desired
boundary values. This puts us very close to solving the steady-state heat problem.
It also provides a stronger reason for calling u(r, θ) an extension of f.

440 Fourier Series and Physics
13.6.1. POISSON’S THEOREM.
Let f be a continuous 2π-periodic function on [−π, π] with harmonic extension
u(r, θ). Then f
r
(θ) := u(r, θ) converges uniformly to f on [−π, π] as r → 1
−
.
Consequently, the function u(r, θ) may be defined on the boundary of the closed
disk by u(1, θ) = f(θ) to obtain a continuous function on D that is harmonic on D
and agrees with f on the boundary.
PROOF. Let ε > 0 be given. We will find an r
0
< 1 so that
|f(θ) − f
r
(θ)| ≤ ε for all r
0
≤ r < 1, −π ≤ θ ≤ π,
which will establish uniform convergence.
Let M = kf k
∞
and set ε
0
= (4πM +1)
−1
ε. By Theorem 5.5.9, f is uniformly
continuous. Therefore, there is a δ > 0 such that
|f(θ) − f(t)| ≤ ε
0
for all |θ − t| ≤ δ.
By property (5), there is an R < 1 such that
max
δ≤|t|≤π
P (r, t) ≤ ε
0
for all R ≤ r < 1.
Now, using the Poisson formula, compute
f(θ) − f
r
(θ) = f (θ)
Z
π
−π
P (r, t) dt −
Z
π
−π
f(θ − t)P (r, t) dt
=
Z
π
−π
¡
f(θ) − f(θ − t)
¢
P (r, t) dt.
For R ≤ r < 1, split this integral into two pieces to estimate |f (θ) − f
r
(θ)|
≤
Z
δ
−δ
|f(θ) − f(θ − t)|P (r, t) dt +
Z
−δ
−π
+
Z
π
δ
|f(θ) − f(θ − t)|P (r, t) dt
≤
Z
δ
−δ
ε
0
P (r, t) dt +
Z
−δ
−π
+
Z
π
δ
2Mε
0
dt
≤ ε
0
+ 2π(2Mε
0
) = (4πM + 1)ε
0
= ε.
This establishes that kf − f
r
k
∞
< ε for R ≤ r < 1. Hence f
r
(θ) converges
uniformly to f(θ) on [−π, π].
Extend the definition of u(r, θ) to the boundary of the closed disk by setting
u(1, θ) = f(θ). The previous paragraphs show that this function is continuous on
the closed disk
D. By Theorem 13.4.3, u is harmonic on the interior of the disk. ¥
This results yields several important consequences quite easily. The first is
the existence of a solution to the heat problem. The question of uniqueness of the
solution will be handled in the next section.
13.6.2. COROLLARY. Let f be a continuous 2π-periodic function. Then the
steady-state heat equation ∆u = 0 and u(1, θ) = f(θ) has a continuous solution.

13.6 Poisson’s Theorem 441
PROOF. By Theorem 13.4.3, the function u(r, θ) is differentiable on the open disk
D and satisfies ∆u = 0. By Poisson’s Theorem, u extends to be continuous on the
closed unit disk D and attains the boundary values u(1, θ) = f(θ). ¥
This next corollary shows that the Fourier series of a continuous function de-
termines the function uniquely. Continuity is essential for this result. For example,
the function that is zero except for a discontinuous point f(0) = 1 will have the
zero Fourier series but is not the zero function.
13.6.3. COROLLARY. If two continuous 2π-periodic functions on R have equal
Fourier series, then they are equal functions.
PROOF. Suppose that f and g have the same Fourier series. Since the harmonic
extension u(r, θ) is defined only in terms of the Fourier coefficients, this is the same
function for both f and g. Now, by Poisson’s Theorem,
f(θ) = lim
r→1
−
u(r, θ) = g(θ) for − π ≤ θ ≤ π.
Thus f = g. ¥
The final application concerns the case in which the Fourier series itself con-
verges uniformly. This is not always the case, and the delicate question of the
convergence of the Fourier series will be dealt with later.
13.6.4. COROLLARY. Suppose that the Fourier series
f ∼ A
0
+
∞
X
n=1
A
n
cosnθ + B
n
sinnθ
of a continuous function f converges uniformly. Then the series in fact converges
uniformly to f.
PROOF. Let the uniform limit be g. By Theorem 8.2.1, g is continuous; and it is
2π-periodic as it is the limit of 2π-periodic functions. Compute the Fourier series
of g using Theorem 8.3.1:
1
π
Z
π
−π
g(t) sinnt dt = lim
N→∞
1
π
Z
π
−π
³
A
0
+
N
X
k=1
A
k
coskt + B
k
sinkt
´
sinnt dt
= lim
N→∞
B
n
= B
n
.
The other coefficients are computed in the same way. It follows that f and g have
the same Fourier series. Thus by the previous corollary, they are equal. That is, the
Fourier series converges uniformly to f. ¥

442 Fourier Series and Physics
13.6.5. EXAMPLE. Recall Example 13.3.2. It was shown that
|θ| ∼
π
2
−
4
π
∞
X
k=0
cos((2k + 1)θ)
(2k + 1)
2
.
Since
π
2
+
4
π
∞
P
k=0
1
(2k+1)
2
converges (by the integral test or by comparison with
1
n
2
),
it follows from the Weierstrass M -test (8.4.7) that this series converges uniformly.
By Corollary 13.6.4, it follows that the Fourier series converges uniformly to |θ|.
Let us evaluate this at θ = 0.
0 =
π
2
−
4
π
∞
X
k=0
1
(2k + 1)
2
Therefore,
∞
X
k=0
1
(2k + 1)
2
=
π
2
8
.
A little manipulation yields that
∞
X
k=1
1
k
2
=
∞
X
k=0
1
(2k + 1)
2
+
∞
X
k=1
1
(2k)
2
=
π
2
8
+
1
4
∞
X
k=1
1
k
2
.
Solving, we obtain a famous identity due to Euler (by different methods):
∞
X
k=1
1
k
2
=
π
2
6
.
We conclude this section with an important application of Poisson’s Theorem
to approximation by trigonometric polynomials. Another proof of this fact will be
given in Theorem 14.6.4.
13.6.6. COROLLARY. Every continuous 2π-periodic function is the uniform
limit of a sequence of trigonometric polynomials.
PROOF. Let f be a continuous 2π-periodic function. Given n ∈ N, Poisson’s
Theorem (Theorem 13.6.1) shows that there is an r < 1 such that kf −f
r
k
∞
<
1
2n
.
Let M = kfk
1
and choose K so large that
∞
P
k=K+1
4Mr
k
<
1
2n
. Then
t
n
(θ) = A
0
+
K
X
k=1
A
k
r
k
coskθ + B
k
r
k
sinkθ
