Das Sh.P. Statistical Physics of Liquids at Freezing and Beyond
Подождите немного. Документ загружается.

A8.1 Calculation of the spring constant 431
+
˜
φ
α
(|x
− R
1
|)
N
i=2
φ
α
(|x − R
i
|) +
N
i=2
N
j=2
φ
α
(|x − R
i
|)φ
α
(|x − R
j
|)
⎤
⎦
≡
4
i−1
I
i
. (A8.1.3)
With an interchange of the integrated variables x and x
, it follows that the second and
third integrals, denoted by I
2
and I
3
, respectively, are the same. Using (A8.1.2),thesum
of these two integrals is written as
I
2
+ I
3
=−
dx
dx
c(|x −x
|)
˜
φ
α
(|x − R
1
|)
!
n(x
) − φ
α
(|x
− R
1
|)
"
, (A8.1.4)
and
I
4
=−
1
2
dx
dx
[n(x) − φ
α
(|x − R
1
|)]c(|x − x
|)
×
!
n(x
) − φ
α
(|x
− R
1
|)
"
,
=−
dx
dx
c(|x −x
|)
×
!
n(x)n(x
) − φ
α
(|x − R
1
|)n(x
)
− φ
α
(|x
− R
1
|)n(x) + φ
α
(|x − R
1
|)φ
α
(|x
− R
1
|)
"
= F
in
+
dx
dx
c(|x −x
|)
×
φ
α
(|x − R
1
|)n(x
) −
1
2
φ
α
(|x − R
1
|)φ
α
(|x
− R
1
|)
. (A8.1.5)
On adding the four integrals, we obtain the result
βF
in
=
dx
dx
c(|x − x
|)
×
#
n(x
)
φ
α
(|x − R
1
|) −
˜
φ
α
(|x − R
1
|)
+
1
2
˜
φ
α
(|x − R
1
|)φ
α
(|x
− R
1
|) +
˜
φ
α
(|x
− R
1
|)φ
α
(|x − R
1
|)
−
˜
φ
α
(|x − R
1
|)
˜
φ
α
(|x
− R
1
|) − φ
α
(|x − R
1
|)φ
α
(|x
− R
1
|)
$
=
dx
dx
c(|x − x
|)
×
#
n(x
)
φ
α
(|x − R
1
|) −
˜
φ
α
(|x − R
1
|)
432 Appendix to Chapter 8
+
1
2
˜
φ
α
(|x − R
1
|) − φ
α
(|x − R
1
|)
×
˜
φ
α
(|x
− R
1
|) − φ
α
(|x
− R
1
|)
$
. (A8.1.6)
We now expand the difference of the Gaussian functions as a Taylor-series expansion in
δR
1
in the form
˜
φ
α
(|x − R
1
|) − φ
α
(|x − R
1
|) =∇
x
φ
α
(|x − R
1
|) · δR
1
+
1
2
∇
x
∇
x
φ
α
(|x − R
1
|) · δR
1
δR
1
+···. (A8.1.7)
On substituting this expansion into eqn. (A8.1.6) we obtain for the variation in the free
energy
βF
in
=
dx
dx
c(|x −x
|)
×
3
n(x
)∇
x
φ
α
(|x − R
1
|) · δR
1
+
1
2
n(x
)∇
x
∇
x
φ
α
(|x − R
1
|) · δR
1
δR
1
+
1
2
∇
x
φ
α
(|x − R
1
|)∇
x
φ
α
(|x
− R
1
|) · δR
1
δR
1
+···
4
. (A8.1.8)
The first term on the RHS goes to zero since {R
i
}are the equilibrium (metastable) positions
and represent a minimum of the thermodynamic function. The i = 1terminthesummation
expression for n(x
) appearing in the second term on the RHS of eqn. (A8.1.8) actually
cancels out with the third term. The second and third terms combine to give the following
result for the free energy as a quadratic functional of the displacement δR
1
:
βF
in
=
1
2
dx
dx
c(|x −x
|)
×
N
i=2
φ
α
(|x
− R
i
|)∇
x
∇
x
φ
α
(|x − R
1
|)
· δR
1
δR
1
+···
≡
1
2
κ
s
ij
δ R
1i
δ R
1 j
, (A8.1.9)
where κ
s
ij
is the tensor of spring constants. For an isotropic system we denote this as κ
s
,
which is a scalar.
A8.2 Field-theoretic treatment of the DDFT model
The analysis presented in Section 8.1 with the dynamic density-functional model provides
the basic nature of the slow dynamics in the nonlinear case; the FDT relation (8.1.137)
A8.2 Field-theoretic treatment of the DDFT model 433
(Kawasaki and Miyazima, 1997) used is ad hoc. Let us consider the associated renormal-
ization of the field-theoretic model established in subsequent works (Andreanov et al.,
2006; Kim and Kawasaki, 2008).
The time-reversal symmetry
We first construct, following the standard methods introduced in Chapter 7, the action
functional of the MSR field theory for the equation of motion (8.1.144). Using the steps
followed in Section 7.1, the average of a dynamic variable f [ρ] is defined as
f [ρ]
=
Dρ
f [ρ
]δ(ρ − ρ
)
=
Dρ f [ρ
]J [ρ]
-
δ
∂ρ
∂t
−∇
i
D(ρ
)∇
i
δF
δρ
− η
.
η
. (A8.2.1)
In the above equation the Jacobian of the transformation is treated as a constant by adopt-
ing the Itô (Oksendal, 1992) interpretation of the multiplicative noise. The average of the
functional f [ρ] is then given by
f [ρ]
=
Dρ
D ˆρ f [ρ]exp
3
i
dx
dt ˆρ
∂ρ
∂t
−∇
i
D(ρ)∇
i
δF
δρ
4
×
-
exp
−i
dr
dt ˆρ(x, t)η(x, t)
.
η
≡
Dρ
D ˆρ f [ρ]exp
−A[ρ, ˆρ]
. (A8.2.2)
By averaging with respect to the Gaussian noise η, the MSR action A is obtained as
A[ρ, ˆρ]=
dx
dt
3
β
−1
ρ(∇ˆρ)
2
−i ˆρ
∂ρ
∂t
−∇·
D(ρ)∇
δF
δρ
4
=
dx
dt
3
−β
−1
ˆρ ∇ · (D(ρ)∇ˆρ) − i ˆρ
∂ρ
∂t
−∇·
D(ρ)∇
δF
δρ
4
=
dx
dt
3
−i ˆρ
∂ρ
∂t
− β
−1
ˆρ ∇ ·
D(ρ)∇
ˆρ −iβ
δF
δρ
4
. (A8.2.3)
The expression (A8.2.3) for the action A is written as a sum of two parts:
A[ρ, ˆρ]=−i
dx
dt ˆρ(x, t)
∂ρ(x, t)
∂t
+ β
−1
dx
dt ˆρ(x, t)∇ ·
!
D(ρ)∇ˆρ(x, −t)
"
. (A8.2.4)
The above MSR action functional A[ρ, ˆρ] is invariant under the transformation
ρ(x, −t) = ρ(x, t),
ˆρ(x, −t) =−ˆρ(x, t) +iβ
δF
δρ (x, t)
. (A8.2.5)
434 Appendix to Chapter 8
The second integral on the RHS of (A8.2.4) clearly remains invariant under the transforma-
tion (A8.2.5), as can easily be seen by partially integrating twice on the spatial coordinates.
The first term under the same transformation changes to
−i
dx
dt ˆρ(x, t)
∂ρ(x, t)
∂t
→i
dx
dt ˆρ(x, −t)
∂ρ(x, t)
∂t
=−i
dx
dt
ˆρ(x, t) − iβ
δF
δρ (x, t)
∂ρ(x, t)
∂t
=−i
dx
dt
ˆρ(x, t)
∂ρ(x, t)
∂t
+ β
δF
δρ (x, t)
∂ρ(x, t)
∂t
=−i
dx
dt ˆρ(x, t)
∂ρ(x, t)
∂t
+ β
dt
∂ F
∂t
=−i
dx
dt ˆρ(x, t)
∂ρ(x, t)
∂t
. (A8.2.6)
The MSR action (A8.2.3) therefore remains invariant under the transformation (A8.2.5) up
to a constant. Note that we have ignored the last term on the RHS of (A8.2.6) since it can
be expressed with boundary terms and hence is treated as a constant. This is similar to the
case of the time-reversal symmetry transformation in the fluctuating-hydrodynamics case
discussed in Section A7.3.
The linear response function
Let us consider the response to an external field that couples to density in the free-energy
functional,
F
ext
=−
dx
dt δρ (x, t)h
e
(x, t). (A8.2.7)
The corresponding term in the equation of motion (8.1.144) has the form ∇
i
D(ρ)∇
i
h
e
(x, t)
and hence produces a contribution to the MSR action
A = i
dx
dt ˆρ(x, t)∇{D(ρ)∇h
e
(x, t)}. (A8.2.8)
Upon integrating partially twice with respect to spatial coordinates, A is obtained in a
form proportional to the external field,
A = i
dx
dt ∇ · {D(ρ)∇ˆρ(x, t)}h
e
(x, t). (A8.2.9)
The corresponding induced change linear in the external field is given by
ρ (x, t)
= i
dx
dt
ρ(x, t)∇
· {D(ρ)∇
ˆρ(x
, t)}h
e
(x
, t)}
≡
dx
dt
R(x, t;x
, t
)h
e
(x
, t
). (A8.2.10)
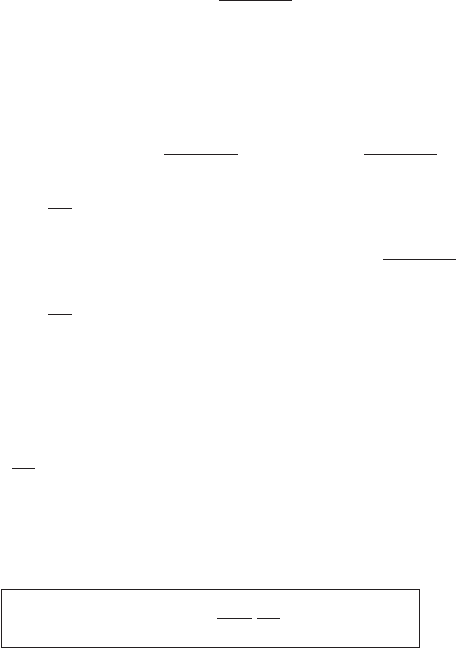
A8.2 Field-theoretic treatment of the DDFT model 435
The last equality follows from the definition of the linear response function R(x, t;x
, t
).
∇
represents the derivative operator with respect to the components of x
. Thus we obtain
R(x, t;x
, t
) = iρ(x, t)∇
· {D(ρ)∇
ˆρ(x
, t
)}. (A8.2.11)
Note that R is the linear response function to an external field h
e
directly coupling to the
density ρ. As discussed earlier (see Section 7.3.1) since the dissipative coefficient D(ρ)
is dependent on density here (multiplicative noise), the physical response function is not a
simple two-point correlation function between a hatted and an unhatted field of the MSR
theory.
The fluctuation–dissipation theorem
It is straightforward to show that R is related to a correlation function by the standard FDT
of equilibrium statistical mechanics discussed in Chapter 1, eqn. (1.3.72). We begin with
the identity
-
ρ(x, t)
δA
δ ˆρ(x
, t
)
.
= 0. (A8.2.12)
Using the expression given in eqn. (A8.2.3) for the MSR action, we obtain from (A8.2.12)
0 =
-
ρ(x, t)
2β
−1
∇ · {D(ρ)∇ˆρ(x
, t
)}
+ i
∂ρ(x
, t
)
∂t
−∇
· D(ρ)∇
δF
δρ (x
, t
)
.
= i
∂
∂t
1
ρ(x, t)ρ(x
, t
)
2
+ β
−1
ρ(x, t)∇ · {D(ρ)∇ˆρ(x
, t
)}
+ β
−1
-
ρ(x, t)∇
· D(ρ)∇
3
ˆρ(x
, t
) − iβ
δF
δρ (x
, t
)
4.
= i
∂
∂t
G
ρρ
(x, t;x
, t
) + β
−1
ρ(x, t)∇ · {D(ρ)∇ˆρ(x
, t
)}
− β
−1
ρ(x, t)∇
· {D(ρ)∇
ˆρ(x
, −t
)}. (A8.2.13)
In reaching the last equality we have used the transformation (A8.2.5). From the definition
(A8.2.11) of the response function R we obtain
β
∂
∂t
G
ρρ
(x, t;x
, t
) = R(x, t ;x
, t
) − R(x
, t
;x, t). (A8.2.14)
For t > t
, causality requires that the response function R(x
, t
;x, t) = 0, and hence we
obtain the standard FDT of equilibrium statistical mechanics relating the physical response
functions and the correlation function,
R(x, t;x
, t
) = (t − t
)
1
k
B
T
∂
∂t
G
ρρ
(x, t;x
, t
), (A8.2.15)
where (t) represents the Heaviside step function.

436 Appendix to Chapter 8
In Section 7.3.1, in the MSR field theory, we showed that there exists a certain set of
MSR-FDT relations linking the correlation functions between (a) two unhatted fields and
(b) a hatted field and an unhatted field. The latter are termed the MSR response functions
and in a nonlinear theory these are different from the physical response functions. From
the time-reversal symmetry (A8.2.5) we obtain the following MSR-FDT relations:
β
−1
G
ˆρρ
(x, t;x
, t
) = i(t − t
)
-
ρ(x, t)
δF
δρ (x
, t
)
.
.
(A8.2.16)
The time-reversal invariance transformations given by (A8.2.5) are nonlinear since the
functional derivative δF/δρ is nonlinear in ρ.
The ergodic–nonergodic transition
Let us now consider the structure of the renormalized theory due to the nonlinear coupling
of the density fluctuations in the MSR action (A8.2.3) and how it affects the ergodic–
nonergodic (ENE) transition. Linear relations between correlation and response functions
are obtained for the nonlinear model by introducing into the fluctuating-hydrodynamics
description the nonlinear constraints involving the larger set of fields. For the nonlin-
ear fluctuating-hydrodynamics (NFH) model described in Chapter 6 with the set {ρ,g},
development of the appropriate field-theoretic model involves introducing the new field
δF/δg
i
=v
i
. The field v appears in the equations of motion for g. In general, introducing
the variable
θ
i
=
δF
δψ
i
(A8.2.17)
gives rise to a nonlinear constraint in the theory if F is non-Gaussian. For example, in
the NFH model the free energy is expressed as a sum of kinetic (F
K
) and potential (F
U
)
parts. The dependence on the momentum density (g) is in the kinetic part F
K
. This term
is non-Gaussian, as shown in expression (6.2.7) for F
K
. Including v thereby imposes the
nonlinear constraint g =ρv in this model. The appropriate field theory in the case of the
DDFT equation (6.3.14) requires ψ
i
≡ ρ. The contribution from the non-Gaussian part of
the free-energy functional F[ρ] in δF[ρ]/δρ is now treated as a new variable θ (Kim and
Kawasaki, 2008). We express the free energy as a sum of two parts,
F = F
id
[ρ]+F
in
[ρ], (A8.2.18)
where
β F
id
[ρ]=
dx ρ(x)
ln
#
3
0
ρ(x)
$
− 1
, (A8.2.19)
β F
in
[ρ]=
1
2
dx
dx
˜
U (x − x
)δρ(x, t)δρ(x
, t), (A8.2.20)
where
0
is the thermal de Broglie wavelength and δρ denotes the fluctuation of the density
around the density of a uniform liquid state.
˜
U (x) represents an interaction term at the
Gaussian level.
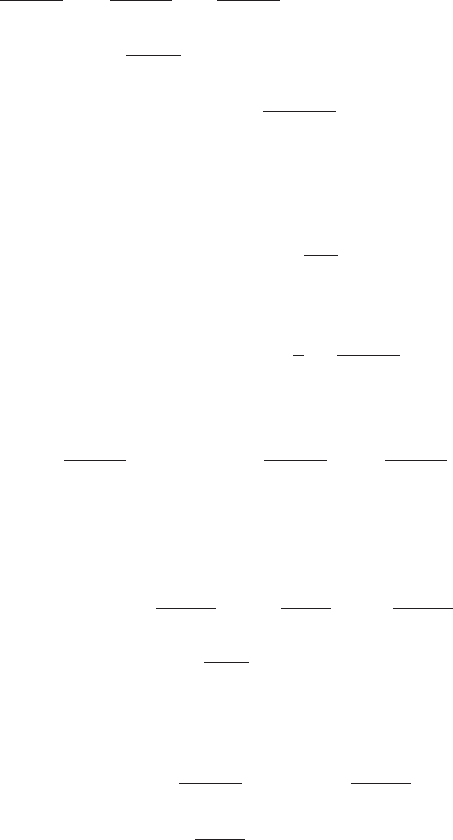
A8.2 Field-theoretic treatment of the DDFT model 437
Then
β
δF[ρ]
δρ (x, t)
= β
δF
id
[ρ]
δρ (x, t)
+ β
δF
in
[ρ]
δρ (x, t)
= ln
ρ(x, t)
ρ
0
+
dx
˜
U (x − x
)δρ(x
, t)
=
dx
˜
U (x − x
) +
δ(x − x
)
ρ
0
δρ (x
, t) + f [δρ (x, t)]
=
dx
B(x − x
)δρ(x
, t) + θ(x, t), (A8.2.21)
where we have defined the kernel B as
B(x) =
˜
U (x) +
δ(x)
ρ
0
. (A8.2.22)
The field θ introduced in eqn. (A8.2.21) is defined in terms of the function f as
θ(x, t) = f (δρ) =−
∞
n=2
1
n
−
δρ (x, t)
ρ
0
n
, (A8.2.23)
or, equivalently,
δρ (x, t)
ρ
0
+ θ(x, t) = ln
δρ (x, t)
ρ
0
≡ β
δF
id
[ρ]
δρ (x, t)
. (A8.2.24)
The field θ introduced above comprises a bigger set of fluctuating variables {ρ,θ} and the
corresponding hatted variables then lead to a set of linear FDTs. Note that the equilibrium
average of the field θ is equal to zero:
θ(x)=β
-
δF
id
[ρ]
δρ (x)
.
= β
-
δF[ρ]
δρ (x)
.
− β
-
δF
in
[ρ]
δρ (x)
.
=−
Dρ
δ
δρ (x)
e
−β F
= 0. (A8.2.25)
Since F
U
is taken to be a quadratic functional of δρ, the functional derivative (δF
in
/δρ) is
linear in δρ and is equal to zero. For the static correlation
S
ρθ
(x − x
) ≡
-
δρ (x)
δF[ρ]
δρ (x, t)
.
= β
-
δρ (x)
δF
id
[ρ]
δρ (x
)
.
= β
-
δρ (x)
δF[ρ]
δρ (x
)
.
−δρ (x)B ⊗ δρ (x
), (A8.2.26)
where B ⊗δρ denotes the convolution on the RHS of eqn. (A8.2.21). On taking the Fourier
transform of the above equation and using the result (A8.2.22) we obtain the result
B
q
S
ρρ
(q) + S
ρθ
(q) = 1, (A8.2.27)
where B
q
=
˜
U (q) + ρ
−1
0
is the Fourier transform of B(x). In the noninteracting case
S
ρθ
=0.

438 Appendix to Chapter 8
The MSR action for the new set of variables {ρ, ˆρ,θ,
ˆ
θ} with the nonlinear constraint
(A8.2.23) is obtained as
A[ρ, ˆρ, θ,
ˆ
θ]=
dx
dt
3
β
−1
ρ(∇ˆρ)
2
−i
ˆ
θ
(
θ − f (δρ)
)
− i ˆρ
∂ρ
∂t
−∇·
(
ρ ∇{B ⊗ δρ + θ }
)
4
= A
g
[ρ, ˆρ, θ,
ˆ
θ]+A
ng
[ρ, ˆρ, θ,
ˆ
θ]. (A8.2.28)
The Gaussian and non-Gaussian parts, denoted by A
g
and A
ng
, respectively, are obtained as
A
g
=
dx
dt
3
β
−1
ρ
0
(∇ˆρ)
2
−i
ˆ
θθ − i ˆρ
∂ρ
∂t
− ρ
0
∇
2
{B ⊗ δρ + θ }
4
, (A8.2.29)
A
ng
=
dx
dt
#
β
−1
δρ(∇ˆρ)
2
+i
ˆ
θ f (δρ) +i ˆρ ∇ · δρ ∇
[
B ⊗ δρ + θ
]
$
. (A8.2.30)
With the extended set of field variables, the set of linear transformations which keeps the
MSR (A8.2.30) action invariant is obtained as
ρ(x, −t) = ρ(x, t), (A8.2.31)
ˆρ(x, −t) =−ˆρ(x, t) +i B ⊗ δρ (x
, t) + i θ(x, t), (A8.2.32)
θ(x, −t) = θ(x, t), (A8.2.33)
ˆ
θ(x, −t) =
ˆ
θ(x, t) + i
∂
∂t
ρ(x, t). (A8.2.34)
Both the Gaussian and the non-Gaussian parts of the action remain separately invariant
under the above set of linear transformations, and the following set of FDTs holds in the
nonlinear theory just as the FDTs (7.3.29) and (7.3.30) hold in the NFH case. We write
them in terms of the spatial Fourier transform as
G
ρ
ˆ
θ
(q, t) = i (t)
∂
∂t
G
ρρ
(q, t), (A8.2.35)
G
ρ ˆρ
(q, t) = i (t)
!
B
q
G
ρρ
(q, t) + G
ρθ
(q, t)
"
, (A8.2.36)
G
θ ˆρ
(q, t) = i (t)
!
B
q
G
θρ
(q, t) + G
θθ
(q, t)
"
, (A8.2.37)
G
θ
ˆ
θ
(q, t) = i (t)
∂
∂t
G
θρ
(q, t). (A8.2.38)
The time-reversal transformations (A8.2.31)–(A8.2.34) are linear with an extended set of
slow modes. However, this inevitably brings in the nonlinear constraint (A8.2.23) linking
θ and δρ.
The dynamics of the various correlation and response functions in the associated field
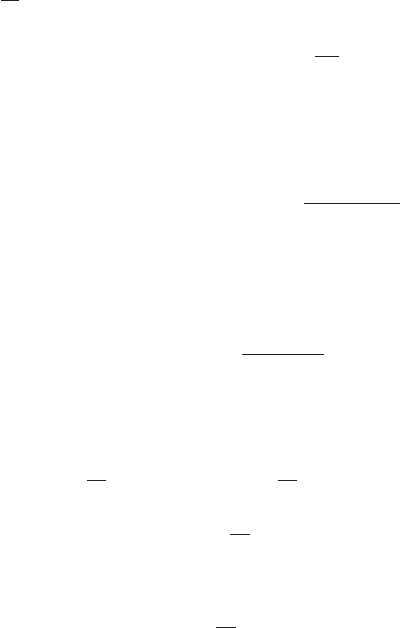
theory is conveniently obtained by the Schwinger–Dyson equation,
¯
2
G
−1
0
(1
¯
2)G(
¯
22) − (1
¯
2)G
¯
22)
= δ(12). (A8.2.39)
A8.2 Field-theoretic treatment of the DDFT model 439
From the large number of equations for the different elements of the correlation function
matrix G, Kim and Kawasaki (2008) showed that the density correlation function G
ρρ
in
the nonlinear theory satisfies the equation
∂
∂t
G
ρρ
(q, t) =−
2
q
G
ρρ
(q, t) −
t
0
dt
˜
ˆρ ˆρ
(q, t − t
)G
ρρ
(q, t
)
−
t
0
dt
ˆρ
ˆ
θ
(q, t − t
)
∂
∂t
G
ρρ
(q, t
), (A8.2.40)
where
2
q
= q
2
/(βmS(q)) is a microscopic frequency of the liquid state. S(q) is the static
structure factor for the liquid, which is related to the interaction term
˜
U (q) through the
Ornstein–Zernike (Ornstein and Zernike, 1914) relation
S
ρρ
(q) ≡ ρ
0
S(q) =
ρ
0
1 + ρ
0
˜
U (q)
, (A8.2.41)
ρ
0
being the equilibrium number density of particles (the mass m is unity in the present
notation). We define the quantity
˜
ˆρ ˆρ
in eqn. (A8.2.40) in terms of the self-energy matrix
element
ˆρ ˆρ
:
˜
ˆρ ˆρ
(q, t) =
1
ρ
0
2
q
S(q)
ˆρ ˆρ
(q, t). (A8.2.42)
In developing the diagrammatic perturbation series for the renormalized theory, a useful
identity for the fields ρ and θ follows from (A8.2.24):
ρ∇
i
ln
ρ
ρ
0
= (ρ
0
+ δρ)∇
i
δρ
ρ
0
+ θ
=∇
i
δρ +
δρ
ρ
0
∇
i
δρ + ρ
0
∇
i
θ + δρ ∇
i
θ. (A8.2.43)
Since the LHS is identically equal to the first term on the RHS, we obtain the identity that
ρ
0
∇
i
θ +
δρ
ρ
0
∇
i
δρ + δρ ∇
i
θ = 0. (A8.2.44)
Note that the first term on the LHS of (A8.2.44) is linear while the other two terms
are nonlinear in the field variables. In the field-theoretic model involving the four fields
{ρ, ˆρ, θ,
ˆ
θ}, as a consequence of (A8.2.44) cancelations occur between the contributions
coming from the Gaussian and non-Gaussian parts of the action (A8.2.28).
Kawasaki rearrangement
An important consequence of the above equation of motion for the density correlation func-
tion is the following: The positive sign of the second term on the RHS of eqn. (A8.2.40)
renders the dynamics unstable if its contribution is large. This equation therefore requires
further tuning in order for it to be physically relevant. This is done through a reorganization
of the kernels, a scheme proposed by Kawasaki (1995, 1997), with the introduction of an
irreducible memory function (Cichocki and Hess, 1987). We will refer to this as Kawasaki

440 Appendix to Chapter 8
rearrangement. Let us first ignore the last term on the RHS of eqn. (A8.2.40) involv-
ing
ˆρ
ˆ
θ
and consider the dynamics of a correlation function C with the memory-function
equation
∂C
∂t
=−τ
−1
0
C(t) − τ
0
t
0
(t − s)C(s)
, (A8.2.45)
where τ
0
> 0 is the relaxation time in the absence of any nonlinearity whose effect is
represented by the kernel . We also ignore the field indices associated with the self-
energies and correlation functions. On taking the Laplace transform of eqn. (A8.2.45) we
obtain
˜
C(z) =
C(0)
z + τ
−1
0
[1 − τ
0
(z)]
, (A8.2.46)
where we have defined the frequency transform of the kernel as
(z) =
dt e
izt
(t). (A8.2.47)
We now replace the memory kernel in the denominator on the RHS of (A8.2.46) with its
irreducible counterpart denoted by
I
such that eqn. (A8.2.46) takes a more physically
meaningful form,
˜
C(z) = C(0)
z +
τ
−1
0
1 +
I
(z)
−1
. (A8.2.48)
The corresponding relation between the kernel and its irreducible counterpart
I
is
given by
1 − τ
0
(z) =
1
1 +
I
(z)
, (A8.2.49)
or, equivalently,
(z) = τ
−1
0
I
(z)
1 +
I
(z)
. (A8.2.50)
For →τ
−1
0
, we obtain
I
→∞. Hence from eqn. (A8.2.46) it follows that
˜
C(z) develops
a1/z pole. In the time space eqn. (A8.2.50) implies the relation
(t) =
I
− τ
0
t
0
dt
(t − t
)
I
(t
). (A8.2.51)
The equation of motion for the correlation function in terms of the irreducible memory
function is obtained as
∂C
∂t
=−τ
−1
0
C(t) − τ
0
t
0
I
(t − s)
∂C(s)
∂s
, (A8.2.52)
which, on Laplace transforming, reduced to eqn. (A8.2.48). Note that eqn. (A8.2.52) fol-
lows if we replace C in the second term on the RHS of eqn. (A8.2.45) in the lowest-order
approximation with −τ
0
∂C/∂t.
