Dake L.P. Fundamentals of reservoir engineering
Подождите немного. Документ загружается.

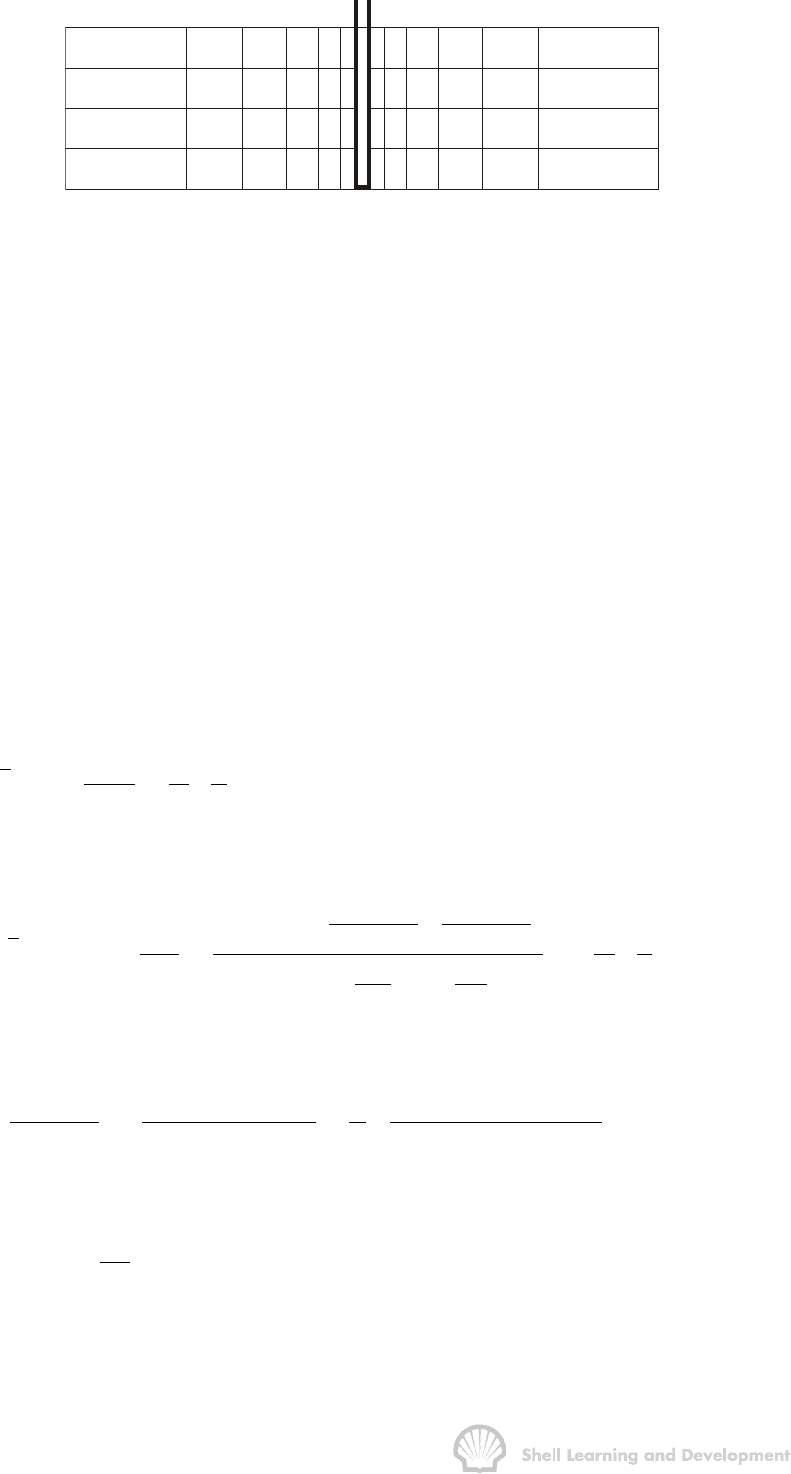
REAL GAS FLOW: GAS WELL TESTING 241
r
w
r
r
e
Fig. 8.1 Radial numerical simulation model for real gas inflow
The flow equations from block to block were solved numerically, using a finite
difference approximation, making due allowance for the variation of µ and Z as
functions of pressure. This is equivalent to solving the non-linear second order
differential equation (5.1). The results may be expected to be in slight error due to the
use of finite difference calculus, but the errors were minimized by making the grid
blocks smaller in the vicinity of the wellbore, where the pressure gradients are largest,
thus providing a higher resolution of solution in this region. With this model it was
hoped that some correcting factor could be found which could be used to match the
approximate analytical results, obtained by making the same assumptions as for a
single phase liquid, with the more exact results from the numerical simulation.
As an example of the approach taken by Russell and Goodrich, consideration will be
given to adapting the semi-steady state inflow equation, developed in chapter 6, sec. 2,
for the flow of oil, to an equivalent form which will be appropriate for the flow of gas.
The equation of interest, expressed in Darcy units, is
e
wf
w
r
q3
pp ln S
2kh r 4
µ
π
æö
−= −+
ç÷
èø
(6.12)
which, when expressed in the field units specified in the previous section, becomes
()
e
wf
w
s.cc / sec r.cc / sec
Q Mscf / d
r
atm 3
Mscf / d s.cc / sec
pp psi ln S
Dcm
psi r 4
2kmD hft
mD ft
µ
π
éùéù
êúêú
æö
éù
ëûëû
−= −+
ç÷
êú
éù éù
ëû
èø
êú êú
ëû ëû
(8.1)
In this conversion the ratio
r.cc / sec reservoir cc / sec 1 1
s.cc / sec s tandard cc / sec E Gas expansion factor
éù
éù
===
êú
êú
ëû
ëû
and in field units
p
E35.37
ZT
=
(1.25)
and
p , the pressure at which E is evaluated, is as yet undefined. The full conversion of
the rate term in equation (8.1 ) can be expressed as
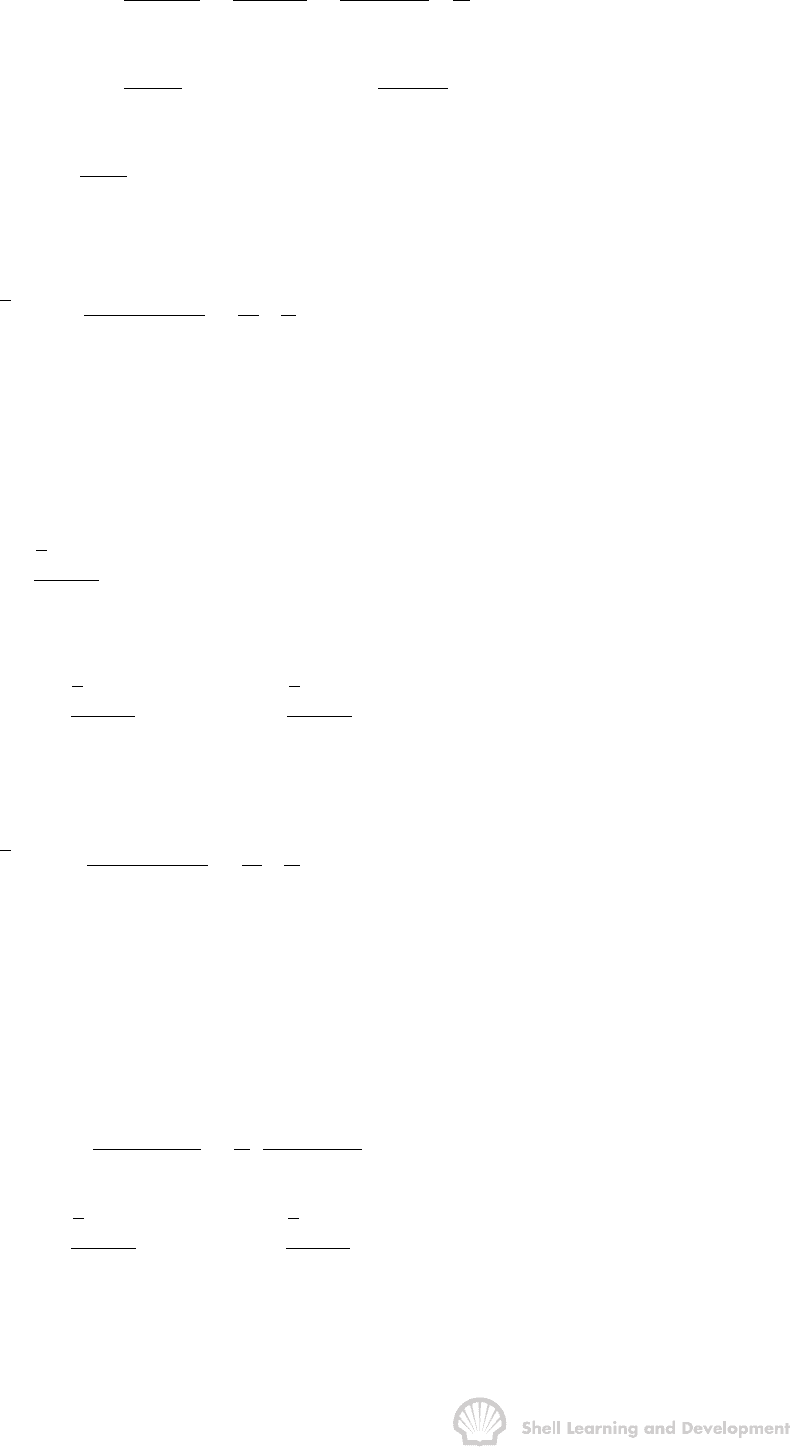
REAL GAS FLOW: GAS WELL TESTING 242
Mstb / d stb / d s.cc / sec 1
Q Mscf / d q r.cc / sec
Mscf / d Mstb / d stb / d E
éùéùé ù
=
êúêúê ú
ëûëûë û
[][]
1ZT
Q Mscf / d 1000 1.84 q r.cc / sec
5.615
35.37p
éù
×× =
êú
ëû
QZT
9.265 Mscf / d q r.cc / sec
p
=
Including the remaining conversion factors in equ. (8.1 ) yields
e
wf
w
r
711 Q ZT 3
pp ln S
r4
khp
µ
æö
−= −+
ç÷
èø
(8.2)
Russell and Goodrich, comparing equ. (8.2) with the numerical simulation, found that
for the same reservoir and flow conditions the two were in close agreement providing
that the pressure
p
, at which the gas expansion factor was evaluated, was set equal to
the average of the current, average reservoir pressure and the bottom hole flowing
pressure i.e.
wf
pp
p
2
+
=
(8.3)
Furthermore, both µ and Z should also be evaluated at this same pressure so that
wf wf
pp pp
and Z Z
22
µµ
éù éù
++
==
êú êú
ëû ëû
(8.4)
and substituting these values of
p , µ and Z in equ. (8.2) gives
22
e
wf
w
r
1422 Q ZT 3
pp ln S
kh r 4
µ
æö
−= −+
ç÷
èø
(8.5)
Equ. (8.5) is the familiar p
2
formulation of the well inflow equation, under semi-steady
state conditions, which was tested by Russell and Goodrich and found to be applicable
over a wide range of reservoir conditions and flow rates.
Similarly, the transient line source solution for the same initial and boundary conditions
detailed in chapter 7, sec. 2, is
()
22
iwf
2
w
i
711 Q ZT 4 .000264kt
pp ln 2S
kh c r
µ
γφµ
æö
−= +
ç÷
ç÷
èø
(8.6)
wf wf
pp pp
and Z Z
22
µµ
éù éù
++
==
êú êú
ëû ëû
(8.4)

REAL GAS FLOW: GAS WELL TESTING 243
which is the real gas equivalent of equ. (7.10) in field units. This equation was also
found to compare favourably with the numerical simulation results, providing the
viscosity-compressibility product was evaluated as (µc)
i
, at the initial pressure p
i
,
(ref. sec. 8.8).
One obvious practical disadvantage in using the p
2
formulation can be appreciated by
considering a frequently occurring problem in gas inflow calculations, namely, the
calculation of p
wf
if both p and Q are known using, in this case, the semi-steady state
inflow equation. A schematic of the calculation procedure is shown in fig. 8.2. If it is
assumed that
p has been determined in the drainage volume of the well from material
balance considerations then, for a fixed offtake rate, it will be necessary to solve the
inflow equation by iteration to determine p
wf
since both µ and Z must be evaluated at
the pressure defined in equ. (8.4). In any iteration cycle
k
wf
p is calculated using values
of µ
k
and Z
k
evaluated at the pressure
k1
wf
(p p ) / 2
−
+ , where k is the iteration counter. For
k = 1, both µ
1
and Z
1
can be evaluated at some convenient starting pressure, which in
this case has been selected as
p . When the difference between successive values of
k
wf
p is less than some tolerance value (TOL) the iteration is terminated. Other
disadvantages in using the p
2
formulation for inflow equations will be discussed in
section 8.5.
8.4 THE AL-HUSSAINY, RAMEY, CRAWFORD SOLUTION TECHNIQUE
In their approach the authors attempted to linearize the basic flow equation, (5.1), using
the following version of the Kirchhoff integral transformation
()
b
p
p
pdp
mp 2
Z
µ
=
ò
(8.7)
which was given the name, in this present context, of "the real gas pseudo pressure".
REAL GAS FLOW: GAS WELL TESTING 244
p
Z
p
k = 1
µ
1
= ( )
Z
µ
p
1
= Z( )p
k = 1
1
/
2
2
e
wf
w
r
1422 Q Z T 3
plnS
p
kh r 4
ìü
æö
ïï
=− −+
íý
ç÷
ïï
èø
îþ
µ
kk
k
k
k = 1k +
k > 1
-1
wf
p
p
2
éù
+
=
êú
êú
ëû
µ
µ
k
k
-1
wf
p
p
Z
Z
2
éù
+
=
êú
êú
ëû
k
k
k = 1k +
-+
accept p
wf
-1
wf wf
TOL
pp
−−
kk
- from material balance
Fig. 8.2 Iterative calculation of p
wf
using the p
2
formulation of the radial,
semi-steady state inflow equation, (8.5)
The limits of integration are between a base pressure p
b
and the pressure of interest p.
The value of the base pressure is arbitrary since in using the transformation only
differences in pseudo pressures are considered i.e.
()
()
wf
bbwf
p
pp
wf
ppp
pdp pdp pdp
mp mp 2 2 2
ZZZ
µµµ
−= − =
òòò
As will be seen presently, it is possible, and indeed advantageous, to express all flow
equations in terms of these pseudo pressures rather than in the p
2
formulation of
Russell and Goodrich. However, conceptually it is more difficult and generally
engineers feel more comfortable dealing with p
2
rather than an integral transformation.
Therefore, it is worthwhile, at this stage, to examine the ease with which these
functions can be generated and used.

REAL GAS FLOW: GAS WELL TESTING 245
PVT data Numerical Integration Pseudo
pressures
p µ Z
2
ρ
µ
Ζ
2p
µ
Ζ
p∆
2p
p
µ
×∆
Ζ
2p
m(p) p
µ
=Σ ∆
Ζ
(psia) (cp) (psia)
2
/cp
400 .01286 .937 66391 33196 400 13.278×10
6
13.278×10
6
800 .01390 .882 130508 98449 " 39.380 " 52.658 "
1200 .01530 .832 188537 159522 " 63.809 " 116.467 "
1600 .01680 .794 239894 214216 " 85.686 " 202.153 "
2000 .01840 .770 282326 261110 " 104.444 " 306.597 "
2400 .02010 .763 312983 297655 " 119.062 " 425.659 "
2800 .02170 .775 332986 322985 " 129.194 " 554.853 "
3200 .02340 .797 343167 338079 " 135.231 " 690.084 "
3600 .02500 .827 348247 345707 " 138.283 " 828.367 "
4000 .02660 .860 349711 348979 " 139.592 " 967.958 "
4400 .02831 .896 346924 348318 " 139.327 " 1107.285 "
TABLE 8.1
Generation of the real gas pseudo pressure, as a function of the actual pressure;
(Gas gravity, 0.85, temperature 200°F)
All the parameters in the integrand of equ. (8.7) are themselves functions of pressure
and can be obtained directly from PVT analysis of the gas at reservoir temperature or,
knowing only the gas gravity, from standard correlations of µ and Z, again at reservoir
temperature. Table 8.1 lists a set of typical PVT data and shows how, using a simple
graphical method for numerical integration (trapezoidal rule), a table of values of m(p)
can be generated as a function of the actual pressures.
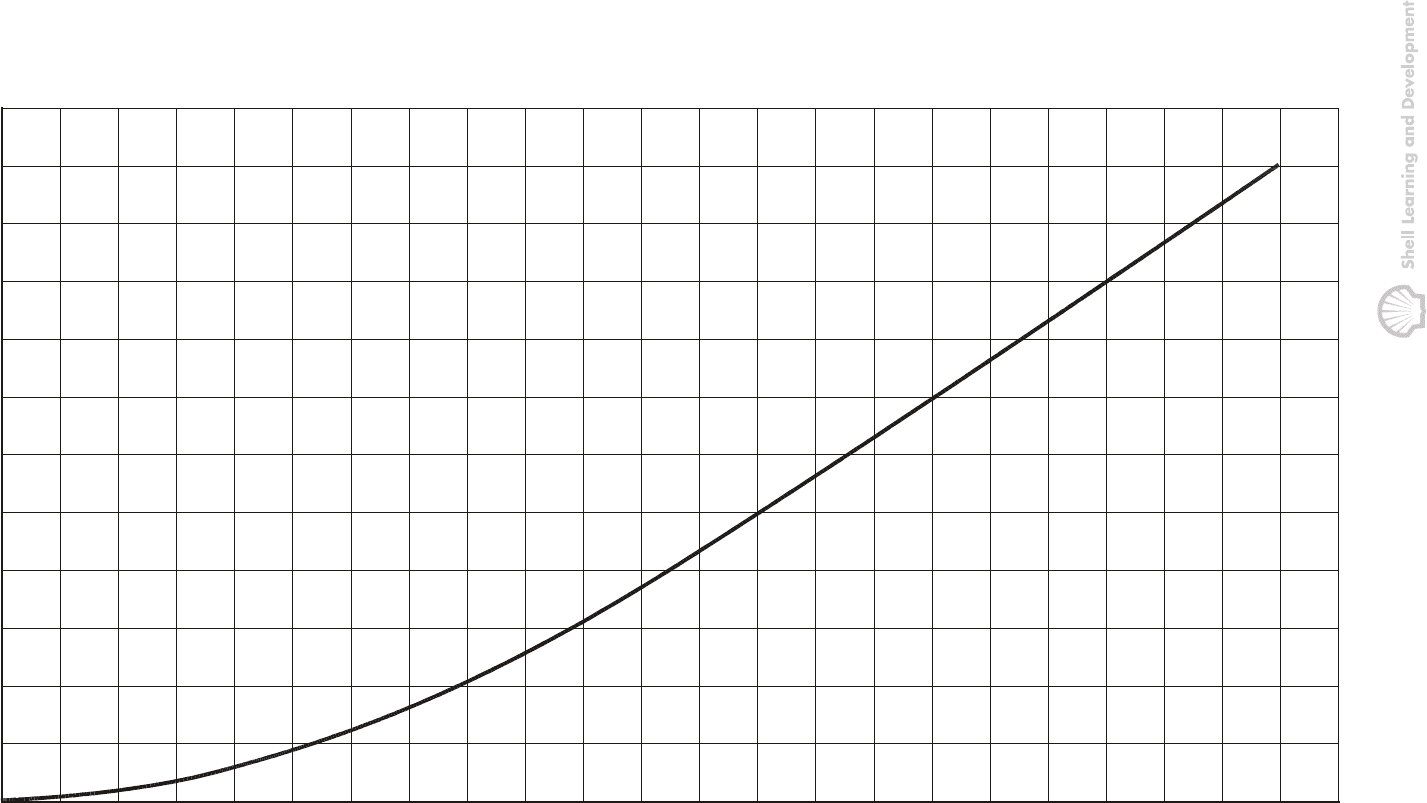
A graph of the values of m(p) versus pressure, corresponding to table 8.1, is included
as fig. 8.3. This plot is used in the gas well test exercises 8.1−3, (secs. 8.10−11), in
which it is assumed that for high pressures, in excess of 2800 psia, the function is
almost linear and can be described by
m (p) = (0.3457p - 414.76)× 10
6
psia
2
/cp
Having once obtained this relationship, the resulting plot should be preserved since it
will be relevant for the entire lifetime of the reservoir. Using the plot, it is always quite
straightforward to convert from real to pseudo pressures and vice versa.

REAL GAS FLOW: GAS WELL TESTING 246
In attempting to linearize the basic radial flow equation (5.1), (using, for the moment,
Darcy units), Al-Hussainy, Ramey and Crawford replaced the dependent variable p by
the real gas pseudo pressure m(p) in the following manner.
REAL GAS FLOW: GAS WELL TESTING 247
1200
0
800
600
400
200
1000
0
x10
6
m(p)
psia /cp
2
1000
2000
3000
4000 PRESSURE (psia)

REAL GAS FLOW: GAS WELL TESTING 248
Fig. 8.3 Real gas pseudo pressure, as a function of the actual pressure, as derived in
table 8.1; (Gas gravity, 0.85; Temperature 200°F)
m (p)
2p
Z
µ
2p
Z
µ
∆∆
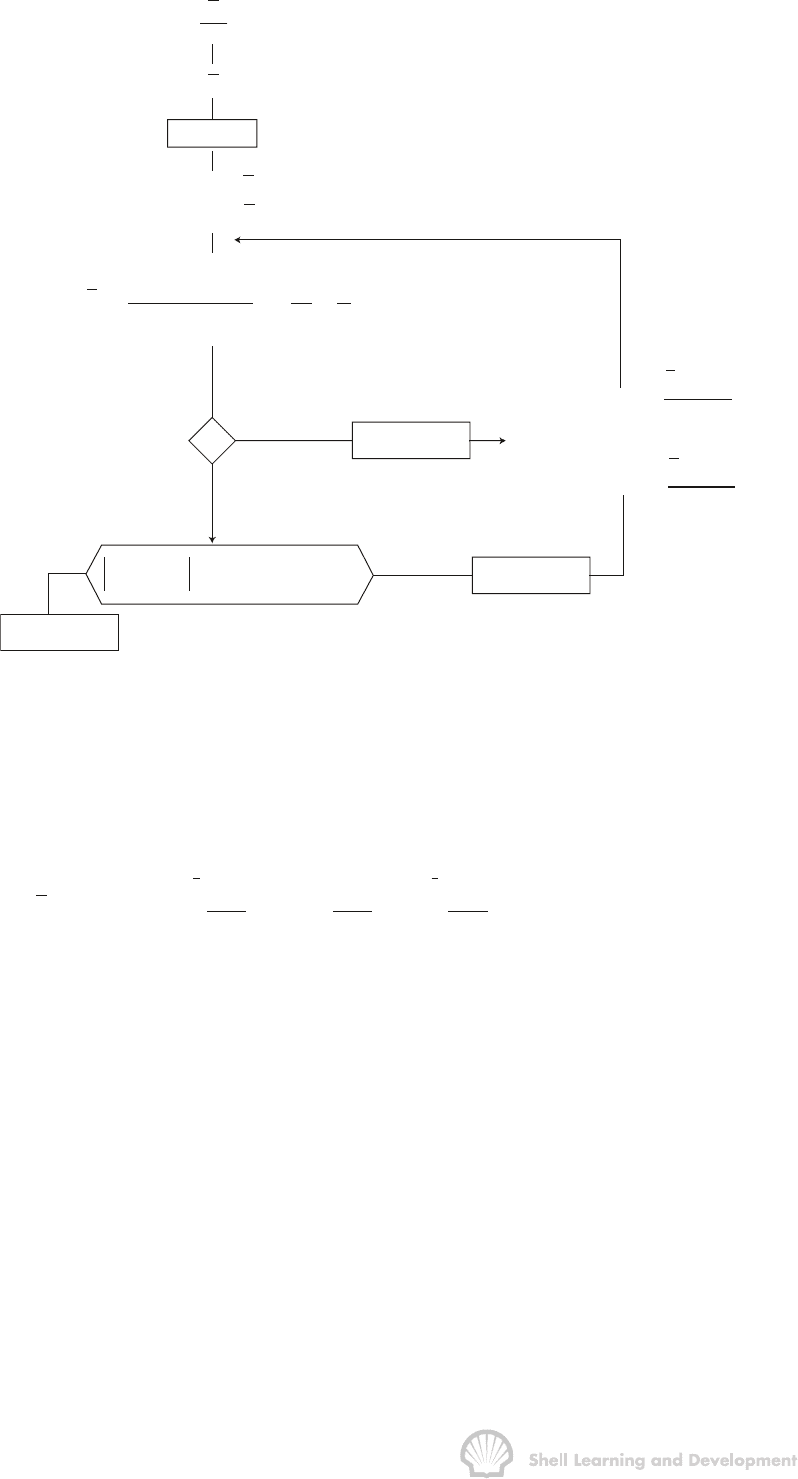
µ
(Area) = m (p) = (2p / Z)
p
∆
p
Pressure
∆
p
Fig. 8.4 2p/µZ as a function of pressure
Since
() ()
mp mp
p
rpr
∂∂
∂
=⋅
∂∂∂
and
()
mp
2p
p
µ
∂
=
∂Ζ
Then
()
mp
2p p
rr
µ
∂
∂
=
∂Ζ∂
(8.8)
and similarly
()
mp
2p p
tt
µ
∂
∂
=
∂Ζ∂
(8.9)
These relations are evident from fig. 8.4 and, substituting for ∂p/∂r and ∂p/∂t in
equ. (5.1), using equs. (8.8) and (8.9) gives
() ()
mp mp
1k
rc
rr 2p r 2p t
ρµ µ
φρ
µ
æö
∂∂
∂Ζ Ζ
=
ç÷
ç÷
∂∂ ∂
èø
(8.10)
Finally, using the equation of state for a real gas
Mp
ZRT
ρ
=
and substituting this expression for
ρ
in equ. (8.10) leads, after some cancellation of
terms, to the simplified expression
() ()
mp mp
1c
r
rr r k t
φµ
æö
∂∂
∂
=
ç÷
ç÷
∂∂ ∂
èø
(8.11)
Equation (8.11) has precisely the same form as the diffusivity equation, (5.20), except
that the dependent variable has been replaced by m(p).

REAL GAS FLOW: GAS WELL TESTING 249
Note that in reaching this stage it has not been necessary to make any restrictive
assumptions about the viscosity being independent of pressure or that the pressure
gradients are small and hence squared pressure gradient terms are negligible, as was
implicit in the approach of Russell and Goodrich.
Therefore, the problem has already been partially solved but it should be noted that the
term
φµ
c/k in equ. (8.11) is not a constant, as it was in the case of liquid flow, since for
a real gas both
µ
and c are highly pressure dependent. Equation (8.11) is therefore, a
non-linear form of the diffusivity equation.
Continuing with the argument; in order to derive an inflow equation under semi-steady
state flow conditions, then applying the simple material balance for a well draining a
bounded part of the reservoir at a constant rate
pV
cV q
tt
∂∂
=− =−
∂∂
(5.8)
and for the drainage of a radial volume element
2
e
pq
t
rh c
π
φ
∂
=−
∂
(5.10)
Also, using equ. (8.9)
()
2
e
mp
2p p 2p q
tt
rh c
µµ
π
φ
∂
∂
=⋅=−⋅
∂Ζ∂Ζ
(8.12)
and substituting equ. (8.12) in (8.11) gives
()
2
e
mp
1c2pq
r
rr r k rhc
φµ
µπ
φ
æö
∂
∂
=− ⋅ ⋅
ç÷
ç÷
∂∂ Ζ
èø
or
()
2
eres
mp
12pq
r
rr r rkh
π
æö
∂
∂
æö
=−
ç÷
ç÷
ç÷
∂∂ Ζ
èø
èø
(8.13)
Furthermore, using the real gas equation of state,
sc sc
sc
res
pq T
pq
T
æö
=
ç÷
Ζ
èø
equ. (8.13) can be expressed as
()
sc sc
2
sce
mp
2p q
1T
r
rr r Trkh
π
æö
∂
∂
=− ⋅
ç÷
ç÷
∂∂
èø
(8.14)
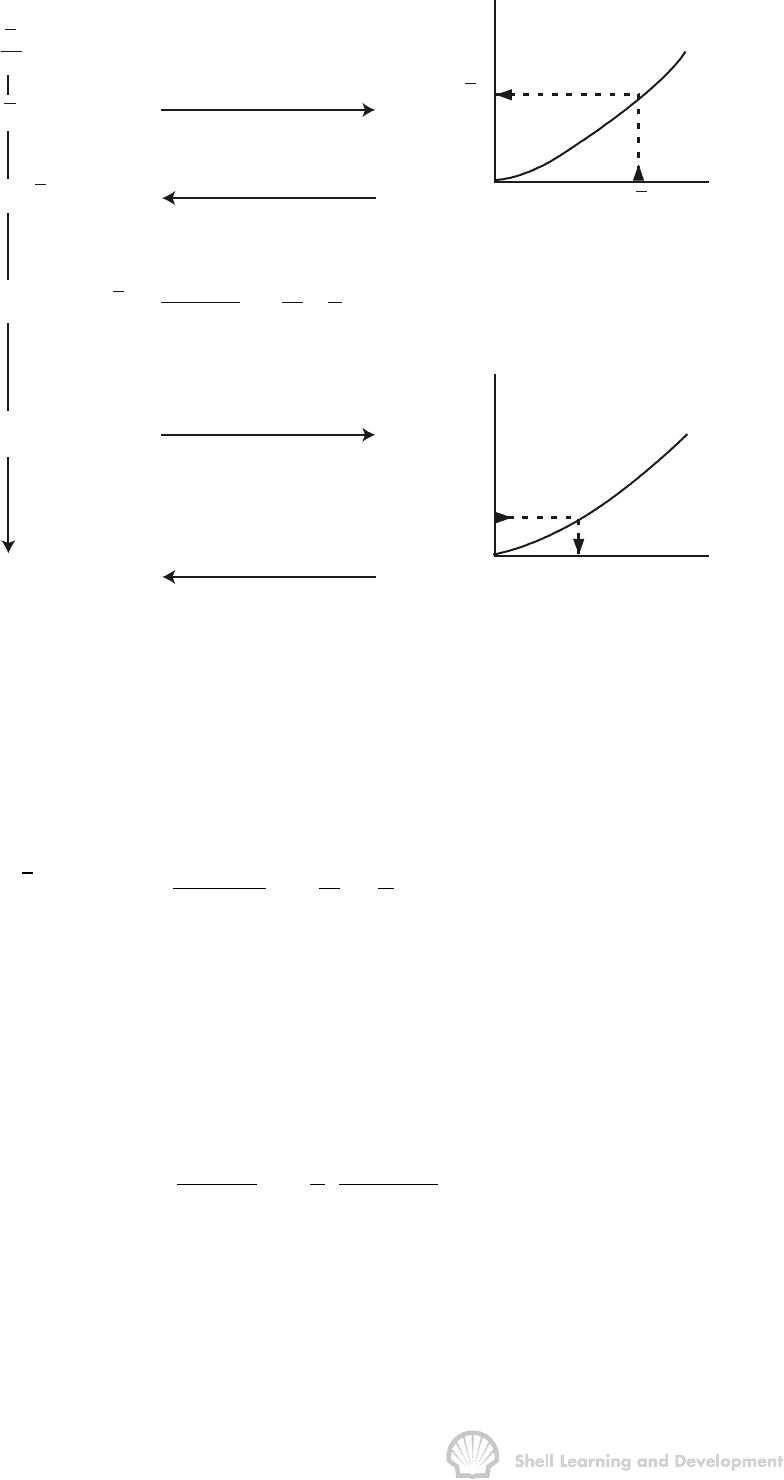
REAL GAS FLOW: GAS WELL TESTING 250
- from material balance
m (p)
m ( )p
p p
m (p)
m (p )
wf
p
wf
p
p
wf
m (p )
wf
m (p ) = m ( )
wf
p
m ( )p
p
p
Z
e
w
r
1422 QT 3
ln S
kh r 4
æ
ö
−−+
ç÷
è
ø
Fig. 8.5 Calculation of p
wf
using the radial semi-steady state inflow equation
expressed in terms of real gas pseudo pressures, (equ. 8.15)
For isothermal reservoir depletion, the right hand side of equ. (8.14) is a constant, and
the differential equation has been linearized. A solution can now be obtained using
precisely the same technique as applied in Chapter 6, sec. 2, for liquid flow. If, in
addition, field units are employed then the resulting semi-steady state inflow equation
can be expressed as
()
()
e
wf
w
r
1422 QT 3
mp mp ln S
kh r 4
æö
−= −+
ç÷
èø
(8.15)
Note that this equation has a similar form to the p
2
formulation of equ. (8.5), except that
the right hand side no longer contains the pressure dependent
µ
Z term which is now
implicit in the pseudo pressures. Because of this, the practical difficulty in having to
iterate when solving the inflow equation for p
wf
is removed. The relevant steps
corresponding to fig. 8.2 are shown in fig. 8.5. Similarly, the transient line source
solution, when expressed in pseudo pressures and field units, becomes
() ( )
()
iwf
2
w
i
711 QT 4 .000264kt
mp mp ln 2S
kh c r
γφµ
æö
−= +
ç÷
ç÷
èø
(8.16)
