Brewster H.D. Fluid Mechanics
Подождите немного. Документ загружается.

282
Wave Motions in Fluids Free from Viscosity
front
of
the
rI"A.-
Term indicates a wave moving from the exciter line in positive
r-direction, whereas the positive sign describes a wave moving towards the
exciter line.
Many general properties
of
wave motions known from physics can be
transferred to wave propagations in fluids. Nevertheless, in a book meant as
an introduction into fluid mechanics special considerations are required, in
particular is it necessary to arouse the deeper comprehension
of
the causes
of
the considered wave motions and to show how to deal with them on the basis
of
the Navier-Stokes equations.
The derivations
of
properties
of
theses wave motions shall show in which
way to proceed in fluid mechanics to derive the properties from the basic
equations
of
fluid mechanics. The aim
ofthe
descriptions/explications thus is
not
a broad consideration
of
different
wave motions in fluids,
but
an
introduction into the mathematical treatment
of
longitudinal and transverse
waves in fluids.
LONGITUDINAL
WAVES:
SOUND
WAVES IN
GASES
In order to be able to deal theoretically with the properties
of
longitudinal
sound waves in ideal gases. These can be stated for ideal gases as follows:
Continuity equation:
Momentum equations:
(j
=
1,2,3)
[
au}
aUj
1 ap (Tti}
p
--+U;r=-
=
----+pgj
at
ax;
ax}
ax;
Thermal energy equation:
State equation:
p
- =
RT
and e = c . T
P v
The above system
of
partial differential equations and thermo-dynamic
state equations comprises 7 unknowns, namely
U
1
'
U
2
'
U
3
'
P , p, e and
T,
for
the determination
of
which 7 equations are at disposal. Thus we have a closed
equation system which, with sufficient starting and boundary conditions, can
be solved in principle, therefore allows one to treat fluid motions.
The above-indicated equation system is considerably simplified when one
neglects the molecule-dependent heat and momentum transport, so that all
ternls
of
the momentum and energy equation provided with
qj
and
ti}'
can be
Wave Motions in Fluids Free from Viscosity
283
dropped and mass forces are neglected. When maintaining the Tensor way
of
writing, the equations can, after introduction
of
this neglect, be written as
follows:
Continuity equation:
ap
a(pu;)
-+
-0
at
ax;
-
Momentum equations:
(j =
1,2,3)
DU·
[aU
j
au
j
]
ap
p
__
J = P
--+U;--
=_
Dt
at
ax;
ax;
Energy equation:
De
DT
[aT
aT]
au·
p-
= pC - = pC
v
-+U;-
=
-P-'
Dt v
Dt
at
ax;
ax.
,
State equation:
P
- =
RT
and e = C
V
•
T
p
Taking into consideration that the continuity equation can be written as
follows:
ap +
u;
ap + p
au;
= Dp + P
au;
= 0
at
ax;
ax;
Dt
ax;
the following relation holds:
au;
=
_!Dp
ax;
pDt
Inserting into the energy equation and considering the state equation, the
energy equation can be written in the following form:
or:
PCv[DP
(~)]
=
p[!
DP]
Dt pR
p
Dt
~
DP =
(R+C
v
)!
Dp
P
Dt
C
v
P Dt
or considering R = (c
p
-
c)
and k = (cp/c
v
)
transcribed:
~
DP =
k!
Dp
P
Dt
P
Dt
Equation allows the following generally holding solution:
D D k
- (In
P)
=
-(lnp
)
Dt
Dt
284 Wave Motions in Fluids Freefrom Viscosity
or,
!H:.)]
~
0
~
:.
~
cons!.
The above relation gives expression to that the energy equation can be
reduced to the adiabatic equation
of
thermodynamics, assuming that no
molecule-dependent heat and momentum transport takes place and taking into
account the continuity equation and the state equation for ideal gases. Along
a streamline
of
a flow thus the following relation holds for the indicated
conditions:
P
k = const.
p
There exist now a number
of
fluid-mechanic processes in compressible
media that can be dealt with by means
of
equations, which result by further
simplifications from the above equations.
Assuming that there are flow processes that preferably take place such
that the velocity field only depends on a position coordinate, then it holds
U
I
= U
I
(Xl)'
U
2
= U
2
(Xl)
as well asU
3
= U
3
=
(Xl)
Moreover it shall hold that
aU
2
aU
I
aU
3
?lUI
-a
¢:
-a
and
-a
¢:
-a
so that the following equations can be stated:
xl
Xl Xl
Xl
Continuity equation:
Momentum equation:
Energy equation:
P
k = const.
p
By sound waves one understands the propagation
of
small disturbances
in gases.
The sound velocity thus is the velocity with which small disturbances
propagate in a fluid at rest. While for an incompressible fluid an infinitely
large propagation velocity results for small fluids, for compressible fluids
results a finite propagation velocity defined by the
kind'
of
gas and its
temperature.
The quantitative determination
of
the propagation velocity
of
small
disturbances in fluids at rest can be derived as stated below via the equations

Wave
Motions in Fluids Free from Viscosity
285
which
hold
for
non-stationary
one-dimensional flows
of
viscosity-free,
compressible fluids:
When computing the pressure variation
cap/axI).
in
consideration
of
equation, one obtaini:
ap =
(dP)
ap
ax
i
dp
ad
ax
i
'
as the pressure with the allowance
of
adiabatic conditions is only a function
of
a thermodynamical quantity, like the density. The derivation (dP/dp) has to
be formed for the adiabatic system condition required by equation. The
continuity equation and the momentum equation can thus be written
fqr one-
dimensional fluid flows:
ap
ap
aU
I
-+UI-+p-
=0
at
ax
i
aXI
aU
I
+U
I
aU
I
+.!.(dP)
ap
=0
at
ax
i
P dp
ad
aXI
With this two conditional equations are available for the two unknowns
U
I
arid P whose analytical solution is sought
on
the hypothesis that small
pressure and density fluctuations exist, i.e. that it holds:
U
I
= 0 + u'(x
I
,
t) P =
Po
+
p'
(xl'
t)
P =
Po
+ p'
(X
l'
t)
This relation inserted in the above conditional equations leads to:
a (
')'
a (
')
( ') au'
-
Po
+ P + u -
Po
+ P +
Po
+ P - = 0
at
ax
i
ax
i
-+u'-+
- P
+P'
-
au'
au'
(dP)
1 a
at
ax
i
dp
ad
(PO
+
p')
aXI
(0
) - 0
When considering that the disturbance variables p' and u' depend on place
and time, while the quantities
Po
and 70 depend neither on place nor time, the
following partial differential equation system results,
on
the hypothesis that
products
of
fluctuation quantities are negligible with reference to linear terms:
ap'
au' au'
(dP)
1
ap'
-+Po-=Oand-+
-
---=0
at
ax
i
at dp ad
Po
ax
i
These now are two conditional equations for
p'
and
u'that
can be brought
to a solution. In order to obtain this solution for the propagation
of
sound
waves, the first
of
the above two equations is derived with respect to
t:
a
2
p'
a
2
u'
--+Po--
=0
at
2
axlat
286
Wave Motions in Fluids Free from Viscosity
The second equation multiplied by
Po
and differentiated to xI yields:
a
2
u'
(dP)
a
2
p'
Po--+
-
-2-
= 0
aXIat
dp ad
ax
I
The subtraction
of
equations from equation results in the conditional
equation for p':
a2~'
_(dP)
(a2~'J
= 0
at dp ad
aXI
Furthermore, the derivation
of
equation to xI yields
a
2
p' a
2
u'
ataxI
+
Po
axt = 0
and multiplication
of
equation
by
(p
{ : L J and differentiation to t leads
to:
a
2
p'
p a
2
u'
--+
0
=0
ataxI
(dP)
at
2
dp
ad ( )
The subtraction
of
equation from and multiplication
by.!.
dP results
p
dp
ad
10
a
2
u'
(dP)
a
2
u'
aP
-
dp
ad axr = 0,
of
the conditional equation for the velocity fluctuation
u'.
When comparing the conditional equations for
p'
and u', one sees that
both are described
by
one and the same form
of
conditional equation, i.e.
p'
and u will show the same dependency on place and time. The solution is stated
by
the one-dimensional wave equation, i.e. there exists a wave propagation
velocity with a propagation velocity which generally reads:
J(dP)
~
~kpk-l
P
~
r;;E
~
Fkiif
dp
ad
pk
V"'p
The general solutions
of
the differential equations for p'(x
I
, t) and u(xI'
t)
can be stated as follows:
p'
=
fl
x
1 - ct) +
gp(X
I
+ct)
and u' = fu(x
I
-
ct)+glx
I
+ ct)
./p'u(x
I
- c
t
)
represents the respective wave which propagates in xcdirection
and g ,u
(xI
+ ct) the wave moving in the negative XI-direction.
further
considerations on the propagation
of
disturbances in compressible
Wave Motions in Fluids Free from Viscosity
287
media at rest can now be made on the basis
of
the above results. To this effect
one computes from the general solution for
u (wave
in
positive xcdirection):
and
aaUt'
=-c(!)
VII
mit
II
=
Xl
-ct
au' au'
-=-c-
at
ax!
From the momentum equation follows:
au' c
2
ap' ap'
-=---=-c-
at
Po
aXI
aXI
or
transcribed
-=--
au'
cap'
=>
-=-
u' p'
aXI
Po
aXI
c
Po
When we have a disturbance in the form
of
a compression wave, i.e.
p'
>
0,
then also u' >
O,and
this means that the fluid particles move in the direction
of
the disturbance when a compression disturbance occurs. When on the other
hand an expansion disturbance occurs, i.e.
p'
<
0,
then also
u'
<
0,
and in this
case the fluid particles move opposite to the direction
of
the propagation
of
the disturbance.
The most important result
of
the above derivations was that small
disturbances
in
non-viscose and compressible fluids at rest propagate with
sound velocity that can be computed as follows:
c=
FdP)
=JkiiT
VldP
)ad
TRANSVERSAL
WAVES:
SURFACE
WAVES
General Solution Set-up
On the free surfaces
of
fluids wave appearances can occur, i.e. propagation
of
transversal waves owing to introduced disturbances. These can be two-
or
three-dimensional, however, the analytical treatment
of
surface waves
presented here concentrates on two-dimensional surfaces. By linearization
of
the basic equations written in potential form one obtains the partial differential
equations solved normally for surface waves. These indicate that the field
of
propagation
of
surface waves belongs to the potential theory.
Their treatment takes place separately nevertheless, as a special problem
is concerned, i.e. a special class
of
flow appearances whose treatment
correspondingly requires a special methodology. The latter is shown below
in
288
Wave Motions in Fluids Freefrom Viscosity
an introducing way. The relations stated in the following can again be derived
from the basic equations,
which
can
be stated as follows for a fluid-
mechanically ideal fluid, i.e. a fluid free from viscosity:
au
aU
j
1 ap
-+U
j
·_-
=
---+g.
at
ax;
p
aXj
J
When integrating this equation over a period
of
time
't,
one obtains
_
't
aU
j
1 a
't 't
U
j
+
fUj--dt=---
f
Pdt
+
fgjdt
o
ax;
p
aXj
0 0
't
This equation can now be interpreted with 1t = f
Pdt
as the pressure
o
impulse during the time interval
't,
for small time intervals
't
as follows for p
= const:
- a P .
't
f
aU
j
u·
=--mit
U·--dt'l:;JO
J
ax
j
PO'
aXj
and
Thus the fluid motion generated as a result
of
pressure impulses on free
surfaces is described by a velocity potential, by
U
j
= U
j
:
-
84>
. P
U·
=
--
mIt
~=-
J
aXj
P
The motion thus is irrotational. Strictly speaking all this holds only at the
free surface and the determination
of
<I>
in the entire flow area requires further
considerations still. The continuity equation can be written as follows for
<1>.
a2~ a2~ a2~
a2~
---'--=0=-+-+-
ax
j
•
ax;
axfax?
axj
The momentum equation can be written as stated below:
DU
j
1
ap
--=-_·_+g·/·U·
Dt P
aXj
J J
or
can be transcribed after multiplication by
~
as follows:
.!l.-(.!.u~)=-.!.
DP
_.!.
ap _
DG
Dt 2 J P Dt P at Dt
DG
ag
Withg.=
-P-
for - = 0
'J
Dt at '
Wave Motions in Fluids Freefrom Viscosity
or
transcribed:
a<\>
P 1 2
-+-+-u·
+G
=F(t)
at
p 2 J
289
The
function F (I) introduced by the integration can be included into the
potential
<\>,
so
that
it holds:
a<\>
P 1 2
-+-+-U·
+G
==0-
at
p 2 ]
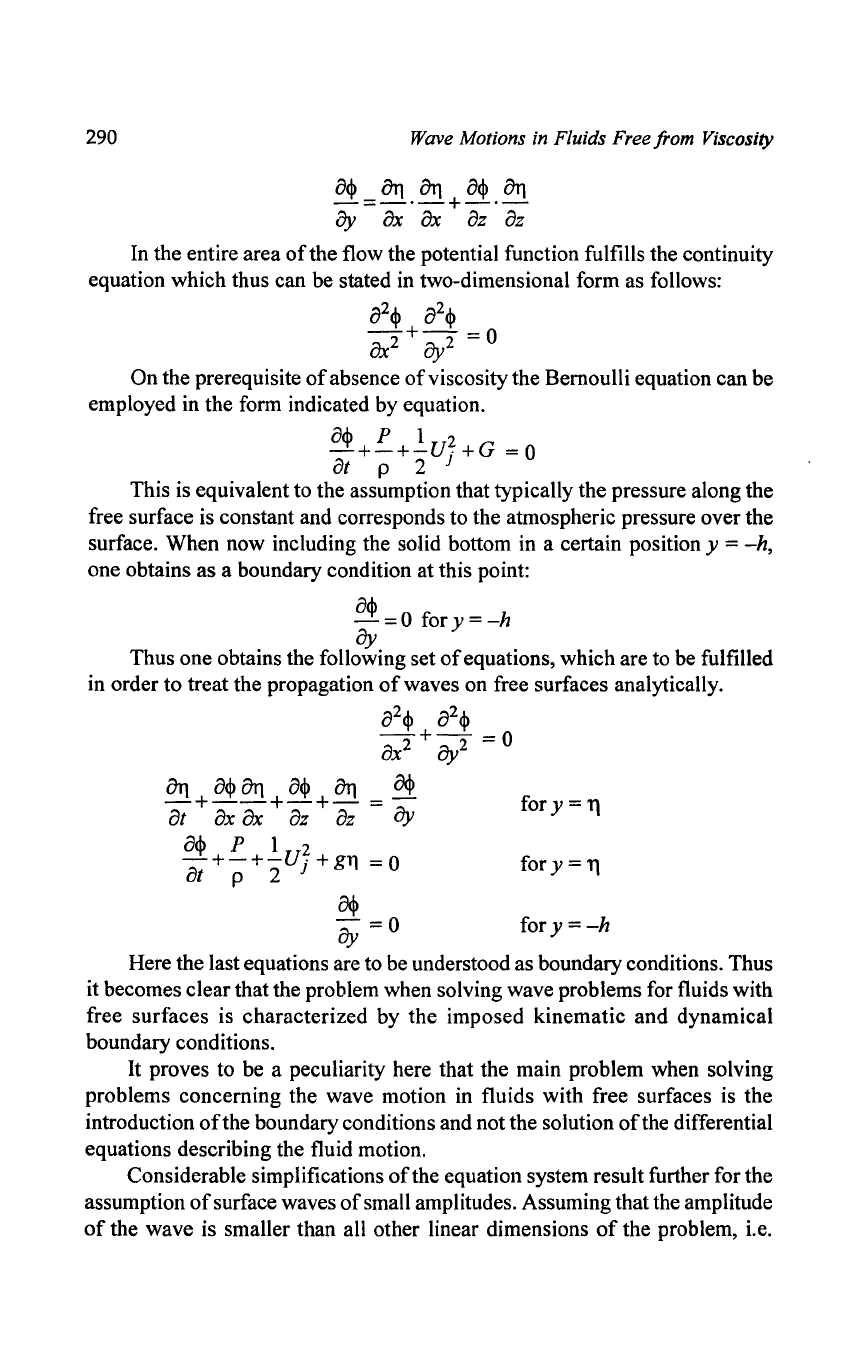
Represents a two-dimensional surface wave whose deflection, measured
from the. position
of
rest x
2
==
0 can be stated a follows:
x
2
==
Y
==
h(xl'
t)
==
h(x, t)
~
"\
><s
= z
u
3
=W
~=y
u
2
= V
Fig. Two-dimensional Surface Wave
The
kinematic boundary condition
of
the flow problem
to
be solved
can
thus
be
stated
as
follows:
Y = ll(x, t) = 0
This means that a fluid particle which belonged
to
the fluid surface
at
a
point in time
t will always belong
to
the free surface. From equation results
with u
j
as fluid velocity
of
the considered wave motion
D a a
-(Y-ll)=O=-(Y-ll)+U;-(Y-ll)
= 0
Dt at
ax;
or
the deflections carried out:
a..,
a.., a..,
---Ul-+
U
2
-U3-
= 0
at
ax
1
ax
3
When introducing now the potential function
<I>
with
U =
8<\>,
u2
==
8<\>
and u3
8<\>
1
aXl
ax
2
ax
3
the following relation results for the free surface with
Xl
=.
X,
x
2
= Y and
x3
==
z:
290
Wave Motions in Fluids Free from Viscosity
8<\>==<711.<711+
8
<\>.<711
By
ax ax
8z 8z
In the entire
area
of
the
flow the potential function fulfills the continuity
equation
which
thus
can
be
stated in two-dimensional form as follows:
8
2
<\>
8
2
<\>
-2+-2
=0
ax
ay
On
the prerequisite
of
absence
of
viscosity
the
Bernoulli equation
can
be
employed in
the
form indicated
by
equation.
8<\>
+ P
+~u~
+G
= 0
8t p 2 }
This is equivalent to the assumption
that
typically
the
pressure along
the
free surface is constant and corresponds to the atmospheric pressure over
the
surface.
When
now
including the solid
bottom
in a certain position y =
-h,
one
obtains
as
a boundary condition
at
this point:
8<\>
-
==
0 for y =
-h
ay
Thus
one
obtains the following set
of
equations,
which
are to
be
fulfilled
in
order
to
treat
the propagation
of
waves
on
free surfaces analytically.
8
2
<\>
8
2
<\>
-2
+-2
=0
8x
ay
<711+8<\><711+8<\>+<711
=
8<\>
8t
ax
ax
8z
8z
ay
8<\>
P 1 2
-+-+-U·
+gll
=0
8t p 2 }
8<\>
--0
ay-
for y =
11
for y =
11
for
y=-h
Here the last equations are to
be
understood as boundary conditions.
Thus
it becomes
clear
that
the problem when solving wave problems for fluids
with
free
surfaces
is
characterized
by
the
imposed
kinematic
and
dynamical
boundary conditions.
It
proves to be a peculiarity here
that
the
main problem
when
solving
problems
concerning
the
wave
motion
in fluids
with
free surfaces is
the
introduction
of
the boundary conditions and
not
the solution
of
the differential
equations describing the fluid motion.
Considerable simplifications
of
the equation system result further for
the
assumption
of
surface waves
of
small amplitudes. Assuming
that
the amplitude
of
the
wave
is smaller
than
all
other
linear dimensions
of
the problem, i.e.
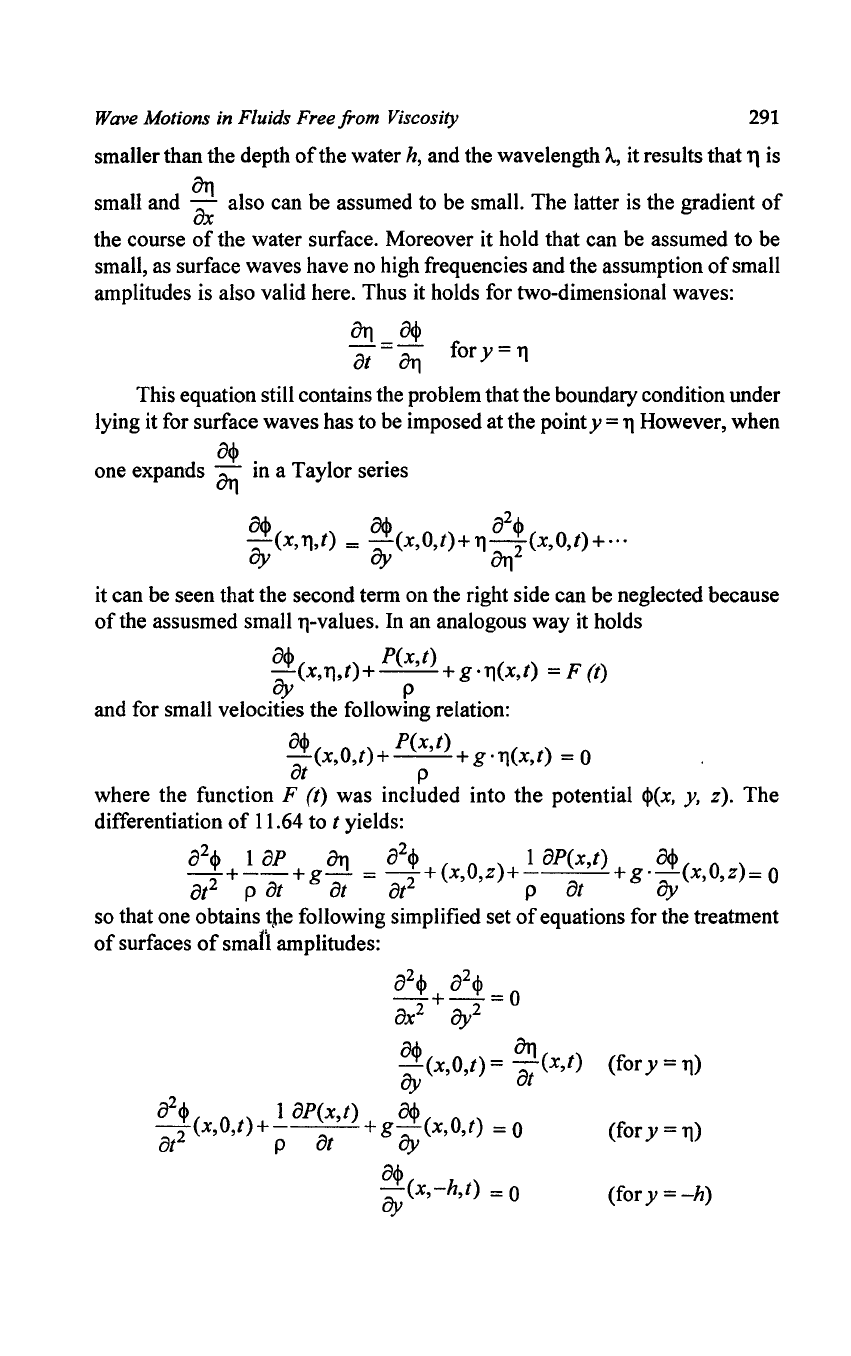
Wave
Motions in Fluids Free from Viscosity
291
smaller than the depth
ofthe
water
h,
and the wavelength
A,
it results that
11
is
small and
: also can be assumed to be small. The latter is the gradient
of
the course
of
the water surface. Moreover it hold that can be assumed to be
small, as surface waves have no high frequencies and the assumption
of
small
amplitudes is also valid here. Thus it holds for two-dimensional waves:
for
y =
11
This equation still contains the problem that the boundary condition under
lying it for surface waves has to be imposed at the
pointy
=
11
However, when
d
8
4>.
TI
.
one expan' s
BTl
10
a
ay
or
serIes
84>
aq,
8
2
4>
-(x,l1,l)
=
-(x,0,1)+11-
2
(x,O,I)+···
By
By
BTl
it can be seen that the second term on the right side can be neglected because
of
the assusmed small 11-values. In an analogous way it holds
84>
(x,l1,I)+
P(x,t)
+ g.l1(x,t) = F (t)
By
p
and for small velocities the following relation:
aq,
(x,O,t) +
P(x,l)
+ g . l1(x,t) = °
8t P
where the function F (I)
was
included into
the
potential
<I>(x,
y,
z).
The
differentiation
of
11.64 to t yields:
8
2
4>
1 8P
BTl
8
2
4>
1 8P(x,t)
aq,
-+--+g-
=
-+(x,O,z)+
+g·_(x,O,z)=
°
81
2
P 8t 8t 8t
2
p 8t
By
so that one obtains
t}le
following simplified set
of
equations for the treatment
of
surfaces
of
smaI'l amplitudes:
8
2
4>
8
2
4>
-+-=0
8x2
By2
: (x,O,t) = :
(x,t)
(for y =
11)
8
2
4>
1 8P(x,l)
aq,
8t
2
(x,O,t)+ p 8t + g
By
(x,O,t) = ° (for y =
11)
84>
-(x,-h,t)
= ° (for y =
-h)
By
