Blum W., Riegler W., Rolandi L. Particle Detection with Drift Chambers

Подождите немного. Документ загружается.

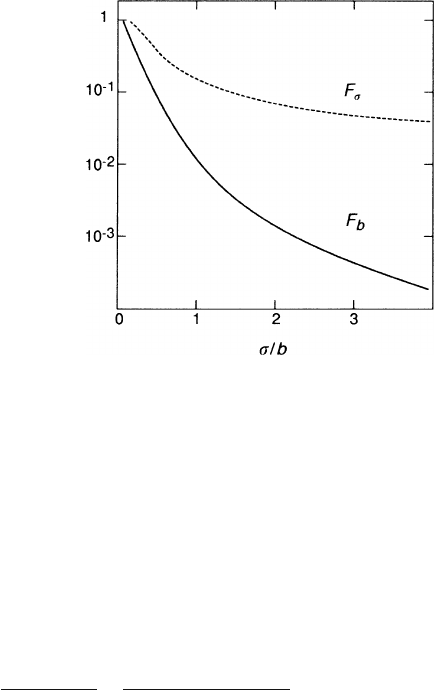
288 7 Coordinate Measurement and Fundamental Limits of Accuracy

Fig. 7.20 Declustering as a

function of

/b.Plotofthe

functions deﬁned in (7.82)

and (7.83)

the average (7.10) is only N

eff

; when the diffusion is large compared to the width b

of the drift cell, there is no correlation and the reduction factor of the angular term

is N, the total number of collected electrons. The functions F

and F

are shown in

Fig. 7.20.

If we had calculated the plot of Fig. 7.3 with (7.81) (instead of using the numer-

ical simulation (see Sect. 7.2.3)), we would have found a similar behaviour but a

somewhat steeper increase of N

eff

(

) with

/l. This is a consequence of the as-

sumption introduced: we have assumed that the average number m

of electrons

originated by clusters of size i and collected by the wire is constant. If we do not

make this assumption, then (7.65) contains an additional term,

Var(X

∑

Var( ¯x

)

∑

( ¯x

−X

)

Var(m

)

. (7.84)

The second term plays a role when the rare large clusters are present, since they

dominate the average. The numerical simulation introduced in Sect. 7.2.3 and used

to produce Fig. 7.3 takes into account all the various effects due to the peculiar

shape of the cluster-size distribution and then correctly produces a variance larger

than that evaluated in (7.81) and consequently a smaller N

eff

(

References

[ABR 64] M. Abramovitz and I. Stegun (eds.), Handbook of Mathematical Functions (Dover,

New York 1964) p. 297.

[AME 83] S.R. Amendolia et al. E×B and angular effects in the avalanche localization along the

wire with cathode pad readout, Nucl. Instrum. Methods 217, 317 (1983)

[ATL 00] M. Aleksa, M. Deile, J. Dubbert, C.W. Fabjan, C. Gruhn, N.P. Hessey, W. Riegler and

T. Sammer, Rate effects in high-resolution drift chambers, Nucl. Instr. Meth. Phys.

References 289

Res. A 446 435-443 (2000), also M. Aleksa, Doctoral Diss. Performance of the ATLAS

Muon Spectrometer, Techn. Univ. Wien (Sept. 1999)

[ATL 97] ATLAS Muon spectrometer: Technical Design Report, CERN, Geneva, Switzerland,

CERN/LHC 97-22, 1997

[BAR 82] A. Barbaro Galtieri, Tracking with the PEP-4 TPC, TPC-LBL 82-24, Berkeley preprint

1982 (unpublished)

[BLU 86] W. Blum, U. Stiegler, P. Gondolo and L. Rolandi, Measurement of the avalanche

broadening caused by the wire E×B effect, Nucl. Instrum. Methods A 252, 407 (1986)

[BOY 95] I. R. Boyko, G. A. Chelkov, V. I. Dodonov, M. A. Ignatenko, M. Yu. Nikolenko, Vi-

bration of signal wires in wire detectors under irradiation, Nucl. Instrum. Methods in

Phys. Res. A367, 321 (1995)

[CAM 09] N. R. Campbell, The study of discontinuous phenomena, Proc. Cambridge Phys. Soc.,

15, 117–136 (1909)

[CRA 51] H. Cramer, Mathematical Methods of Statistics (Princeton Univ. Press 1951), Sec. 28.6

[HAR 84] C.K. Hargrove et al., The spatial resolution of the time projection chamber at TRIUMF,

Nucl. Instrum. Methods 219, 461 (1984)

[RIC 44] S.O. Rice, Mathematical Analysis of Random Noise, Bell Syst. techn. J. 23, 282

(1994), 24, 46 (1945), reprinted in: N. Wax, Selected Papers on Noise and Stochas-

tic Processes, (Dover Publications, New York 1954, 2003)

Chapter 8

Geometrical Track Parameters and Their Errors

Now we come to the point to which all the efforts of drift-chamber design lead up –

the determination of the track parameters. The point of origin of a track, its angles of

orientation, and its curvature in a magnetic ﬁeld are the main geometric properties

that one aims to measure. The proof of the pudding is in the eating, and the proof of

the drift chamber is in the track parameters.

In a particle experiment a track is often measured by several detectors, and the

accuracy of its parameters depends on the integrity of the whole ensemble, and in

particular on the knowledge of the relative detector positions. Likewise, the track

parameters determined with a drift chamber depend on its overall geometry, the

electrode positions, ﬁelds and electron drift paths. If one wants to calculate the

achievable accuracy of a drift chamber in all parts of its volume, one needs to

have quantitative knowledge of these factors in addition to the point-measuring ac-

curacy. Such knowledge is often difﬁcult to get, and then one may want to work

the other way around: starting from a measurement of the achieved accuracy of

track parameters, one can compare it to that expected from the point-measuring

accuracy alone. If they agree, then the other factors make only a small contri-

bution, if they do not, the contribution of the other factors is larger, or perhaps

dominant.

The achieved accuracy can be measured in a number of ways. The common meth-

ods include the following: vertex localization by comparing tracks from the same

vertex, momentum resolution by measuring tracks with known momentum, a com-

bination of momentum and angular precision with a measurement of the invariant

mass of a decaying particle.

It is obviously our ﬁrst task to ascertain the accuracy that can be achieved in a

given geometry when considering the resolution of each measuring point alone, ig-

noring all the other factors by assuming they are negligible or have been corrected

for. We will do this in two sections, one for the situation without magnetic ﬁeld,

using straight-line ﬁts, the other for the situation inside a magnetic ﬁeld, where a

quadratic ﬁt is appropriate. One section is devoted to accuracy limitations due to

multiple Coulomb scattering in parts of the apparatus. Finally, the results on spec-

trometer resolution are summarized.

W. Blum et al., Particle Detection with Drift Chambers, 291

doi: 10.1007/978-3-540-76684-1

 Springer-Verlag Berlin Heidelberg 2008

292 8 Geometrical Track Parameters and Their Errors

A general treatment of the methods of track ﬁtting using different statistical ap-

proaches is presented in the book of B

ock, Grote, Notz and Regler [BOC 90]. Here

our aim is more modest and more speciﬁc: on the basis of the least-squares method

we would like to understand how the point-measuring errors of a drift chamber

propagate into the track parameters.

8.1 Linear Fit

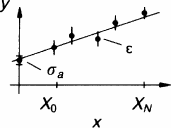
Referring to Fig. 8.1, there are N + 1 points at positions x

(i = 0,1,...,N) where

the coordinates y

of a given track were measured. We ask for the accuracy with

which the coordinate and the direction of the track are determined at x = 0. If there

is a magnetic ﬁeld, the track is curved. As a ﬁrst step we imagine the curvature to

be well measured outside the vertex detector so that we are allowed to consider a

straight-line extrapolation; the inﬂuence of an error of curvature will be estimated

in Sect. 8.2.

A least-squares ﬁt to the straight line

y = a+ bx (8.1)

is obtained by minimizing

∑

−a −bx

)

, (8.2)

where the

are the uncorrelated point-measuring errors whose reciprocal squares

are used as weights. The two conditions

∂χ

∂

a = 0 and

∂χ

∂

b = 0 lead to the

following linear equations:

+ bS

∑

+ bS

∑

where S

∑

, S

∑

, S

∑

, S

∑

, S

∑

(all sums from 0 to N); also D = S

−S

. The two linear equations are solved for

the best estimates of the coefﬁcients a and b by

a =(S

−S

)/D ,

b =(S

−S

)/D .

Fig. 8.1 Straight-line ﬁt to

the origin

8.1 Linear Fit 293

Let us now consider the ﬂuctuations of a and b. They are caused by the ﬂuctua-

tions of the y

, which can be characterized by the (N +1)

covariances [y

] deﬁned

as the average over the product minus the product of the averages:

]=y

−y

y

 ; (8.3)

they vanish when i = k because the y

ﬂuctuate independently of one another, and

] is what was called

before.

The ﬂuctuations of a and b are described by their covariance matrix,



(

)

[ab]

[ab][b

]



and we have to determine how it depends on the quantities [y

To compute [a

]=a

−a

we use (8.3):



∑

y

−y





+ S

∑

y

−x

y





−2S

∑

y

−y







∑

+ S

∑

−2S

∑



= S

/D .

(8.4)

The other two elements are calculated in a similar way, and the covariance matrix

of the ﬁtted parameters is



(

)

[ab]

[ab][b

]





−S



. (8.5)

Since the ﬁt is linear, the result (8.4) and (8.5) depends only on the covariances

of the y

and not on their averages.

8.1.1 Case of Equal Spacing Between x

and x

We evaluate (8.3) for N + 1 equally spaced points with equal point errors

.Inthis

case S

=(N + 1)/

, S

=(N + 1)(x

+ x

)/(2

) and S

=(N + 1)[x

−x

)

(2 + 1/N)/6]/

. We see that the result will depend on the number of

points and on the ratio of the two extreme distances x

and x

. Let us introduce the

ratio r, which expresses how far – in units of the chamber length – the centre of the

chamber is away from the origin:

r =

+ x

2(x

−x

)

. (8.6)

294 8 Geometrical Track Parameters and Their Errors

Table 8.1 Values of the factor Z(r,N) in (8.7) for various distances r of the vertex from the centre

of the chamber, in units of the chamber length, and for various numbers of N + 1 equally spaced

sense wires

N r: 5.0 3.0 2.0 1.5 1.0 0.75 0.60 0.50

1 10.1 6.08 4.12 3.16 2.24 1.80 1.56 1.41

2 12.3 7.42 5.00 3.81 2.65 2.09 1.78 1.58

5 14.7 8.84 5.94 4.50 3.09 2.41 2.02 1.77

9 15.7 9.45 6.35 4.81 3.29 2.55 2.13 1.86

19 16.5 9.94 6.67 5.04 3.44 2.67 2.22 1.93

inﬁn. 17.3 10.4 7.00 5.29 3.61 2.78 2.31 2.00

Inserting the sums into (8.4) and taking the square root we obtain the vertex local-

ization accuracy



(N + 1)

Z(r,N) , (8.7)

with

Z(r,N)=



12r

+ 1 +



1/2

Values of Z have been computed for some r and N; results are listed in Table 8.1.

We observe that the strongest functional variation is with the ratio r; this is under-

standable since it has the meaning of a lever–arm ratio. For any given r, the factor Z

is more favourable for small N; this means that the dependence on N is weaker than

the 1/

√

(N + 1) rule. The error in the direction is given by

−x

√

N + 1



12N

N + 2

. (8.8)

8.2 Quadratic Fit

In a magnetic spectrometer the particle momenta are determined from a measure-

ment of the track curvature. We recall that in a homogeneous magnetic ﬁeld B the

trajectory is a helix with radius of curvature

R = p

/eB , (8.9)

where e is the electric charge of the particle and p

its momentum transverse to the

magnetic ﬁeld. A remark concerning units: relation (8.9) is in m, if e is in As, B in T

(i.e. Vs/m

) and p

in N s (i.e. V A s

/m). If one wants to express the momentum

in units of GeV/c, then one must multiply p

by the factor f that says how many

GeV/c there are to one N s. This factor is

f =

1GeV/c

1Ns

e(As)(V)

(VAs

/m)2.9979×10

(m/s)

, (8.10)

8.2 Quadratic Fit 295

so that (8.9), to better than one tenth of a percent, takes on the simple form

R =



GeV/c



. (8.11)

This radius has to be measured in a plane perpendicular to B. Our interest is in the

large momenta because they are the most difﬁcult to measure precisely. Therefore

we approximate the helix trajectory, which is actually a circle in a plane perpendic-

ular to its axis, by a parabola in such a plane,

y = a+ bx +(c/2)x

, (8.12)

up to terms of the order of x

, where c = 1/R. The measured y

at positions x

are ﬁtted to (8.12) with the aim of determining a, b and c.

8.2.1 Error Calculation

One is interested in the errors of a, b and c. Our procedure is similar to that of

Gluckstern [GLU 63], but is more general in that it includes the extrapolation to a

vertex point at an arbitrary distance.

The linear equations for a, b and c are derived from the conditions of a minimal

and read as follows (the weighting factor at each point is denoted by f

+ bF

+(c/2)F

∑

+ bF

+(c/2)F

∑

(8.13)

+ bF

+(c/2)F

∑

where

∑

)

. (8.14)

All sums run from n = 0ton = N. The solution of (8.13) for our three quantities is

a =

∑

b =

∑

, (8.15)

∑

where the G

, P

and Q

are expressed using determinants of the F

= f



− f



+ f



296 8 Geometrical Track Parameters and Their Errors

= −f



+ f



− f



= f



− f



+ f



They have the property that

∑

= 0 (8.16)

and

∑

. (8.17)

Using (8.16), the variance of the ﬁrst coefﬁcient is given by

∑∑

]



∑

, (8.18)

where [y

] denotes the covariance of the measurements at two points x

and x

The other elements are formed in the same manner.

The point-measurement errors are equal and uncorrelated. We write

(8.19)

and obtain

(

)

∑



∑

[ab]=

∑



∑

(

)

∑



∑

, (8.20)

[ac]=2

∑



∑

[bc]=

∑



∑

¨ x

(

)

∑



∑

8.2.2 Origin at the Centre of the Track – Uniform Spacing of Wires

In order to facilitate the evaluation of (8.20) we begin by setting the origin of the x

coordinates at the centre of the track. Let

8.2 Quadratic Fit 297

= x

−x

, with x

∑

/(N + 1) , (8.21)

and

L = x

−x

For uniform spacing and uniform weights f

= 1, the subdeterminants are easily

calculated to be

= F

−q

= q

−F

) ,

= −F

+ q

with F

∑

. Note that F

= F

= 0 for symmetry. The other F

are

= N + 1 ,

= L

(N + 1)(N + 2)

12N

= L

(N + 1)(N + 2)(3N

+ 6N −4)

240N

S = F

−F

= L

(N −1)(N + 1)

(N + 2)(N + 3)

180N

(8.22)

A combination S, needed later, has been included here. Inserting the subdetermi-

nants into (8.20) we ﬁnd the six elements of the covariance matrix, valid at the

origin, that is the centre of the track:

(

)

/(F

−F

) ,

[ab]=0 ,

(

)

[ac]=−2

/(F

−F

) ,

[bc]=0 ,

(

)

= 4F

/(F

−F

) .

(8.23)

Note that there is no correlation between the direction b and the offset a or the

curvature c in the middle of the track. The elements of the covariance matrix (8.23)

will assume different values outside the centre, and this will be treated in Sect. 8.2.4.

The variance of the curvature, however, is the same all along the track, and we can

evaluate it by inserting (8.22) into the last of the equations (8.23). The variance of

298 8 Geometrical Track Parameters and Their Errors

Table 8.2 Values of the factors A

in (8.24) and

√

)/8 in (8.26) for various values of N.(The

number of measuring points is N + 1.)

√

)/8

2961.22

3811.12

4 73.1 1.07

5 67.0 1.02

6 61.7 0.982

8 53.2 0.912

10 46.6 0.853

inﬁn.

720

N + 5

3.35

√

N + 5

the curvature is equal to

720N

(N −1)(N + 1)(N + 2)(N + 3)

, (8.24)

where we have deﬁned a factor A

, some values of which are enumerated in

Table 8.2.

8.2.3 Sagitta

The maximum excursion of a piece of a circle over the corresponding chord is called

its sagitta. The sagitta s of a track with length L and radius of curvature R  L

is proportional to the square of the track length, as can be seen from the sketch

of Fig. 8.2 when developing the cosine of the small angle (L/2R) into powers of

(L/2R):

s = L

/8R , (8.25)

so its r.m.s. error becomes

s =

√

. (8.26)

This ratio between the errors of the sagitta and the individual point coordinates is

also contained in Table 8.2.

Fig. 8.2 Sagitta s