Blank B.E., Krantz S.G. Calculus: Single Variable
Подождите немного. Документ загружается.

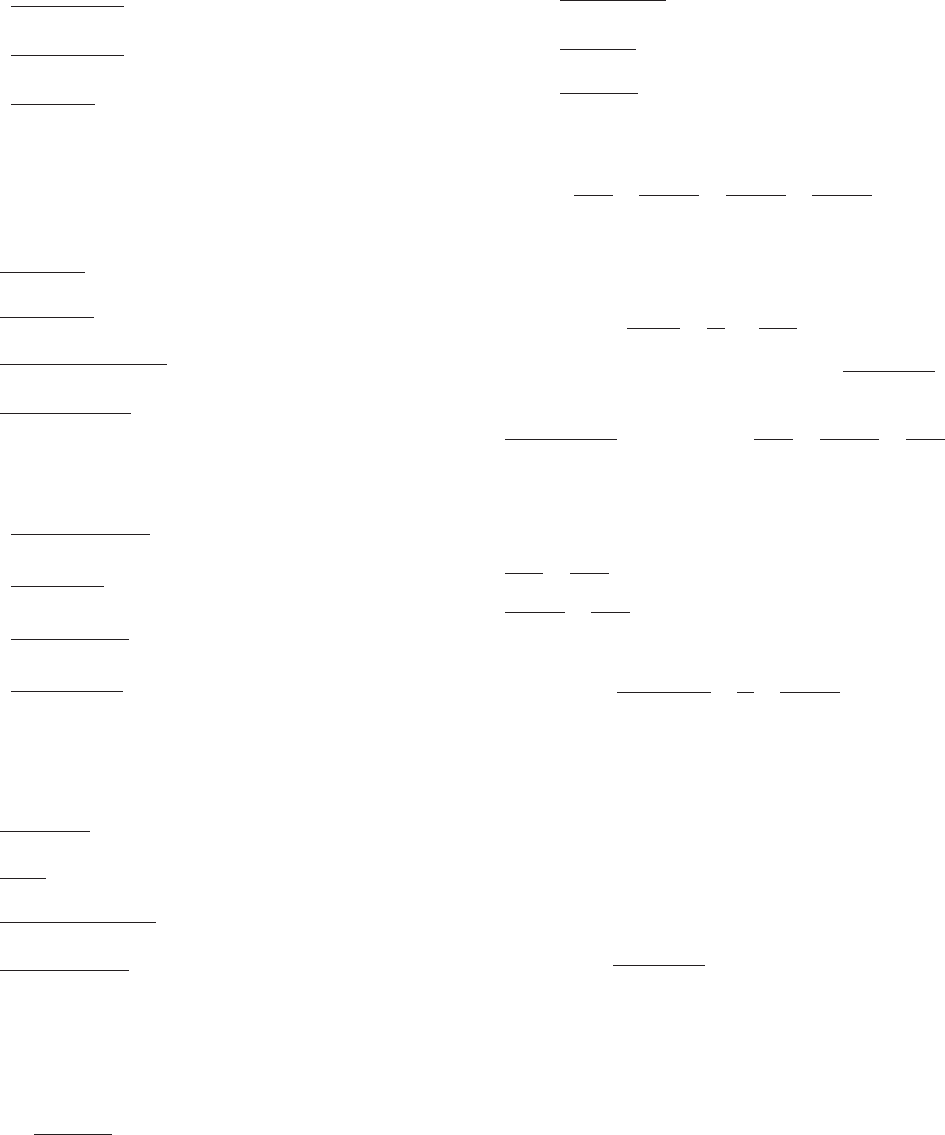
42.
Z
1
0
1
ðx 1 1Þð2x 1 1Þ
dx
43.
Z
2
1
2x
2
1 5x 1 4
xðx 1 1Þðx 1 2Þ
dx
44.
Z
2
1
x
2
2 2x 2 2
x
2
ðx 1 1Þ
dx
Further Theory and Practice
c Calculate each of the indefinite integrals in Exercises
45248. b
45.
Z
4x
2
1 x 1 2
x
3
ðx 1 2Þ
dx
46.
Z
3x
2
1 5x 1 3
x
3
ðx 1 1Þ
dx
47.
Z
3x
3
2 16x
2
1 26x 2 14
ðx 2 1Þ
2
ðx 2 2Þ
2
dx
48.
Z
5x
3
1 5x
2
2 x 2 2
ðx
2
1 xÞ
2
dx
c Calculate each of the definite integrals in Exercises
49252. b
49.
Z
2
1
2x
3
1 6x
2
1 9x 1 2
xðx 1 1Þ
3
dx
50.
Z
3
2
x
2
1 10x 1 1
ðx
2
2 1Þ
2
dx
51.
Z
1
0
3x
2
1 8x 1 6
ðx 1 1Þ
2
ðx 1 2Þ
dx
52.
Z
9
4
64x
ðx 1 1Þðx 2 3Þ
3
dx
c In each of Exercises 53256, make a substitution before
applying
the method of partial fractions to calculate the given
integral. b
53.
Z
expðxÞ
expð2xÞ2 1
dx
54.
Z
2
x12
4
x
2 4
dx
55.
Z
cosðxÞ
sin
2
ðxÞ2 5sinðxÞ1 6
dx
56.
Z
2lnðxÞ1 5
x ln
2
xðÞ1 ln xðÞ
dx
c In each of Exercises 57261, two functions f and g are
given.
Calculate
R
f ðxÞdx by first making the substitution
u 5 g (x) and then applying the method of partial fractions. b
57. f ðxÞ5 1=ðx
3=2
2 xÞ; gðxÞ5 x
1=2
58. f ðxÞ5
1
x
4=3
2 x
2=3
; gðxÞ5 x
1=3
59. f ðxÞ5
1
ffiffiffiffiffiffiffiffiffiffiffi
x 1 2
p
ðx 1 1Þ
; gðxÞ5
ffiffiffiffiffiffiffiffiffiffiffi
x 1 2
p
60. f ðxÞ5
ffiffiffi
x
p
1 2
ffiffiffi
x
p
ðx 2 1Þ
; gðxÞ5
ffiffiffi
x
p
61. f ðxÞ5
1
x
1=2
2 x
1=6
; gðxÞ5 x
1=6
62. What happens if you attempt a partial fraction decom-
position of 1/(x 2 3)
4
into
A
x 2 3
1
B
ðx 2 3Þ
2
1
C
ðx 2 3Þ
3
1
D
ðx 2 3Þ
4
?
(A long calculation should be your last resort.)
63. Assume that a is a positive constant and that jxj, a. Use
the method of partial fractions to derive the formula
Z
dx
a
2
2 x
2
5
1
2a
ln
a 1 x
a 2 x
1 C:
64. Find the partial fraction decomposition of
1
x
3
2 3x
2
1 2
:
The correct partial fraction decomposition of
5x
2
1 18x 2 1
ðx 1 4Þ
2
ðx 2 3Þ
is of the form
A
1
x 1 4
1
A
2
ðx 1 4Þ
2
1
B
x 2 3
:
The values of the coefficients A
1
, A
2
, and B are deter-
mined in Example 6. In Exercises 65 and 66, show that the
given incorrect form does not admit a solution for the
unknown coefficients.
65.
A
1
x 1 4
1
B
x 2 3
66.
A
2
ðx 1 4Þ
2
1
B
x 2 3
67. Mr. Woodman set up a partial fraction decomposition
5x
2
2 4x 1 2
x
2
ðx 2 1Þ
2
5
A
x
2
1
B
ðx 2 1Þ
2
and correctly solved A 5 2, B 5 3.
a. What is wrong with his method of solution?
b. Why did the right answer result even though the
method of solution was incorrect?
c. Show that if the numerator is 5x
2
2 4x 1 1insteadof
5x
2
2 4x 1 2, then the procedure used will fail. What is
the correct partial fraction decomposition in this case?
Calculator/Computer Exercises
68. Calculate
Z
5
3
x 1 3
x
3
2 3x
2
1 3
dx by finding a partial fraction
decomposition of the integrand. (Find the roots of the
denominator of the integrand numerically. Then use
Heaviside’s method to determine the coefficients of the
partial fraction decomposition.)
69. Let q(x) 5 (x 1 2)(x 1 3)(x 1 5). Find the area of the
region in the first quadrant that is bounded on the left
by the y-axis, above by y 5 (2x
2
1 5)/q(x), and below y 5
(x
3
1 2)/q(x), and below by y 5 (x
3
1 2)/q(x).
6.4 Partial Fractions—Linear Factors 505

70. Find the area of the region that is bounded above by the
graph of y 5 ln(x) and below by the graph of y 5 x
3
/
(x
2
1 3x 1 2).
71. Let f(x) 5 4 1 2x 2 x
2
, let gðxÞ5
2x
3
1 x 1 2
x
4
2 5x
3
1 6x 2 1
; and
let a be the solution of f(x) 5 g(x) in [0.25, 0.5]. Use a
partial fraction decomposition to find the area of the
region bounded above by the graph of y 5 f ( x), a # x # 1
and below by the graph of y 5 g(x), a # x # 1.
c Computer algebra systems can calculate the partial frac-
tion
decomposition of a rational function when the arithmetic
can be done with rational numbers. For example, the Maple
command for obtaining the partial fraction decomposition of
a rational expression R in the variable x is
convertðR; parfrac; xÞ;
In Exercises 72275, use a computer algebra system to find the
partial fraction decomposition of the given rational
functions. b
72.
x
3
2 9x
2
1 25x 2 19
x
4
2 5x
3
1 6x
2
1 4x 2 8
73.
x
3
1 10x
2
1 27x 1 24
x
4
1 10x
3
1 35x
2
1 50x 1 24
74.
x
5
1 2x
4
2 10x
3
2 7x
2
1 9x 2 24
x
4
1 2x
3
2 11x
2
2 12x 1 36
75.
3x
4
1 12x
3
1 17x
2
1 14x 1 2
x
6
1 2x
5
2 x
4
2 4x
3
2 x
2
1 2x 1 1
6.5 Partial Fractions—Irreducible Quadratic Factors
In Section 6.4, you learned how to evaluate integrals of the form
Z
pðxÞ
ðx 2 a
1
Þ
m
1
ðx 2 a
2
Þ
m
2
ðx 2 a
K
Þ
m
K
dx; ð6:5:1Þ
where p(x) is a polynomial. To do so, we decomposed the integrand into a sum of
simpler expressions using the method of partial fractions. The purpose of this
section is to extend that technique to denominators that include irreducible
quadratic factors. Because the Fundamental Theorem of Algebra tells us that any
polynomial can be split into a product of linear and irreducible quadratic factors,
our study of the method of partial fractions will therefore be complete. As a result,
we will be able to integrate any rational function p(x)/q(x).
Rational Functions with
Quadratic Terms in the
Denominator
In evaluating integrals of the form (6.5.1), we have made use of two simple alge-
braic expressions: the simple linear building block A/(x 2 a) and the repeated linear
building block A/(x 2 a)
m
. To find a partial fraction decomposition of the rational
function p(x)/q(x) when q(x) has one or more irreducible quadratic factors, we
need two new building blocks.
Simple Quadratic Building Blocks
Bx 1 C
ax
2
1 bx 1 c
; ð6:5:2Þ
assuming that the denominator ax
2
1 bx 1 c cannot be factored; otherwise, we would be in the situation of linear
building blocks that we studi ed in Section 6.4. Recall that we learned how to integrate expressions of the form
(6.5.2) in Section 6.3.
Repeated Quadratic Building Blocks
Bx 1 C
ðax
2
1 bx 1 cÞ
n
;
where n is an integer greater than 1. Integrands of this form have been treated in Section 6.3. Because n .1we
will have to make certain adjustments in the partial fractions representation.
506 Chapter 6 Techniques of Integration
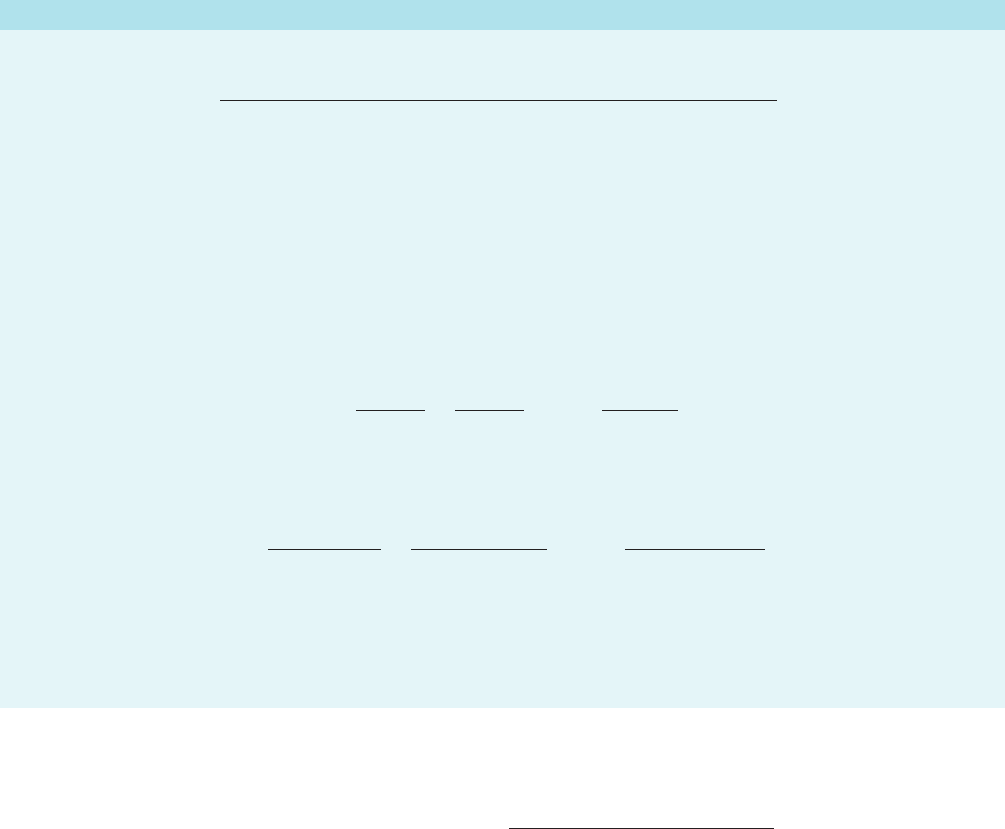
We can now state our complete partial fractions rule. You will find that this
rule just builds upon steps that you are already familiar with: The presence of
irreducible quadratic terms in the denominator does not change the way we treat
the linear terms.
The Method of Partial Fractions
To integrate a rational function of the form
pðxÞ
ðx 2 a
1
Þ
m
1
ðx 2 a
K
Þ
m
K
ða
1
x
2
1 b
1
x 1 c
1
Þ
n
1
ða
L
x
2
1 b
L
x 1 c
L
Þ
n
L
;
where the factors x 2 a
1
, x 2 a
2
,...,x 2 a
K
, a
1
x
2
1 b
1
x 1 c
1
, a
2
x
2
1 b
2
x 1 c
2
, ...,a
L
x
2
1 b
L
x 1 c
L
are all distinct,
perform the follow ing four steps:
1. Be sure that the degree of the numerator p is less than the degree of the denominator; if it is not, then divide
the denominator into the numerator.
2. Be sure that the quadratic factors a
j
x
2
1 b
j
x 1 c
j
cannot be factored into linear factors with real coefficients.
(Do this by verifying that b
j
2
2 4a
j
c
j
, 0; if b
j
2
2 4a
j
c
j
$ 0 for any quadratic term a
j
x
2
1 b
j
x 1 c
j
, then that
expression must be factored into a product of two linear terms.)
3. For each of the factors (x 2 a
j
)
m
j
in the denominator of the rational function being considered, the partial
fraction decomposition must contain terms of the form
A
1
ðx 2 a
j
Þ
1
1
A
2
ðx 2 a
j
Þ
2
1 1
A
m
j
ðx 2 a
j
Þ
m
j
:
4. For each of the factors (x
2
1b
j
x1c
j
)
n
j
in the denominator of the integrand being considered, the partial
fraction decomposition must contain terms
B
1
x 1 C
1
a
j
x
2
1 b
j
x 1 c
j
1
B
2
x 1 C
2
ða
j
x
2
1 b
j
x 1 c
j
Þ
2
1 1
B
n
j
x 1 C
n
j
ða
j
x
2
1 b
j
x 1 c
j
Þ
n
j
:
The first three examples will give you a more concrete idea of how to set up decompositions into partial
fractions (without the distraction of determining the unknown coefficients or performing subsequent
integrations).
⁄ EX
AMPLE 1 State the form of the partial fraction decomposition of
8x
9
ðx 1 4Þðx 1 5Þ
3
ðx
2
1 6Þðx
2
1 7Þ
2
:
Solution The
degree of the denominator is 10. Because the degree of the
numerator is less than 10, division is not needed . The factors of the denominator
include a nonrepeated linear term, x 1 4, and a repeated linear term, (x 1 5)
3
.Ina
partial frac tion decomposition, the numerators of the summan ds that correspond to
such factors are always constants. Among the factors of the denominator of the
given rational function, we also find a nonrepeated irreducible quadratic, x
2
1 6,
and a repeated irreducible quadratic, (x
2
1 7)
2
. In a partial fraction decomposition,
6.5 Partial Fractions—Irreducible Quadratic Factors 507

the numerators of the summands that correspond to such factors are always linear
polynomials. Thus
8x
9
ðx 1 4Þðx 1 5Þ
3
ðx
2
1 6Þðx
2
1 7Þ
2
5
A
x 1 4
1
B
x 1 5
1
C
ðx 1 5Þ
2
1
D
ðx 1 5Þ
3
1
Ex 1 F
x
2
1 6
1
Gx 1 H
x
2
1 7
1
Ix 1 J
ðx
2
1 7Þ
2
:
Notice that the number of unknown factors in the numerators is 10, which is the same
as the degree of the denominator polynomial in the original rational function.
¥
Checking for
Irreducibility
It is very important to begin with the right form of a partial fraction decomposition
before attempting to solve for the unknown coefficients. Usually, it is impossible to
solve for the unknown coefficients when the partial fraction decomposition has not
been set up correctly.
⁄ EX
AMPLE 2 Find the correct form of the partial fraction decomposition
for the rational expression
3x
2
1 11x 1 10
ðx 1 1Þðx
2
1 4x 1 3Þ
:
Solution Because
the degree of the numerator is 2 and the degree of the
denominator is 3, a preliminary divi sion is not necessary. Notice that the correct
partial fraction decomposition is not
3x
2
1 11x 1 10
ðx 1 1Þðx
2
1 4x 1 3Þ
5
A
x 1 1
1
Bx 1 C
x
2
1 4x 1 3
: ð6:5:3Þ
The reason is that we have not factored the denominator as far as possible. The
quadratic polynomial x
2
1 4x 1 3 factors as
x
2
1 4x 1 3 5 ðx 1 1Þðx 1 3 Þ:
Thus the given rational function really falls under the case of distinct linear factors,
which we studied in Section 6.4. The correct form of the partial fraction decom-
position is
3x
2
1 11x 1 10
ðx 1 1Þðx
2
1 4x 1 3Þ
5
3x
2
1 11x 1 10
ðx 1 1Þ
2
ðx 1 3Þ
5
A
x 1 1
1
B
ðx 1 1Þ
2
1
C
x 1 3
: ð6:5:4Þ
You may want to practice the methods of determining the unknown coefficients
that we studied in Section 6.4. You will find that the values A 5 2, B 5 1, and C 5 1
turn equation (6.5.4) into an identity in x. By contrast, there are no values of A, B,
and C for which equation (6.5.3) is an identity in x.
¥
INSIGHT
Remember that the Quadratic Formula tells us whether a quadratic factor
ax
2
1 bx 1 c is irreducible or not. If the discriminant b
2
2 4ac is negative, then the
polynomial is irreducible; otherwise, it can be factored into linear terms. A quick
calculation of the discriminant of x
2
1 4x 1 3 in Example 2 would result in the positive
number 4
2
2 4 1 3 5 4. That would alert us to the reducibility of x
2
1 4x 1 3.
⁄ EXAMPLE 3 Find the correct form of the partial fraction decomposition
of 3/(x
3
1 1).
Solution This
expression may appear to have an irreducible denominator, but it
does not. Cubic (and higher-degre e) polynomials are never irreducible. Notice
508 Chapter 6 Techniques of Integration
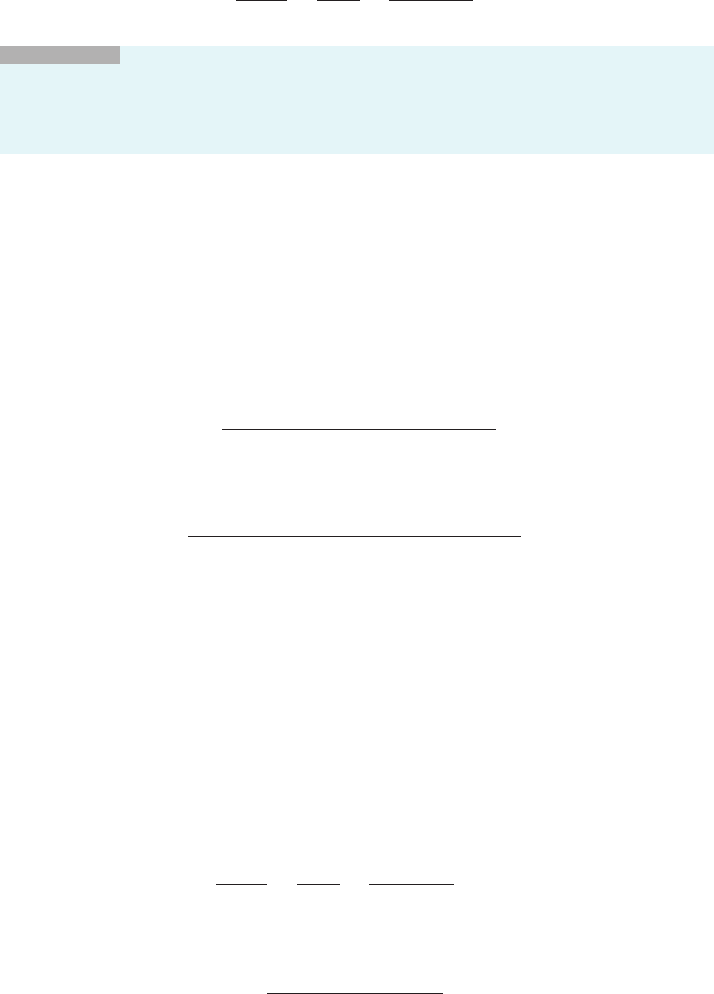
that 21 is a root of the polynomial x
3
1 1. Therefore (x 1 1) is a divisor. After
performing the polynomial division, we find that
x
3
1 1 5 ðx 1 1Þðx
2
2 x 1 1Þ:
The quadratic factor has negative discriminant (21)
2
2 4 1 1 523, so it is
irreducible. Thus we see that the correct partial fraction decomposition for our
rational expression is
3
x
3
1 1
5
A
x 1 1
1
Bx 1 C
x
2
2 x 1 1
: ð6:5:5Þ ¥
INSIGHT
It is important for you to realize that the Fundamental Theorem of
Algebra guarantees that any real polynomial can be factored into linear and quadratic
factors. In the theory of partial fractions, we never have to use building blocks that
involve irreducible cubic, quartic, or higher order factors—they do not exist.
Calculating the
Coefficients of a
Partial Fraction
Decomposition
Now that we have considered the forms that decompositions into partial fracti ons
can take, let us see how the unknown coefficients can be computed in practice.
⁄ EX
AMPLE 4 Find the partial fraction decomposition of the rational
function 3/(x
3
1 1).
Solution In
equation (6.5.5) of Example 3, we determined the correct form of the
partial fraction decomposition of 3/(x
3
1 1). The right side of equation (6.5.5) can
be written as
Aðx
2
2 x 1 1Þ1 ðBx 1 CÞðx 1 1Þ
ðx 1 1Þðx
2
2 x 1 1Þ
or, after expanding both the numerator and denominator,
ðA 1 BÞx
2
1 ð2A 1 B 1 CÞx 1 ðA 1 CÞ
x
3
1 1
:
The numerator of this expression must eq ual the numerator of the left side of
(6.5.5):
0x
2
1 0x 1 3 5 ðA 1 BÞx
2
1 ð2A 1 B 1 CÞx 1 ðA 1 CÞ:
Equating the coefficients of like powers of x on each side of this equation leads to
the simultaneous equations
A 1 B 5 0; 2A 1 B 1 C 5 0; A 1 C 5 3:
The first equation allows us to replace B with 2A in the second equation to
obtain 22A 1 C 5 0. Thus C 5 2A. The third equation then tells us that A 1 (2A) 5
3orA 5 1. Consequently, B 52A 521andC 5 2A 5 2. In conclusion, the partial
fraction decomposition is
3
x
3
1 1
5
1
x 1 1
1
2x 1 2
x
2
2 x 1 1
: ¥
⁄ EX
AMPLE 5 Calculate
Z
x
3
2 x
2
2 3x 1 3
ðx
2
1 4x 1 5Þðx
2
1 9Þ
dx:
6.5 Partial Fractions—Irreducible Quadratic Factors 509
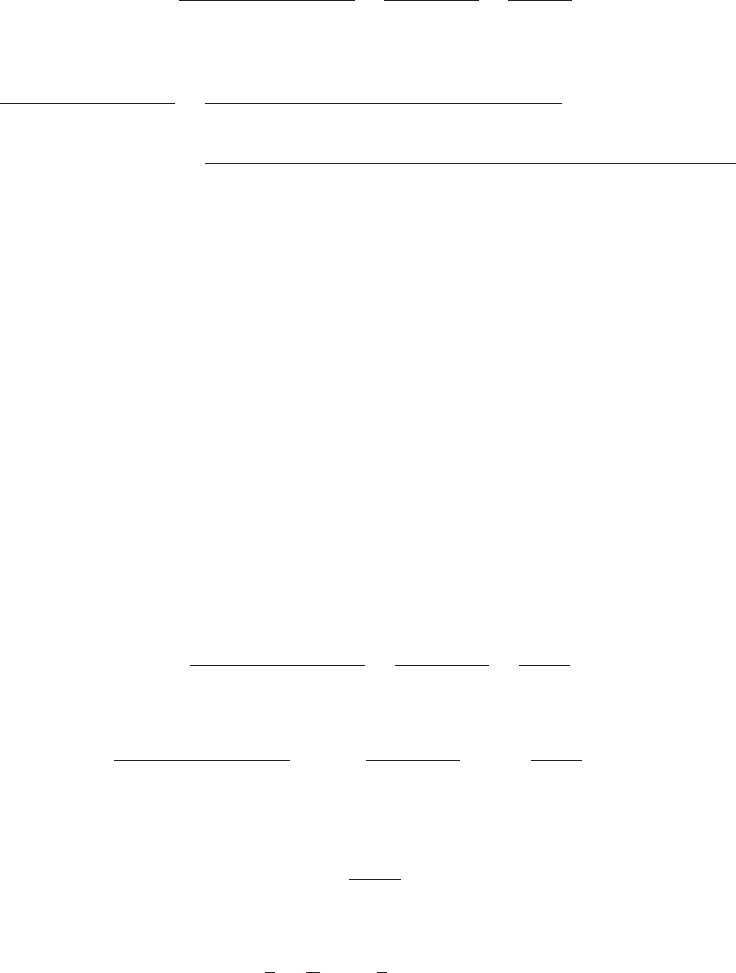
Solution The degree of the denominator is 4, one greater than the degree of the
numerator. Division is therefore not necessary. Because the discriminants of x
2
1 4x 1 5
and x
2
1 9 are negative numbers, 4
2
2 4 1 5 524and0
2
2 4 1 9 5236,
respectively, we can be sure that further factoring of the denominator is impossible.
We may therefore proceed directly to the partial fraction decomposition:
x
3
2 x
2
2 3x 1 3
ðx
2
1 4x 1 5Þðx
2
1 9Þ
5
Ax 1 B
x
2
1 4x 1 5
1
Cx 1 D
x
2
1 9
:
We combine the two summands on the right to obtain
x
3
2 x
2
2 3x 1 3
ðx
2
1 4x 1 5Þðx
2
1 9Þ
5
ðAx 1 BÞðx
2
1 9Þ1 ðCx 1 DÞðx
2
1 4x 1 5Þ
ðx
2
1 4x 1 5Þðx
2
1 9Þ
5
ðA 1 CÞx
3
1 ðB 1 4C 1 DÞx
2
1 ð9A 1 5C 1 4DÞx 1 ð9B 1 5DÞ
ðx
2
1 4x 1 5Þðx
2
1 9Þ
:
We may now equate the numerators on the left with the numerator on the right:
x
3
2 x
2
2 3x 1 3 5 ðA 1 CÞx
3
1 ðB 1 4C 1 DÞx
2
1 ð9A 1 5C 1 4DÞx 1 ð9B 1 5 DÞ:
By equating coefficients of like powers of x, we obtain the four equations
A 1 C 5 1; B 1 4C 1 D 521; 9A 1 5C 1 4D 523; 9B 1 5D 5 3:
We may use the first and second of these equations to solve for A and B in terms of
C and D. We obtain A 5 1 2 C and B 521 2 4C 2 D. By us ing these expressions in
C and D to replace A and B in the third and fourth equations, we obtain two
equations in the two unknowns C and D. Thus we have
9ð1 2 CÞ1 5C 1 4D 523; or 2 4C 1 4D 5212
an
d
9ð21 2 4C 2 DÞ1 5D 5 3; or 236C 2 4D 5 12 :
We solve these last two equations in C and D, obtaining C 5 0 and D 523. It
follows that A 5 1 2 C 5 1 2 0 5 1 and B 521 2 4C 2 D 521 2 4(0) 2 (23) 5 2.
Thus
x
3
2 x
2
2 3x 1 3
ðx
2
1 4x 1 5Þðx
2
1 9Þ
5
x 1 2
x
2
1 4x 1 5
2
3
x
2
1 9
and
Z
x
3
2 x
2
2 3x 1 3
ðx
2
1 4x 1 5Þðx
2
1 9Þ
dx 5
Z
x 1 2
x
2
1 4x 1 5
dx 2
Z
3
x
2
1 9
dx: ð6:5:6Þ
We complete the square x
2
1 4x 1 5 5 (x 1 2)
2
1 1 and make the substitution
u 5 x 1 2, du 5 dx to convert the first integral on the right side of equation (6.5.6) to
Z
u
u
2
1 1
du:
By means of the substitution w 5 u
2
1 1, dw 5 2udu, this last integral is converted to
1
2
Z
1
w
dw 5
1
2
ln ðjwjÞ1 C:
We resubstitute, observing that w 5 u
2
1 1 5 x
2
1 4x 1 5 is positive, to obtain
510 Chapter 6 Techniques of Integration
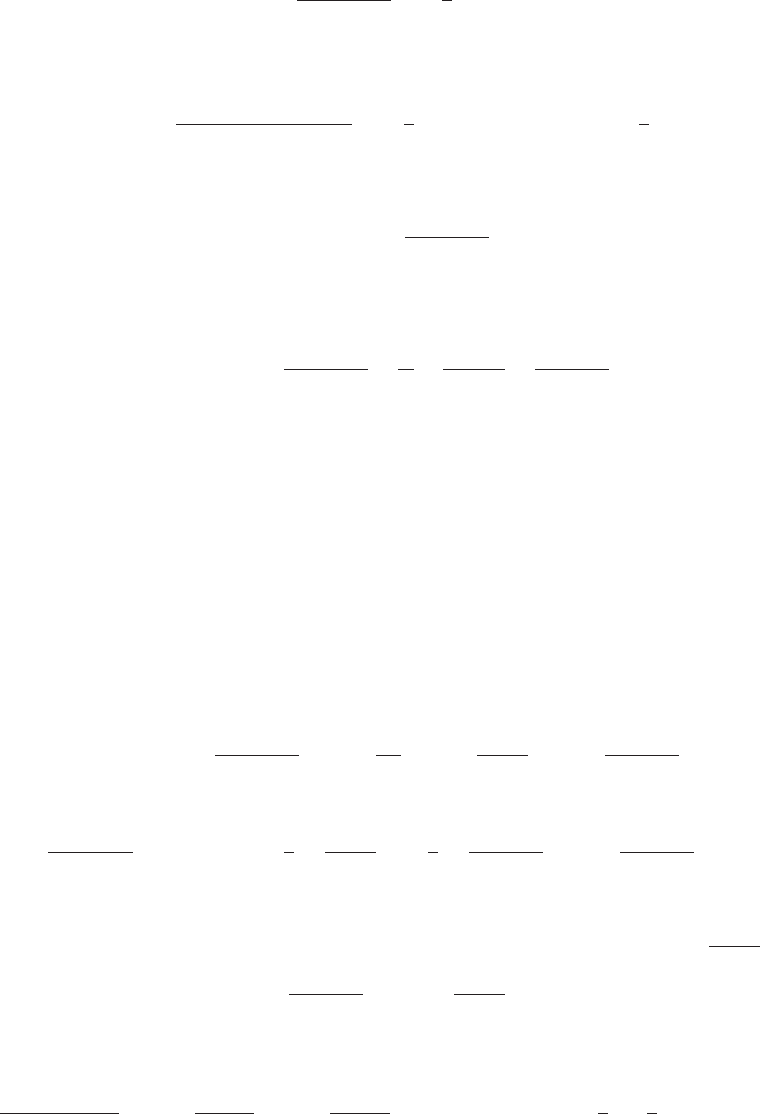
Z
x 1 2
x
2
1 4x 1 5
dx 5
1
2
ln ðx
2
1 4x 1 5Þ1 C:
Finally, we use formula (6.3.2) to see that
R
3=ðx
2
1 9Þdx 5 arctanðx=3Þ1 C. After
substituting for the two integrals on the right side of equation (6.5.6), we have
Z
x
3
2 x
2
2 3x 1 3
ðx
2
1 4x 1 5Þðx
2
1 9Þ
dx 5
1
2
lnðx
2
1 4x 1 5Þ2 arctan
x
3
1 C: ¥
⁄ EX
AMPLE 6 Calculate the integral
Z
x 2 1
xðx
2
1 1Þ
2
dx:
Solution The
degree of the numerator is less than that of the denominator, so we
proceed directly to the partial fraction decomposition:
x 2 1
xðx
2
1 1Þ
2
5
A
x
1
Bx 1 C
x
2
1 1
1
Dx 1 E
ðx
2
1 1Þ
2
:
Putting the right side over a common denominator leads to
x 2 1 5 Aðx
2
1 1Þ
2
1 ðBx 1 CÞðx
2
1 1Þx 1 ðDx 1 EÞx
5 Aðx
4
1 2x
2
1 1Þ1 ðBx 1 CÞðx
3
1 xÞ1 Dx
2
1 Ex
5 x
4
ðA 1 B Þ1 x
3
ðCÞ1 x
2
ð2A 1 B 1 DÞ1 xðC 1 EÞ1 A:
Equating like coefficients of x leads to the system
A 1 B 5 0
C 5 0
2A 1 B 1 D 5 0
C 1 E 5 1
A 521.
We conclude that A 521, B 5 1, C 5 0, D 5 1, E 5 1. Therefore
Z
x 2 1
xðx
2
1 1Þ
2
dx 5
Z
21
x
dx 1
Z
x
x
2
1 1
dx 1
Z
x 1 1
ðx
2
1 1Þ
2
dx;
or
Z
x 2 1
xðx
2
1 1Þ
2
dx 52lnðjxjÞ1
1
2
Z
2x
x
2
1 1
dx 1
1
2
Z
2x
ðx
2
1 1Þ
2
dx 1
Z
1
ðx
2
1 1Þ
2
dx: ð6:5:7Þ
The first and second integrals on the right side are handled with the substitution
u 5 x
2
1 1, du 5 2xdx. The results of these integrations are
Z
2x
x
2
1 1
dx 5
lnðx
2
1 1Þ1 C and
Z
2x
ðx
2
1 1Þ
2
dx 5 C 2
1
x
2
1 1
: For the last integral on the right
side of equation (6.5.7), we make the substitution x 5 tan (θ), dx 5 sec
2
(θ)dθ. This
substitution converts the integral into
Z
sec
2
ðθÞ
tan
2
ðθÞ1 1
2
dθ 5
Z
sec
2
ðθÞ
sec
4
ðθÞ
dθ 5
Z
1
sec
2
ðθÞ
dθ 5
Z
cos
2
ðθÞdθ 5
ð6:2:6Þ
1
2
θ 1
1
2
sinðθÞcosðθÞ1 C:
6.5 Partial Fractions—Irreducible Quadratic Factors 511
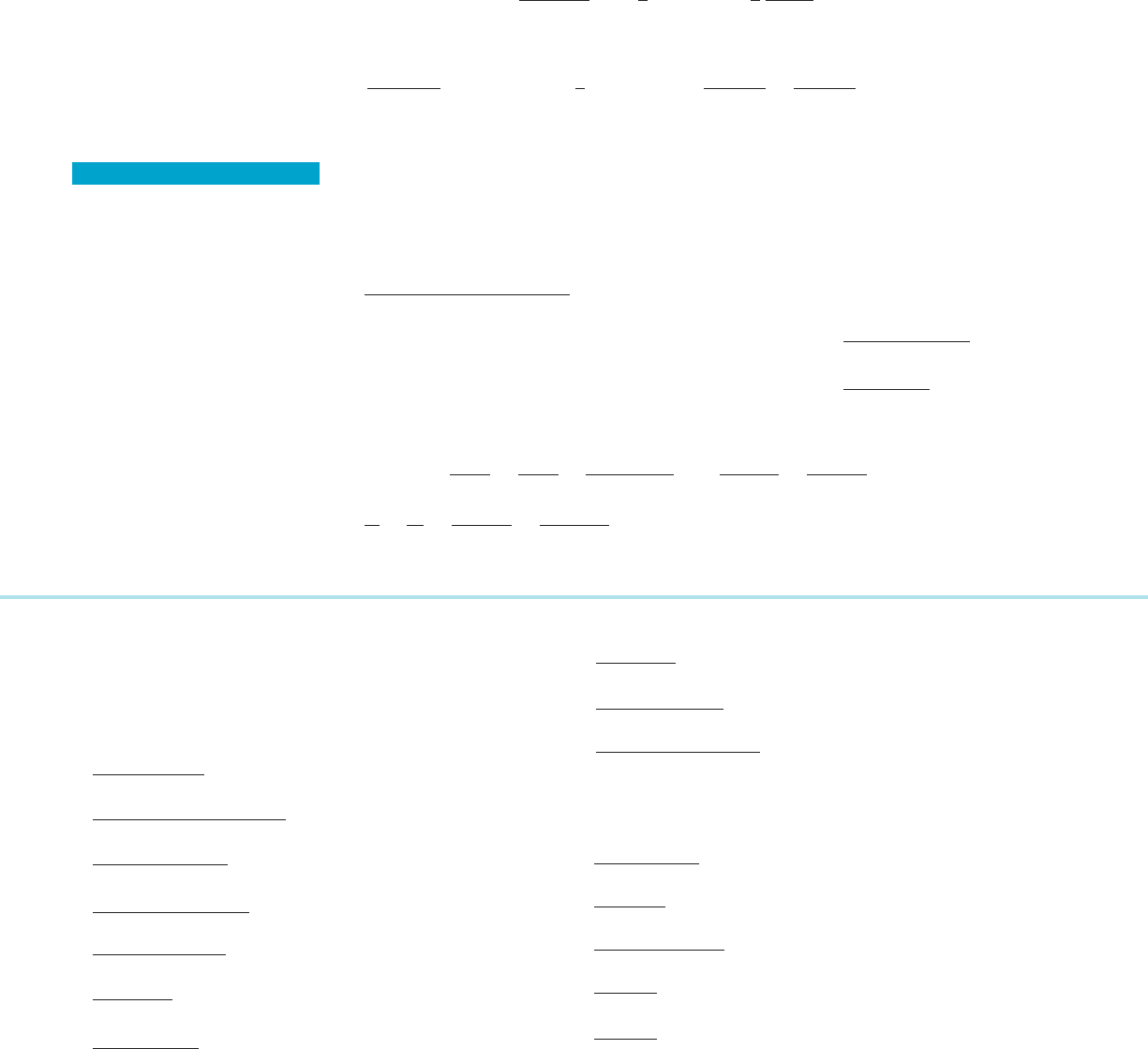
After resubstituting for x, we have
Z
1
ðx
2
1 1Þ
2
dx 5
1
2
arctanðxÞ1
1
2
x
x
2
1 1
1 C:
Assembling all the pieces, we conclude that our original integral calculat es to
Z
x 2 1
xðx
2
11Þ
2
dx 52lnðjxjÞ1
1
2
lnðx
2
1 1Þ2
1
ðx
2
1 1Þ
1
x
ðx
2
1 1Þ
1 arctanðxÞ
1 C:
QUICK QUIZ
1. True or false: If q(x) is a polynomial of degree n that factors into irreducible
quadratic terms and if p(x) is a polynomial of degree m with m , n, then an
explicitly calculated partial fraction decomposition of p(x)/q(x) requires the
determination of n unknown constants.
2. What is the form of the partial fraction decomposition of
x
2
ðx
2
2 3x 2 4Þðx
2
2 3x 1 4Þ
?
3. What is the form of the partial fraction decomposition of
2x
2
1 1
ðx
2
1 1Þðx
2
1 4Þ
?
4. What is the form of the partial fraction decomposition of
2x
2
1 1
x
2
ðx
2
1 4Þ
2
?
Answers
1. True 2.
A
x 1 1
1
B
x 2 4
1
Cx 1 D
x
2
2 3x 1 4
3.
Ax 1 B
x
2
1 1
1
Cx 1 D
x
2
1 4
4.
A
x
1
B
x
2
1
Cx 1 D
x
2
1 4
1
Ex 1 F
ðx
2
1 4Þ
2
EXERCISES
Problems for Practice
c In each of Exercises 1210, write down the form of the
partial fraction decomposition of the given rational function.
Do not explicitly calculate the coefficients. b
1.
2x
3
1 x 1 1
ðx
2
1 1Þðx
2
1 4Þ
2.
2x
4
ðx
2
1 2x 1 2Þð2x
2
1 5x 1 3Þ
3.
2x
3
1 x 1 1
ðx
2
1 1Þðx
2
1x11Þ
2
4.
2x
4
ðx
2
1 1Þð5x
2
1 4x 1 1Þ
5.
2x 1 1
ðx
2
1 x 1 3Þðx 2 4Þ
6.
3x
3
1 x 1 1
x
2
ðx11Þ
2
7.
2x
6
ðx
2
14Þ
3
ðx 2 2Þ
8.
3x
5
1 x 1 1
x
2
ðx
2
2 1Þ
2
9.
3x
5
1 x 1 1
x
2
ðx
2
2 1Þðx
2
1 1Þ
10.
7x
8
ðx 2 3Þ
3
ðx
2
1 6x 1 10Þ
3
c In each of Exercises 11220, explicitly calculate the partial
fraction decomposition of the given rational function. b
11.
3x
2
2 5x 1 4
ðx 2 1Þðx
2
1 1Þ
12.
x
2
1 2
ðx
2
1 1Þx
2
13.
7x
3
2 3x
2
1 9x 2 6
ðx
2
1 2Þðx
2
1 1Þ
14.
x
2
1 2x
ðx
2
1 1Þ
2
15.
x
3
2 x
ðx
2
1 1Þ
2
512 Chapter 6 Techniques of Integration
16.
2x
2
ðx 1 1Þ
2
ðx
2
1 1Þ
17.
x
3
1 12x
2
2 9x 1 48
ðx 2 3Þðx
2
1 4Þ
18.
2x
2
1 4x 1 2
ðx
2
11Þ
3
19.
3x
3
2 5x
2
1 10x 2 19
ðx
2
1 4Þðx
2
1 3Þ
20.
2x
4
1 15x
2
1 30
ðx
2
1 4Þðx
2
1 3Þ
2
c In each of Exercises 21230, use the method of partial
fractions to decompose the integrand. Then evaluate the
given integral. b
21.
Z
3x
2
2 5x 1 4
ðx 2 1Þðx
2
1 1Þ
dx
22.
Z
x
2
1 2
ðx
2
1 1Þx
2
dx
23.
Z
7x
3
1 9x 2 3x
2
2 6
ðx
2
1 2Þðx
2
1 1Þ
dx
24.
Z
x
2
1 2x
ðx
2
1 1Þ
2
dx
25.
Z
x
3
2 x
ðx
2
1 1Þ
2
dx
26.
Z
2x
2
ðx 1 1Þ
2
ðx
2
1 1Þ
dx
27.
Z
x
3
1 12x
2
2 9x 1 48
ðx 2 3Þðx
2
1 4Þ
dx
28.
Z
2x
2
1 4x 1 2
ðx
2
1 1Þ
3
dx
29.
Z
3x
3
2 5x
2
1 10x 2 19
ðx
2
1 4Þðx
2
1 3Þ
dx
30.
Z
2x
4
1 15x
2
1 30
ðx
2
1 4Þðx
2
1 3Þ
2
dx
c In each of Exercises 31238, evaluate the given definite
integral. b
31.
Z
1
0
x
2
1 3
ðx
2
1 1Þðx
2
1 2Þ
dx
32.
Z
3
2
2x
3
2 x
2
1 2x 1 1
ðx
2
2 1Þðx
2
1 1Þ
dx
33.
Z
1
0
7x
2
1 4x 1 6
ðx 1 1Þðx
2
1 2Þ
dx
34.
Z
2
1
3x
4
1 8x
2
1 3
xðx
2
11Þ
2
dx
35.
Z
2
1
2x
2
2 x 1 2
xðx
2
1 1Þ
dx
36.
Z
2
1
7x
2
1 6
x
2
ðx
2
1 1Þ
dx
37.
Z
1
0
x
2
1 x 1 1
ðx
2
1 1Þ
2
dx
38.
Z
1
0
x
3
1 x 1 1
ðx
2
1 1Þ
2
dx
Further Theory and Practice
c In Exercises 39244, calculate the given integral. b
39.
Z
3x
2
1 6x 1 4
ðx 1 1Þðx
2
1 2x 1 2Þ
dx
40.
Z
4x
2
1 5x 1 3
ðx 1 1Þðx
2
1 x 1 1Þ
dx
41.
Z
2x
2
1 4x 1 9
x
3
2 1
dx
42.
Z
5x
2
2 2x 1 2
x
3
1 1
dx
43.
Z
8x
x
5
2 x
4
2 x 1 1
dx
44.
Z
48
ðx
2
1 1Þ
4
dx
45. Show that equation (6.5.3) is not an identity in x for any
values of A, B, and C.
46. What happens if you attempt a partial fraction decom-
position of 1/(x
2
1 3)
4
into
A
1
x 1 B
1
x
2
1 3
1
A
2
x 1 B
2
ðx
2
1 3Þ
2
1
A
3
x 1 B
3
ðx
2
1 3Þ
3
1
A
4
x 1 B
4
ðx
2
1 3Þ
4
?
(Brute force calculation should be a last resort.)
Calculator/Computer Exercises
47. Let b be the abscissa of the point of intersection of the
graphs of y 5 x
4
1 2 and y 5
5x
3
1 3x 1 2
x
3
1 x
2
1 x 1 1
for 0 , x , 1.
Calculate the area of the region between the two curves
for 0 # x # b.
48. Let b be the abscissa of the first-quadrant point of inter-
section of the graphs of y 5 x
2
and y 5
3x
3
1 4x
x
4
1 3x
2
1 2
.
Calculate the area of the region between the two curves
for 0 # x # b.
49. Find the area of the region in the first quadrant that is
bounded above by y 5 1 2 x
2
and below by
y 5
3x
2
1 4x 1 5
x
3
1 3x
2
1 7x 1 5
:
50. Find the four points of intersection of the curves
y 5 1 2 x
2
/16 and y 5
x
3
1 x
2
1 x 1 2
x
4
1 3x
2
1 2
. Let a be the least
abscissa of these points and b the greatest. Calculate the
total area of the regions that are bounded above by one of
the curves and below by the other for a # x # b.
6.5 Partial Fractions—Irreducible Quadratic Factors 513
6.6 Improper Integrals—Unbounded Integrands
The theory of the integral that you learned in Chapter 5 enables you to integrate a
continuous function f (x) on a closed, bounded interval [a, b]. However, often it is
necessary to integrate an unbounded function, or a function that is defined on an
unbounded interval. In this section and the next, you learn to do so.
Integrals with Infinite
Integrands
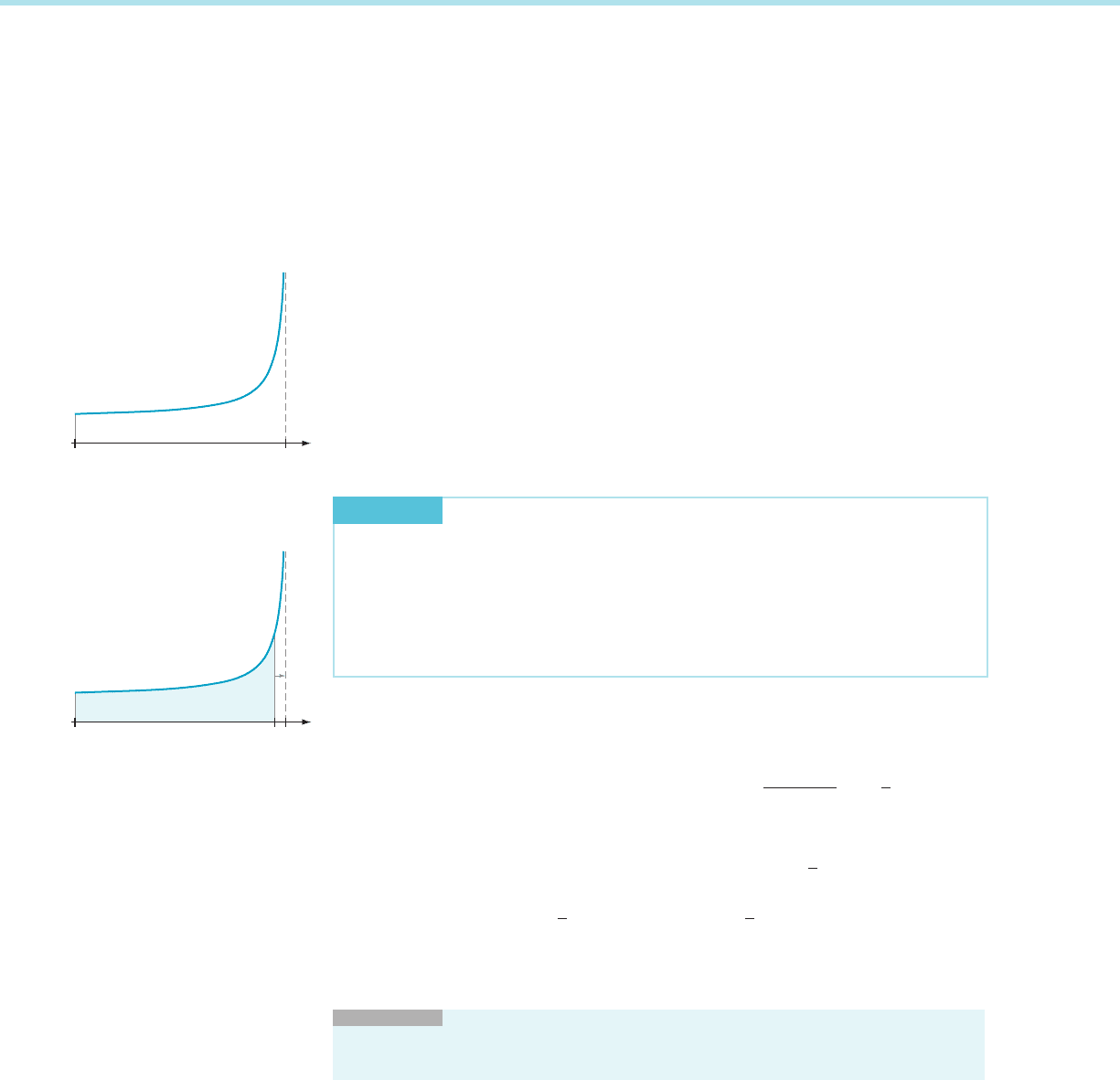
Let f be a continuous function on the interval [a, b). Suppose that f is unbounded
as x approaches b from the left side (see Figure 1). It can be shown that the
Riemann sums of an unboun ded function do not have a limit as the order of the
uniform partition tends to infinity. In other words, f does not have a Riemann
integral over the interval [a, b]. We say that
R
b
a
f ðxÞdx is an improper integral with
infinite integrand at b. Figure 2 illustrates the method by which we define and
evaluate this type of integral. The idea is to integrate almost up to the singularity —
within ε—and then to let ε tend to 0. Thus let ε be a small positive number. Because
f is continuous on the interval [a, b 2 ε ], we can form the Riemann integral
R
b2ε
a
f ðxÞdx. Now we let the upper limit of integration, b 2 ε, ap proach b, which is
the same thing as letting ε tend to 0. Recall that we write this limit as ε - 0
1
because ε approaches 0 through positive values. If the limit exists, then we say that
the improper integral converges. Otherwise we say it diverges.
DEFINITION
If f (x) is continuous on [a, b), and unbounded as x approaches b
from the left, then the value of the improper integral
R
b
a
f ðxÞdx is defined to be
Z
b
a
f ðxÞdx 5 lim
ε-0
1
Z
b2ε
a
f ðxÞdx; ð6:6:1Þ
provided that this limit exists and is finite. In this case, we say that the improper
integral converges. Otherwise, the integral is said to diverge.
⁄ EX
AMPLE 1 Evaluate the integral
R
8
0
ð8 2 xÞ
21=3
dx:
Solution The
given inte gral is improper with infinite integrand f (x) 5 (8 2 x)
21/3
as x approaches 8. Usin g the antiderivative FðxÞ52
ð8 2 xÞ
2=3
2=3
52
3
2
ð8 2 xÞ
2=3
of
f (x) on the interval [0, 8), we calculate
Z
8
0
ð8 2 xÞ
21=3
dx 5 lim
ε-0
1
Z
82ε
0
ð8 2 xÞ
21=3
dx 5 lim
ε-0
1
2
3
2
ð8 2 xÞ
2=3
82ε
0
52
3
2
lim
ε-0
1
ε
2=3
2 8
2=3
52
3
2
ð0 2 4Þ5 6:
We conclude that the given improper integral is convergent and its value is 6.
¥
INSIGHT
In Example 1, we calculate the antiderivative F(x)off (x) 5 (8 2 x)
21/3
and apply the Fundamental Theorem of Calculus on the closed interval [0, 8 2 ε]. We are
justified in doing so because f is continuous on that interval. However, because f is not
f
ab
m Figure 1 The line x 5 b is a
vertical a asymptote.
b e
f
ab
m Figure 2 The improper inte-
gral
R
b
a
f ðxÞdx is defined to be the
limit of the area of the shaded
region as b 2 ε approaches b.
514 Chapter 6 Techniques of Integration
