Blank B.E., Krantz S.G. Calculus: Single Variable

Подождите немного. Документ загружается.

We apply reduction formula (6.2.12) again, this time with n 5 8:

cos

ðxÞdx 5

sinðxÞcos

ðxÞ1

cos

ðxÞdx: ð6:2:20Þ

Therefore by substituting equation (6.2.20) into (6.2.19), we have

sin

ðxÞcos

ðxÞdx 5

sinðxÞcos

ðxÞþ

cos

ðxÞdx 2



sinðxÞcos

ðxÞþ

cos

ðxÞdx



sinðxÞcos

ðxÞ2

sinðxÞcos

ðxÞþ



1 2



cos

ðxÞdx

sinðxÞcos

ðxÞ2

sinðxÞcos

ðxÞþ

cos

ðxÞdx:

Instead of using reduction formula (6.2.12) three more times to evaluate

cos

ðxÞdx, we can now use the result of Example 4:

sin

ðxÞcos

ðxÞdx 5

sinðxÞcos

ðxÞ2

sinðxÞcos

ðxÞ



sinðxÞcos

ðxÞ1

sinðxÞcos

ðxÞ1

sinðxÞcosðxÞ1



1 C: ¥

Converting to Sines

and Cosines

Sometimes, when other trigonometric functions are converted to sines and cosines,

the methods that have been discussed can be brought to bear. The next exampl e

illustrates this idea.

⁄ EX

AMPLE 9 Evaluate

tan

ðxÞsec

ðxÞdx:

Solution We

have

tan

ðxÞsec

ðxÞ5

sin

ðxÞ

cos

ðxÞ

sec

ðxÞ5 cos

ðxÞ



sin

ðxÞ



sinðxÞ5 cos

ðxÞ



1 2 cos

ðxÞ



sinðxÞ:

The substitution u 5 cos(x), du 52sin(x) dx therefore converts the given integral to

ð1 2 u

du 52

ðu

2 2u

1 u

Þdu 5

1 C:

We resubstitute u 5 cos(x) to obtain

tan

ðxÞsec

ðxÞdx 5

7cos

ðxÞ

5cos

ðxÞ

3cos

ðxÞ

1 C;

tan

ðxÞsec

ðxÞdx 5

sec

ðxÞ2

sec

ðxÞ1

sec

ðxÞ1 C:

QUICK QUIZ

1. What trig onometric identity is used to evaluate

sin

ðxÞdx without using a

reduction formula?

2. Evaluate

2π

cos

ðxÞdx:

6.2 Powers and Products of Trigonometric Functions 485

3. The equation

π=2

sin

ðxÞcos

ðxÞdx 5

ðu

2 u

Þdu results from what

substitution?

4. For what value c is

sin

ðxÞdx 5 c

sin

ðxÞdx? (Do not integrate!)

Answers

1. sin

(x) 5



1 2 cos(2x)



/2 2. π 3. u 5 sin( x ) 4. 7/8

EXERCISES

Problems for Practice

c In each of Exercises 126, evaluate the given integral. b

sin

ðx=2Þdx

sin

ð4xÞdx

cos

ð2xÞdx

cos

ðx 1 π=3Þdx

tan

ðx=2Þdx



cos

ðxÞ2 sin

ðxÞ



c In each of Exercises 7216, evaluate the given indeﬁnite

integral. b

sin

ð2xÞdx

3cos

ðx 1 2Þdx

sin

ðx=3Þdx

10.

5cos

ðx=3Þdx

11.

3sin

ðx=5Þdx

12.

7sin

ðx=10Þdx

13.

5sin

ðx=3Þcos

ðx=3Þdx

14.

7sin

ð2x=3Þ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

cosð2x=3Þ

15.

cos

ðxÞ

sinðxÞ

16.

5cos

ð2xÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

sinð2xÞ

c In each of Exercises 17222, evaluate the given deﬁnite

integral. b

17.

sin

ðxÞcos

ðxÞdx

18.

π=2

sin

ðxÞcos

ðxÞdx

19.

sin

ðxÞcos

ðxÞdx

20.

π=2

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

sinðxÞ

cos

ðxÞdx

21.

π=2

15sin

ðxÞcos

ðxÞdx

22.

3π=2

5sin

ðx=3Þcos

ðx=3Þdx

23. Use a reduction formula to show that

cos

ðuÞdu 5

cos

ðuÞsinðuÞ1

cosðuÞsinðuÞ1

u 1 C:

24. Use a reduction formula to show that

sin

ðuÞdu 52

sin

ðuÞcosðuÞ2

cosðuÞsinðuÞ1

u 1 C:

c In each of Exercises 25229, use Exercise 23 or 24 to

evaluate

the given deﬁnite integral. b

25.

2π

cos

ðxÞdx

26.

cos

ðπxÞdx

27.

π=2

sin

ðxÞdx

28.

π=2

sin

ðx=2Þdx

29.

π=4

cos

ðtÞsin

ðtÞdt

c In each of Exercises 30233, use a reduction formula and

Exercise 23 or 24 to evaluate the given deﬁnite integral. b

30.

π=4

8sin

ðxÞdx

31.

cos

ðx=2Þdx

32.

π=2

cos

ðtÞsin

ðtÞdt

33.

π=3

π=6

cos

ðxÞsin

ðxÞdx

c In each of Exercises 34237, use reduction formula

(6.2.13),

formula (6.2.14), or a substitution to evaluate the

given indeﬁnite integral. b

34.

tan

ðxÞdx

35.

tan

ðxÞdx

36.

3sec

ðxÞdx

37.

sec

ðxÞtanðxÞdx

c In each of Exercises 38241, use reduction formula (6.2.13)

formula (6.2.14) to evaluate the given indeﬁnite integral. b

38.

π=4

2tan

ðxÞdx

39.

π=4

sec

ðxÞdx

40.

π=4

12tan

ðxÞdx

41.

π=3

10sec

ðxÞdx

c In each of Exercises 42245, use the identity 1 1 tan

(x) 5

sec

(x) to convert the given integral to one that involves only

486 Chapter 6 Techniques of Integration

tan(x) or only sec (x). Then use reduction formula (6.2.13) or

formula (6.2.14) to evaluate the given indeﬁnite integral. b

42.

π=4

sec

ðxÞtan

ðxÞdx

43.

π=4

sec

ðxÞtan

ðxÞdx

44.

π=4

sec

ðxÞtan

ðxÞdx

45.

π=3

2secðxÞtan

ðxÞdx

c In each of Exercises 46256, evaluate the given integral by

converting

the integrand to an expression in sines and cosines. b

46.

tanðxÞsec

ðxÞdx

47.

8tanðxÞsec

ðxÞdx

48.

tan

ðx=2Þdx

49.

3tan

ðxÞsecðxÞdx

50.

tanðxÞsinðxÞdx

51.

cotðx=3Þcsc

ðx=3Þdx

52.

4cotðx=2Þcsc

ðx=2Þdx

53.

cot

ðx=3Þcscðx=3Þdx

54.

2sin

ðxÞtanðxÞdx

55.

6cos

ðxÞtan

ðxÞdx

56.

5sin

ðx=3Þcot

ðx=3Þdx

Further Theory and Practice

c In each of Exercises 57261, the denominator of the given

integrand is of the form a 6 b. Multiply numerator and

denominator by a7b to obtain a difference of squares in the

denominator. Then use an appropriate trigonometric identity

before integrating. b

57.

1 2 sinðxÞ

58.

1 1 cosðxÞ

59.

cosðxÞ

1 2 cosðxÞ

60.

secðxÞ2 1

61.

tanðxÞ

secðxÞ1 1

c Identities (6.2.1) and (6.2.2) are the basis of an alternative

method

for calculating the integrals

sin

ðxÞdx and

cos

2‘

ðxÞdx for positive integers k and ‘. The cited identities

yield the equations

sin

ðxÞ5



sin

ðxÞ





1 2 cosð2xÞ



and

cos

2‘

ðxÞ5



cos

ðxÞ



‘



1 1 cosð2xÞ



‘

After the binomials on the right sides have been expanded, the

same manipulation is applied to all even powers of cos(2x).

The process is continued until only odd powers of cos(2x),

cos(4x), . . . remain. The method of Example 5 is then used to

integrate the odd powers of cosine. Use the procedure just

outlined to calculate the integrals in Exercises 62265. b

62.

sin

ðxÞdx

63.

cos

ðxÞdx

64.

sin

ðxÞdx

65.

cos

ðxÞsin

ðxÞdx

c When sin(x)

and cos(x) are both raised to the same positive

power in an integrand, the identity sin(2x) 5 2 sin(x) cos(x)

may be used to simplify the integral. Use this observation as

the basis for calculation of the integrals in Exercises 66269. b

66.

cosðxÞsinðxÞdx

67.

cos

ðxÞsin

ðxÞdx

68.

cos

ðxÞsin

ðxÞdx

69.

cos

ðxÞsin

ðxÞdx

70. Sketch the graphs of y 5 sin

(x)andy 5 cos

(x)for

0 # x # 2π. Use the identity sin

(x) 1 cos

(x) 5 1togivea

geometric proof of the equations in line (6.2.9).

71. By making the substitution u 5 sin(x), show that

sinðxÞcos ð xÞdx 5

sin

ðxÞ1 C:

By making the substitution u 5 cos(x), show that

sinðxÞcosðx Þdx 52

cos

ðxÞ1 C:

By using the formula for sin(2x), show that

sinðxÞcos ð xÞdx 52

cosð2xÞ1 C:

Explain why these answers are not contradictory.

72. Calculate

π=2

4xcos

ðxÞdx:

73. Calculate

π=2

2π=2

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 1 2sinðxÞ

cos

ðxÞdx:

74. Find the area of the region bounded by y 5 sin(x) and

y 5 sin

(x) for 0 # x # π/2.

75. Find the area of the region bounded by y 5 sin

(x) and

y 5 sin

sin

(x) for 0 # x # π/2.

76. Derive reduction formula (6.2.12).

77. Derive reduction formula (6.2.13).

78. Derive reduction formula (6.2.14).

79. Let m and n be integers such that m 6¼2n Prove the

reduction formula

sin

ðxÞcos

ðxÞdx 52

sin

n21

ðxÞcos

m11

ðxÞ

m 1 n

n 2 1

m 1 n

sin

n22

ðxÞcos

ðxÞdx:

6.2 Powers and Products of Trigonometric Functions 487

80. Suppose that j and k are nonnegative integers, not both

zero. Derive the reduction formula

tan

ðxÞsec

2jþ1

ðxÞdx 5

2k þ 2j

tan

2kþ1

ðxÞsec

2j21

ðxÞ

2j 2 1

2k þ 2j

tan

ðxÞsec

2j21

ðxÞdx:

81. Suppose that A and B are constants, not both of which are

0. Notice that

Acosð xÞ1 BsinðxÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 B



cosðxÞξ 1 sinðxÞη



where ξ 5 A=

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 B

and η 5 B=

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 B

: By observing

that (ξ, η) is a point on the unit circle, deduce that AcosðxÞ1

BsinðxÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 B

cosðx 1 φÞ for some φ.Evaluate



AcosðxÞ1BsinðxÞ



for k 5 1andk 5 2.

82. For each natural number n, let

π=2

sin

ðxÞdx:

a. Use formula (6.2.11) to show that

n 2 1

n22

ðn $ 2Þ:

b. By calculating W

and using part a of this exercise,

show that

1  3  5 ð2N 2 1Þ

2  4  6 ð2NÞ



c. By calculating W

and using part a of this exercise,

show that

2N11

2  4  6 ð2NÞ

3  5  7 ð2N 1 1Þ

d. By comparing integrands, deduce that

2N11

# W

2N21

e. Use parts b, c, and d to show that





ð2NÞ

ð2N21Þ



ð2N 1 1Þ

and





ð2NÞ

ð2N21Þ



f. Prove that there is a number θ

in [0, 1] such that





ð2NÞ

ð2N21Þ



2N 1 θ

g. Deduce the following formula for π that John Wallis

published in 1655:

π 5 lim

N-N







ð2NÞ

ð2N21Þ





Note: Wallis’s limit formula is beautiful, but because the

convergence is so slow, it is useless for numerically

approximating π.

Calculator/Computer Exercises

83. Let a be the least positive abscissa of a point of intersection

of the curves y 5 cos

(x)andy 5 sin

(x).Findtheareaof

the region between the two curves for 2a # x # a.

84. The curves y 5 10 sin

(x) 1 2 and y 5 sec

(x) have a point

of intersection with abscissa a in [20.5, 0] and a point of

intersection with abscissa b in [1, 1.5]. Find the area of the

region between the two curves for a # x # b.

85. The curves y 5 sin

(x) cos

(x) and y 5 sin

(x) cos

(x) have

a point of intersection with abscissa b in [0.5, 1]. Find the

area of the region between the two curves for 0 # x # b.

86. The line y 5 0.6x intersects the curve y 5 sin

(x) at the

origin and at two points with positive coordinates. Find

the total area of the regions between the two curves in the

ﬁrst quadrant.

87. Let x

be the least positive value of x such that the tan-

gent line to y 5 sin

(x)at



, sin

)



passes through the

origin. Find the area enclosed by this tangent line and

y 5 sin

(x) for 0 # x # x

6.3 Trigonometric Substitution

In this section, you will learn to treat integrands that involve expressions of the form

1 Bx 1 C; A 6¼ 0:

We have already encountered a few isolated instances of integrals that contain

quadratic expressions. For example, if A 521or1,B 5 0, and C 5 a

. 0, then

1Bx 1C becomes a

2 x

or a

1 x

. Formulas (3.10.5) and (3.10.8) of Section

3.10 in Chapter 3 tell us that

488 Chapter 6 Techniques of Integration

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 x

5 arcsin





1 C ð6:3:1Þ

and

1 x

arctan





1 C: ð6:3:2Þ

As these two examples show, inverse trigonometric functions can appear in the

antiderivatives of functions that involve the expression Ax

1 Bx 1 C.

Trigonometric

Substitution

Suppose that a is a positive constant. Consider the three quadratic polynomials

(a) a

2 x

where 2a # x # a , (b) a

, and (c) x

2 a

where a # jxj. By making an

appropriate substitution in each of these expressions, we may exploit a trigono-

metric identity to reduce these sums to a single square:

a. The expression a

2 x

is simpliﬁed by substituting x 5 a sin(θ)with2π/2 # x # π/2.

The simpliﬁcation is realized as follows:

2 x

5 a

2 a

sin

(θ) 5 a



1 2 sin

(θ)



5 a

cos

(θ).

Notice that the range of θ implies that 0 # cos(θ). Therefore

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 x

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

cos

ðθÞ

5 jacosðθÞj5 acosðθÞ :

b. The expression a

1 x

is simpliﬁed by substituting x 5 a tan(θ) with

2π/2 , x , π/2. The simpliﬁcation is realized as follows:

1 x

5 a

1 a

tan

(θ) 5 a



1 1 tan

(θ)



5 a

sec

(θ)

Notice that the range of θ implies that 0 , sec (θ). Therefore

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 x

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

sec

ðθÞ

5 jasecðθÞj5 a secðθÞ:

c. The expression x

2 a

is simpliﬁed by substituting x 5 a sec(θ). The simpliﬁca-

tion is realized as follows:

2 a

5 a

sec

(θ) 2 a

5 a

(sec

(θ) 2 1) 5 a

tan

(θ).

If a # jxj, then we take θ in the interval [0, π/2). For these values of θ, we have

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 a

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

tan

ðθÞ

5 jatanðθÞj5 atan ðθÞ:

These calculations suggest a new substitution technique. To integrate a function

that contains the expression a

2 x

, we consider the change of variable x 5 a sin(θ),

dx 5 a cos(θ) dθ. Similarly, the expression a

1 x

in an integral suggests the change of

variable x 5 a tan(θ), dx 5 a sec

(θ) dθ. Finally, the expression x

2 a

points to the

substitution x 5 a sec(θ), dx 5 a sec(θ)tan(θ) dθ. These substitutions are examples of

a type of change of variable that is known as the method of inverse (or indirect)

substitution. Here is a summary of the trigonometric substitutions that we will use:

Expression Substitution Result of Substitution After Simpliﬁcation

2 x

x 5 a sin(θ), dx 5 a cos(θ)dθ a



1 2 sin

(θ)



cos

(θ)

1 x

x 5 a tan(θ), dx 5 a sec

(θ)dθ a



1 1 tan

(θ)



sec

(θ)

2 a

x 5 a sec(θ), dx 5 a sec(θ) tan(θ)dθ a



sec

(θ) 2 1



tan

(θ)

Table 1

6.3 Trigonometric Substitution 489

If you already know the fundamental trigonometric identities that are the basis of

these three substitutions, then Table 1 requires no memorization. Instead, work

enough practice problems so that you recognize when a particular trigonometric

substitution is called for.

⁄ EX

AMPLE 1 Calculate

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

dx:

Solution T

he expression 1 2 x

suggests the substitution x 5 1 sin(θ), dx 5 cos(θ) dθ

for 2π/2 , θ , π/2. With this change of variable, the given integral becomes

sin

ðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 sin

ðθÞ

cosðθÞdθ 5

sin

ðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

cos

ðθÞ

cosðθÞdθ 5

sin

ðθÞ

cosðθÞ

cosðθÞdθ 5

sin

ðθÞdθ 5



θ 2 sinðθÞcosðθÞ



1 C:

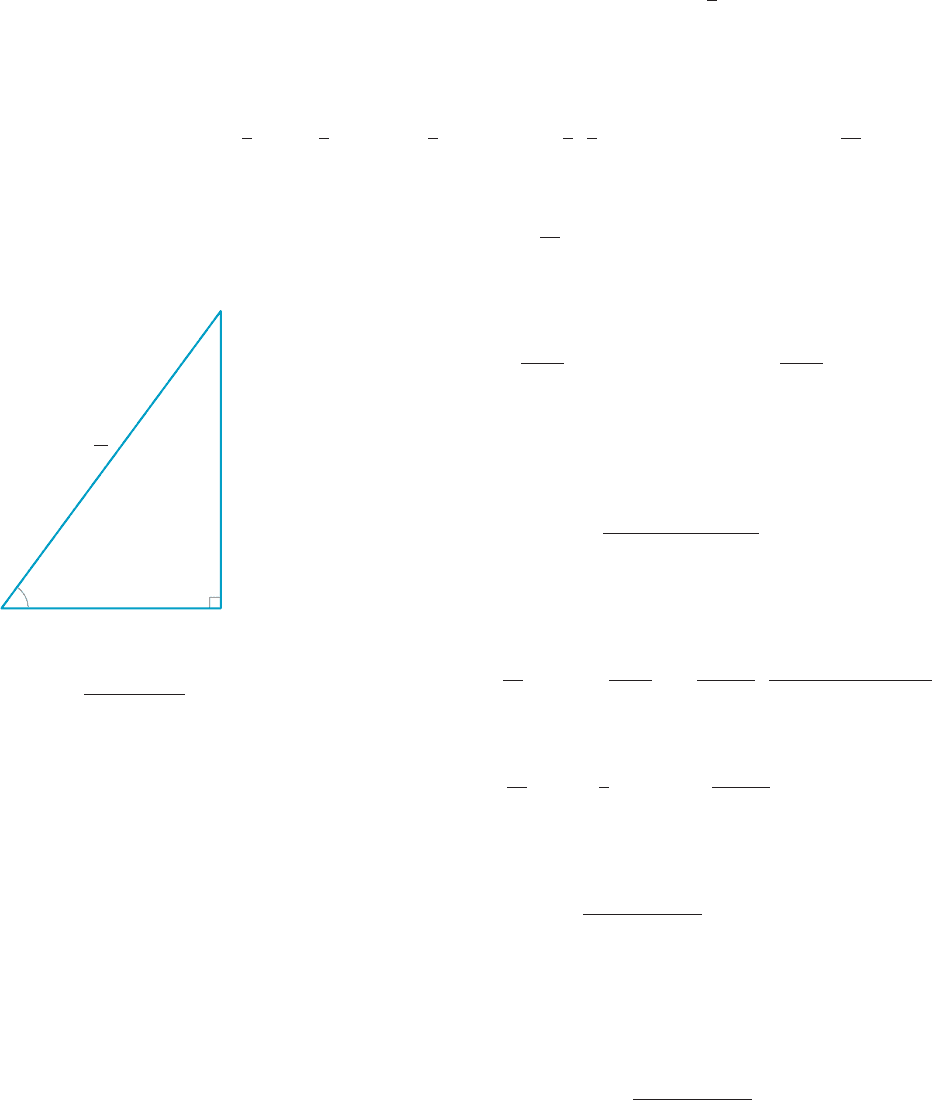
We ﬁnish the calculation by resubstituting. Because x/1 5 sin(θ), we draw a right

triangle with angle θ, opposite side x, and hypotenuse 1. See Figure 1. By Pytha-

goras, the adjacent side b equals

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

. It follows that cosðθÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

=1: We

therefore resubstitute θ 5 arcsin(x ), x 5 sin(θ), and cosðθÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

to obtain

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

dx 5



arcsinðxÞ2 x

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x



1 C: ¥

⁄ EX

AMPLE 2 Calculate

ﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

dx:

Solution Th

e expression 1 1 x

suggests the substitution x 5 1 tan(θ), dx 5 sec

(θ)dθ.

To obtain the limits of integration for θ,wenotethattan(θ) 5 1whenθ 5 π/4 and

tan(θ) 5

ﬃﬃﬃ

when θ 5 π/3 The given integral becomes

ﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

dx 5

π=3

π=4

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 tan

ðθÞ

sec

ðθÞdθ 5

π=3

π=4

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

sec

ðθÞ

sec

ðθÞdθ 5

π=3

π=4

sec

ðθÞdθ:

We can now ﬁnish the calculation by appealing to formula (6.1.8):

ﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

dx 5



secðθÞtanðθÞ1 ln



jsecðθÞ1tanðθÞj







θ5π=3

θ5π=4



ﬃﬃﬃ





2 1

ﬃﬃﬃ

1 1

ﬃﬃﬃ



: ¥

⁄ EX

AMPLE 3 Calculate

ﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 4

dx:

Solution T

he expression x

2 4, when written as x

2 2

, suggests the substitution

x 5 2sec(θ), dx 5 2 sec (θ)tan(θ)dθ. The limit of integration x 5 2

ﬃﬃﬃ

, corresponds

to sec (θ) 5

ﬃﬃﬃ

or θ 5 π/4. The limit of integration x 5 4 corresponds to sec (θ) 5 2, or

θ 5 π/3. Therefore

m Figure 1

5 sinðθÞ.cosðθÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

490 Chapter 6 Techniques of Integration

ﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 4

dx 5

π=3

π=4

2secðθÞtanðθÞ

2secðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

4sec

ðθÞ2 4

dθ 5

π=3

π=4

tanðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

sec

ðθÞ2 1

dθ

π=3

π=4

tanðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

tan

ðθÞ

dθ 5

π=3

π=4

1dθ



π=3

π=4





: ¥

⁄ EX

AMPLE 4 Calculate the integrals

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx and

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx:

Solution Both

integrands involve the expression 9 1 x

, which we treat as 3

1 x

Therefore the substitution x 5 3 tan(θ), dx 5 3 sec

(θ)dθ is indicated. With this

substitution, the ﬁrst integral becomes

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 9tan

ðθÞ

3sec

ðθÞdθ 5

sec

ðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 tan

ðθÞ

dθ 5

sec

ðθÞ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

sec

ðθÞ

dθ

sec

ðθÞ

jsecðθÞj

dθ 5

secðθÞdθ 5 ln



jsecðθÞ1 tanðθÞj



1 C:

We complete the integration by resubstituting. Because x/3 5 tan(θ), we draw a right

triangle with angle θ, opposite side x, adjacent side 3, and hypotenuse H (Figure 2).

By Pythagoras, H equals

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

. It follows that secðθÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

=3. Therefore

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx 5 ln





ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x





1 C 5 ln





x 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x





1 C:

Using the logarithm law ln(u/v) 5 ln( u) 2 ln(v), we can simplify this a bit with

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx 5 ln





x 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x





2 lnð3Þ1 C 5 ln





x 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x





1 C

;

where we have written the (arbitrary) constant C 2 ln(3) as C

. Finally, we observe

that

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

ﬃﬃﬃﬃﬃ

5 jxj. Therefore x 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

. 0, and we may omit the unne-

cessary absolute values from our answer. Writing the arbitrary constant as C again,

we have

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx 5 ln



x 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x



1 C:

It is possible to calculate the second integral,

ðx=

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

Þdx, by making the

same trigonometric substitution, x 5 3 tan(θ). However, we notice that a constant

multiple of the derivative 2x of 9 1 x

is present in the integral. The direct sub-

stitution u 5 9 1 x

, du 5 2xdx is therefore more efﬁcient. With this substitution,

the integral

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx becomes

21=2

du,or

ﬃﬃﬃ

1 C. Therefore

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx 5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

1 C: ¥

m Figure 2

5 tanðθÞ.

secðθÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 x

6.3 Trigonometric Substitution 491

b A LOOK BACK In the method of substitution (Section 5.6 of Chapter 5), we select

an expression that is already present in a given integral and replace that expression

with a new vari able. For example, we treat the integral

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx of Example 4

by making the (direct) substitution u 5 9 1 x

for an expression that is already in

the integral. In the method of inverse (or indirect) substitution, we replace the

original variable of integration with a function that is nowhere to be found in the

original integral. In the integral

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

dx of Example 4, we make the indirect

substitution x 5 3 tan(θ). In this substitution, the term tan(θ) does not have any

apparent connection to the integrand. In theory, the two methods of substitution

are distinct. However, in practice, the two methods are carried out in exactly the

same manner.

General Quadratic

Expressions That

Appear Under a

Radical

Because a general quadratic expression Ax

1 Bx 1 C can be converted (by com-

pleting the square) to a sum or difference of squares, the trigonometric substitu-

tions that we have just learned can be applied to integrals that involve these general

quadratic expressions. Let us see how this works in practice.

⁄ EX

AMPLE 5 Calculate the integral

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 8t 1 25

Solution The

ﬁrst step is to complete the square under the radical sign:

2 8t 1 25 5



2 8t 1 ð8=2 Þ



1 25 2 ð8=2Þ

5 ðt24Þ

1 9:

To evaluate the inte gral

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ðt 2 4Þ

1 9

;

we ﬁrst make the direct substitution x 5 t 2 4, dx 5 dt. This results in the integral

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 9

Here the substitution x 5 3 tan(θ), dx 5 3 sec

(θ) dθ is called for. With that sub-

stitution, we calculated this integral to be lnðx 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

9 1 x

Þ1 C in Example 3.

Resubstituting x 5 t 2 4, we obtain

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 8t 1 25

5 ln



t 2 4 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ðt2 4 Þ

1 9



1 C 5 ln



t 2 4 1

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

2 8t 1 25



1 C: ¥

⁄ EXAMPLE 6 Calculate

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

21 1 8x 2 4x

dx:

Solution First

we complete the square:

21 1 8x 2 4x

5 21 2 4ðx

2 2xÞ5 21 1 4 2 4ðx

2 2x 1 1Þ5 25 2 4ðx 2 1Þ

5 4





2 ðx 2 1Þ



492 Chapter 6 Techniques of Integration

Therefore

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

21 1 8x 2 4x

dx 5 2

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ





2 ðx 2 1Þ

dx:

Next, we make the substitution x 2 1 5 ð5= 2 ÞsinðθÞ; dx 5 ð5=2ÞcosðθÞdθ. The last

integral becomes

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ









sin

ðθÞ



cosðθÞdθ 5 2 



ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 sin

ðθÞ

cosðθÞdθ 5

cos

ðθÞdθ:

The last of these integrals evaluates to



θ 1 sinð θÞ cosðθÞ



1 C ð6:3:3Þ

by equation (6.2.6). The value of the original integral is obtained by resubstituting

for θ in expression (6.3.3). The change of variable x 2 1 5 (5/2) sin(θ) gives us

sinðθÞ5

x 2 1

5=2

and θ 5 arcsin



x 2 1

5=2



: ð6:3:4Þ

To resubstitute for cos(θ) in expression (6.3.3), we refer to the triangle associated

with the change of variable, which is shown in Figure 3. By calculating the length of

the adjacent side, we ﬁnd that

cosðθÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ð5=2Þ

2 ðx21Þ

5=2

: ð6:3:5Þ

Using lines (6.3.4) and (6.3.5) to substitute for θ, sin(θ), and cos(θ) in expression

(6.3.3), we have

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

21 1 8x 2 4x

dx 5



arcsin



x 2 1

5=2



ðx 2 1Þ

ð5=2Þ



ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ð5=2Þ

2 ðx 2 1Þ

ð5=2Þ



1 C:

After a little bit of algebraic simpliﬁcation, this equation becomes

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

21 1 8x 2 4x

dx 5

arcsin



ðx 2 1Þ



ðx 2 1Þ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

21 1 8x 2 4x

1 C: ¥

Quadratic Expressions

Not Under a

Radical Sign

Suppose that P(x) is a polynomial. To calculate

PðxÞ

1 Bx 1 C

dx;

it is best not to attempt trigonometric substitutions immediately. Instead, use the

following three steps:

1. If the degree of P is less than or equal to 1, then proceed directly to Step 2.

Otherwise, divide the denominator into the numerator. This division will result in

QðxÞ1

RðxÞ

1 Bx 1 C

x  1

m Figure 3

ðx 2 1Þ=ð5=2Þ5 sinðθÞ.cosðθÞ5

b=ð5=2Þ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ð5=2Þ

2 ðx21Þ

ð5=2Þ

6.3 Trigonometric Substitution 493

where Q(x) is a polynomial and R(x) has degree less than or equal to 1. The

polynomial Q(x) is easy to integrate. Continue by applying Step 2 to the new

fraction RðxÞ=ðAx

1 Bx 1 CÞ:

2. If the degree of P(x) is 0, or if you have divided in Step 1 and the degree of R(x)is

0, then proceed to Step 3. If P(x)orR(x) has degree 1, then separate the numerator

into a multiple of (2Ax1B)plusaconstantK. Integrate the expression

2Ax 1 B

1 Bx 1 C

by making the substitution u 5 Ax

1Bx1C, du 5 (2Ax1B) dx.

3. Integrate the expression

1 Bx 1 C

by completing the square in the denominator.

The only way to understand these steps is to see them used in practice:

⁄ EX

AMPLE 7 Integrate

2 8x

1 20x 2 5

2 4x 1 8

dx:

Solution The

degree of the numerator is not of 0 or 1, so we start with Step 1. First

we divide:

After division, we obtain

2 8x

1 20x 2 5

2 4x 1 8

5 2x 1

4x 2 5

2 4x 1 8

Therefore

2 8x

1 20x 2 5

2 4x 1 8

dx 5 x

4x 2 5

2 4x 1 8

dx:

Note that the deriv ative of the denominator in the last integrand is 2x 2 4.

Therefore we rewrite the integral on the right side as

2ð2x 2 4Þ1 3

2 4x 1 8

dx 5 2 

ð2x 2 4Þ

2 4x 1 8

dx 1

2 4x 1 8

dx: ð6:3:6Þ

Now we can see the motivat ion for this step more clearly. With the substitution

u 5 x

2 4x 1 8, du 5 2 x 2 4 dx, the ﬁrst integral in (6.3.6) is

5 2  lnðjujÞ1 C 5 2lnðx

2 4x 1 8Þ1 C: ð6:3:7Þ

In the last logarithm, we discard the redundant absolute values because we observe

by completing the square that x

2 4x 1 8 5 (x 2 2)

1 4 is positive .

494 Chapter 6 Techniques of Integration