Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

517.3 Theory of Diffusion in Gases at Low Density

527

The dimensionless quantity a9,,,-the "collisional integral" for diffusion-is a func-

tion of the dimensionless temperature KT/&,,. The parameters

aAB

and

EAB

are those ap-

pearing in the Lennard-Jones potential between one molecule of

and one of

(cf. Eq.

1.4-1 0):

This function In,,,, is given in Table E.2 and Eq. E.2-2. From these results one can com-

pute that

9JAB

increases roughly as the 2.0 power of T at low temperatures and as the 1.65

power of

at very high temperatures; see the

0 curve in Fig. 17.2-1. For rigid

spheres, would be unity at all temperatures and a result analogous to Eq. 17.3-10

would be obtained.

The parameters

o,,

and

EAB

could, in principle, be determined directly from accurate

measurements of

9,,

over a wide range of temperatures. Suitable data are not yet avail-

able for many gas pairs, and one may have to resort to using some other measurable

property, such as the viscosity4 of a binary mixture of A and

In the event that there are

no such data, then we can estimate

a,,

and

E,,

from the following combining rules:5

for nonpolar gas pairs. Use of these combining rules enables us to predict values of

9,,

within about 6%

use of viscosity data on the pure species A and

or within about

10% if the Lennard-Jones parameters for

and

are estimated from boiling point data

by use of Eq. 1.4-12.~

For isotopic pairs, u,,*

a,,

and

EM.

.FA

that is, the intermolecular

force fields for the various pairs A-A*, A"-A*, and A-A are virtually identical, and the

parameters

and may be obtained from viscosity data on pure A. If, in addition, MA

is large, Eq. 17.3-11 simplifies to

The corresponding equation for the rigid-sphere model is given in Eq. 17.3-9.

Comparison of Eq. 17.3-16 with Eq. 1.4-14 shows that the self-diffusivity BAA* and

the viscosity

(or kinematic viscosity

are related as follows for heavy isotopic gas

pairs at low density:

in which

1.1I1,,,* over a wide range of KT/&,, as may be seen in Table E.2. Thus

9AA*

1.32~ for the self-difusivify. The relation between

and the

binary

difusivity '?JAB is

not so simple, because

may vary considerably with the composition. The Schmidt

number Sc

p/p9,,

is in the range from

0.2

to 5.0 for most gas pairs.

Equations 17.3-11, 12, 16, and 17 were derived for monatomic nonpolar gases but

have been found useful for polyatomic nonpolar gases as well. In addition, these equa-

tions may be used to predict QAB for interdiffusion of a polar gas and a nonpolar gas by

using combining laws different7 from those given in Eq. 17.3-14 and 15.

S. Weissman and

Mason,

Chem. Pkys.,

37,1289-1300 (1962);

S. Weissman,

Ckem. Pkys.,

40,

3397-3406 (1964).

Hirschfelder,

Bird, and

Spotz,

Ckem. Revs.,

44,205-231 (1949);

S. Gotoh,

Manner,

P. Sdrensen, and W.

Stewart,

Ckem.

Eng.

Data,

19,169-171 (1974).

Reid,

M. Prausnitz, and

Poling,

The Properties

Gases and Liquids,

4th

edition,

McGraw-Hill, New York

(1987).

Hirschfelder,

Curtiss, and

Bird,

Molecular Theoy

Gases and Liquids,

2nd corrected

printing, Wiley, New York

(1964), #.6b

and p.

1201.

Polar gases and gas mixtures are discussed

Mason and

Monchick,

Chem. Pkys.

36,2746-2757 (1962).

528

Chapter

Diffusivity and the Mechanisms of Mass Transport

Predict the value of

Eb,,

for the system

CO-CO,

296.1K

and

1.0

atm total pressure.

Computation of Mass

SOLUTION

Diffusiviiyfor

From Table

E.l

we obtain the following parameters:

Density Gases

co:

co,:

The mixture parameters are then estimated from Eqs.

17.3-14

and

15:

The dimensionless temperature is then

KT/s~~

(296.1)/(144.6)

2.048. From Table

E.2

can find the collision integral for diffusion,

flgpB

1.067.

Substitution of the preceding values

Eq. 17.3-12

gives

s17.4

THEORY

OF DIFFUSION IN BINARY LIQUIDS

The kinetic theory for diffusion in simple liquids is not as well developed as that for di-

lute gases, and it cannot presently give accurate analytical predictions of diffusivities.'-3

As a result our understanding of liquid diffusion depends primarily on the rather crude

hydrodynamic and activated-state models. These in turn have spawned

number of em-

pirical correlations, which provide the best available means for prediction. These corre-

lations permit estimation of diffusivities in terms of more easily measured properties

such as viscosity and molar volume.

The

hydrodynamic

theory

takes as its starting point the Nernst-Einstein equation:

which states that the diffusivity of a single particle or solute molecule

through a sta-

tionary medium

is given by

KT(~A/FA)

(17.4-1)

in which uA/FA is the "mobility" of a particle

(that is, the steady-state velocity attained

by the particle under the action of a unit force). The origin of

Eq.

17.4-1

is discussed in

$17.5

in connection with the Brownian motion of colloidal suspensions. If the shape and

size of

are known, the mobility can be calculated by the solution of the creeping-flow

equation of motion5 (Eq. 3.5-8). Thus,

is spherical and if one takes into account the

possibility of "slip" at the fluid-solid interface, one obtains6

Bearman and

Kirkwood,].

Chem. Phys.,

28,136-145 (1958).

Bearman,

Phys. Chem.,

65,1961-1968 (1961).

Curtiss and

Bird,

Chem. Phys.,

111,10362-10370 (1999).

See 517.7 and

A. Moelwyn-Hughes,

Physical Chemistry,

2nd edition, corrected printing, Maanillan,

New York (1964), pp. 62-74. See also

Silbey and

A. Alberty,

Physical Chemisty,

3rd edtion, Wiley,

New York (2001), 520.2. Apparently the Nemst-Einstein equation cannot be generalized to polymeric fluids

with appreciable velocity gradients, as has been noted by

&linger,

AKhE Journal,

35,279-286 (1989).

S. Kim and S.

Karrila,

Microhydrodynamics: Principles and Selected Applications,

Butterworth-

Heinemann, Boston (1991

Lamb,

Hydrodynamics,

6th edition, Cambridge University Press (1932), reprinted (1997), 5337.

7.4

Theory of Diffusion in Binary Liquids

529

in which

is the viscosity of the pure solvent, RA is the radius of the solute particle, and

PA,

is the "coefficient of sliding friction" (formally the same as the

p/c

of problem 2B.9).

The limiting cases of

DAB

and

DAB

are of particular interest:

fiAB

(no-slip condition)

In this case Eq. 17.4-2 becomes Stokes' law (Eq. 2.6-15) and Eq. 17.4-1 becomes

which is usually called the Stokes-Einstein equation. This equation applies well to the dif-

fusion of very large spherical molecules in solvents of low molecular weight7 and to sus-

pended particles. Analogous expressions developed for nonspherical particles have been

used for estimating the shapes of protein

molecule^.^,'

fiAB

(complete slip condition)

In this case Eq. 17.4-1 leads to (see Eq. 4B.3-4)

If the molecules

and

are identical (that is, for self-diffusion) and if they can be as-

sumed to form a cubic lattice with the adjacent molecules just touching, then 2RA

and

Equation 17.4-5 has been found'' to agree with self-diffusion data for a number of liq-

uids, including polar and associated substances, liquid metals, and molten sulfur, to

within about 12%. The hydrodynamic model has proven less useful for binary diffusion

(that is, for

not identical to

although the predicted temperature and viscosity depen-

dences are approximately correct.

Keep in mind that the above formulas apply only to dilute solutions of

Some

attempts have been made, however, to extend the hydrodynamic model to solutions of

finite concentrations."

The Eyring activated-state theory attempts to explain transport behavior via a quasi-

crystalline model of the liquid state.12 It is assumed in this theory that there is some uni-

molecular rate process in terms of which diffusion can be described, and it is further

assumed that in this process there is some configuration that can be identified as the "ac-

tivated state." The Eyring theory of reaction rates is applied to this elementary process in

a manner analogous to that described in s1.5 for estimation of liquid viscosity.

modifi-

Polson,

Pkys. Colloid Ckem.,

54,649-652 (1950).

Tyrrell,

Diffusion and Heat Flow

Liquids,

Butterworths, London (1961), Chapter 6.

Creeping motion around finite bodies in a fluid of infinite extent has been reviewed by

Happel

and H. Brenner,

Low Reynolds Number Hydrodynamics,

Prentice-Hall, Englewood Cliffs,

N.J.

(1965); see

also

Kim and

Karrila,

Microkydrodynarnics: Principles and Selected Applications,

Butterworth-

Heinemann, Boston (1991).

Youngren and

Acrivos,

Ckem. Pkys.

63,3846-3848 (1975) have

calculated the rotational friction coefficient for benzene, supporting the validity of the no-slip condition

at molecular dimensions.

Li and

Chang,

Ckm. Phys.,

23,518-520 (1955).

C. W.

Pyun

and M. Fixman,

Clzem. Pkys.,

41,937-944 (1964).

Glasstone, K.

Laidler, and

Eyring,

Tkeoy

of Rate Processes,

McGraw-Hill, New York (1941),

Chapter

IX.

530

Chapter 17 Diffusivity and the Mechanisms of Mass Transport

EXAMPLE

17.4-1

Estimation of Liquid

Diffusivity

cation of the original Eyring model by Ree, Eyring, and

coworker^'^

yields an expression

similar to Eq. 17.4-5 for traces of

in solvent

Here

is a "packing parameter," which in the theory represents the number of nearest

neighbors of a given solvent molecule. For the special case of self-diffusion,

is found to

be very close to

27r,

so that Eqs. 17.4-5 and

are in good agreement despite the difference

between the models from which they were developed.

The Eyring theory is based on an oversimplified model of the liquid state, and con-

sequently the conditions required for its validity are not clear. However, Bearman has

shown2 that the Eyring model gives results consistent with statistical mechanics for "reg-

ular solutions," that is, for mixtures of molecules that have similar size, shape, and inter-

molecular forces. For this limiting situation, Bearman also obtains an expression for the

concentration dependence of the diffusivity,

in which

9,,

and

are the diffusivity and viscosity of the mixture at the composition

XA,

and

is the thermodynamic activity of species

For regular solutions, the partial

molar volumes,

and

V,,

are equal to the molar volumes of the pure components.

Bearman suggests on the basis of his analysis that Eq. 17.4-7 should be limited to regular

solutions, and it has in fact been found to apply well only to nearly ideal solutions.

Because of the unsatisfactory nature of the theory for diffusion in liquids, it is neces-

sary to rely on

empirical

expressions. For example, the Wilke-Chang equation14 gives the

diffusivity for small concentrations of

Here

is the molar volume of the solute

in cm3/g-mole as liquid at its normal boiling

point,

is the viscosity of the solution in centipoises,

t+!~~

is an llassociation parameter"

for the solvent, and

is the absolute temperature in

Recommended values of

are:

2.6

for water; 1.9 for methanol;

1.0

for benzene, ether, heptane, and other unassociated

solvents. Equation 17.4-8 is good only for dilute solutions of nondissociating solutes. For

such solutions, it is usually good within

210%.

Other empiricisms, along with their relative merits, have been summarized by Reid,

Prausnitz, and Poling.I5

Estimate

9,,

for a dilute solution of

TNT

(2,4,6-trinitrotoluene) in benzene

15°C.

SOLUTION

Use the equation of Wilke and Chang, taking TNT as component

and benzene as compo-

nent

The required data are

0.705~~ (the viscosity for pure benzene)

140 cm3/g-mole (for TNT)

Eyring,

Henderson,

Stover,

and

Eyring,

Statistical Mechanics and Dynamics,

Wiley,

New

York

(1964), 516.8.

'v.

Wilke,

Chem.

Eng.

Pvog.,

45,218-224 (1949);

Wilke

and

P. Chang,

AIChE

Journal,

264-270

(1955).

Reid,

Prausnitz,

and

Poling,

The Properties

Gases

find

Liquids,

4th

edition,

McGraw-Hill,

New

York

(1987), Chapter 11.

517.5

Theory of Diffusion in Colloidal Suspensions

531

1.0

(for benzene)

78.11

(for benzene)

Substitution into

Eq.

17.4-8

gives

This result compares well with the measured value of

1.39

cm2/s.

517.5

THEORY

DIFFUSION IN COLLOIDAL SUSPENSIONS~~~

Next we turn to the movement of small colloidal particles in a liquid. Specifically we

consider a finely divided, dilute suspension of spherical particles of material

sta-

tionary liquid

When the spheres of

are sufficiently small (but still large with respect

to the molecules of the suspending medium), the collisions between the spheres and the

molecules of

will result in an erratic motion of the spheres. This random motion is re-

ferred to as Brownian rnoti~n.~

The movement of each sphere can be described by an equation of motion, called the

Langevin

equation:

which u, is the instantaneous velocity of the sphere of mass

The term -luA gives

the Stokes' law drag force:

6rpBR,

being the "friction coefficient." Finally F(t) is the

rapidly oscillating, irregular Brownian motion force. Equation 17.5-1 cannot be "solved"

in the usual sense, since it contains the randomly fluctuating force F(t). Equations such

as Eq. 17.5-1 are called "stochastic differential equations."

If it is assumed that

(i)

F(t) is independent of

and that (ii) the variations in F(t) are

much more rapid than those of u,, then it is possible to extract from Eq. 17.5-1 the proba-

bility W(uA,t;uA0)duA that at time t the particle will have a velocity in the range of uA to

du,. Physical reasoning requires that the probability density W(U~,~;U~~) approach a

Maxwellian (equilibrium) distribution as t

Here,

is the temperature of the fluid in which the particles are suspended.

A. Einstein,

Ann. d. Phys,

17,549-560 (1905), 19,371-381 (1906);

Investigations on the Theory of the

Brownian Movement,

Dover, New York (1956).

S. Chandrasekhar,

Rev. Mod. Phys.,

15,l-89 (1943).

Russel, D. A. Saville, and

Schowalter,

Colloidal Dispersions,

Cambridge University Press

(1989);

Ottinger,

Stochastic Processes in Polymeric Fluids,

Springer, Berlin (1996).

Named after the botanist

Brown,

Phil. Mag.

(4), p. 161 (1828);

Ann. d. Phys.

Chem.,

14,294-313

(1828). Actually the phenomenon had been discovered and reported earlier

1789 by Jan Ingenhousz

(1730-1799) in the Netherlands.

%s can

seen from Example 4.2-1, Stokes' law is valid only for the steady, unidirectional motion

of a sphere through a fluid. For a sphere moving in

arbitrary manner, there are, in addition to the

Stokes' contribution, an inertial term and a memory-integral term (the Basset force). See A.

Basset,

Phil. Trans.,

179,43-63 (1887);

Lamb,

Hydrodynamics,

6th edition, Cambridge University Press (1932),

reprinted (1997),

644;

Villat and

Kravtchenko,

Lecons sur les Fluides Visqueux,

Gauthier-Villars,

Paris (1943),

213, Eq.

(62);

L. Landau and

Lifshitz,

Fluid Mechanics,

2nd edition, Pergamon, New

York (1987), p. 94. In applying the Langevin equation to polymer kinetic theory, the role of the Basset

force has been investigated by

D. Schieber,

Chem. Phys.,

94,7526-7533 (1991).

532

Chapter

Diffusivity and the Mechanisms of Mass Transport

Another quantity of interest that can be obtained from the Langevin equation is the

probability, W(r,t;ro,uAo)dr, that at time

the particle will have a position in the range r to

dr if its initial position and velocity were ro and

UAO.

For long times, specifically t

m/{,

this probability is given by

However, this expression turns out to have just the same form as the solution of Fick's

second law of diffusion (see Eq. 19.1-18 and Problem

20B.5)

for the diffusion from

point source. One simply has to identify

with the concentration cA, and KT/~ with

B,,.

In this way Einstein (see Ref. 1 on page 531) arrived at the following expression for the

diffusivity of a dilute suspension of spherical colloid particles:

Thus,

is related to the temperature and the friction coefficient

(the reciprocal of the

friction coefficient is called the "mobility"). Equation 17.5-4 was already given in

Eq.

17.4-3

for the interdiffusion of liquids.

s17.6

THEORY OF DIFFUSION OF POLYMERS

For a dilute solution of a polymer

in a low-molecular-weight solvent

there is a de-

tailed theory,' in which the polymer molecules are modeled as bead-spring chains (see

Fig. 8.6-2). Each chain is a linear arrangement of N beads and

1 Hookean springs.

The beads are characterized by a friction coefficient

which describes the Stokes' law

resistance to the bead motion through the solvent. The model further takes into account

the fact that, as a bead moves around, it disturbs the solvent in the neighborhood of all

the other beads; this is referred to as hydrodynamic interaction. The theory ultimately

predicts that the diffusivity should be proportional to

N-I'2

for large

Since the num-

ber of beads is proportional to the polymer molecular weight M, the following result is

obtained:

The inverse square-root dependence is rather well borne out by experiment.' If hydrody-

namic interaction among beads were not included, then one would predict

%,,

1 /M.

The theory of self-diffusion in an undiluted polymer has been studied from several

points of

vie^.^,^

These theories, which are rather crude, lead to the result that

G. Kirkwood,

Macromolecules,

Gordon and Breach, New York (1967),

pp.

13,41,76-77,95,

101-102. The original Kirkwood theory has been reexamined and slightly improved by

&tinger,

Chem. Phys.,

87,3156-3165 (1987).

B. Bird,

Curtiss,

C. Armstrong, and

Hassager,

Dynamics of Polymeuic Liquids, Vol.

Kinetic Theory,

2nd edition, Wiley, New York (19871, pp. 174-175.

P.-G.

de Gennes and

Lbger,

Ann.

Rev. Phys. Chem.,

49-61

(1982);

P.-G.

de Gennes,

Physics Today,

36,3539 (1983). De Gennes introduced the notion of

reptation,

according to which the polymer molecules

move back and forth along their backbones in a snake-like Brownian motion.

B. Bird,

Curtiss,

Armstrong,

and

Hassager,

Dynamics of Polymeric Liquids, Vol.

Kinetic Theory,

2nd edition, Wiley, New York (1987),

pp.

326-327;

Curtiss and

Bird,

Puoc. Nat.

Acad. Sci.,

93,7440-7445 (1996).

s17.7

Mass and Molar Transport

Convection

533

Experimental data agree more or less with this result," but the exponent on the molecular

weight may be as great as

for some polymers.

Although a very general theory for diffusion of polymers has been de~eloped,~ not

very much has been done with it. So far it has been used to show that, in flowing dilute

solutions of flowing polymers, the diffusivity tensor (see Eq. 17.1-1

becomes anisotropic

and dependent on the velocity gradients. It has also been shown how to generalize the

Maxwell-Stefan equations (see 517.9 and s24.1) for multicomponent polymeric liquids.

Further advances in this subject can be expected through use of molecular

simulation^.^

517.7

MASS

AND MOLAR TRANSPORT

CONVECTION

In 517.1, the discussion of Fick's (first) law of diffusion was given in terms of

mass

units:

mass concentration, mass flux, and the mass average velocity. In this section we extend the

previous discussion to include molar units. Thus most of this section deals with questions

of notation and definitions. One might reasonably wonder whether or not this dual set of

notation is really necessary. Unfortunately, it really is. When chemical reactions are in-

volved, molar units are usually preferred. When the diffusion equations are solved to-

gether with the equation of motion, mass units are usually preferable. Therefore it is

necessary to acquire familiarity with both. In this section we also introduce the concept of

the convective flux of mass or moles.

Mass and Molar Concentrations

Earlier we defined the mass concentration p, as the mass of species

per unit volume of

solution. Now we define the molar concentration c,

p,/M, as the number of moles of

per unit volume of solution.

Similarly, in addition to the mass fraction

pJp, we will use the mole fraction

c,/c.

Here p

Zap,

is the total mass of all species per unit volume of solution, and c

Z,c,

is the total number of moles of all species per unit volume of solution. By the word

"solution" we mean a one-phase gaseous, liquid, or solid mixture. In Table 17.7-1 we

summarize these concentration units and their interrelation for multicomponent systems.

It is necessary to emphasize that p, is the mass concentration of species

in a mix-

ture. We use the notation

p'"i

for the density of pure species

when the need arises.

Mass Average and Molar Average Velocity

In a diffusing mixture, the various chemical species are moving at different velocities. By v,,

the "velocity of species

a,"

we do not mean the velocity of an individual molecule of species

Rather, we mean the average of all the velocities of molecules of species

within a small

volume. Then, for a mixture of

species, the local mass average velocity v is defined as

P. F.

Green, in

Diffusion in Polymers

(P.

Neogi, ed.), Dekker, New York

(1996),

Chapter

According to

~odge,

Phys.

Rev. Letters,

86,3218-3221 (1999),

measurements on undiluted polymers

show that the exponent on the molecular weight should be about

2.3.

". F.

Curtiss and

Bird,

Adv.

Polym.

Sci., 125,l-101 (1996)

and

Chem.

Phys.,

111,10362-10370

(1999).

N. Theodorou,

Diffusion in Polymers

(P.

Neogi,

ed.),

Dekker, New York

(19961,

Chapter

534

Chapter

Diffusivity and the Mechanisms of Mass Transport

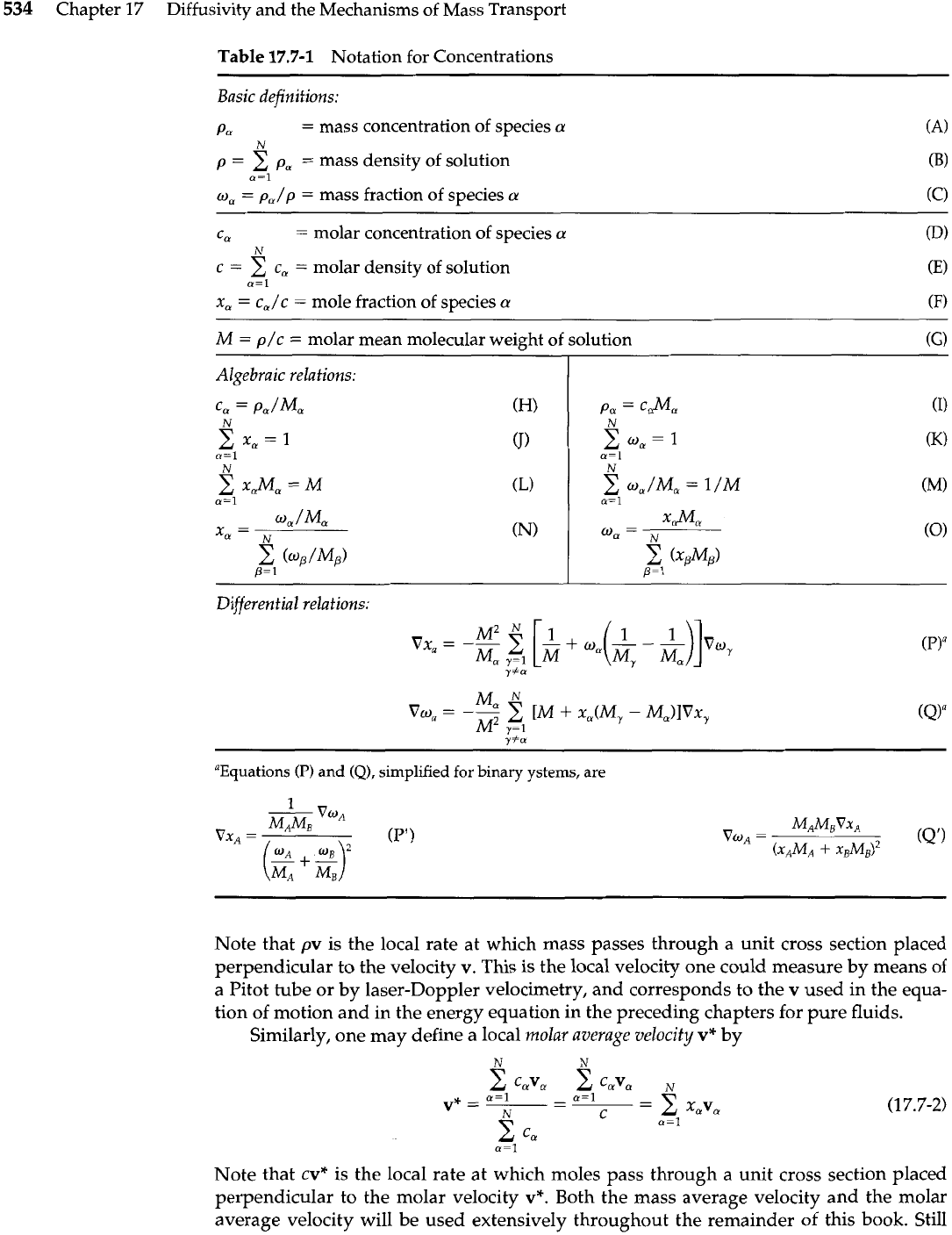
Table

17.7-1

Notation for Concentrations

Basic definitions:

mass concentration of species

(A)

mass density of solution

a=l

p,/p

mass fraction of species

(C)

molar concentration of species

molar density of solution

a=l

c,/c

mole fraction of species

p/c

molar mean molecular weight of solution

(GI

Algebraic relations:

Differential relations:

"Equations

(P)

and

(Q),

simplified for binary ystems, are

Note that

is the local rate at which mass passes through a unit cross section placed

perpendicular to the velocity

This is the local velocity one could measure by means

a Pitot tube or by laser-Doppler velocimetry, and corresponds to the

used in the equa-

tion of motion and in the energy equation in the preceding chapters for pure fluids.

Similarly, one may define a local

molar average velocity

Note that

cv*

is the local rate at which moles pass through

unit cross section placed

perpendicular to the molar velocity

v*.

Both the mass average velocity and the molar

average velocity

will

be used extensively throughout the remainder of this book. Still

g17.7 Mass and Molar Transport by Convection

535

Table

17.7-2

Notation for Velocities in Multicomponent Systems

Basic

definitions:

velocity of species

with respect to fixed coordinates

(A)

O,V,

mass average velocity

a=l

X,V,

molar average velocity

a=l

(C)

diffusion velocity of species

with respect to the mass average

velocity

(D)

diffusion velocity of species

with respect to the molar average

velocity

(E)

Additional relations:

other average velocities are sometimes used, such as the

volume average velocity

(see

Problem 17C.1). In Table 17.7-2 we give a summary of the various relations among

these velocities.

Molecular Mass and Molar Fluxes

In 517.1 we defined the molecular mass flux of

as the flow of mass of

through a unit

area per unit time:

p,(v,

v).

That is, we include only the velocity of species

rela-

tive to the mass average velocity

Similarly, we define the molecular molar flux of

species

as the number of moles of

flowing through a unit area per unit time:

cA(vA

vr).

Here we include only the velocity of species

relative to the molar average

velocity

v*.

Then in 517.1 we presented Fick's (first) law of diffusion, which describes how the

mass of species

in a binary mixture is transported by means of molecular motions.

This law can also

expressed in molar units. Hence we have the pair of relations for

bi-

nary

systems:

The differences

and

are sometimes referred to as

diffusion velocities.

Equa-

tion 17.7-4 can be derived from

Eq.

17.7-3 by using some of the relations in Tables 17.7-1

and

Convective Mass and Molar Fluxes

In addition to transport by molecular motion, mass may also be transported

the bulk

motion of the fluid. In Fig. 9.7-1 we show three mutually perpendicular planes of area

point

where the fluid

mass average velocity

The volume rate of flow across the

plane perpendicular to the surface element

perpendicular to the x-axis is

v,dS.

The

rate at which mass of species

is being swept across the same surface element is then

p,v,dS.

can write similar expressions for the mass flows of species

across the sur-

face elements perpendicular to the

and z-axes as

p,v,dS

and

p,v,dS,

respectively. If we

536

Chapter

Diffusivity and the Mechanisms of Mass Transport

now multiply each of these expressions by the corresponding unit vector, add them, and

divide by dS, we get

as the convective mass flux vector, which has units of kg/m2

If one goes back and repeats the story of the preceding paragraph, but using every-

where molar units and the molar average velocity v*, then we get

as the convective molar flux vector, which has units of kg-mole/m2 s.

To get the convective mass and molar fluxes across a unit surface whose normal unit

vector is n, we form the dot products (n

p,v) and (n

c,v*), respectively.

517.8

SUMMARY OF MASS AND MOLAR FLUXES

In Chapters 1 and

we introduced the combined momentum flux tensor

and the com-

bined energy flux vector e, which we found useful in setting up the shell balances and

equations of change. We give the corresponding definitions here for the mass and molar

flux vectors. We add together the molecular mass flux vector and the convective mass

flux vector to get the combined mass flux vector, and similarly for the combined molar

flux

vector:

Com bined mass flux:

Combined molar flux:

In the first three lines of Table 17.8-1 we summarize the definitions of the mass and

molar fluxes discussed so far.

the shaded squares we also give the definitions of the

fluxes

(mass flux with respect to the molar average velocity) and

(molar flux with re-

spect to mass average velocity). These "hybrid" fluxes should normally not be used.

In the remainder of Table 17.8-1 we give a summary of other useful relations, such

as the sums of the fluxes and the interrelations among the fluxes. By using Eqs.

and

(M)

we can rewrite Eqs. 17.8-1 and

When simplified for binary systems, these relations can be combined with Eqs. 17.7-3

and 17.7-4, to get Eqs.

(C)

and

(D)

of Table 17.8-2, which are equivalent forms of Fick's

(first) law. The forms given in Eqs.

(E)

and (F) of Table 17.8-2, in terms of the relative ve-

locities of the species, are interesting because they involve neither v nor vr.

In Chapter

we will write Fick's law exclusively in the form of Eq.

(D)

of Table

17.8-2. It is this form that has generally been used in chemical engineering. In many

problems something is known about the relation between

and

from the stoi-

chiometry or from boundary conditions. Therefore

can be eliminated from Eq.

(D),

giving

direct relation between

and VxA for the particular problem.

In s1.7 we pointed out that the total molecular momentum flux through a surface of

orientation n is the vector In

m].

In 59.7 we mentioned the analogous quantity for the mol-

ecular heat flux-namely, the scalar (n

q).

The analogous mass transport quantities are

the scalars (n

j,)

and (n

J:),

which give the total mass and molar fluxes through

surface

of orientation

Similarly, for the combined fluxes through a surface of orientation n, we

have for momentum [n

+I,

for energy (n e), and for species

n,) and (n

N,).